Bhagya

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 6 Determinants Ex 6.2 Questions and Answers.

Maharashtra State Board 11th Commerce Maths Solutions Chapter 6 Determinants Ex 6.2

Question 1.
Without expanding, evaluate the following determinants.
(i) \(\left|\begin{array}{lll}
1 & a & b+c \\
1 & b & c+a \\
1 & c & a+b
\end{array}\right|\)
Solution:

(ii) \(\left|\begin{array}{ccc}
2 & 3 & 4 \\
5 & 6 & 8 \\
6 x & 9 x & 12 x
\end{array}\right|\)
Solution:

(iii) \(\left|\begin{array}{lll}
2 & 7 & 65 \\
3 & 8 & 75 \\
5 & 9 & 86
\end{array}\right|\)
Solution:

Question 2.
Using properties of determinants, show that \(\left|\begin{array}{ccc}
a+b & a & b \\
a & a+c & c \\
b & c & b+c
\end{array}\right|\) = 4abc
Solution:

Question 3.
Solve the following equation.
\(\left|\begin{array}{ccc}
x+2 & x+6 & x-1 \\
x+6 & x-1 & x+2 \\
x-1 & x+2 & x+6
\end{array}\right|=0\)
Solution:
\(\left|\begin{array}{ccc}
x+2 & x+6 & x-1 \\
x+6 & x-1 & x+2 \\
x-1 & x+2 & x+6
\end{array}\right|=0\)
Applying R₂ → R₂ – R₁ and R₃ → R₃ – R₁, we get
\(\left|\begin{array}{ccc}
x+2 & x+6 & x-1 \\
4 & -7 & 3 \\
-3 & -4 & 7
\end{array}\right|=0\)
∴ (x + 2)(-49 + 12) – (x + 6)(28 + 9) + (x – 1)(-16 – 21) = 0
∴ (x + 2) (-37) – (x + 6) (37) + (x – 1) (-37) = 0
∴ -37(x + 2 + x + 6 + x – 1) = 0
∴ 3x + 7 = 0
∴ x = \(\frac{-7}{3}\)

Question 4.
If \(\left|\begin{array}{lll}
4+x & 4-x & 4-x \\
4-x & 4+x & 4-x \\
4-x & 4-x & 4+x
\end{array}\right|=0\), then find the values of x.
Solution:

∴ (12 – x)[1(4x² – 0) – (4 – x)(0 – 0) + (4 – x)(0 – 0)] = 0
∴ (12 – x)(4x²) = 0
∴ x²(12 – x) = 0
∴ x = 0 or 12 – x = 0
∴ x = 0 or x = 12

Question 5.
Without expanding determinants, show that
\(\left|\begin{array}{ccc}
1 & 3 & 6 \\
6 & 1 & 4 \\
3 & 7 & 12
\end{array}\right|+4\left|\begin{array}{lll}
2 & 3 & 3 \\
2 & 1 & 2 \\
1 & 7 & 6
\end{array}\right|=10\left|\begin{array}{lll}
1 & 2 & 1 \\
3 & 1 & 7 \\
3 & 2 & 6
\end{array}\right|\)
Solution:

Question 6.
Without expanding determinants, find the value of
(i) \(\left|\begin{array}{lll}
10 & 57 & 107 \\
12 & 64 & 124 \\
15 & 78 & 153
\end{array}\right|\)
Solution:

(ii) \(\left|\begin{array}{lll}
2014 & 2017 & 1 \\
2020 & 2023 & 1 \\
2023 & 2026 & 1
\end{array}\right|\)
Solution:

Question 7.
Without expanding determinants, prove that
(i) \(\left|\begin{array}{lll}
a_{1} & b_{1} & c_{1} \\
a_{2} & b_{2} & c_{2} \\
a_{3} & b_{3} & c_{3}
\end{array}\right|=\left|\begin{array}{lll}
b_{1} & c_{1} & a_{1} \\
b_{2} & c_{2} & a_{2} \\
b_{3} & c_{3} & a_{3}
\end{array}\right|=\left|\begin{array}{lll}
c_{1} & a_{1} & b_{1} \\
c_{2} & a_{2} & b_{2} \\
c_{3} & a_{3} & b_{3}
\end{array}\right|\)
Solution:

(ii) \(\left|\begin{array}{lll}
1 & y z & y+z \\
1 & z x & z+x \\
1 & x y & x+y
\end{array}\right|=\left|\begin{array}{lll}
1 & x & x^{2} \\
1 & y & y^{2} \\
1 & z & z^{2}
\end{array}\right|\)
Solution:

In 1st determinant, taking (x + y + z) common from C₃ and in 2nd determinant, taking \(\frac{1}{x}, \frac{1}{y}, \frac{1}{z}\) common from R₁, R₂, R₃ respectively, we get

Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 6 Determinants Ex 6.1 Questions and Answers.

Maharashtra State Board 11th Commerce Maths Solutions Chapter 6 Determinants Ex 6.1

Question 1.
Evaluate the following determinants:


Solution:
(i) \(\left|\begin{array}{cc}
4 & 7 \\
-7 & 0
\end{array}\right|\)
= 4(0) – (-7)(7)
= 0 + 49
= 49

= 3(0 – 63) – 5(0 – 27) + 2(7 – 24)
= 3(-63) + 5 (-27) + 2(-17)
= – 189 – 135 – 34
= -358

= 1(2 – 10) – i(-i – 15) + 3(-2i – 6)
= -8 + i² + 15i – 6i – 18
= i² – 26 + 9i
= -1 – 26 + 9i …[∵ i² = -1]
= -27 + 9i

= 5(32 – 16) – 5(40 – 20) + 5(20 – 20)
= 5(16) – 5(20) + 5(0)
= 80 – 100
= -20

(v) \(\left|\begin{array}{cc}
2 \mathrm{i} & 3 \\
4 & -\mathrm{i}
\end{array}\right|\)
= 2i(-i) – 3(4)
= -2i² – 12
= -2(-1) – 12 …[∵ i² = -1]
= 2 – 12
= -10

= 3(1 + 6) + 4(1 + 4) + 5(3 – 2)
= 3(7)+ 4(5) + 5(1)
= 21 + 20 + 5
= 46

= a(bc – f²) – h(hc – gf) + g(hf – gb)
= abc – af² – h²c + fgh + fgh – g²b
= abc + 2fgh – af² – bg² – ch²

= 0 – a(0 + bc) – b(-ac – 0)
= -a(bc) – b(-ac)
= -abc + abc
= 0

Question 2.
Find the value(s) of x, if

Solution:
(i) \(\left|\begin{array}{ll}
2 & 3 \\
4 & 5
\end{array}\right|=\left|\begin{array}{cc}
x & 3 \\
2 x & 5
\end{array}\right|\)
∴ 10 – 12 = 5x – 6x
∴ -2 = -x
∴ x = 2

Check:
We can check if our answer is right or wrong.
In order to do so, substitute x = 2 in the given determinant.
For x = 2,
L.H.S. = \(\left|\begin{array}{ll}
2 & 3 \\
4 & 5
\end{array}\right|\)
= 10 – 12
= -2
R.H.S. =\(\left|\begin{array}{cc}
x & 3 \\
2 x & 5
\end{array}\right|\)
= \(\left|\begin{array}{ll}
2 & 3 \\
4 & 5
\end{array}\right|\)
= 10 – 12
= -2
Thus, our answer is correct.

∴ 2(9 – 20) – 1 (-3 – 0) + (x + 1) (5 – 0) = 0
∴ 2(-11) – 1(-3) + (x + 1)(5) = 0
∴ -22 + 3 + 5x + 5 = 0
∴ 5x = 14
∴ x = \(\frac{14}{5}\)

∴ (x – 1)[(x – 2)(x – 3) – 0] – x(0 – 0) + (x – 2)(0 – 0) = 0
∴ (x – 1)(x – 2)(x – 3) = 0
∴ x – 1 = 0 or x – 2 = 0 or x – 3 = 0
∴ x = 1 or x = 2 or x = 3

Question 3.
Solve the following equations.

Solution:

∴ x(x² – 4) – 2(2x – 4) + 2(4 – 2x) = 0
∴ x(x² – 4) – 2(2x – 4) – 2(2x – 4) = 0
∴ x(x + 2)(x – 2) – 4(2x – 4) = 0
∴ x(x + 2)(x – 2) – 8(x – 2) = 0
∴ (x – 2)[x(x + 2) – 8] = 0
∴ (x – 2)(x² + 2x – 8) = 0
∴ (x – 2)(x² + 4x – 2x – 8) = 0
∴ (x – 2)(x + 4)(x – 2) = 0
∴ (x – 2)² (x + 4) = 0
∴ (x – 2)² = 0 or x + 4 = 0
∴ x – 2 = 0 or x = -4
∴ x = 2 or x = -4

∴ 1(-10x² – 10x) – 4(5x² – 5) + 20(2x + 2) = 0
∴ -10x² – 10x – 20x² + 20 + 40x + 40 = 0
∴ -30x² + 30x + 60 = 0
∴ x² – x – 2 = 0 …..[Dividing throughout by (-30)]
∴ x² – 2x + x – 2 = 0
∴ (x – 2)(x + 1) = 0
∴ x – 2 = 0 or x + 1 = 0 x = 2 or x = -1

Question 4.
Find the value of x, if
\(\left|\begin{array}{ccc}
x & -1 & 2 \\
2 x & 1 & -3 \\
3 & -4 & 5
\end{array}\right|\) = 29
Solution:

∴ x(5 – 12) + 1(10x + 9) + 2(-8x – 3) = 29
∴ -7x + 10x + 9 – 16x – 6 = 29
∴ -13x + 3 = 29
∴ -13x = 26
∴ x = -2

Question 5.
Find x and y if \(\left|\begin{array}{ccc}
4 i & i^{3} & 2 i \\
1 & 3 i^{2} & 4 \\
5 & -3 & i
\end{array}\right|\) = x + iy, where i = √-1.
Solution:

= 4i(-3i + 12) + i(i – 20) + 2i(-3 + 15)
= -12i² + 48i + i² – 20i + 24i
= -11i² + 52i
= -11(-1) + 52i …..[∵ i² = -1]
= 11 + 52i
Comparing with x + iy, we get
x = 11, y = 52

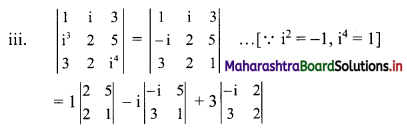
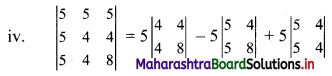
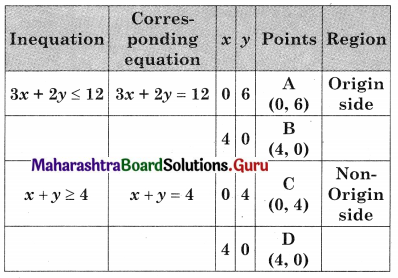
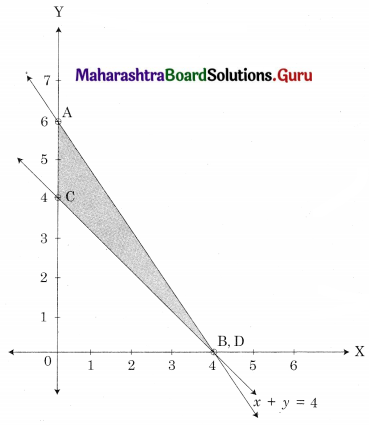
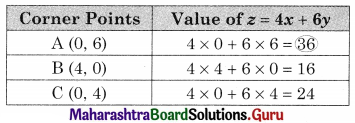
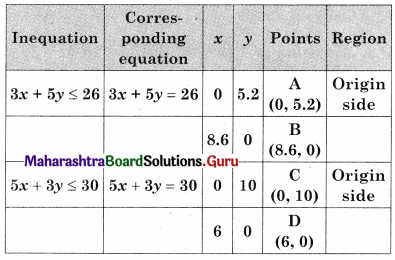
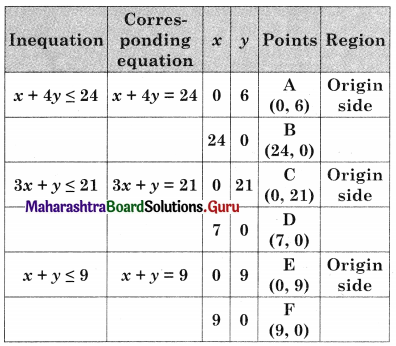
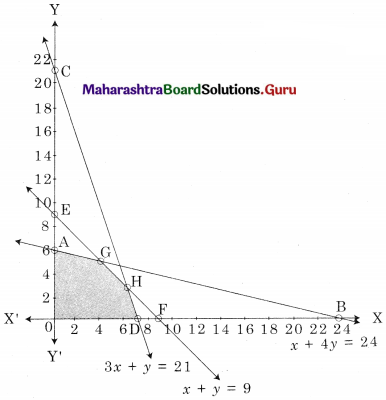
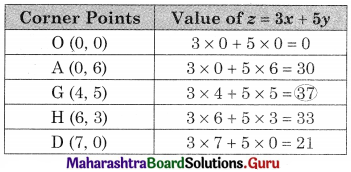
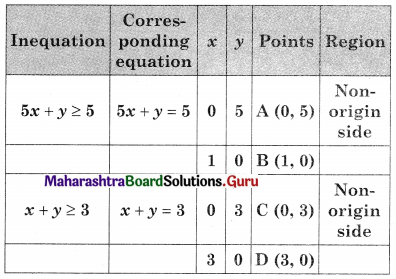
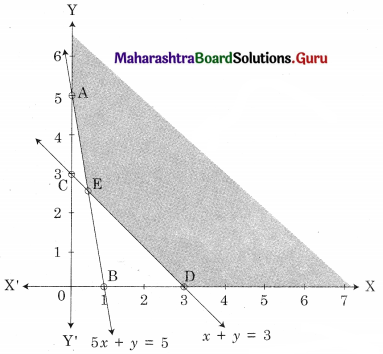
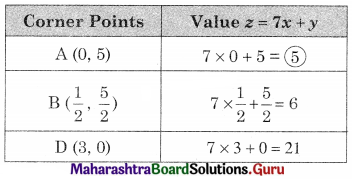
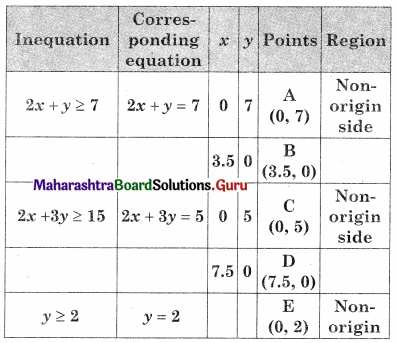
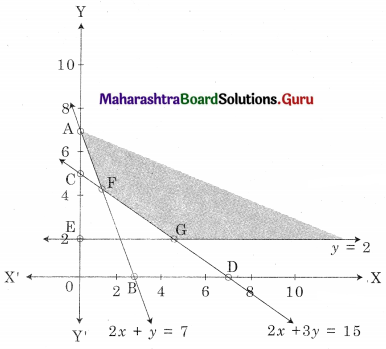
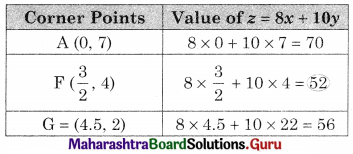
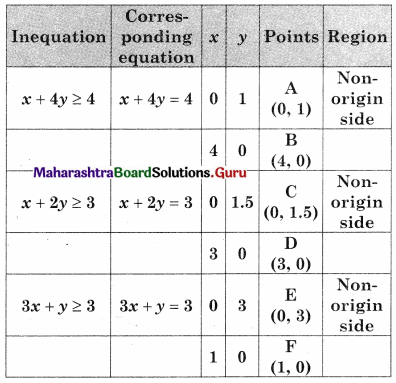
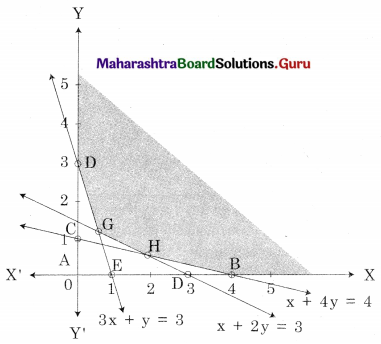
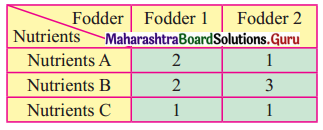
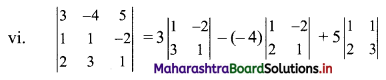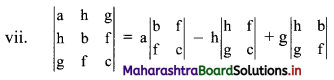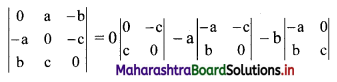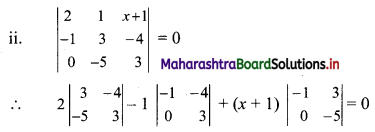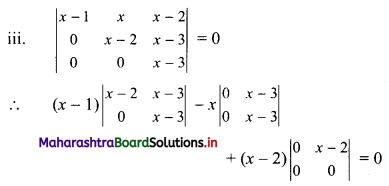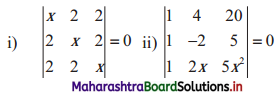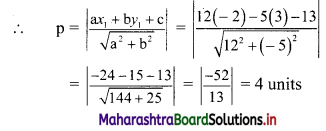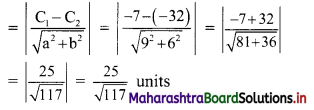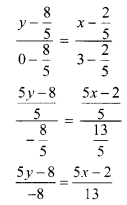Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 5 Locus and Straight Line Miscellaneous Exercise 5 Questions and Answers.

Maharashtra State Board 11th Commerce Maths Solutions Chapter 5 Locus and Straight Line Miscellaneous Exercise 5

Question 1.
Find the slopes of the lines passing through the following points:
(i) (1, 2), (3, -5)
(ii) (1, 3), (5, 2)
(iii) (-1, 3), (3, -1)
(iv) (2, -5), (3, -1)
Solution:
(i) Let A = (1, 2) = (x₁, y₁) and B = (3, -5) = (x₂, y₂) say.
Slope of line AB = \(\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{-5-2}{3-1}=\frac{-7}{2}\)

(ii) Let C = (1, 3) = (x₁, y₁) and D = (5, 2) = (x₂, y₂) say.
Slope of line CD = \(\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{2-3}{5-1}=\frac{-1}{4}\)

(iii) Let E = (-1, 3) = (x₁, y₁) and F = (3, -1) = (x₂, y₂) say.
Slope of line EF = \(\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{-1-3}{3-(-1)}=\frac{-4}{4}\) = -1

(iv) Let P = (2, -5) = (x₁, y₁) and Q = (3, -1) = (x₂, y₂) say.
Slope of line PQ = \(\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{-1-(-5)}{3-2}\) = \(\frac{-1+5}{1}\) = 4

Question 2.
Find the slope of the line which
(i) makes an angle of 120° with the positive X-axis.
(ii) makes intercepts 3 and -4 on the axes.
(iii) passes through the points A(-2, 1) and the origin.
Solution:
(i) θ = 120°
Slope of the line = tan 120°
= tan (180° – 60°)
= -tan 60° …..[tan(180° – θ) = -tan θ]
= -√3

(ii) Given, x-intercept of line is 3 and y-intercept of line is -4
∴ The line intersects X-axis at (3, 0) and Y-axis at (0, -4).
∴ The line passes through (3, 0) = (x₁, y₁) and (0, -4) = (x₂, y₂) say.
∴ Slope of line = \(\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{-4-0}{0-3}=\frac{-4}{-3}=\frac{4}{3}\)

(iii) Required line passes through O(0, 0) = (x₁, y₁) and A(-2, 1) = (x₂, y₂) say.
Slope of line OA = \(\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{1-0}{-2-0}=\frac{1}{-2}\) = \(\frac{-1}{2}\)

Question 3.
Find the value of k:
(i) if the slope of the line passing through the points (3, 4), (5, k) is 9.
(ii) the points (1, 3), (4, 1), (3, k) are collinear.
(iii) the point P(1, k) lies on the line passing through the points A(2, 2) and B(3, 3).
Solution:
(i) Let P(3, 4), Q(5, k).
Slope of PQ = 9 …….[Given]
∴ \(\frac{\mathrm{k}-4}{5-3}\) = 9
∴ \(\frac{\mathrm{k}-4}{2}\) = 9
∴ k – 4 = 18
∴ k = 22

(ii) The points A(1, 3), B(4, 1) and C(3, k) are collinear.
∴ Slope of AB = Slope of BC
∴ \(\frac{1-3}{4-1}=\frac{k-1}{3-4}\)
∴ \(\frac{-2}{3}=\frac{\mathrm{k}-1}{-1}\)
∴ 2 = 3k – 3
∴ k = \(\frac{5}{3}\)

(iii) Given, point P(1, k) lies on the line joining A(2, 2) and B(3, 3).
∴ Slope of AB = Slope of BP
∴ \(\frac{3-2}{3-2}=\frac{3-k}{3-1}\)
∴ 1 = \(\frac{3-k}{2}\)
∴ 2 = 3 – k
∴ k = 1

Question 4.
Reduce the equation 6x + 3y + 8 = 0 into slope-intercept form. Hence, find its slope.
Solution:
Given equation is 6x + 3y + 8 = 0, which can be written as
3y = -6x – 8
∴ y = \(\frac{-6 x}{3}-\frac{8}{3}\)
∴ y = -2x – \(\frac{8}{3}\)
This is of the form y = mx + c with m = -2
∴ y = -2x – \(\frac{8}{3}\) is in slope-intercept form with slope = -2

Question 5.
Verify that A(2, 7) is not a point on the line x + 2y + 2 = 0.
Solution:
Given equation is x + 2y + 2 = 0.
Substituting x = 2 and y = 7 in L.H.S. of given equation, we get
L.H.S. = x + 2y + 2
= 2 + 2(7) + 2
= 2 + 14 + 2
= 18
≠ R.H.S.
∴ Point A does not lie on the given line.

Question 6.
Find the X-intercept of the line x + 2y – 1 = 0.
Solution:
Given equation of the line is x + 2y – 1 = 0
To find the x-intercept, put y = 0 in given equation of the line
∴ x + 2(0) – 1 = 0
∴ x + 0 – 1 = 0
∴ x = 1
∴ X-intercept of the given line is 1.
Alternate method:
Given equation of the line is x + 2y – 1 = 0
i.e. x + 2y = 1
∴ \(\frac{x}{1}+\frac{y}{\frac{1}{2}}=1\)
Comparing with \(\frac{x}{\mathrm{a}}+\frac{y}{\mathrm{~b}}=1\), we get a = 1
X-intercept of the line is 1.

Question 7.
Find the slope of the line y – x + 3 = 0.
Solution:
Equation of given line is y – x + 3 = 0
i.e. y = x – 3
Comparing with y = mx + c, we get
m = Slope = 1

Question 8.
Does point A(2, 3) lie on the line 3x + 2y – 6 = 0? Give reason.
Solution:
Given equation is 3x + 2y – 6 = 0.
Substituting x = 2 and y = 3 in L.H.S. of given equation, we get
L.H.S. = 3x + 2y – 6
= 3(2)+ 2(3) – 6
= 6
≠ R.H.S.
∴ Point A does not lie on the given line.

Question 9.
Which of the following lines passes through the origin?
(a) x = 2
(b) y = 3
(c) y = x + 2
(d) 2x – y = 0
Solution:
Any line passing through origin is of the form y = mx or ax + by = 0.
Here in the given option, 2x – y = 0 is in the form ax + by = 0.

Question 10.
Obtain the equation of the line which is:
(i) parallel to the X-axis and 3 units below it.
(ii) parallel to the Y-axis and 2 units to the left of it.
(iii) parallel to the X-axis and making an intercept of 5 on the Y-axis.
(iv) parallel to the Y-axis and making an intercept of 3 on the X-axis.
Solution:
(i) Equation of a line parallel to X-axis is y = k.
Since, the line is at a distance of 3 units below X-axis.
∴ k = -3
∴ the equation of the required line is y = -3
i.e., y + 3 = 0.

(ii) Equation of a line parallel to Y-axis is x = h.
Since, the line is at a distance of 2 units to the left of Y-axis.
∴ h = -2
∴ the equation of the required line is x = -2
i.e., x + 2 = 0.

(iii) Equation of a line parallel to X-axis with y-intercept ‘k’ is y = k.
Here, y-intercept = 5
∴ the equation of the required line is y = 5.

(iv) Equation of a line parallel to Y-axis with x-intercept ‘h’ is x = h.
Here, x-intercept = 3
∴ the equation of the required line is x = 3.

Question 11.
Obtain the equation of the line containing the point:
(i) (2, 3) and parallel to the X-axis.
(ii) (2, 4) and perpendicular to the Y-axis.
(iii) (2, 5) and perpendicular to the X-axis.
Solution:
(i) Equation of a line parallel to X-axis is of the form y = k.
Since, the line passes through (2, 3).
∴ k = 3
∴ the equation of the required line is y = 3.

(ii) Equation of a line perpendicular to Y-axis
i.e., parallel to X-axis, is of the form y = k.
Since, the line passes through (2, 4).
∴ k = 4
∴ the equation of the required line is y = 4.

(iii) Equation of a line perpendicular to X-axis
i.e., parallel to Y-axis, is of the form x = h.
Since, the line passes through (2, 5).
∴ h = 2
∴ the equation of the required line is x = 2.

Question 12.
Find the equation of the line:
(i) having slope 5 and containing point A(-1, 2).
(ii) containing the point (2, 1) and having slope 13.
(iii) containing the point T(7, 3) and having inclination 90°.
(iv) containing the origin and having inclination 90°.
(v) through the origin which bisects the portion of the line 3x + 2y = 2 intercepted between the co-ordinate axes.
Solution:
(i) Given, slope (m) = 5 and the line passes through A(-1, 2).
Equation of the line in slope point form is y – y₁ = m(x – x₁)
∴ the equation of the required line is y – 2 = 5(x + 1)
∴ y – 2 = 5x + 5
∴ 5x – y + 7 = 0

(ii) Given, slope (m) = 13 and the line passes through (2, 1).
Equation of the line in slope point form is y – y₁ = m(x – x₁)
∴ the equation of the required line is y – 1 = 13(x – 2)
∴ y – 1 = 13x – 26
∴ 13x – y = 25.

(iii) Given, Inclination of line = θ = 90°
∴ the required line is parallel to Y-axis (or lies on the Y-axis.)
Equation of a line parallel to Y-axis is of the form x = h.
Since, the line passes through (7, 3).
∴ h = 7
∴ the equation of the required line is x = 7.

(iv) Given, Inclination of line = θ = 90°
∴ the required line is parallel to Y-axis (or lies on the Y-axis.)
Equation of a line parallel to Y-axis is of the form x = h.
Since, the line passes through origin (0, 0).
∴ h = 0
∴ the equation of the required line is x = 0.

(v) Given equation of the line is 3x + 2y = 2.
∴ \(\frac{3 x}{2}+\frac{2 y}{2}=1\)
∴ \(\frac{x}{\frac{2}{3}}+\frac{y}{1}=1\)

This equation is of the form \(\frac{x}{\mathrm{a}}+\frac{y}{\mathrm{~b}}=1\), with
a = \(\frac{2}{3}\), b = 1
∴ the line 3x + 2y = 2 intersects the X-axis at A(\(\frac{2}{3}\), 0) and Y-axis at B(0, 1).
Required line is passing through the midpoint of AB.
∴ Midpoint of AB = \(\left(\frac{\frac{2}{3}+0}{2}, \frac{0+1}{2}\right)=\left(\frac{1}{3}, \frac{1}{2}\right)\)
∴ Required line passes through (0, 0) and \(\left(\frac{1}{3}, \frac{1}{2}\right)\).
Equation of the line in two point form is
\(\frac{y-y_{1}}{y_{2}-y_{1}}=\frac{x-x_{1}}{x_{2}-x_{1}}\)
∴ the equation of the required line is
\(\frac{y-0}{\frac{1}{2}-0}=\frac{x-0}{\frac{1}{3}-0}\)
∴ 2y = 3x
∴ 3x – 2y = 0

Question 13.
Find the equation of the line passing through the points A(-3, 0) and B(0, 4).
Solution:
Since, the required line passes through the points A(-3, 0) and B(0, 4).
Equation of the line in two point form is
\(\frac{y-y_{1}}{y_{2}-y_{1}}=\frac{x-x_{1}}{x_{2}-x_{1}}\)
Here, (x₁, y₁) = (-3, 0) and (x₂, y₂) = (0, 4)
∴ the equation of the required line is
∴ \(\frac{y-0}{4-0}=\frac{x-(-3)}{0-(-3)}\)
∴ \(\frac{y}{4}=\frac{x+3}{3}\)
∴ 4x + 12 = 3y
∴ 4x – 3y + 12 = 0

Question 14.
Find the equation of the line:
(i) having slope 5 and making intercept 5 on the X-axis.
(ii) having an inclination 60° and making intercept 4 on the Y-axis.
Solution:
(i) Since, the x-intercept of the required line is 5.
∴ it passes through (5, 0).
Also, slope(m) of the line is 5
Equation of the line in slope point form is y – y₁ = m(x – x₁)
∴ the equation of the required line is y – 0 = 5(x – 5)
∴ y = 5x – 25
∴ 5x – y – 25 = 0

(ii) Given, Inclination of line = θ = 60°
∴ Slope of the line (m) = tan θ
= tan 60°
= √3
and the y-intercept of the required line is 4.
∴ it passes through (0, 4).
Equation of the line in slope point form is y – y₁ = m(x – x₁)
∴ the equation of the required line is y – 4 = √3(x – 0)
∴ y – 4 = √3x
∴ √3x – y + 4 = 0

Question 15.
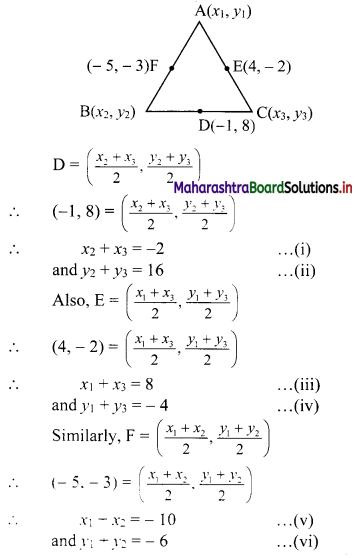
The vertices of a triangle are A(1, 4), B(2, 3), and C(1, 6). Find equations of
(i) the sides
(ii) the medians
(iii) Perpendicular bisectors of sides
(iv) altitudes of ∆ABC
Solution:
Vertices of ∆ABC are A(1, 4), B(2, 3), and C(1, 6)
(i) Equation of the line in two-point form is

Since, both the points A and C have same x co-ordinates i.e. 1
∴ the points A and C lie on a line parallel to Y-axis.
∴ the equation of side AC is x = 1.

(ii) Let D, E, and F be the midpoints of sides BC, AC, and AB respectively of ∆ABC.

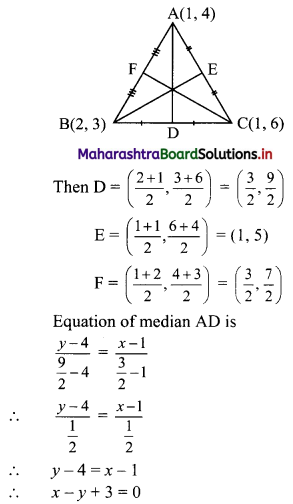
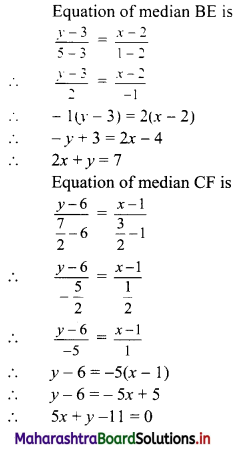
(iii) Slope of side BC = \(\left(\frac{6-3}{1-2}\right)=\left(\frac{3}{-1}\right)\) = -3
∴ Slope of perpendicular bisector of BC is \(\frac{1}{3}\) and the line passes through \(\left(\frac{3}{2}, \frac{9}{2}\right)\)
∴ Equation of the perpendicular bisector of side BC is \(\left(y-\frac{9}{2}\right)=\frac{1}{3}\left(x-\frac{3}{2}\right)\)
∴ \(\frac{2 y-9}{2}=\frac{1}{3}\left(\frac{2 x-3}{2}\right)\)
∴ 3(2y – 9) = (2x – 3)
∴ 2x – 6y + 24 = 0
∴ x – 3y + 12 = 0
Since, both the points A and C have the same x co-ordinates i.e. 1
∴ the points A and C lie on the line x = 1.
AC is parallel to Y-axis and therefore, the perpendicular bisector of side AC is parallel to X-axis.
Since, the perpendicular bisector of side AC passes through E(1, 5).
∴ the equation of the perpendicular bisector of side AC is y = 5.
Slope of side AB = \(\left(\frac{3-4}{2-1}\right)\) = -1
∴ Slope of perpendicular bisector of AB is 1 and the line passes through \(\left(\frac{3}{2}, \frac{7}{2}\right)\).
∴ Equation of the perpendicular bisector of side AB is \(\left(y-\frac{7}{2}\right)=1\left(x-\frac{3}{2}\right)\)
∴ \(\frac{2 y-7}{2}=\frac{2 x-3}{2}\)
∴ 2y – 7 = 2x – 3
∴ 2x – 2y + 4 = 0
∴ x – y + 2 = 0

(iv) Let AX, BY and CZ be the altitudes through the vertices A, B, and C respectively of ∆ABC.

Slope of BC = -3
∴ Slope of AX = \(\frac{1}{3}\) …..[∵ AX ⊥ BC]
Since, altitude AX passes through (1, 4) and has slope \(\frac{1}{3}\)
∴ equation of altitude AX is y – 4 = \(\frac{1}{3}\)(x – 1)
∴ 3y – 12 = x – 1
∴ x – 3y + 11 = 0
Since, both the points A and C have the same x co-ordinates i.e. 1
∴ the points A and C lie on the line x = 1.
AC is parallel to Y-axis and therefore, altitude BY is parallel to X-axis.
Since, the altitude BY passes through B(2, 3).
∴ the equation of altitude BY is y = 3.
Also, slope of AB = -1
∴ Slope of CZ = 1 …..[∵ CZ ⊥ AB]
Since, altitude CZ passes through (1, 6) and has slope 1
∴ equation of altitude CZ is y – 6 = 1(x – 1)
∴ y – 6 = x – 1
∴ x – y + 5 = 0

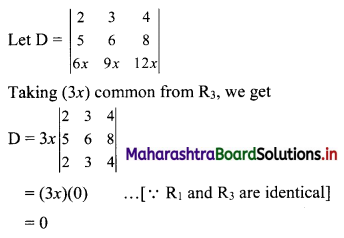
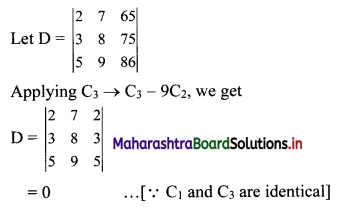
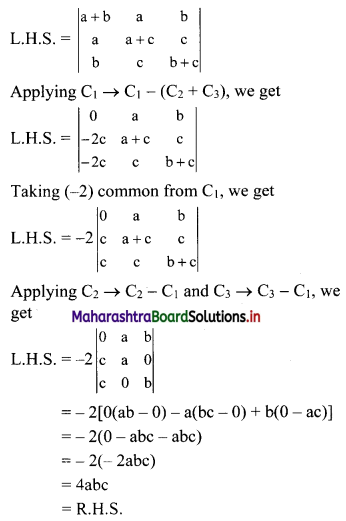
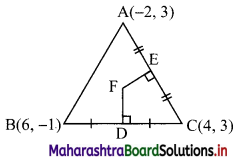
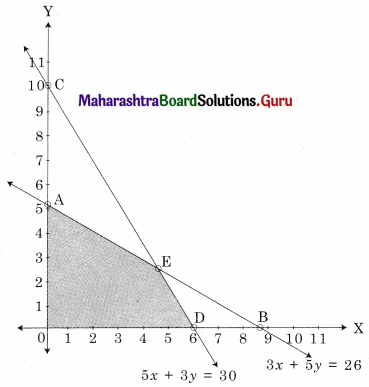
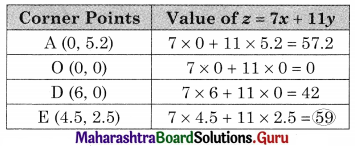
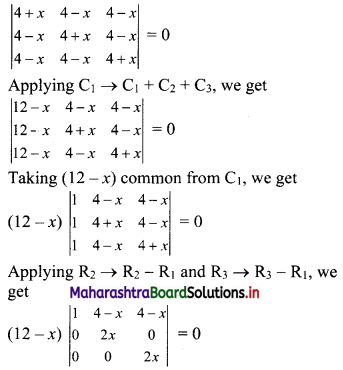
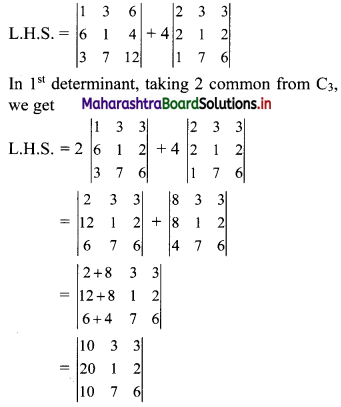
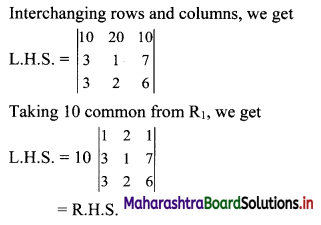
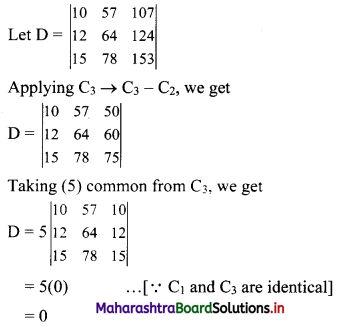
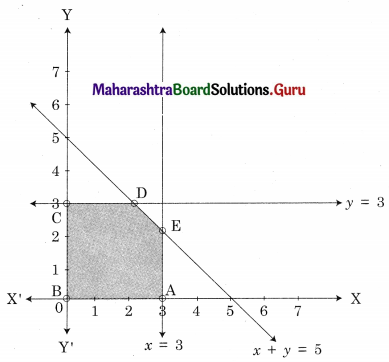
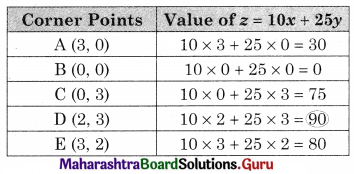
Balbharati Maharashtra State Board 11th Commerce Maths Solution Book Pdf Chapter 5 Locus and Straight Line Ex 5.4 Questions and Answers.

Maharashtra State Board 11th Commerce Maths Solutions Chapter 5 Locus and Straight Line Ex 5.4

Question 1.
Find the slope, x-intercept, y-intercept of each of the following lines.
(a) 2x + 3y – 6 = 0
(b) x + 2y = 0
Solution:
(a) Given equation of the line is 2x + 3y – 6 = 0
Comparing this equation with ax + by + c = 0, we get
a = 2, b = 3, c = -6
∴ Slope of the line = \(\frac{-a}{b}=\frac{-2}{3}\)
x-intercept = \(\frac{-\mathrm{c}}{\mathrm{a}}=\frac{-(-6)}{2}\) = 3
y-intercept = \(\frac{-\mathrm{c}}{\mathrm{b}}=\frac{-(-6)}{3}\) = 2

(b) Given equation of the line is x + 2y = 0
Comparing this equation with ax + by + c = 0, we get
a = 1, b = 2, c = 0
∴ Slope of the line = \(\frac{-a}{b}=\frac{-1}{2}\)
x-intercept = \(\frac{-c}{a}=\frac{-0}{1}\) = 0
y-intercept = \(\frac{-\mathrm{c}}{\mathrm{b}}=\frac{-0}{2}\) = 0

Question 2.
Write each of the following equations in ax + by + c = 0 form.
(a) y = 2x – 4
(b) y = 4
(c) \(\frac{x}{2}+\frac{y}{4}=1\)
(d) \(\frac{x}{3}=\frac{y}{2}\)
Solution:
(a) y = 2x – 4
∴ 2x – y – 4 = 0 is the equation in ax + by + c = 0 form.

(b) y = 4
∴ 0x + 1y – 4 = 0 is the equation in ax + by + c = 0 form.

(c) \(\frac{x}{2}+\frac{y}{4}=1\)
∴ \(\frac{2 x+y}{4}=1\)
∴ 2x + y = 4
∴ 2x + y – 4 = 0 is the equation in ax + by + c = 0 form.

(d) \(\frac{x}{3}=\frac{y}{2}\)
∴ 2x = 3y
∴ 2x – 3y + 0 = 0 is the equation in ax + by + c = 0 form.

Question 3.
Show that the lines x – 2y – 7 = 0 and 2x – 4y + 5 = 0 are parallel to each other.
Solution:
Let m₁ be the slope of the line x – 2y – 7 = 0.
∴ m₁ = \(\frac{-1}{-2}=\frac{1}{2}\)
Let m₂ be the slope of the line 2x – 4y + 5 = 0.
∴ m₂ = \(\frac{-2}{-4}=\frac{1}{2}\)
Since, m₁ = m₂
∴ The given lines are parallel to each other.

Question 4.
If the line 3x + 4y = p makes a triangle of area 24 square units with the co-ordinate axes, then find the value of p.
Solution:
Let the line 3x + 4y = p cuts the X and Y-axes at points A and B respectively.

3x + 4y = p
∴ \(\frac{3 x}{\mathrm{p}}+\frac{4 y}{\mathrm{p}}=1\)
∴ \(\frac{x}{\frac{p}{3}}+\frac{y}{\frac{p}{4}}=1\)
The equation is of the form \(\frac{x}{a}+\frac{y}{b}=1\), with a = \(\frac{p}{3}\) and b = \(\frac{p}{4}\)
∴ A = (a, 0) = (\(\frac{p}{3}\), 0) and B = (0, b) = (0, \(\frac{p}{4}\))
∴ OA = \(\frac{p}{3}\) and OB = \(\frac{p}{4}\)
Given, A(∆OAB) = 24 sq. units
∴ \(\frac{1}{2}\) × OA × OB = 24
∴ \(\frac{1}{2}\) × \(\frac{p}{3}\) × \(\frac{p}{4}\) = 24
∴ p² = 576
∴ p = ±24

Question 5.
Find the co-ordinates of the circumcentre of the triangle whose vertices are A(-2, 3), B(6, -1), C(4, 3).
Solution:
Here, A(-2, 3), B(6, -1), C(4, 3) are the vertices of ∆ABC.
Let F be the circumcentre of ∆ABC.
Let FD and FE be the perpendicular bisectors of the sides BC and AC respectively.

∴ D and E are the midpoints of side BC and AC respectively.
∴ D = \(\left(\frac{6+4}{2}, \frac{-1+3}{2}\right)\) = (5, 1)
and E = \(\left(\frac{-2+4}{2}, \frac{3+3}{2}\right)\) = (1, 3)
Now, slope of BC = \(\frac{-1-3}{6-4}\) = -2
∴ slope of FD = \(\frac{1}{2}\) …..[∵ FD ⊥ BC]
Since, FD passes through (5, 1) and has slope \(\frac{1}{2}\)
∴ Equation of FD is y – 1 = \(\frac{1}{2}\)(x – 5)
∴ 2(y – 1) = x – 5
∴ x – 2y – 3 = 0 ……(i)
Since, both the points A and C have same y co-ordinates i.e. 3
∴ the points A and C lie on the liney = 3.
Since, FE passes through E(1, 3).
∴ the equation of FE is x = 1. …….(ii)
To find co-ordinates of circumcentre, we have to solve equations (i) and (ii).
Substituting the value of x in (i), we get
1 – 2y – 3 = 0
∴ y = -1
∴ Co-ordinates of circumcentre F = (1, -1).

Question 6.
Find the equation of the line whose x-intercept is 3 and which is perpendicular to the line 3x – y + 23 = 0.
Solution:
Slope of the line 3x – y + 23 = 0 is 3.
∴ slope of the required line which is perpendicular to 3x – y + 23 = 0 is \(\frac{-1}{3}\).
Since, the x-intercept of the required line is 3.
∴ it passes through (3, 0).
∴ the equation of the required line is
y – 0 = \(\frac{-1}{3}\) (x – 3)
∴ 3y = -x – 3
∴ x – 3y = 3

Question 7.
Find the distance of the point A(-2, 3) from the line 12x – 5y – 13 = 0.
Solution:
Let p be the perpendicular distance of the point A(-2, 3) from the line 12x – 5y – 13 = 0
Here, a = 12, b = -5, c = -13, x₁ = -2, y₁ = 3

Question 8.
Find the distance between parallel lines 9x + 6y – 1 = 0 and 9x + 6y – 32 = 0.
Solution:
Equations of the given parallel lines are 9x + 6y – 7 = 0 and 9x + 6y – 32 = 0.
Here, a = 9, b = 6, C₁ = -7 and C₂ = -32
∴ Distance between the parallel lines

Question 9.
Find the equation of the line passing through the point of intersection of lines x + y – 2 = 0 and 2x – 3y + 4 = 0 and making intercept 3 on the X-axis.
Solution:
Given equations of lines are
x + y – 2 = 0 ……(i)
and 2x – 3y – 4 = 0 ……(ii)
Multiplying equation (i) by 3, we get
3x – 3y – 6 = 0 …..(iii)
Adding equation (ii) and (iii), we get
5x – 2 = 0
∴ x = \(\frac{2}{5}\)
Substituting x = \(\frac{2}{5}\) in equation (i), we get
\(\frac{2}{5}\) + y – 2 = 0
∴ y = 2 – \(\frac{2}{5}\) = \(\frac{8}{5}\)
∴ The required line passes through point (\(\frac{2}{5}\), \(\frac{8}{5}\)).
Also, the line makes intercept of 3 on X-axis
∴ it also passes through point (3, 0).
∴ required equation of line passing through points (\(\frac{2}{5}\), \(\frac{8}{5}\)) and (3, 0) is

∴ 13(5y – 8) = -8(5x – 2)
∴ 65y – 104 = -40x + 16
∴ 40x + 65y – 120 = 0
∴ 8x + 13y – 24 = 0 which is the equation of the required line.

Question 10.
D(-1, 8), E(4, -2), F(-5, -3) are midpoints of sides BC, CA and AB of ∆ABC. Find
(i) equations of sides of ∆ABC.
(ii) co-ordinates of the circumcentre of ∆ABC.
Solution:
(i) Let A(x₁, y₁), B(x₂, y₂) and C(x₃, y₃) be the vertices of ∆ABC.
Given, points D, E and F are midpoints of sides BC, CA and AB respectively of ∆ABC.

For x-coordinates:
Adding (i), (iii) and (v), we get
2x₁ + 2x₂ + 2x₃ = -4
∴ x₁ + x₂ + x₃ = -2 …..(vii)
Solving (i) and (vii), we get x₁ = 0
Solving (iii) and (vii), we get x₂ = -10
Solving (v) and (vii), we get x₃ = 8
For y-coordinates:
Adding (ii), (iv) and (vi), we get
2y₁ + 2y₂ + 2y₃ = 6
∴ y₁ + y₂ + y₃ = 3 …..(viii)
Solving (ii) and (viii), we get y₁ = -13
Solving (iv) and (viii), we get y₂ = 7
Solving (vi) and (viii), we get y₃ = 9
∴ Vertices of ∆ABC are A(0, -13), B(-10, 7), C(8, 9)

(ii) Here, A(0, -13), B(-10, 7), C(8, 9) are the vertices of ∆ABC.
Let F be the circumcentre of ∆ABC.
Let FD and FE be perpendicular bisectors of the sides BC and AC respectively.

∴ D and E are the midpoints of side BC and AC.
∴ D = \(\left(\frac{-10+8}{2} \cdot \frac{7+9}{2}\right)\) = (-1, 8)
and E = \(\left(\frac{0+8}{2}, \frac{-13+9}{2}\right)\) = (4, -2)
Now, slope of BC = \(\frac{7-9}{-10-8}=\frac{1}{9}\)
∴ slope of FD = -9 ……[∵ FD ⊥ BC]
Since, FD passes through (-1, 8) and has slope -9
∴ Equation of FD is y – 8 = -9(x + 1)
∴ y – 8 = -9x – 9
∴ y = -9x – 1 …..(i)
Also, slope of AC = \(\frac{-13-9}{0-8}=\frac{11}{4}\)
∴ Slope of FE = \(\frac{-4}{11}\) ….[∵ FE ⊥ AC]
Since, FE passes through (4, -2) and has slope \(\frac{-4}{11}\)
∴ Equation of FE is y + 2 = \(\frac{-4}{11}\)(x – 4)
∴ 11(y + 2) = -4(x – 4)
∴ 11y + 22 = -4x + 16
∴ 4x + 11y = -6 ….(ii)
To find co-ordinates of circumcentre, we have to solve equations (i) and (ii).
Substituting the value of y in (ii), we get
∴ 4x + 11(-9x – 1) = -6
∴ 4x – 99x – 11 = -6
∴ -95x = 5
∴ x = \(\frac{-1}{19}\)
Substituting the value of x in (i), we get
y = -9(\(\frac{-1}{19}\)) – 1 = \(\frac{-10}{19}\)
∴ Co-ordinates of circumcentre F = \(\left(\frac{-1}{19}, \frac{-10}{19}\right)\)

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 6 Linear Programming Ex 6.2 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 6 Linear Programming Ex 6.2

Solve the following LPP by graphical method.

Question 1.
Maximize z = 11x + 8y, Subject to x ≤ 4 ,y ≤ 6 x + y ≤ 6, x ≥ 0, y ≥ 0.
Solution:


The feasible solution is AOBE
Where A(4, 0) O(0, 0) B(0, 6)
E is the point of intersection of x + y = 6 and x = 4.
∴ 4 + y = 6
∴ y = 2
∴ E = (4, 2)

∴ z is maximum at (4, 12) and the maximum value of z = 60

Question 2.
Maximize z = 4x + 6y, Subject to 3x + 2y ≤ 12, x + y ≥ 4 x, y ≥ 0.
Solution:


From figure, ABC is the feasible region
Where A(0, 6) B(4, 0) C(0, 4)

Maximum value of z = 36 at A(0, 6)

Question 3.
Maximize z = 7x + 11y, Subject to 3x + 5y ≤ 26, 5x + 3y ≤ 30, x ≥ 0, y ≥ 0.
Solution:


∴ AODE is the feasible region where
A(0, 5.2) O(0, 0) D(6, 0) and E is the intersection of 3x + 5y = 26 and 5x + 3y = 30
For E,
Solving 3x + 5y = 26 ……(i)
5x + 3y = 30 ……(ii)
We get, x = 4.5, y = 2.5
∴ E = (4.5, 2.5)

∴ Maximum value of z = 59 at E(4.5, 2.5)

Question 4.
Maximize z = 10x + 25y, Subject to 0 ≤ x ≤ 3, 0≤ y ≤ 3, x + y ≤ 5.
Solution:
The constraints can be written as, x ≤ 3, x ≥ 0, y ≥ 0, y ≤ 3, x + y ≤ 5


ABCDE is the feasible region where A(3, 0) B(0, 0) and C(0, 3) D is the intersection of y = 3 and x + 5y = 5 and E is the intersection of x = 3 and x + 7 = 5
For D,
Solving y = 3 ………(i)
x + y = 5 ……..(ii)
We get x = 2, y = 3
∴ D = (2, 3)
For E,
Solving x = 3 …….(i)
x + y = 5 ……….(ii)
We get x = 3, y = 2
∴ E = (3, 2)

∴ Maximum value of z = 90 at D(2, 3)

Question 5.
Maximize z = 3x + 5y, Subject to x + 4y ≤ 24, 3x + y ≤ 21, x + y ≤ 9, x ≥ 0, y ≥ 0.
Solution:


OAGHD is the feasible region where O(0, 0), A(0, 6), D(7, 0) G is the intersecting point of x + 4y = 24 and x + y = 9
H is the intersecting points of 3x + y = 21 and x + y = 9.
For G, Solving x + 4y = 24 …….(i)
x + y = 9 ………(ii)
We get, x = 4, y = 5
∴ G (4, 5)
For H, Solving x + y = 9 ………(i)
3x + y = 21 ……..(ii)
We get x = 6, y = 3
∴ H(6, 3)

∴ Maximum value of z = 37 at the point G(4, 5)

Question 6.
Minimize z = 7x + y Subject to 5x + y ≥ 5, x + y ≥ 3, x ≥ 0, y ≥ 0
Solution:


AED is the feasible region where A(0, 5) D(3, 0) and E is the point of intersection of 5x + y = 5 and x + y = 3.
For E, Solving 5x + y = 5 ………(i)
x + y = 3 ……..(ii)
We get, x = \(\frac{1}{2}\), y = \(\frac{5}{2}\)
∴ E(\(\frac{1}{2}\), \(\frac{5}{2}\))

∴ Minimum value of z = 5 at A(0, 5)

Question 7.
Minimize z = 8x + 10y, Subject to 2x + y ≥ 7, 2x + 3y ≥ 15 ,y ≥ 2, x ≥ 0, y ≥ 0
Solution:


AEG is the feasible solution where A(0, 7)
F is the point of intersection of 2x + y = 7 and 2x + 3y = 15
G is the point of intersection of y = 2 and 2x + 3y = 15
For F, Solving 2x + y = 7 ……..(i)
2x + 3y = 15 ……..(ii)
We get x = \(\frac{3}{2}\), y = 4
∴ F = (\(\frac{3}{2}\), 4)
For G, Solving 2x + 3y = 15 ……..(i)
y = 2 …….(ii)
We get x = 4.5, y = 2
∴ G = (4.5, 2)

∴ Minimum value of z = 52 at F(\(\frac{3}{2}\), 4)

Question 8.
Minimize z = 6x + 2y, Subject to x + 2y ≥ 3, x + 4y ≥ 4, 3x + y ≥ 3, x ≥ 0, y ≥ 0.
Solution:


DGHB is the feasible region where D(0, 3), B(4, 0)
G is the point of intersection of 3x + y = 3 and x + 2y = 3 and H is the point of intersection of x + 2y = 3 and x + 4y = 4
For G, Solving 3x + y = 3 ……(i)
x + 2y = 3 ………(ii)
W e get x = \(\frac{3}{5}\), y = \(\frac{6}{5}\)
∴ G(\(\frac{3}{5}\), \(\frac{6}{5}\))
For H, Solving x + 2y = 3 ……..(i)
x + 4y = 4 ………(ii)
We get, x = 2, y = \(\frac{1}{2}\)
∴ H(2, \(\frac{1}{2}\))

∴ Minimum value of z = 22.5 at H(2, \(\frac{1}{2}\))

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 6 Linear Programming Ex 6.1 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 6 Linear Programming Ex 6.1

Question 1.
A manufacturing firm produces two types of gadgets A and B, which are first processed in the foundry and then sent to a machine shop for finishing. The number of man-hours of labour required in each shop for production of A and B and the number of man-hours available for the firm is as follows.

Profit on the sale of A is ₹ 30 and B ₹ 20 Per unit Formulate the LPP to have maximum profit.
Solution:
Let the manufacturing firm produce x gadgets of type A and y gadgets of type B.
On selling x gadgets of type A the firm gets ₹ 30 and that on type B is ₹ 20.
∴ Total profit is z = ₹ 30x + 20y.
Since x and y are the numbers of gadgets, x ≥ 0, y ≥ 0
From the given table, the availability of man-hours of labour required in each shop and for the firm is given as 60 and 35.
∴ The inequation are 10x + 6y ≤ 60 and 5x + 4y ≤ 35.
Hence the given LPP can be formulated as Maximize z = 30x + 20y
Subject to 10x + 6y ≤ 60, 5x + 4y ≤ 35, x ≥ 0, y ≥ 0.

Question 2.
In a cattle breeding farm, it is prescribed that the food ratio for one animal must contain 14, 22, and 1 unit of nutrients A, B, and C respectively. Two different kinds of fodder are available. Each unit weight of these two contains the following:

The cost of fodder 1 is ₹ 3 per unit and that of fodder 2 is ₹ 2 per unit. Formulate the LPP to minimize the cost.
Solution:
Let x unit of fodder 1 and y unit of fodder 2 be included in the ration of an animal
The cost of 1 unit of fodder 1 is ₹ 3 and the cost of 1 unit of fodder 2 is ₹ 2.
∴ The total cost is ₹ 3x + 2y.
The minimum requirement of the nutrients A, B, and C is given as 14 units, 22 units, and 1 unit.
∴ From the given table, the daily food ration will include (2x + 2y) unit of Nutrient A, (2x + 3y) unit of Nutrient B, and (x + y) of Nutrient C.
The total cost is 2 = ₹ 3x + 2y
Hence the given LPP can be formulated as Minimize z = 3x + 2y
subject to 2x + y ≥ 14, 2x + 3y ≥ 22, x + y ≥ 1, x ≥ 0, y ≥ 0.

Question 3.
A Company manufactures two types of chemicals A and B. Each chemical requires two types of raw materials P and Q. The table below shows a number of units of P and Q required to manufacture one unit of A and one unit of B.

The company gets profits of ₹ 350/- and ₹ 400/- by selling one unit of A and one unit of B respectively. Formulate the problem as LPP to maximize the profit.
Solution:
∴ Let the company manufactures x unit of chemical A and y unit of chemical B.
The availability of the raw materials for the production of chemicals A and B are given as 120 and 160 units.
The company gets ₹ 350 as profit on selling one unit of chemical A and ₹ 400 as profit on selling one unit of chemical B.
∴ Total profit is ₹ (350x + 400y).
The inequation can be written as.
3x + 2y ≤ 120
2x + 5y ≤ 160
and x & y cannot be negative
Hence the LPP can be formulated as follows,
Maximize z = 350x + 400y
Subject to 3x + 2y ≤ 120, 2x + 5y ≤ 160, x ≥ 0, y ≥ 0.

Question 4.
A printing company prints two types of magazines A and B. The company earns ₹ 10 and ₹ 15 on magazines A and B per copy. These are processed on three machines I, II, III. Magazine A requires 2 hours on the machine I, 5 hours on machine II, and 2 hours on machine III. Magazine B requires 3 hours on machine 1, 2 hours on machine II and 6 hours on machine III. Machines I, II, III are available for 36, 50, 60 hours per week respective. Formulate the linear programming problem to maximize the profit.
Solution:
Let the company print x magazine of type A and y magazines of type B.
Then the total earnings of the company are ₹ 10x + 15y.
The given problem can be tabulated as follows.

From the table, the total time required for Machine I is (2x + 3y) hours, for machine II is (5x + 2y) hours, and for machine III is (2x + 6y) hours.
The machine I, II, and III are available for 36, 50, and 60 hours per work.
∴ The constraints are 2x + 3y ≤ 36, 5x + 2y ≤ 50 and 2x + 6y ≤ 60.
Since x and y cannot be negative, we have x ≥ 0, y ≥ 0.
Hence the given LPP can be formulated as
Maximize z = 10x + 15y
Subject to 2x + 3y ≤ 36, 5x + 2y ≤ 50, 2x + 6y ≤ 60, x ≥ 0, y ≥ 0.

Question 5.
Manufacture produces bulbs and tubes. Each of these must be processed through two machines M₁ and M₂. A package of bulbs requires 1 hour of work on machine M₁ and 3 hours of work on M₂. A package of tubes requires 2 hours on machine M₁ and 4 hours on machine M₂. He earns a profit of ₹ 13.5 per package of bulbs and ₹ 55 per package of tubes. Formulate the LPP to maximize the profit. He operates M₁ for at most 10 hours and M₂ for at most 12 hours a day.
Solution:
Let the manufacturer produce x packages of bulbs and y packages of tubes.
He earns a profit of ₹ 13.5 per packages of bulbs and ₹ 55 per package of tubes.
∴ His total profit = ₹ (13.5x + 55y).
The given problem can be tabulated as follows.

From the above table, the total time required for M₁ is (x + 2y), and that of M₂ is (3x + 4y).
M₁ and M₂ are available for at most 10 hrs per day and 12 hours per day.
∴ The constraint for the objective function is x + 2y ≤ 10, 3x + 4y ≤ 12
Hence the give LPP can be formulated as
Maximize z = 13.5x + 55y
Subject to x + 2y ≤ 10, 3x + 4y ≤ 12, x ≥ 0, y ≥ 0.

Question 6.
A Company manufactures two types of fertilizers F₁ and F₂. Each type of fertilizer requires two raw materials A and B. The number of units of A and B required to manufacture one unit of fertilizer F₁ and F₂ and availability of the raw materials A and B per day are given in the table below

By selling one unit of F₁ and one unit of F₂, the company gets a profit of ₹ 500 and ₹ 750 respectively. Formulate the problem as LPP to maximize the profit.
Solution:
Let the company manufacture x units of Fertilizers F₁ and y units of fertilizer F₂.
The company gets a profit of ₹ 500 and ₹ 750 by selling a unit of F₁ and F₂.
∴ Total profit = ₹ (500x + 750y)
The availability of raw materials A and B per day is given as 40 and 70.
∴ From the given table the constraints can be written as 2x + 3y ≤ 40 and x + 4y ≤ 70.
Since x & y cannot be negative, x ≥ 0, y ≥ 0
Hence the given LPP can be formulated as
Maximize z = 500x + 750y
Subject to 2x + 3y ≤ 40, x + 4y ≤ 70, x ≥ 0, y ≥ 0.

Question 7.
A doctor has prescribed two different kinds of feeds A and B to form a weekly diet for a sick person. The minimum requirement of fats, carbohydrates, and proteins are 18, 28,14 units respectively. One unit of food A has 4 units of fat, 14 units of carbohydrates, and 8 units of protein. One unit of food B has 6 units of fat, 12 units of carbohydrates and 8 units of protein. The price of food A is ₹ 4.5 per unit and that of food B is ₹ 3.5 per unit. Form the LPP so that the sick person’s diet meets the requirements at minimum cost.
Solution:
Let x unit of food A and y unit of food B be consumed by a sick person.
The cost of food A in ₹ 4.5 per unit and food B is ₹ 3.5 per unit.
∴ Total cost = ₹ (4.5x + 3.5y)
The given conditions can be tabulated as follows.

∴ The given LPP can be formulated as
Minimise z = 4.5x + 3.5y
Subject to 4x + 6y ≥ 18, 14x + 12y ≥ 28, 8x + 8y ≥ 14, x ≥ 0, y ≥ 0.

Question 8.
If John drives a car at a speed of 60 kms/hour he has to spend ₹ 5 per km on petrol. If he drives at a faster speed of 90 km/ hour, the cost of petrol increases to ₹ 8 per km. He has ₹ 600 to spend on petrol and wishes to travel the maximum distance within an hour. Formulate the above problem as LPP.
Solution:
Let John drive x km at a speed of 60 km/hr and y km at a speed of 90 km/hr.
∴ Time required to drive a distance of x km is \(\frac{x}{60}\) hours and the time require to drive at a distance of y km is \(\frac{y}{90}\) hours.
∴ Total time required \(\left(\frac{x}{60}+\frac{y}{90}\right)\) hours.
Since he wishes to drive maximum distance within an hour,
\(\frac{x}{60}+\frac{y}{90} \leq 1\)
He has to spend ₹ 5 per km at a speed of 60 km/hr and ₹ 8 per km at a speed of 90 km/hr.
He has ₹ 600 on petrol to spend, 5x + 8y ≤ 600
The total distance he wishes to travel is (x + y) hours.
∴ The given LPP can be formulated as
Maximize z = x + y
Subject to \(\frac{x}{60}+\frac{y}{90}\) ≤ 1, 5x + 8y ≤ 600, x ≥ 0, y ≥ 0.

Question 9.
The company makes concrete bricks made up of cement and sand. The weight of a concrete brick has to be at least 5 kg. Cement costs ₹ 20 per kg. and sand costs ₹ 6 per kg. Strength considerations dictate that a concrete brick should contain a minimum of 4 kg of cement and not more than 2 kg of sand. Formulate the LPP for the cost to be minimum.
Solution:
Let the concrete brick contain x kg of cement and y kg of sand.
The cost of cement is ₹ 20 per kg and sand is ₹ 6 per kg.
∴ The total cost = ₹ (20x + 6y)
Since the weight of the concrete brick has to be at least 5 kg, therefore, x + y ≥ 5
Also, the concrete brick should contain a minimum of 4 kg of cement, i.e. x ≥ 4, and not more than 2 kg of sand, i.e, y ≤ 2.
∴ The LPP can be formulated as
Minimize z = 20x + 6y
Subject to x + y ≥ 5, x ≥ 4, y ≤ 2, x ≥ 0, y ≥ 0.

Balbharati Maharashtra State Board 12th Commerce Maths Digest Pdf Chapter 1 Commission, Brokerage and Discount Ex 1.2 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 1 Commission, Brokerage and Discount Ex 1.2

Question 1.
What is the present worth of a sum of ₹ 10,920 due six months hence at 8% p.a simple interest?
Solution:
Given, SD = ₹ 10,920
n = \(\frac{6}{12}\) year = \(\frac{1}{2}\) year
r = 8%
We have,

Thus the present worth is ₹ 10,500

Question 2.
What is the sum due of ₹ 8,000 due 4 months at 12.5% simple interest?
Solution:
Given, PW = ₹ 8,000, n = \(\frac{4}{12}\) year = \(\frac{1}{3}\) year, r = 12.5%
We have,

Thus, the sum due is ₹ 8,333.33

Question 3.
The true discount on the sum due 8 months hence at 12% p.a. is ₹ 560. Find the sum due and present worth of the bill.
Solution:
Given, TD = ₹ 560, n = \(\frac{8}{12}\) year = \(\frac{2}{3}\) year, r = 12%
We have,
TD = \(\frac{\mathrm{PW} \times n \times r}{100}\)
∴ 560 = \(\frac{\mathrm{PW} \times 2 \times 12}{3 \times 100}\)
∴ PW = 560 × \(\frac{25}{2}\) = ₹ 7,000
Now, SD = PW + TD
= 7,000 + 560
= ₹ 7,560

Question 4.
The true discount on a sum is \(\frac{3}{8}\) of the sum due at 12% p.a. Find the period of the bill.
Solution:

8 × n × 12 = 3(100 + n × 12)
96n = 300 + 36n
60n = 300
∴ n = 5
∴ Period of the bill = 5 years.

Question 5.
20 copies of a book can be purchased for a certain sum payable at the end of 6 months and 21 copies for the same sum in ready cash. Find the rate of interest.
Solution:
Given, n = \(\frac{6}{12}\) year = \(\frac{1}{2}\) year
Let the sum payable be ₹ x
Let the rate of interest be r%
According to given condition,
PW of one book = \(\frac{x}{21}\)
SD of one book = \(\frac{x}{20}\)

Thus, the rate of interest is 10%.

Question 6.
Find the true discount, Banker’s discount, and Banker’s gain on a bill of ₹ 4,240 due 6 months hence at 9% p.a.
Solution:

And, Banker’s Gain (BG) = BD – TD
= 190.80 – 182.58
= ₹ 8.22

Question 7.
The true discount on a bill is ₹ 2,200 and bankers discount is ₹ 2,310. If the bill is due 10 months, hence, find the rate of interest.
Solution:
Given, TD = ₹ 2,200, BD = ₹ 2,310
n = \(\frac{10}{12}=\frac{5}{6}\) year

∴ \(\frac{r}{120}=\frac{1}{20}\)
∴ r = 6%
Thus, rate of interest is 6%

Question 8.
A bill of ₹ 6,395 drawn on 19th January 2015 for 8 months was discounted on 28th February 2015 at 8% p.a. interest. What is the banker’s discount? What is the cash value of the bill?
Solution:
Face value = ₹ 6,395
Date of drawing = 19/01/2015
Period of the bill = 8 months
Nominal Due date = 19/09/2015
Legal due date = 22/09/2015
Date of discounting = 28/02/2015
Now, the unexpired period = Legal due date – Date of discounting
= 22/09/2015 – 28/02/2015
= days (as shown below)

Cash Value = FV – BD
= 6,395 – 313.12
= ₹ 6,621.38

Question 9.
A bill of ₹ 8,000 drawn on 5th January 1998 for 8 months was discounted for ₹ 7,680 on a certain date. Find the date on which it was discounted at 10% p.a.
Solution:
Bankers discount (BD) = FV – cash value
= 8,000 – 7,680
= ₹ 320
Let the unexpired period be x days
∴ BD = \(\frac{\mathrm{FV} \times x \times r}{365 \times 100}\)
∴ 320 = \(\frac{8,000 \times x \times 10}{365 \times 100}\)
∴ x = 146 days
∴ The unexpired days = 146 days
Date of drawing = 05/01/1998
Period of bill = 8 months
Nominal due date = 05/09/1998
Legal due date = 08/09/1998
Thus, the date of discounting is 146 days before the legal due date

∴ Date of discounting of the bill is 15th April 1998

Question 10.
A bill drawn on 5th June for 6 months was discounted at the rate of 5% p.a. on 19th October. If the cash value of the bill is ₹ 43,500, find the face value of the bill.
Solution:
Date of drawing = 5th June
Period of bill = 6 months
Nominal due date = 5th December
Legal due date = 8th December
Date of discounting = 19th October
Rate of interest = 5% p.a.
Let the face value of the bill be ₹ x
The unexpired period

Question 11.
A bill was drawn on 14th April for ₹ 7,000 and was discounted on 6th July at 5% p.a. The Banker paid ₹ 6,930 for the bill. Find the period of the bill.
Solution:
Face value = ₹ 7,000, cash value = ₹ 6,930
∴ Banker’s discount = 7,000 – 6,930 = ₹ 70
Date of drawing = 14/04
Date of discounting = 06/07
Rate of interest = 5%
Let the unexpired period = x days
∴ BD = \(\frac{7,000 \times x \times 5}{365 \times 100}\)
∴ 70 = \(\frac{70 \times x}{73}\)
∴ x = 73 days
∴ Legal due date of the bill is 73 days after the date of discounting.

∴ Legal due date = 17/09
∴ Nominal due date = 14/09
∴ Period of the bill = 5 months

Question 12.
If the difference between true discount and banker’s discount on a sum due 4 months hence is ₹ 20. Find true discount, banker’s discount and amount of bill, the rate of simple interest charged is 5% p.a.
Solution:
Banker’s gain (BG) = Banker’s discount (BD) – True Discount (TD)
∴ BG = ₹ 20
Also, BG = \(\frac{\mathrm{TD} \times n \times r}{100}\)
∴ 20 = \(\frac{\mathrm{TD} \times 4 \times 5}{12 \times 100}\)
∴ 20 = \(\frac{\mathrm{TD}}{60}\)
∴ TD = ₹ 1200
Now, BD = BG + TD
= 20 + 1,200
= ₹ 1,220
Also, BD = \(\frac{\mathrm{FV} \times n \times r}{100}\)
∴ 1,220 = \(\frac{\mathrm{FV} \times 4 \times 5}{12 \times 100}\)
∴ FV = 1,200 × 60 = ₹ 73,200
∴ Amounting the bill = ₹ 73,200

Question 13.
A bill of ₹ 51,000 was drawn on 18th February 2010 for 9 months. It was encashed on 28th June 2010 at 5% p.a. Calculate the banker’s gain and true discount.
Solution:
Face Value = ₹ 51,000
Date of drawing = 18/02/2010
Period of the bill = 9 months
Nominal due date = 18/11/2010
Legal due date = 21/11/2010
Date of discounting = 28/06/2010
Unexpired period

∴ TD = ₹ 1,000
∴ BG = BD – TD
= 1,020 – 1,000
= ₹ 20

Question 14.
A certain sum due 3 months hence is \(\frac{21}{20}\) of the present worth, what is the rate of interest.
Solution:

Question 15.
A bill of a certain sum drawn on 28th February 2007 for 8 months was encashed on 26th March 2007 for ₹ 10,992 at 14% p.a. Find the face value of the bill.
Solution:
Date drawing = 28/02/2007
Period of the bill = 8 months
Nominal due date = 28/10/2007
Legal due date = 31/10/2007
Date of discounting = 26/03/2007
Cash value = ₹ 10,992
Rate of interest = 14%
Let face value of the bill = ₹ x
Bankers discount = Face value – Cash value = x – 10,992
Also, Banker s discount = \(\frac{F V \times n \times r}{365 \times 100}\)
Where n is the unexpired days

Thus face value of the bill = ₹ 12,000

Balbharati Maharashtra State Board 12th Commerce Maths Digest Pdf Chapter 1 Commission, Brokerage and Discount Ex 1.1 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 1 Commission, Brokerage and Discount Ex 1.1

Question 1.
An agent charges a 12% commission on the sales. What does he earn if the total sale amounts to ₹ 48,000? What does the seller get?
Solution:
Rate of commission = 12%
Total sales = ₹ 48,000
Agent’s commission = \(\frac {12}{100}\) × 48,000
= ₹ 5,760
Amount received by the seller = Total sales – commission
= ₹ 8,000 – ₹ 5760
= ₹ 2,240

Question 2.
A salesman receives a 3% commission on sales up to ₹ 50,000 and a 4% commission on sales over ₹ 50,000. Find his total income on the sale of ₹ 2,00,000.
Solution:
Total sales = ₹ 2,00,000
Rate of commission upto ₹ 50,000 = 3%
= \(\frac{3}{100}\) × 50,000
= ₹ 1,500
Rate of commission on the sales over ₹ 50,000 = 4%
Sales over ₹ 50,000 is 2,00,000 – 50,000 = ₹ 1,50,000
Commission on sales over ₹ 50,000 = \(\frac{4}{100}\) × 1,50,000 = ₹ 6,000
His total income = ₹ 1,500 + ₹ 6,000 = ₹ 7,500

Question 3.
Ms. Saraswati was paid ₹ 88,000 as commission on the sale of computers at the rate of 12.5%. If the price of each computer was ₹ 32,000, how many computers did she sell?
Solution:
Total commission = ₹ 88,000
Rate of commission = 12.5%
Let the number of computers sold be x
since price of each computer = ₹ 32,000
Total sales = ₹ 32,000x
Total commission = 12.5% of total sales
88,000 = \(\frac{12.5}{100}\) × 32,000x
= \(\frac{125}{1000}\) × 32,000x
x = \(\frac{88,000}{125 \times 32}\)
x = 22

Question 4.
Anita is allowed 6.5% commission on the total sales made by her, plus, a bonus of \(\frac{1}{2}\)% on the sale over ₹ 20,000. If her total commission amounts to ₹ 3,400. Find the sales made by her.
Solution:
Let the total sales made by Anita be ₹ x
Rate of commission = 6.5% of total sales
= \(\frac{6.5}{100} \times x\)
= \(\frac{65 x}{1,000}\)
= \(\frac{13 x}{200}\)

Question 5.
Priya gets a salary of ₹ 15,000 per month and a commission of 8% on sales over ₹ 50,000. If she gets ₹ 17,400 in a certain month. Find the sales made by her in that month.
Solution:
Let the total sales made by Priya be ₹ x
Salary of Priya = ₹ 15,000
Commission = Total earning – salary
= ₹ 17,400 – ₹ 15,000
= ₹ 2,400
Commission = 8% on the sales over ₹ 50,000
2400 = \(\frac{8}{100}\) (x – 50000)
\(\frac{2,400 \times 100}{8}\) = x – 50,000
30,000 = x – 50,000
30,000 + 50,000 = x
∴ x = ₹ 80,000

Question 6.
The income of the broker remains unchanged though the rate of commission is increased from 4% to 5%. Find the percentage reduction in the value of the business.
Solution:
Let the original value of business be ₹ 100
Original rate of commission = 4%
∴ Original commission = \(\frac{4}{100}\) × 100 = ₹ 4
Let the new value of business be ₹ x
The new rate of commission = 5%
∴ New commission = \(\frac{5}{100}\) × x = \(\frac{x}{20}\)
Given, original income = New income
4 = \(\frac{x}{20}\)
∴ x = ₹ 80
Thus there is 20% reduction in the value of the business.

Question 7.
Mr. Pavan is paid a fixed weekly salary plus commission based on a percentage of sales made by him. If on the sale of ₹ 68,000 and ₹ 73,000 in two successive weeks, he received in all ₹ 9,880 and ₹ 10,180. Find his weekly salary and the rate of commission paid to him.
Solution:
Let the weekly salary of Mr. Pavan be ₹ x and the rate of commission paid to him be y%
Income = Weekly salary + Commission on the sales
∴ 9,880 = x + \(\frac{y}{100}\) × 68,000
i.e. 9,880 = x + 680y …….(1)
Also, 10,180 = x + \(\frac{y}{100}\) × 73,000
i.e 10,180 = x + 730y ………(2)
Subtracting (1) from (2), we get
50y = 300
∴ y = 6
Substituting y = 6 in equation (1)
9,880 = x + 680(6) ‘
∴ 9,880 – 4,080 = x
∴ x = 5,800
Weekly salary = ₹ 5,800
Rate of commission = 6%

Question 8.
Deepak’s salary was increased from ₹ 4,000 to ₹ 5,000. The sales being the same, due to a reduction in the rate of commission from 3% to 2%, his income remained unchanged. Find his sales.
Solution:
Let Deepak’s total sales be ₹ x
Original salary of Deepak = ₹ 4,000
Original rate of commission = 3%
His new salary = ₹ 5,000
New rate of commission = 2%
Original income = New income (given)
4000 + \(\frac{3 x}{100}\) = 5000 + \(\frac{2 x}{100}\)
\(\frac{3 x}{100}-\frac{2 x}{100}\) = 5,000 – 4,000
\(\frac{x}{100}\) = 1000
x = ₹ 1,00,000
∴ His total sales = ₹ 1,00,000

Question 9.
An agent is paid a commission of 7% on cash sales and 5% on credit sales made by him. If on the sale of ₹ 1,02,000 the agent claims a total commission of ₹ 6,420, find his cash sales and credit sales.
Solution:
Total Sales = ₹ 1,02,000
Let cash sales ₹ x
∴ Credit sales = ₹ (1,02,000 – x)
Agent’s commission on cash sales = 7%
= \(\frac{7}{100}\) × x
= \(\frac{7x}{100}\)
Commission on credit sales = 5%
= \(\frac{5}{100}\)(1,02,000 – x)
Given, Total commission = ₹ 6,420
∴ \(\frac{7x}{100}\) + \(\frac{5}{100}\)(1,02,000 – x) = 6420
∴ \(\frac{7x}{100}\) + 5100 – \(\frac{5x}{100}\) = 6,420
∴ \(\frac{2x}{100}\) = 6,420 – 5,100
∴ \(\frac{2x}{100}\) = 1320
∴ x = ₹ 66,000
∴ Cash sales = ₹ 66,000
∴ Credit sales = 1,02000 – 66,000 = ₹ 36,000

Question 10.
Three cars were sold through an agent for ₹ 2,40,000, ₹ 2,22,000 and ₹ 2,25,000 respectively. The rates of the commission were 17.5% on the first, 12.5% on the second. If the agent overall received 14% commission on the total sales, find the rate of commission paid on the third car.
Solution:
Total selling price of three cars = 2,40,000 + 2,22,000 + 2,25,000 = ₹ 6,87,000
Commission on total sales = 14%
= \(\frac{14}{100}\) × 6,87,000
= ₹ 96,180
Selling price of first car = ₹ 2,40,000
Rate of commission = 17.5% = \(\frac{17.5}{100}\) × 2,40,000
∴ Commission on first car = ₹ 42,000
Selling price of second car = ₹ 2,22,000
Rate of commission = 12.5% = \(\frac{12.5}{100}\) × 2,22,000
∴ Commission on second car = ₹ 27,750
Selling price of third car = ₹ 2,25,000
Let the rate of commission be x%
Commission on third car = \(\frac{x}{100}\) × 2,25,000
96,180 – (42,000 + 27,750) = \(\frac{x}{100}\) × 2,25,000
\(\frac{26,430 \times 100}{2,25,000}\) = x
∴ x = 11.75
∴ Rate of commission on the third car = 11.75%

Question 11.
Swatantra Distributors allows a 15% discount on the list price of the washing machines. Further 5% discount is giver for cash payment. Find the list price of the washing machine if it was sold for the net amount of ₹ 38,356.25.
Solution:
Let the list price of the washing machine be ₹ 100
Trade discount = 15% = \(\frac{15}{100}\) × 100 = ₹ 15
∴ Invoice price =100 – 15 = ₹ 85
Cash discount = 5% = \(\frac{5}{100}\) × 85 = ₹ 4.25
∴ Net price = 85 – 4.25 = ₹ 80.75
Thus if List price is 100 than Net price is 80.75
if List price is x than Net price is 38,356.25.
∴ x = \(\frac{38356.25 \times 100}{80.75}\)
∴ x = ₹ 47,500
The list price of the washing machine is ₹ 47,500

Question 12.
A bookseller received ₹ 1,530 as a 15% commission on the list price. Find the list price of the books.
Solution:
Let the list price of the books be ₹ x
Rate of commission = 15%
Book seller’s commission = ₹ 1,530
∴ \(\frac{15}{100}\) × x = 1,530
∴ x = \(\frac{1,530 \times 100}{15}\)
∴ x = ₹ 10,200

Question 13.
A retailer sold a suit for ₹ 8,832 after allowing an 8% discount on market price and a further 4% cash discount. If he made 38% profit, find the cost price and the market price of the suit.
Solution:
Let the marked price of the suit be ₹ 100
Trade discount = 8% = \(\frac{8}{100}\) × 100 = ₹ 8
Invoice price = 100 – 8 = ₹ 92
Cash discount = 4% = \(\frac{4}{100}\) × 92 = ₹ 3.68
∴ Net price = 92 – 3.68 = ₹ 88.32
Thus if list price is 100 then net price is 88.32, if list price is x then net price is 8,832
∴ x = \(\frac{8,832 \times 100}{88.32}\)
∴ x = ₹ 10,000
The retailer made 38% profit.
Let the CP of the suit be ₹ 100
∴ SP of the suit = 100 + 38 = ₹ 138
Thus if the SP of the suit is ₹ 138 then its CP is ₹ 100
If the SP of the suit is 88.32 then its
CP = \(\frac{88.32 \times 100}{138}\) = ₹ 6400

Question 14.
An agent charges 10% commission plus 2% delcredere. If he sells goods worth ₹ 37,200, find his total earnings.
Solution:
Total sales = ₹ 37,200
Rate of commission = 10%
Agents commission = \(\frac{4}{100}\) × 37200 = ₹ 3720
Rate of delcredere = 2%
Amount of delcredere = \(\frac{2}{100}\) × 37,200 = ₹ 744
Total earning of the agent = ₹ 3,720 + ₹ 744 = ₹ 4,464

Question 15.
A whole seller allows a 25% trade discount and 5% cash discount. What will be the net price of an article marked at ₹ 1600?
Solution:
Marked price of the article = ₹ 1,600
Trade discount = 25%
= \(\frac{25}{100}\) × 1,600
= ₹ 400
∴ Invoice price = 1,600 – 400 = ₹ 1,200
Cash discount = 5%
= \(\frac{5}{100}\) × 1,200
= ₹ 60
∴ Net price = 1,200 – 60 = ₹ 1,140

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 8 Probability Distributions Ex 8.4 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 8 Probability Distributions Ex 8.4

Question 1.
If X has Poisson distribution with m = 1, then find P(X ≤ 1) given e^-1 = 0.3678.
Solution:
∵ m = 1
∵ X follows Poisson Distribution

= e^-m × 1 + e^-m × 1
= e^-1 + e^-1
= 2 × e^-1
= 2 × 0.3678
= 0.7356

Question 2.
If X ~ P(\(\frac{1}{2}\)), then find P(X = 3) given e^-0.5 = 0.6065.
Solution:

Question 3.
If X has Poisson distribution with parameter m and P(X = 2) = P(X = 3), then find P(X ≥ 2). Use e^-3 = 0.0497
Solution:
∵ X follows Poisson Distribution

Question 4.
The number of complaints which a bank manager receives per day follows a Poisson distribution with parameter m = 4. Find the probability that the manager receives (i) only two complaints on a given day, (ii) at most two complaints on a given day. Use e^-4 = 0.0183.
Solution:
∵ m = 1
∵ X ~ P(m = 4)
∴ p(x) = \(\frac{e^{-m} \cdot m^{x}}{x !}\)
X = No. of complaints recieved
(i) P(Only two complaints on a given day)

(ii) P(Atmost two complaints on a given day)
P(X ≤ 2) = p(0) + p(1) + p(2)
= \(\frac{e^{-4} \times 4^{0}}{0 !}+\frac{e^{-4} \times 4^{1}}{1 !}\) + 0.1464
= e^-4 + e^-4 × 4 + 0.1464
= e^-4 [1 + 4] + 0.1464
= 0.0183 × 5 + 0.1464
= 0.0915 + 0.1464
= 0.2379

Question 5.
A car firm has 2 cars, which are hired out day by day. The number of cars hired on a day follows a Poisson distribution with a mean of 1.5. Find the probability that
(i) no car is used on a given day.
(ii) some demand is refused on a given day, given e^-1.5 = 0.2231.
Solution:
Let X = No. of demands for a car on any day
∴ No. of cars hired
n = 2
m = 1.5
∵ X ~ P(m = 1.5)

Question 6.
Defects on plywood sheets occur at random with an average of one defect per 50 sq. ft. Find the probability that such a sheet has (i) no defect, (ii) at least one defect. Use e^-1 = 0.3678.
Solution:
∵ X = No. of defects on a plywood sheet
∵ m = -1
∵ X ~ P(m = -1)
∴ p(x) = \(\frac{e^{-m} \cdot m^{x}}{x !}\)
(i) P(No defect)
P(X = 0) = \(\frac{e^{-1} \times 1^{0}}{0 !}\)
= e^-1
= 0.3678

(ii) P(At least one defect)
P(X ≥ 1) = 1 – P(X < 1)
= 1 – p(0)
= 1 – 0.3678
= 0.6322

Question 7.
It is known that, in a certain area of a large city, the average number of rats per bungalow is five. Assuming that the number of rats follows Poisson distribution, find the probability that a randomly selected bungalow has
(i) exactly 5 rats
(ii) more than 5 rats
(iii) between 5 and 7 rats, inclusive. Given e^-5 = 0.0067.
Solution:
X = No. of rats
∵ m = 5
∴ X ~ P(m = 5)
∴ p(x) = \(\frac{e^{-m} \cdot m^{x}}{x !}\)
(i) P(Exactly five rats)

(ii) P(More than five rats)
P(X > 5) = 1 – P(X ≤ 5)

(iii) P(between 5 and 7 rats, inclusive)
P(5 ≤ x ≤ 7) = p(5) + p(6) + p(7)

= 0.0067 × 3125 × 0.02
= 0.0067 × 62.5
= 0.42

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 8 Probability Distributions Ex 8.3 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 8 Probability Distributions Ex 8.3

Question 1.
A die is thrown 4 times. If ‘getting an odd number’ is a success, find the probability of (i) 2 successes (ii) at least 3 successes (iii) at most 2 successes.
Solution:
X: Getting an odd no.
p: Probability of getting an odd no.
A die is thrown 4 times
∴ n = 4
∵ p = \(\frac{3}{6}=\frac{1}{2}\)
∴ q = 1 – p = 1 – \(\frac{1}{2}\) = \(\frac{1}{2}\)
∵ X ~ B(3, \(\frac{1}{2}\))
∴ p(x) = \({ }^{n} \mathrm{C}_{x} p^{x} q^{n-x}\)
(i) P(Two Successes)

(ii) P(Atleast 3 Successes)

(iii) P(Atmost 2 Successes)

Question 2.
A pair of dice is thrown 3 times. If getting a doublet is considered a success, find the probability of two successes.
Solution:
n: No. of times die is thrown = 3
X: No. of doublets
p: Probability of getting doublets
Getting a doublet means, same no. is obtained on 2 throws of a die
There are 36 outcomes
No. of doublets are (1, 1), (2, 2), (3, 3), (4, 4), (5, 5), (6, 6)

Question 3.
There are 10% defective items in a large bulk of items. What is the probability that a sample of 4 items will include not more than one defective item?
Solution:
n: No of sample items = 4
X: No of defective items
p: Probability of getting defective items
∴ p = 0.1
∴ q = 1 – p = 1 – 0.1 = 0.9
X ~ B(4, 0.1)
∴ p(x) = \({ }^{n} \mathrm{C}_{x} p^{x} \mathrm{q}^{n-x}\)
P(Not include more than 1 defective)
P(X ≤ 1) = p(0) + p(1)
= ⁴C₀ (0.1)⁰ (0.9)⁴ + ⁴C₁ (0.1)¹ (0.9)^4-1
= 1 × 1 × (0.9)⁴ + 4 × 0.1 × (0.9)³
= (0.9)³ [0.9 + 0.4]
= (0.9)³ × 1.3
= 0.977

Question 4.
Five cards are drawn successively with replacement from a well-shuffled deck of 52 cards. Find the probability that (i) all the five cards are spades, (ii) only 3 cards are spades, (iii) none is a spade.
Solution:
X: No. of spade cards
Number of cards drawn
∴ n = 5
p: Probability of getting spade card

(i) P(All five cards are spades)

(ii) P(Only 3 cards are spades)

(iii) P(None is a spade)

Question 5.
The probability that a bulb produced by a factory will use fuse after 200 days of use is 0.2. Let X denote the number of bulbs (out of 5) that fuse after 200 days of use. Find the probability of (i) X = 0, (ii) X ≤ 1, (iii) X > 1, (iv) X ≥ 1.
Solution:
X : No. of bulbs fuse after 200 days of use
p : Probability of getting fuse bulbs
No. of bulbs in a sample
∴ n = 5
∴ p = 0.2
∴ q = 1 – p = 1 – 0.2 = 0.8
∵ X ~ B(5, 0.2)
∴ p(x) = \({ }^{n} \mathrm{C}_{x} p^{x} q^{n-x}\)
(i) P(X = 0) = ⁵C₀ (0.2)⁰ (0.8)^5-0
= 1 × 1 × (0.8)⁵
= (0.8)⁵

(ii) P(X ≤ 1) = p(0) + p(1)
= ⁵C₀ (0.2)⁰ (0.8)^5-0 + ⁵C₁ (0.2)¹ (0.8)^5-1
= 1 × 1 × (0.8)⁵ + 5 × 0.2 × (0.8)⁴
= (0.8)⁴ [0.8 + 1]
= 1.8 × (0.8)⁴

(iii) P(X > 1) = 1 – [p(0) + p(1)]
= 1 – 1.8 × (0.8)⁴

(iv) P(X ≥ 1) = 1 – p(0)
= 1 – (0.8)⁵

Question 6.
10 balls are marked with digits 0 to 9. If four balls are selected with replacement. What is the probability that none is marked 0?
Solution:
X : No. of balls drawn marked with the digit 0
n : No. of balls drawn
∴ n = 4
p : Probability of balls marked with 0.
∴ p = \(\frac{1}{10}\)
∴ q = 1 – p = 1 – \(\frac{1}{10}\) = \(\frac{9}{10}\)
p(x) = \({ }^{n} C_{x} p^{x} q^{n-x}\)
P(None of the ball is marked with digit 0)

Question 7.
In a multiple-choice test with three possible answers for each of the five questions, what is the probability of a candidate getting four or more correct answers by random choice?
Solution:
n: No. of Questions
∴ n = 5
X: No. of correct answers by guessing
p: Probability of getting correct answers

Question 8.
Find the probability of throwing at most 2 sixes in 6 throws of a single die.
Solution:
X : No. of sixes in 6 throws
n : No. of times dice thrown
∴ n = 6
p : Probability of getting six
∴ p = \(\frac{1}{6}\)
∴ q = 1 – p = 1 – \(\frac{1}{6}\) = \(\frac{5}{6}\)
∵ X ~ B(6, \(\frac{1}{6}\))
∴ p(x) = \({ }^{n} \mathrm{C}_{x} p^{x} q^{n-x}\)
P(At most 2 sixes)
P(X ≤ 2) = p(0) + p(1) + p(2)

Question 9.
Given that X ~ B(n, p),
(i) if n = 10 and p = 0.4, find E(X) and Var(X).
(ii) if p = 0.6 and E(X) = 6, find n and Var(X).
(iii) if n = 25, E(X) = 10, find p and Var(X).
(iv) if n = 10, E(X) = 8, find Var(X).
Solution:
∵ X ~ B (n, p), E(X) = np, V(X) = npq, q = 1 – p
(i) E(X) = np = 10 × 0.4 = 4
∵ q = 1 – p = 1 – 0.4 = 0.6
V(X) = npq = 10 × 0.4 × 0.6 = 2.4

(ii) ∵ p = 0.6
∴ q = 1 – p = 1 – 0.6 = 0.4
E(X) = np
∴ 6 = n × 0.6
∴ n = 10
∴ V(X) = npq = 10 × 0.6 × 0.4 = 2.4

(iii) E(X) = np
∴ 10 = 25 × p
∴ p = 0.4
∴ q = 1, p = 1 – 0.4 = 0.6
∴ S.D.(X) = √V(X)
= \(\sqrt{n p q}\)
= \(\sqrt{25 \times 0.4 \times 0.6}\)
= √6
= 2.4494

(iv) ∵ E(X) = np
∴ 8 = 10p
∴ p = 0.8
∴ q = 1 – p = 1 – 0.8 = 0.2
∵ V(X) = npq = 10 × 0.8 × 0.2 = 1.6