Important Questions

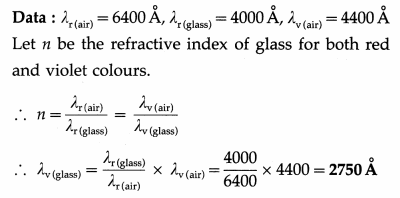
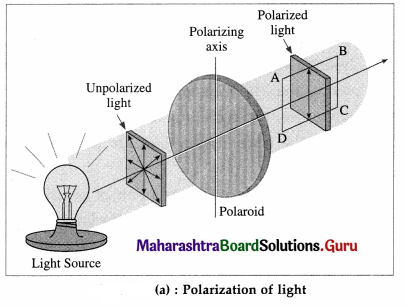
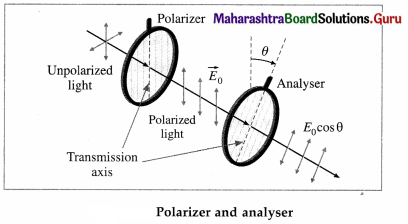
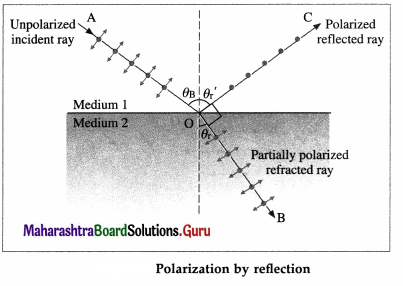
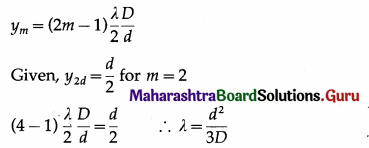
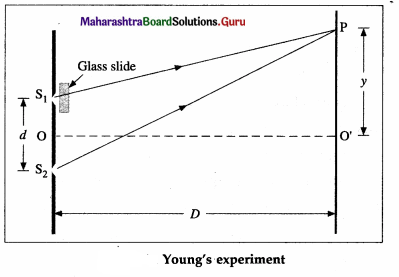
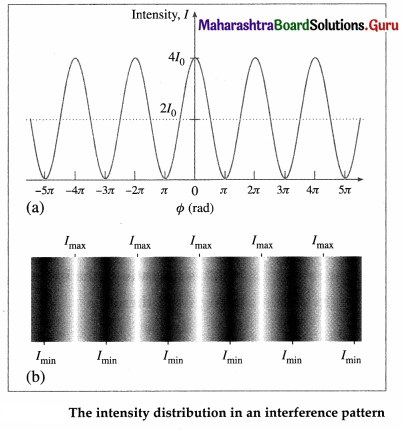
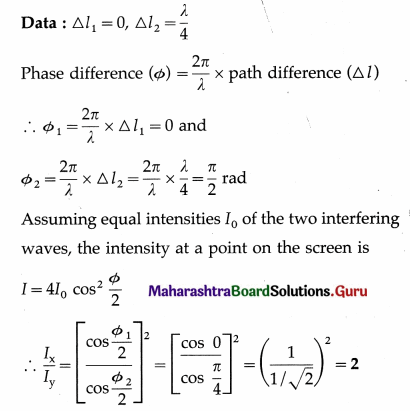
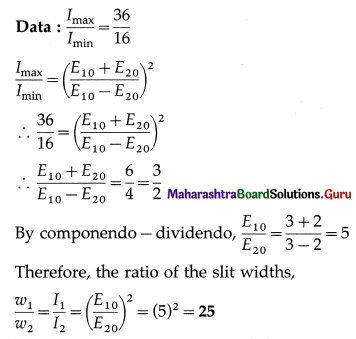
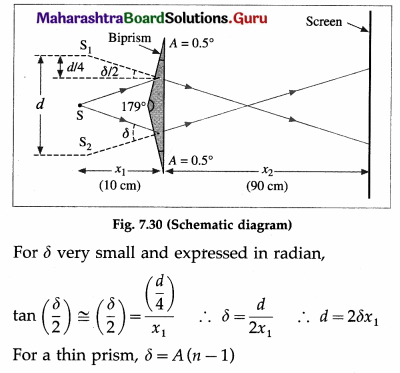
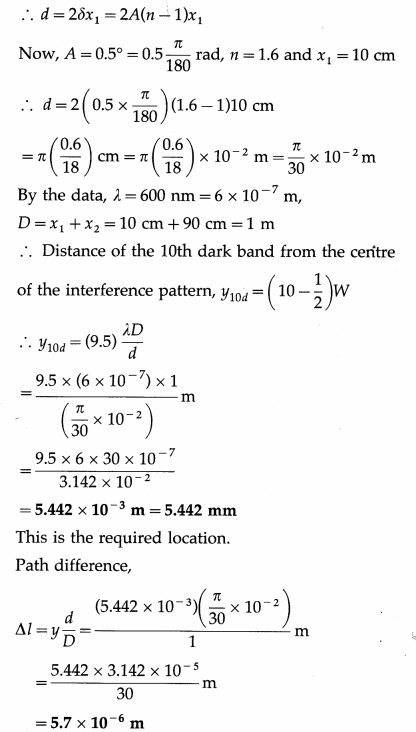
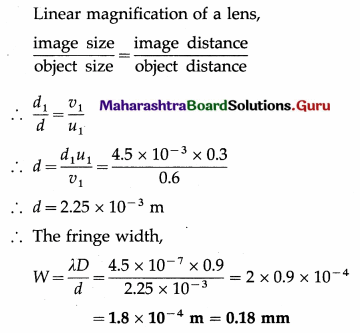
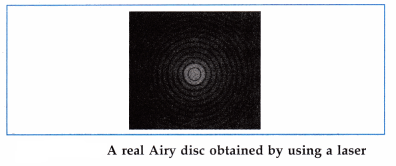
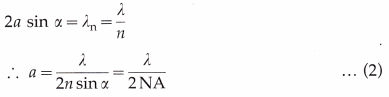
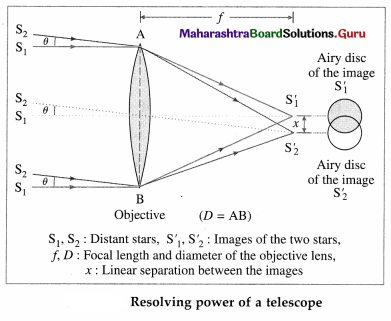
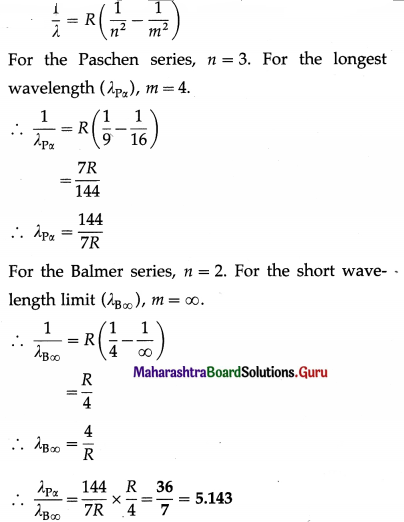
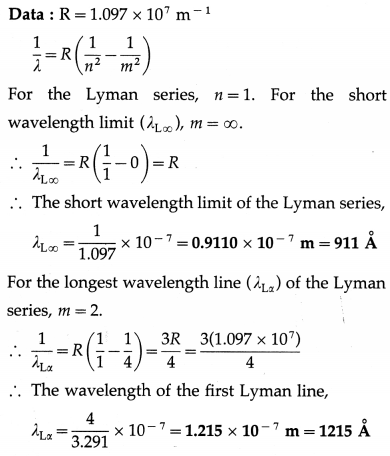
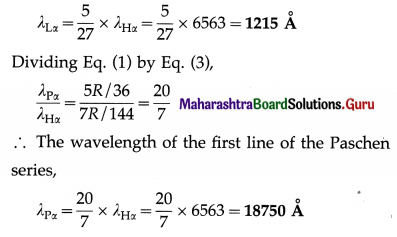
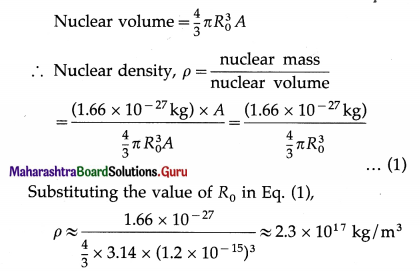
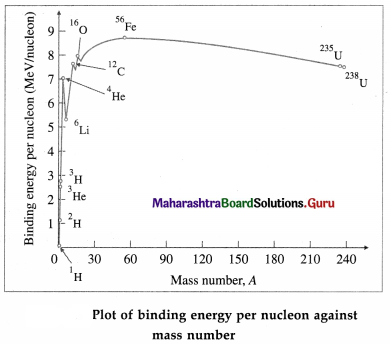
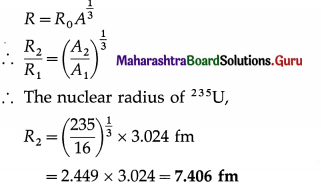
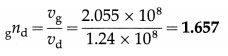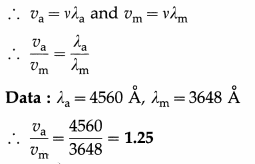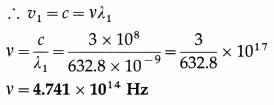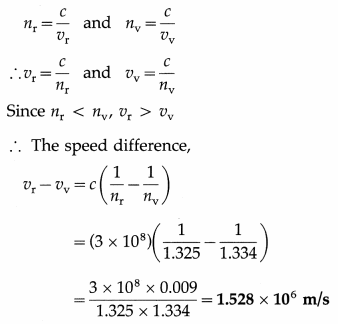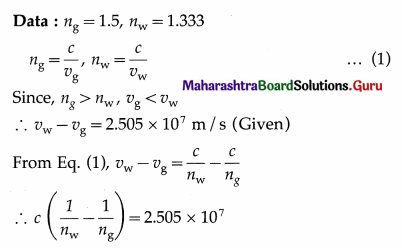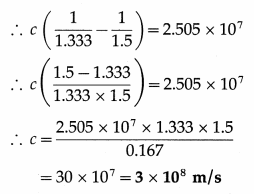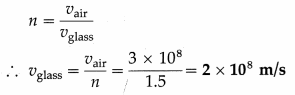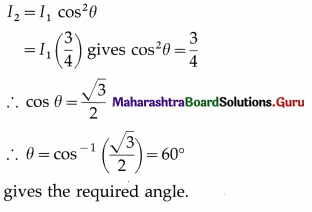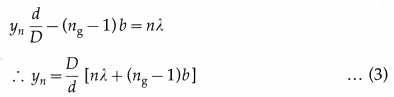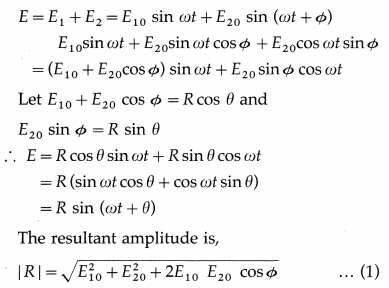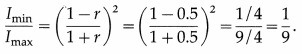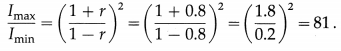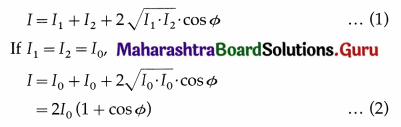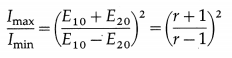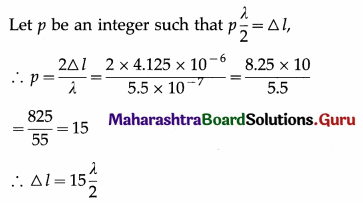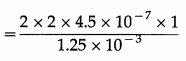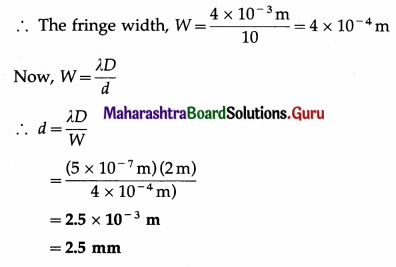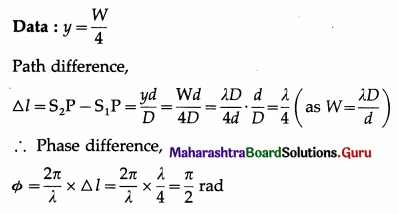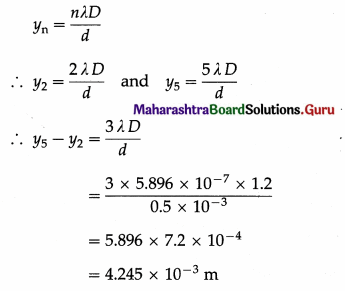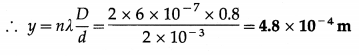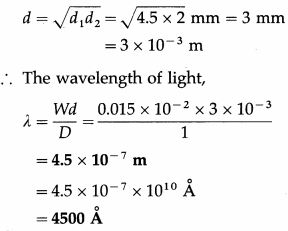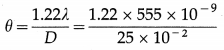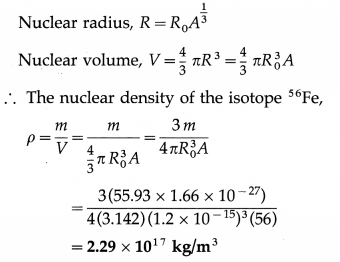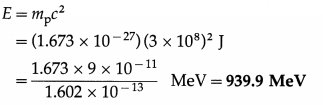Balbharti Maharashtra State Board 12th Physics Important Questions Chapter 6 Superposition of Waves Important Questions and Answers.

Maharashtra State Board 12th Physics Important Questions Chapter 6 Superposition of Waves

Question 1.
What is a progressive wave?
Answer:
A progressive wave or wave motion is a periodic or oscillatory disturbance in a medium or in a vacuum which is propagated without any damping and obstruction from one place to another at a finite speed.
[Note: A progressive wave is also called a traveling wave.]

Question 2.
Define :

1. transverse
2. longitudinal progressive wave.

Answer:

1. A progressive wave in which the vibration of the individual particles of the medium is perpendicular to the direction of propagation of the wave is called a transverse progressive wave.
2. A progressive wave in which the vibration of the individual particles of the medium is along the line of propagation of the wave is called a longitudinal progressive wave.

Question 3.
What is a mechanical wave ? Explain.
Answer:
A mechanical wave is a wave motion in a material medium.

Such a wave originates in the displacement of some portion of an elastic medium from its normal position. This causes the layers of matter to oscillate about their equilibrium positions. Because of the elastic properties of the material, the disturbance is transmitted from one layer to the next and so the waveform progresses through the medium.

Question 4.
What is a simple harmonic progressive wave ?
Answer:
A simple harmonic progressive wave is a periodic disturbance in a medium or in vacuum which propagates at a finite speed and in which the vibrations of the particles of the medium, as in a mechanical wave, or the oscillations of the electric and magnetic fields as in an electromagnetic wave are simple harmonic.

Question 5.
Define the following physical quantities related to a progressive wave :
(1) wave speed
(2) frequency (n)
(3) wavelength
(4) amplitude
(5) period
(6) wave number.
Answer:
(1) Wave speed : The distance covered by a progressive wave per unit time is called the wave speed.
(2) Frequency : The number of waves that pass per unit time across a given point of the medium is called the frequency of the wave.
[Note : It is equal to the number of vibrations per unit time made by a particle of the medium.]
(3) Wavelength : Wavelength is the distance between consecutive particles of the medium which are moving in exactly the same way at the same time and have the same displacement from their equilibrium positions.
[Note : Such particles are said to be in the same phase (the same state of vibration).]
(4) Amplitude : The magnitude of the maximum displacement of a particle of the medium from its equilibrium position is called the amplitude of the wave.
(5) Period : The time taken for a complete wave (one wavelength long) to pass a given point in the medium is called the period of the wave.
[Note : It is equal to the periodic time of the vibrational motion of a particle of the medium.]
(6) Wave number : The number of waves present per unit distance is called the wave number.

Question 6.
Write the equation of a progressive wave travelling along the positive x-direction.
Answer:
A progressive wave travelling along the positive x-direction is given by
y(x, t) = A sin (kx – ωt)
where A is the amplitude of the wave, k is the wave number and co is the angular frequency.
[Note : y(x, t) = A sin (kx – ωt), y(x, t) = A sin (ωt – kx), y(x, t) = A cos (kx – ωt), y(x, t) = A cos (ωt – kx) also represent a progressive wave travelling in the positive x-direction. Hence, any one of them can be used. y(x, t) can be written simply as y.]

Question 7.
Write the equation of a progressive wave travelling along the negative x-direction.
Answer:
A progressive wave travelling along the negative x-direction is given by y(x, t) = A sin (kx + ωt)
where A is the amplitude of the wave, k is the wave number and ω is the angular frequency.

Question 8.
Express the equation of a simple harmonic progressive wave in different forms.
Answer:
A simple progressive wave travelling along the positive x-direction is given by y = A sin (ωt – kx) … (1)
where A is the amplitude of the wave, k is the wave number and co is the angular frequency.

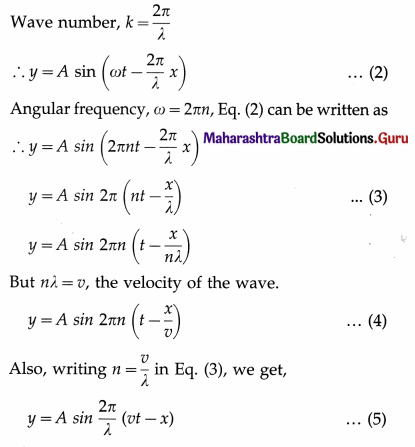
Frequency of vibrations, n = \(\frac{1}{T}\), Eq. (2) can be written as
y = A sin 2π(\(\overline{\mathrm{T}}\) – \(\frac{x}{\lambda}\))
Equations (1), (2), (3), (4), (5) and (6) are the different forms of the equation of a simple harmonic progressive wave.

Question 9.
A simple harmonic progressive wave is given by y = A sin (ωt – kx), where the symbols have their usual meaning. What is

1. the particle velocity at a point x and time t
2. the wave speed ?

Answer:

1. Particle velocity, \(\frac{d y}{d t}\) = ωA cos (ωt – kx)
2. Wave speed, v = \(\frac{\omega}{k}\).

Question 10.
A simple harmonic progressive wave has frequency 25 Hz and wavelength 4 m. If the phase difference between motions of two particles is (π/10) rad, what is the corresponding path difference?
Answer:
Path difference = \(\frac{\lambda}{2 \pi}\) × phase difference
= \(\frac{4 \mathrm{~m}}{2 \pi \mathrm{rad}}\) × \(\frac{\pi}{10}\) rad = 0.2 m

Question 11.
A simple harmonic progressive of frequency 100 Hz and wavelength 0.5 m travels through a medium. If the path difference between two points in the path of the wave is 0.1 m, what is the corresponding phase difference ?
Answer:
Phase difference = \(\frac{2 \pi}{\lambda}\) × path difference
= \(\frac{2 \pi}{0.5 \mathrm{~m}}\) × 0.1 m = 0.4 rad

Question 12.
The displacement of a particle of a medium when sound wave propagates is represented by y = A cos (ax + bt) where A, a and b are positive constants. The wave is reflected by an obstacle situated at x = 0.

1. What is the wavelength and frequency of the incident wave ?
2. Write the equation of the reflected wave.

Answer:
The incident sound wave is represented by y = A cos (ax + bt) where A, a and b are positive constants. The equation of a progressive wave of amplitude A, wavelength λ and frequency n = ω/2π, travelling along the negative direction of the x-axis is
y = A cos (ωt + kx),
where k = 2π/λ is the propagation constant. Comparing the two equations, ω = b and k = a.

1. Therefore, the wavelength of the incident wave,
   λ = \(\frac{2 \pi}{k}\) = \(\frac{2 \pi}{a}\) and its frequency, n = \(\frac{\omega}{2 \pi}\) = \(\frac{b}{2 \pi}\)
2. The equation of a progressive wave travelling along the positive direction of the x-axis is
   y = A cos (ωt – kx)
   ∴ The equation of the reflected wave is
   y = A cos (bt – ax).

Question 13.
Solve the following :

Question 1.
A simple harmonic progressive wave travels along a string. The time for a particle of the string to move from maximum displacement to zero is 0.004 s. What are the period and frequency of the wave ? If the wavelength is 1.2 m, what is the wave speed?
Solution :
Data : t = 0.004 s, λ = 1.2 m
The period T of the wave = the periodic time of the vibrational motion of the particle of the string = 4t = 4 × 0.004 = 0.016
∴ The frequency of the wave,
n = \(\frac{1}{T}\) = \(\frac{1}{0.016}\) = 62.5 Hz
The wave speed, v = nλ = 1.2 × 62.5 = 75 m/s

Question 2.
Write the equation of a simple harmonic progressive wave of amplitude 0.05 m and period 0.04 s travelling along the positive x-axis with a velocity of 12.5 m/s.
Solution:
Data : A = 0.05 m, T = 0.04 s, v = 12.5 m/s
∴ Equation of the wave travelling in the positive direction of the x-axis is

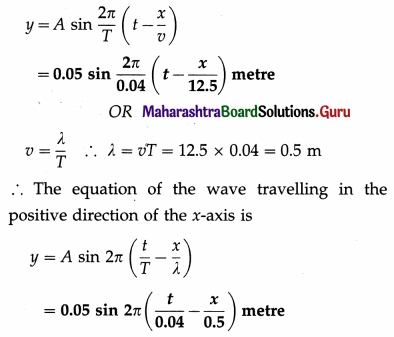
Question 3.
A simple harmonic progressive wave is given by the equation y = 0.1 sin 4π (50t – 0.1 x), in SI units. Find the amplitude, frequency, wavelength and speed of the wave.
Solution :
Data : y = 0.1 sin 4π (50t – 0.1 x)
= 0.1 sin 2π (100t – 0.2 x)
= 0.1 sin 2π( 100t – \(\frac{x}{5}\))
Let us compare this equation with that of a simple harmonic progressive wave.
∴ y = A sin 2π(nt – \(\frac{x}{2}\)) = 0.1 sin2π(100t – \(\frac{x}{5}\))
Comparing the quantities on both sides, we get,

1. amplitude (A) = 0.1 m
2. frequency (n) = 100 Hz
3. wavelength (λ) = 5 m
4. speed (v) = nλ = 100 × 5 = 500 m/s

Question 4.
The equation of a transverse wave on a stretched string is y = 0.2 sin 2π(\(\frac{t}{0.02}\) – \(\frac{x}{20}\)) where distances are in metre and time in second.
Find the

1. amplitude
2. frequency
3. speed of the wave.

Solution:
Let us compare the given equation with the equation of a simple harmonic progressive wave :

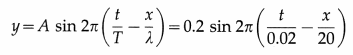
Comparing the quantities on both sides, we get,
A = 0.2 m, T = 0.02 s, λ = 20 m
∴

1. Amplitude (A) = 0.2 m
2. Frequency (n) = \(\frac{1}{T}\) = \(\frac{1}{0.02}\) = 50 Hz
3. Speed (v) = nλ – 50 × 20 = 1000 m/s

Question 5.
The equation of a simple harmonic progressive wave is y = 0.4 sin 100π (t – \(\frac{x}{5}\)) where all quantities are in SI units. Calculate the

1. wavelength
2. speed of the wave.

Solution:

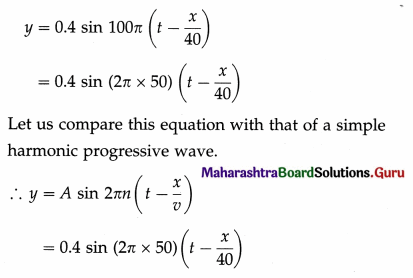
Comparing the quantities on both sides, we get,
A = 0.4 m, n = 50Hz and v = 40m/s
∴

1. Speed (v) =40 m/s
2. Wavelength (λ) = \(\frac{v}{n}\) = \(\frac{40}{50}\) = 0.8 m

Question 6.
The equation of a simple harmonic progressive wave is given by y = 0.05 sin π (20t – \(\frac{x}{6}\)), where all quantities are in SI units. Calculate the displacement of a particle at 5 m from the origin and at the instant 0.1 second. Also find the phase difference between two particles separated by 5 m.
Solution :
Data : x = 5 m, t = 0.1 s
(i) The displacement of the particle,

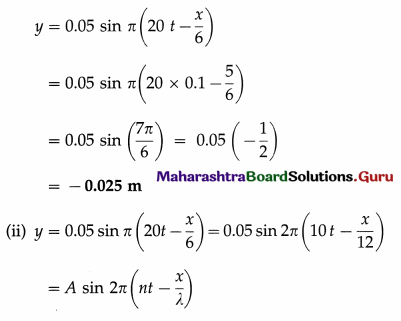
Comparing the two sides, we get, λ = 12 m
The phase difference between two points separated by x = 5 m is given by

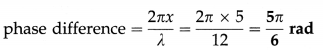
Question 7.
A sound wave of amplitude 0.2 cm, frequency 1000 Hz and wavelength 0.31 m is travelling in air. Calculate the displacement of the particle at 3.1 m from the origin after 1.004 s. What would be the phase difference for two positions of the vibrating particle after an interval of 0.001s?
Solution :
Data : A = 0.2 cm = 0.002 m, n = 1000 Hz, λ = 0.31 m, x = 3.1 m, t = 1.004 s, t₂ – t₁ = 0.001 s
(i) The displacement of the particle,
y = A sin 2π(nt – \(\frac{x}{\lambda}\))
= 0.002 sin 2π(1000 × 1.004 – \(\frac{3.1}{0.31}\))
= 0.002 sin 2π (1004 – 10)
= 0.002 sin 2π(994) = 0 [or y = 0 metre]

(ii) Phase difference = 2πn (t₂ – t₁)
= 2π × 1000 × 0.001 = 2π radians

Question 8.
The equation of a simple harmonic progressive wave is given by y = 4 sin π(\(\frac{t}{0.02}\) – \(\frac{x}{75}\)). Find the displacement and velocity of a particle at 50 cm from the origin and at 0.1 second. (All quantities are expressed in CGS units.)
Solution :

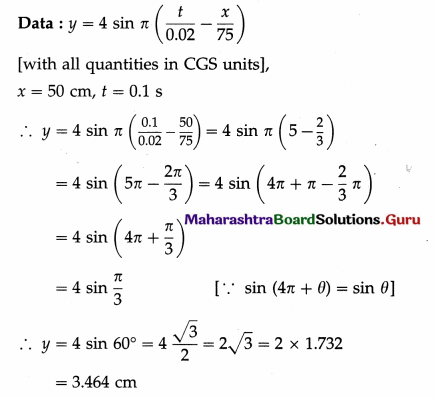
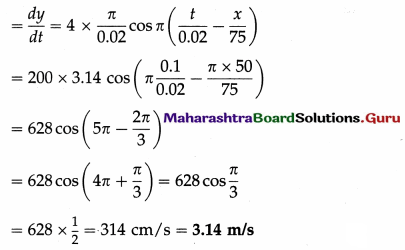
The displacement of the particle, y = 3.464 cm = 3.464 × 10^-2 m
The velocity of the particle,

Question 14.
What is meant by reflection of a wave ?
Answer:
When a wave travelling in a medium is incident on a boundary with another medium, a part of it returns into the original medium with a change in its direction of propagation while a part of it is transmitted into the second medium. The phenomenon in which a part of the wave is returned into the original medium with reduction in its intensity and energy is called reflection.

Question 15.
Explain the reflection of transverse waves at the surface of
(1) a denser medium
(2) a rarer medium.
OR
Explain the effect on the phase of transverse waves reflected from
(1) a denser medium
(2) a rarer medium.
Answer:
(1) Reflection of transverse waves from a denser medium : Suppose that a crest of a transverse wave travels along a string and is incident on the surface of a denser medium such as a rigid wall at point B, as shown in below figure. As the crest cannot travel further, it is reflected.

Since point B is fixed, its displacement is always zero. Therefore, the crest must be reflected in such a way, that the displacement at B due to the reflected

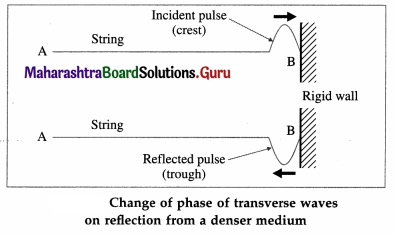
wave is exactly equal in magnitude and opposite in direction to that due to the incident wave. Therefore, the crest is reflected as a trough. Hence, there is a phase change of 180° or π radians when transverse waves are reflected from a denser medium.

(2) Reflection of transverse waves from a rarer medium : In this case, the particles of the medium are free to vibrate. Hence,

1. there is no change of phase
2. a crest is reflected as a crest and a trough is reflected as a trough.

Question 16.
Consider a heavy string X and a light string Y joined together at point O. Explain what happens when a wave pulse
(1) travelling from the string X reaches the junction O
(2) travelling from the string Y reaches the junction O.
Answer:
The tension in both strings is the same. Hence, the junction O is a discontinuity between string X of greater linear density than string Y because the wave speed is less on X than on Y.

(1) When a pulse travelling on the heavy string X reaches O, the light string Y gets pulled upwards. Thus the pulse, gets partially transmitted and partially reflected as a crest, as shown in below figure. However, the amplitude of transmitted pulse is greater than that of the reflected pulse.

(2) When a pulse travelling on a light string Y reaches O, the heavier string X pulled slightly upwards. Thus, the pulse is partly transmitted as a crest but the reflected part is inverted as a trough, as shown in below figure. Here, the amplitude of transmitted pulse is smaller than that of the reflected pulse.

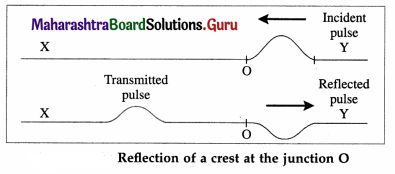
[Note : In either case, the relative heights of the reflected and transmitted pulses depend on the relative densities of the two strings. Obviously, if the strings are identical, there is no discontinuity at the boundary and no reflection takes place.]

Question 17.
A heavy string X is joined to a light string Y at point O. How will a pulse get reflected
(1) travelling on the string X towards O
(2) travelling on the string Y towards O ?
Answer:
(1) A pulse travelling on a heavy string will reflect without inversion at its boundary with a lighter string. Thus, a crest will reflect as a crest and a trough will reflect as a trough.

(2) When a pulse travelling on a light string encounters a boundary with a heavier string, the reflected pulse is inverted. Thus, a crest will reflect as a trough and vice versa.

Question 18.
Explain the reflection of sound waves (i.e., longitudinal waves) at the surface of
(1) a denser medium
(2) a rarer medium.
OR
Explain the effect on the phase of longitudinal waves reflected from
(1) a denser medium
(2) a rarer medium.
Answer:
(1) Reflection of a longitudinal wave from a denser medium : Consider a sound wave incident on a denser medium such as a rigid wall. When a compression is incident on the wall, the particles of air close to the wall are in a compressed state. To return to their normal condition, the particles begin to press in the opposite direction and therefore a compression gets reflected as a compression and a rarefaction is reflected as a rarefaction. However, the displacements of the particles in the reflected wave are opposite to their displacements in the incident wave, so that there is a change of phase of 180° or π radians.

(2) Reflection of a longitudinal wave from a rarer medium : When sound waves are reflected from

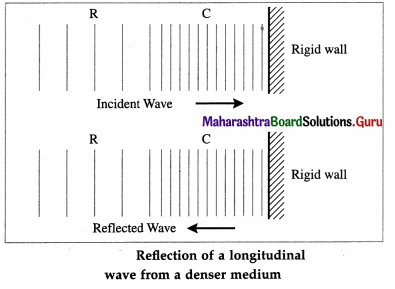
the surface of a rarer medium, there is no change of phase. Therefore, a compression is reflected as a rarefaction and vice versa. The reason is as follows :
When a compression is incident on the surface of a rarer medium, it can pass into that medium. This is because the particles of the rarer medium are free to move and they get compressed, leaving a rarefaction behind, which travels in the opposite direction. In a similar manner an incident rarefaction gets reflected as a compression.

Question 19.
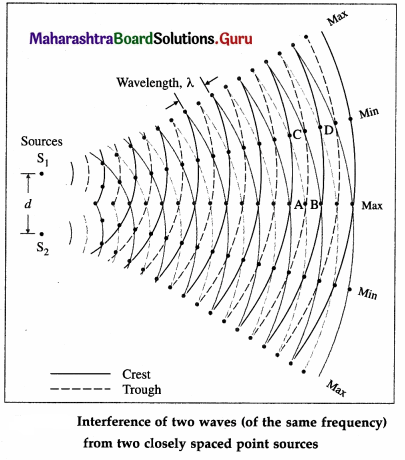
State the principle of superposition of waves.
Answer:
Principle of superposition of waves : The displacement of a particle at a given point in space and time due to the simultaneous influence of two or more waves is the vector sum of the displacements due to each wave acting independently.

Notes :

1. The principle of superposition is applicable to all types of waves.
2. The phenomena of interference, beats, formation of stationary waves, etc. are based on the principle of superposition of waves.

Question 20.
Explain the superposition of two wave pulses of equal amplitude and same phase moving towards each other.
OR
Explain constructive interference when two wave pulses of equal amplitude and same phase are superimposed.
Answer:
Consider two wave pulses of the same amplitude and phase moving towards each other, as shown in below figure.

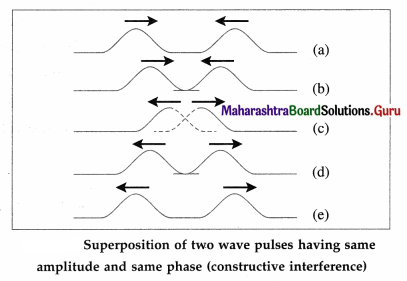
When the two pulses cross each other, stages (b) to (d), the resultant displacement is equal to the vector sum of the displacements due to the individual pulses (shown by dashed lutes). Therefore, the resultant displacement at that point becomes maximum. This phenomenon is called constructive interference. After crossing each other, both the pulses continue to propagate with their initial amplitude.

Question 21.
Explain the superposition of two wave pulses of equal amplitude and opposite phase moving towards each other.
OR
Explain destructive interference when two wave pulses of equal amplitude and opposite phase are superimposed.
Answer:
Consider two wave pulses of the same amplitude but opposite phase moving towards each other, as shown in below figure.

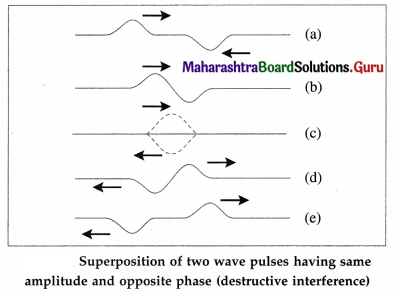
When the two pulses cross each other, stages (b) to (d), the resultant displacement is equal to the vector sum of the displacements due to the individual pulses (shown by dashed lines). Therefore, the resultant displacement at that point becomes minimum, equal to zero, because the individual pulses are exactly 180° out of phase.

[Notes: Music lovers often find many types of ambient sounds that interfere with the sounds coming through their headphones. Active noise-cancelling headphones not only block out some high frequency sound waves but also actively cancel out lower-frequency sound waves by destructive interference. They actually create sound waves with the same amplitude that mimic the incoming noise but with inverted phase (also known as antiphase), i.e., exactly 180° out of phase to the original noise. This inverted signal (in antiphase) is then amplified and a transducer creates a sound wave directly proportional to the amplitude of the original waveform, creating destructive interference. This effectively reduces the volume
of the perceivable noise.]

Question 22.
Derive an equation for the resultant wave produced due to superposition of two waves. Hence, state the expression for the amplitude of the resultant wave when two waves are
(1) in phase
(2) out of phase.
Answer:
Consider two waves of the same frequency, different amplitudes A₁ and A₂ and differing in phase by φ. Let these two waves interfere at x = 0.
The displacement of each wave at x = 0 are
y₁ = A₁ sin ωt
y₂ = A₂ sin (ωt + φ)

According to the principle of superposition of waves, the resultant displacement at that point is
y₁ = y₁ + y₂
= A₁ sin ωt + A₂ sin (ωt + φ)
Using the trigonometrical identity,
sin (C + D) = sin C cos D + cos C sin D,
y = A₁ sin ωt + A₂ ωt cos φ + A₂ cos ωt sin φ
y = (A₁ + A₂ cos φ) sin ωt + A₂ sin φ cos ωt … (1)
Let (A₁ + A₂ cos φ) = A cos θ … (2)
and A₂ sin φ = A sin θ … (3)
Substituting Eqs. (2) and (3) in EQ. (1), we get the equation of the resultant wave as
y = A cos θ sin ωt + A sin θ cos ωt = A sin (ωt + θ) … (4)
It has the same frequency as that of the interfering waves. The amplitude A of the resultant wave is given by squaring and adding Eqs. (2) and (3).

Thus, the amplitude of the resultant wave is maximum when the two interfering waves are in phase.

Case (2) : When the two interfering waves are out of phase, ivarphi = ipi. Then, the amplitude of the resultant wave is,

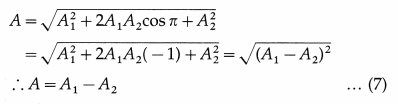
Thus, the amplitude of the resultant wave is minimum when the two interfering waves are in opposite phase.

Question 23.
What is the relation between the amplitude of a wave and its intensity?
Answer:
The intensity of a wave is proportional to the square of its amplitude.

Question 24.
Two interfering waves of the same frequency are out of phase but have different amplitudes A₁ and A₂. What can you say about the intensity of the resultant wave ?
Answer:
The two interfering waves are out of phase. Thus, the amplitude and hence the intensity of the resultant wave is minimum, I_min ∝ (A_min)² where (A_min)² = (A₁ – A₂)².

Question 25.
Two interfering waves of the same frequency are in phase but have different amplitudes A₁ and A₂. What can you say about the intensity of the resultant wave ?
Answer:
The two waves interfere in phase. Thus, the amplitude and hence the intensity of the resultant wave is maximum, I_max ∝ (A_min)² where (A_max)² = (A₁ + A₂)².

Question 26.
What is a stationary wave? Why is it called stationary?
Answer:
When two progressive waves having the same amplitude, wavelength and speed, travel through the same region of a medium in opposite directions, their super-position under certain conditions creates a stationary interference pattern called as a stationary or standing wave.

It is called stationary because the resultant harmonic disturbance of the particles does not travel in any direction and there is no transport of energy in the medium.

Question 27.
Define :

1. transverse stationary wave
2. longitudinal stationary wave.

Answer:

1. When two identical transverse progressive waves travelling in opposite directions along the same line superimpose, the resultant wave produced is called a transverse stationary wave.
2. When two identical longitudinal progressive waves superimpose, the resultant wave produced is called a longitudinal stationary wave.

Question 28.
When stationary waves of wavelength 40 cm are formed in a medium, what is the distance between

1. successive nodes
2. a node and the next antinode?

Answer:

1. 20 cm
2. 10 cm.

Question 29.
The equation of a stationary wave is y = 0.04 cos \(\frac{2 \pi x}{0.6}\) sin 2π (100t) with all quantities in SI units. What is the length of one loop ?
Answer:
Comparing the given equation with

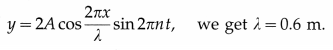
∴ Length of one loop = \(\frac{\lambda}{2}\) = 0.3 m.

Question 30.
What is the speed of the waves superposed ? For data, see Question 29.
Answer:
n = 100 Hz. v = nλ = 100 × 0.6 = 60 m/s.

Question 31.
What is the maximum speed of a particle at an antinode ? For data, see Question 29.
Answer:
v_max = 2A(2πn) = 0.04 × 2π × 100 = 8π m/s.

Question 32.
Distinguish between progressive waves and stationary waves.
Answer:
Progressive and stationary waves :

Progressive waves	Stationary waves
1. They are produced when a disturbance is created in the medium.	1. They are produced due to interference, under certain conditions, between two identical progressive waves travelling in opposite direc­tions.
2. They continuously travel away from the source and transport energy through the medium.	2. They do not move in any direction and hence do not transport energy through the medium.
3. Every particle vibrates with the same amplitude.	3. Amplitude of vibration is different for different par­ticles
4. Phase changes from particle to particle	4. All the particles in the same loop have the same phase, while the particles in adjac­ent loops are in opposite phase.
5. Every particle of the medium is set into vibrations	5. There are some particles of the medium which do not vibrate at all.

Question 33.
Solve the following :

Question 1.
A sound wave of frequency 1000 Hz and travelling with speed 340 m/s is reflected from the closed end of the tube. At what distance from that end will the adjacent node occur?
Solution :
Data : n = 1000 Hz, v = 340 m/s
The wavelength of the stationary wave set up in the tube, λ = \(\frac{v}{n}\).
The distance between successive nodes

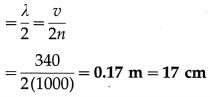
Question 2.
Two simple harmonic progressive waves are represented by y₁ = 2 sin 2π(100 t – \(\frac{x}{60}\)) cm and y₂ = 2 sin 2π(100t + \(\frac{x}{60}\)) cm. The waves combine to form a stationary wave. Find

1. the amplitude at an antinode
2. the distance between adjacent node and antinode
3. the loop length
4. the wave speed.

Solution :

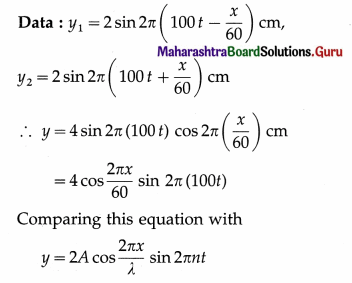
we get, λ = 60 cm and n = 100 Hz. Therefore,

1. the amplitude at an antinode, | 2A | = 4 cm
2. the distance between adjacent node and antinode \(\frac{\lambda}{4}\) = \(\frac{60}{4}\) = 15 cm
3. the loop length = \(\frac{\lambda}{2}\) = \(\frac{60}{2}\) cm = 30 cm
4. the wave speed = nλ= 100 × 60 = 6000 cm/s

Question 3.
The equation of a standing wave is given by y = 0.02 cos (πx) sin (100 πt) m. Find the amplitude of either wave interfering, wavelength, time period, frequency and wave speed of interfering waves.
Solution :
Data : y = 0.02 cos (πx) sin (100 πt) m
Comparing this equation with
y = 2A cos\(\left(\frac{2 \pi x}{\lambda}\right)\) sin (2π nt)
we get for either interfering waves,

1. the amplitude, | A | = \(\frac{0.02}{2}\) = 0.01 m
2. the wavelength, λ = 2 m
3. the time period, T = \(\frac{1}{n}\) = \(\frac{1}{50}\)s = 0.02 s
4. the frequency, n = 50 Hz
5. the wave speed = nλ = 50 × 2 = 100 m/s

Question 34.
Explain
(1) free vibrations
(2) forced vibrations.
Answer:
(1) Free vibrations : A body capable of vibrations is said to perform free vibrations when it is disturbed from its equilibrium position and left to itself.

In the absence of dissipative forces such as friction due to surrounding air and internal forces, the total energy and hence the amplitude of vibrations of the body remains constant. The frequencies of the free vibrations of a body are called its natural frequencies and depend on the body itself.

In the absence of a maintaining force, in practice, the total energy and hence the amplitude decreases due to dissipative forces and the vibration is said to be damped. The frequency of damped vibrations is less than the natural frequency.

(2) Forced vibrations : The vibrations of a body in response to an external periodic force are called forced vibrations.

The external force supplies the necessary energy to make up for the dissipative losses. The frequency of the forced vibrations is equal to the frequency of the external periodic force.

The amplitude of the forced vibrations depends upon the mass of the vibrating body, the amplitude of the external force, the difference between the natural frequency and the frequency of the periodic force, and the extent of damping.

Question 35.
Define resonance.
Answer:
Resonance : If a body is made to vibrate by an external periodic force, whose frequency is equal to the natural frequency (or nearly so) of the body, the body vibrates with maximum amplitude. This phenomenon is called resonance.
The corresponding frequency is called the resonant frequency.

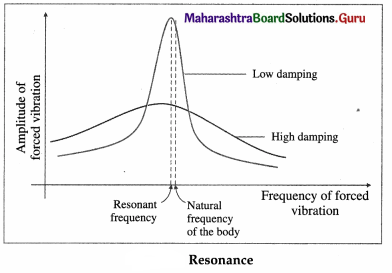
For low damping, the amplitude of vibrations has a sharp maximum at resonance, as shown in above figure. The flatter curve without a pronounced maximum is for high damping.

Question 36.
Distinguish between
(1) free vibrations and resonance
(2) forced vibrations and resonance (Two points of distinction).
Answer:
(1) Free vibrations and resonance:

Free vibrations	Resonance
1. These are produced when a body is distributed from its equilibrium position and released.	1. It is produced by forced vibrations when the external periodic force has the frequency equal to the natural frequency (or nearly so) of the body.
2. The energy of the body remains constant in the absence of dissipative forces.	2. Energy is supplied con­tinuously by the external periodic force to com­pensate the loss of en­ergy due to the dissi­pative forces.

(2) Forced vibrations and resonance :

Forced vibrations	Resonance
1. These are produced by an external periodic force of any frequency.	1. It is produced by an exter­nal periodic force whose frequency is equal to the natural frequency (or nearly so) of the body.
2. The frequency of vibrations is, in general, different from the natural frequency of the body	2. The frequency of vibrations is the same (or nearly so) as the natural frequency of the body.
3. The amplitude of vibrations is usually very small.	3. The amplitude of vibrations is large.
4. Vibrations stop as soon as the external force is removed.	4. Vibrations continue for rela­tively longer time after the external force is removed.

Question 37.
Give any two applications of resonance.
Answer:

1. A radio or TV receiver set is tuned to the frequency of the desired broadcast station by adjusting the resonant frequency of its electrical oscillator circuit.
2. The speed of sound at room temperature can be determined by making the air column of a resonance tube resonate with a vibrating tuning fork of known frequency.
3. The frequency of a tuning fork can be determined by making a sonometer wire, of known mass per unit length and under known tension, resonate with the vibrating fork.
4. The amplitude of the oscillations of a child on a swing is increased by pushing with a frequency equal to the natural frequency of the swing.

Question 38.
Give any two disadvantages of resonance.
Answer:

1. A column of soldiers marching in regular step on a narrow and structurally flexible bridge can set it into dangerously large amplitude oscillations. The bridge may even collapse at the resonance.
2. Structural resonance of a suspension bridge induced by the winds can lead to its catastrophic collapse. Several early suspension bridges were destroyed by structural resonance induced by modest winds.
3. Vibrations of a motor or engine can induce resonant vibrations in its supporting structures if their natural frequency is close to that of the vibrations of the engine. A common example is the rattling sound of a bus body when the engine is left idling. Vibrations in an aircraft are caused by the engine and the aerodynamic effects. The vibrations cause metal fatigue, especially in the fuselage, wings and tail, and eventually lead to metal fracture.
4. Every ship has a natural period of rolling (side to side oscillation about an axis along its length). If the ship encounters a series of waves such that the wave period matches the rolling, it will have no time righting itself before the next wave strikes. Resonant conditions can occur when the combination of wave period, vessel speed and heading with respect to the waves lead to an encounter close to the natural roll period of the vessel. This situation, if not corrected, can lead to severe rolling, with roll angle exceeding 15°.
   Large containerships are particularly vulnerable to rolling. Possible consequences are loss of containers, machinery failure, structural damage and even capsizing of the ship. The speed and direction of the ship can be changed to avoid the consequences of synchronous rolling.

Question 39.
What are overtones? What is the meaning of first overtone ?
Answer:
The higher allowed harmonics above the first harmonic or fundamental are called overtones.
The first overtone is the higher allowed harmonic immediately above the first harmonic.

Question 40.
Distinguish between harmonics and overtones.
Answer:

Harmonics	Overtones
1. The lowest allowed frequency of vibration (fundamental) of a bounded medium and all its integral multiples    are called harmonics.	1. The higher allowed frequencies of vibration above the fundamental are called overtones.
2. The lowest allowed frequency (fundamental), n, is called the first harmonic. The second harmonic is In, third harmonic is 3n, … and so on.	2. Above the fundamental, the first allowed frequency is called the first overtone which may be either the second or third harmonic. Depending on the system, the pth overtone corresponds to (p + 1)th or (2p + 1)th harmonic.

Question 41.
What is end correction ? State the cause of end correction. How is it estimated ?
Answer:
When sound waves are sent down the air column in a narrow closed or open pipe, they are reflected at the ends-without phase reversal at an open end and with a phase reversal at a closed end. Interference between the incident and reflected waves under appropriate conditions sets up stationary waves in the air column. Thus, the stationary waves have an antinode at an open end.

However, because air molecules in the plane of an open end are not free to move in all directions, reflection of the longitudinal waves takes place slightly beyond the rim of the pipe at an open end. The distance of the antinode from the open end of the pipe is called end correction. According to Reynolds, the distance of the antinode from the rim is approximately 30% of the inner diameter of a cylindrical pipe. This distance must be taken into account in accurate determination of the wavelength of sound. Hence, this distance is called the end correction.

Therefore, if d is the inner diameter of a cylindrical pipe, an end correction e = 0.3 d for each open end must be added to the measured length of the pipe. If l is the measured length, the effective length of the air column in the case of a pipe closed at one end is l + 0.3d, while that for a pipe open at both ends is l + 0.6 d.

Question 42.
What are the frequencies of the notes produced in an open and closed pipes in terms of the length of pipe L and velocity of waves v?.
Answer:
The frequencies of all the harmonics present in an open pipe are

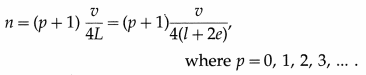
The frequencies of the odd harmonics present in a closed pipe are

where p = 0,1, 2, 3, … .

Question 43.
State the factors on which the fundamental frequency of air column in a pipe depends.
Answer:

1. Speed of sound in air
2. length of the pipe
3. diameter of the pipe.

Question 44.
The fundamental frequency of air column in a pipe closed at one end is 300 Hz. What is the frequency of the

1. second overtone
2. third harmonic ? (Ignore the end correction.)

Answer:
Closed pipe.

1. Second harmonic = fifth harmonic = 5 × 300 = 1500 Hz
2. Third harmonic = 3 × 300 = 900 Hz.

Question 45.
The fundamental frequency of air column in a pipe open at both ends is 200 Hz. What is the frequency of the

1. second harmonic
2. third overtone ? (Ignore the end correction.)

Answer:
Open pipe.

1. Second harmonic = 2 × 200 = 400 Hz
2. Third overtone = fourth harmonic
   = 4 × 200 = 800 Hz.

Question 46.
Stationary waves in the air column inside a pipe of length 50 cm and closed at one end have three nodes and three antinodes. What is the wavelength ?
Answer:
Here, L = 5\(\frac{\lambda}{4}\)
∴ Wavelength λ = \(\frac{4 L}{5}\) = \(\frac{4 \times 50 \mathrm{~cm}}{5}\) = 40 cm

Question 47.
Show that the fundamental frequency of vibration of the air column in a pipe open at both ends is double that of a pipe of the same length and closed at one end.
Answer:
Let L_O and L_C be the lengths of a pipe open at both ends and a pipe closed at one end, respectively. Let n_O and n_C be their corresponding fundamental frequencies. Then, ignoring the end corrections,
n_O = \(\frac{v}{2 L_{\mathrm{O}}}\) and n_C = \(\frac{v}{4 L_{\mathrm{C}}}\)
where v is the speed of the sound in air.
Given that L_O = L_C = L (say),
n_O = \(\frac{v}{2 L}\) and n_O = \(\frac{v}{4 L}\)
∴ n_O = 2\(\left(\frac{v}{4 L}\right)\) = \(2 n_{\mathrm{C}}\)

Question 48.
Prove that a pipe of length 2L open at both ends has the same fundamental frequency as a pipe of length L closed at one end.
Answer:
Let L_O and L_C be the lengths of a pipe open at both ends and a pipe closed at one end, respectively. Let n_O and n_C be their corresponding fundamental frequencies. Then, ignoring the end corrections,
n_O = \(\frac{v}{2 L_{\mathrm{O}}}\) and n_C = \(\frac{v}{4 L_{\mathrm{C}}}\)
where v is the speed of the sound in air.
Given that L_C = L and L_O = 2L,
n_O = \(\frac{v}{4 L}\) and n_C = \(\frac{v}{4 L}\) ∴ n_O = n_C

Question 49.
A pipe open at both ends has the fundamental frequency n. If the pipe is immersed vertically in water up to half its length, what would be the fundamental frequency of the resulting air column?
Answer:
Let L be the length of the pipe open at both ends whose fundamental frequency is n. Then, ignoring the end correction, n = \(\frac{v}{2 L}\)
where v is the speed of sound in air.

When the pipe is immersed vertically in water up to half its length, it becomes a pipe closed at one end with an air column of length L’ = L / 2. Then, its fundamental frequency n’ is

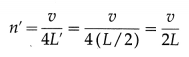
which is equal to n, the fundamental frequency of the open pipe.

Question 50.
The pth overtone of an organ pipe open at both ends has a frequency n. When one end of the pipe is closed, the qth overtone has a frequency N. Show that N = \(\frac{(2 q+1) n}{2(p+1)}\).
Answer:
Let L be the length of an organ pipe and v be the speed of sound in air.
When the pipe has both its ends open, the frequency of the pth overtone, ignoring the end correction, is (p + 1)\(\frac{v}{2 L}\).

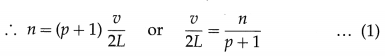
When one end of the pipe is closed, the frequency of the qth overtone of a pipe of length L and closed at one end is (2q + 1)\(\frac{v}{4 L}\)

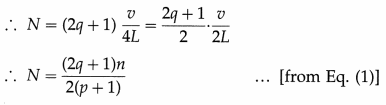
which is the required expression.

Question 51.
Two organ pipes open at both ends and of same length but different radii (or diameters) produce sounds of different frequencies. Why?
Answer:
Stationary waves formed in the air column of a pipe open at both ends have an antinode at each end. These antinodes are slightly beyond the rim of the pipe and an end correction of approximately 30% of the inner diameter must be added to the measured length of the air column for each open end.

Suppose two organ pipes, open at both ends and of same length 1, have inner diameters d₁, and d₂. Then, the effective lengths of the air columns are respectively L₁ = l + 0.6dt and L₂ = l + 0.6d₂. The fundamental frequencies of the corresponding air columns are

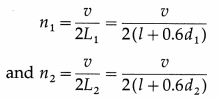
where v is the speed of sound in air. Thus, if d₁ and d₂ are different, n₁ and n₂ will also be different.

Question 52.
Two organ pipes closed at one end have the same diameters but different lengths. Show that the end correction at each end is e = \(\frac{n_{1} l_{1}-n_{2} l_{2}}{n_{2}-n_{1}}\), where the symbols have their usual meanings.
Answer:
Suppose two organ pipes, closed at one end and of the same inner diameter d, have lengths l₁ and l₂.
Then, the effective lengths of the air columns are respectively
L₁ = l₁ + 0.3d and L₂ = l₂ + e = l₂ + 0.3d
where e = 0.3d is the end correction for the open end.
The fundamental frequencies of the corresponding air columns are

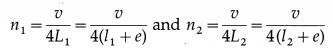
where v j the speed of sound in air.
∴ v = 4n₁(l₁ + e) = 4n₁(l₂ + e)
∴ n₁I₁ + n₁e = n₂I₂ + n₂e
∴ n₁I₁ – n₂I₂ = (n₂ – n₁)e
∴ e = \(\frac{n_{1} l_{1}-n_{2} l_{2}}{n_{2}-n_{1}}\)
which is the required expression.

Question 53.
State any two limitations of end correction.
Answer:
Limitations of end correction :

1. Inner diameter of the tube must be uniform.
2. Effects of air flow and temperature outside the tube are ignored.
3. The prongs of the tuning fork should be perpendicular to the air column in the tube, with their tips at the centre of the tube and a small distance above the rim of the tube.

Question 54.
A tuning fork is in resonance with a closed pipe. But the same tuning fork cannot be in resonance with an open pipe of the same length. Why ?
Answer:
For the same length of air column, and the same speed of sound, the fundamental frequency of the air column in a closed pipe is half that in an open pipe. Hence, a tuning fork in unison with the air column in a closed pipe cannot be in unison with the air column of the same length in an open pipe.

Question 55.
Solve the following :

Question 1.
Calculate the fundamental frequency of an air column in a tube of length 25 cm closed at one end, if the speed of sound in air is 350 m/s.
Answer:
v = 350 m/s, L = 25 cm = 0.25 m
∴ The fundamental frequency of the air column is
n = \(\frac{v}{4 L}\) = \([\frac{350}{4 \times 0.25}]\) = 350 Hz

Question 2.
A pipe which is open at both ends is 47 cm long and has an inner diameter of 5 cm. If the speed of sound in air is 348 m/s, calculate the fundamental frequency of the air column in that pipe.
Solution :
Data : l = 47 cm = 0.47 m, d = 5 cm = 0.05 m, v = 348 m/s
e = 0.3 d = 0.3 × 0.05 = 0.015 m
As the tube is open at both ends, the corrected length (L) is
L = l + 2e = 0.47 + (2 × 0.015) = 0.5 m
∴ The fundamental frequency of the air column is
n = \(\frac{v}{2 L}\) = \(\frac{348}{2 \times 0.5}\) = 348 Hz

Question 3.
A tube open at both ends is 47 cm long. Calculate the fundamental frequency of the air column. (Ignore the end correction. Speed of sound in air is 3.3 × 10² m/s.)
Solution :
Data : L = 47 cm = 0.47 m, v = 330 m/s.
The fundamental frequency of the air column,
n = \(\frac{v}{2 L}\) = \(\frac{330}{2 \times 0.47}\) = \(\frac{165}{0.47}\) = 351.1 Hz

Question 4.
The speed of sound in air at room temperature is 350 m/s. A pipe is 35 cm in length. Find the frequency of the third overtone in the pipe when it is
(i) closed at one end
(ii) open at both ends. Ignore the end correction.
Solution :
Data : v = 350 m/s, L = 35 cm = 35 × 10^-2 m
(i) For a pipe closed at one end, the fundamental frequency is

As only odd harmonics are present in this case, the frequency of pth overtone is n_p = (p × 2 + 1) n_C
∴ The frequency of the 3rd overtone is
n₃ = (3 × 2 + 1)n_C = 7n_C = 7 × 250 = 1750 Hz

(ii) For a pipe open at both ends, the fundamental frequency is

In this case, all harmonics are present.
∴ The frequency of the pth overtone is
n_p = (p + 1) n_O
∴ The frequency of the 3rd overtone is n₃ = (3 + 1) n_O = 4n_O = 4 × 500 = 2000 Hz

Question 5.
Find the frequency of the fifth overtone of an air column vibrating in a pipe closed at one end. The length of the pipe is 42.10 cm and the speed of sound in air at room temperature is 350 m/s. The inner diameter of the pipe is 3.5 cm.
Solution :
Data : L = 42.10 cm = 0.4210 m, v = 350 m/s, d = 3.5 cm = 3.5 × 10^-2 m, pipe closed at one end

Question 6.
Determine the length of a pipe open at both ends which is in unison with a pipe of length 20 cm closed at one end, in the fundamental mode. Ignore the end correction.
Solution :
Let n_O and L_O be the fundamental frequency and length respectively, of the pipe open at both ends and let n_C and L_C be the corresponding values for a pipe closed at one end. If v is the speed of sound in air,
n_O = \(\frac{v}{2 L_{\mathrm{O}}}\) and n_C = \(\frac{v}{4 L_{\mathrm{C}}}\)
As the air columns in the two pipes vibrate in unison,
n_O = n_C
∴ 2L_O = 4L_C ∴ L_O = 2L_C
But L_C = 20cm, ∴ L_O = 2 × 20 = 40 cm

Question 7.
The fundamental frequency of an air column in a pipe closed at one end is in unison with the third overtone of an open pipe. Calculate the ratio of the lengths of their air columns.
Solution :
Pipe closed at one end : fundamental frequency,
n_C = \(\frac{v}{4 L_{C}}\)
column is 51.8 cm.
n_O = \(\frac{v}{2 L_{\mathrm{O}}}\)
In this case, the frequency of the third over-tone = \(\frac{4 v}{2 L_{\mathrm{O}}}\) = \(\frac{2 v}{L_{\mathrm{O}}}\)
By the data, \(\frac{v}{4 L_{C}}\) = \(\frac{2 v}{L_{\mathrm{O}}}\)
∴ \(\frac{L_{\mathrm{O}}}{L_{\mathrm{C}}}\) = 8 or \(\frac{L_{\mathrm{C}}}{L_{\mathrm{O}}}\) = \(\frac{1}{8}\)

Question 8.
The consecutive overtones of an air column closed at one end are 405 Hz and 675 Hz respectively. Find the fundamental frequency of a similar air column but open at both ends.
Solution:
For the air column closed at one end, let
L = the length of the air column,
n_C = the fundamental frequency,
n_q, n_{q + 1} = the frequencies of the qth and (q + 1)th overtones, where q = 1, 2, 3, …
Since only odd harmonics are present as overtones, n_q = (2q + 1)n_C and n_{q + 1} = [2(q + 1) + n]n_C
= (2q + 3)n_C
Data : n_q = 405 Hz, n_q+1 = 675 Hz

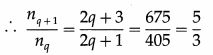
Solving for q, q = 1
Therefore, the two given frequencies correspond to the first and second overtones, i.e., the third and fifth harmonics.
∴ 3n_C = 405 Hz
∴ n_C = 135 Hz
This gives the fundamental frequency of the air column closed at one end.
The fundamental frequency (n_O) of an air column of same length but open at both ends is double that of the air column closed at one end (ignoring the end correction).
∴ n_O = 2n_C = 2 × 135 = 270 Hz
This gives the fundamental frequency of a similar air column but open at both ends.
Solution :
Data : n = 480 Hz, l₁ = 16.8 cm, l₂ = 51.8 cm
(1) The speed of sound in air is v = 2n (l₂ – l₁)
= 2 × 480 × (51.8 – 16.8) = 33600 cm/s = 336 m/s

(2) Let λ be the wavelength of sound waves and e be the end correction.
For the first resonance, l₁ + e = \(\frac{\lambda}{4}\) … (1)
For the second resonance, l₂ + e = \(\frac{3 \lambda}{4}\) … (2)
From Eq. (1), λ = 4(l₁ + e).
Substituting this value in Eq. (2), we get,

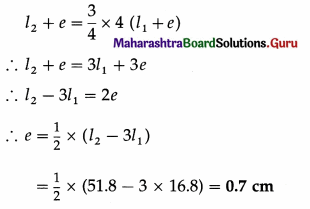
Question 9.
In a resonance tube experiment, a tuning fork v resonates with an air column 10 cm long and again resonates when it is 32.2 cm long. Calculate the wavelength of the wave and the end correction.
Solution:

This gives the wavelength of the wave. We have,

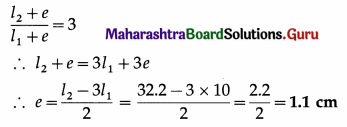
This gives the end correction.

Question 10.
The length of air column in a resonance tube for fundamental mode is 16 cm and that for second resonance is 50.25 cm. Find the end correction.
Solution:
Data: l₁ = 16 cm, l₂ = 50.25 cm
End correction,

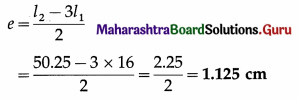
Question 56.
State the formula for the speed of transverse waves on a stretched string (or wire). Hence obtain an expression for the fundamental frequency of the vibrating string (or wire).
Answer:

1. If a string (or a wire) stretched between two rigid supports is plucked at some point, the disturbance produced travels along the string in the form of transverse waves. If T is the tension applied to the string and m is the mass per unit length (i.e., linear density) of the string, the speed of the transverse waves is
   v = \(\sqrt{\frac{T}{m}}\)
2. The transverse waves moving along the string are reflected from the supports. The reflected waves interfere and under certain conditions set up stationary waves in the string. At each support, a node is formed.
3. The possible or allowed stationary waves are subject to the two boundary conditions that there must be a node at each fixed end of the string. The different ways in which the string can then vibrate are called its modes of vibration.
4. In the simplest mode of vibration, there are only two nodes (N), one at each end and an antinode (A) is formed midway between them, as shown in
   
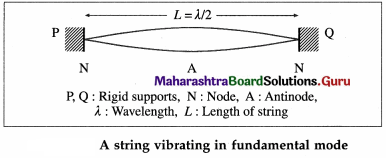
   In this case, the distance between successive nodes is equal to the length of the string (L) and is equal to λ/2, where λ is the wavelength.
   ∴ L = \(\frac{\lambda}{2}\) or λ = 2L
   The frequency of vibrations is n = \(\frac{v}{\lambda}\)
   Substituting v = \(\sqrt{\frac{T}{m}}\) and λ = 2L in this relation, we get,
   n = \(\frac{1}{2 L} \sqrt{\frac{T}{m}}\)
   This is the lowest frequency of the stationary waves on a stretched string and is called the fundamental frequency.

Question 57.
What is the minimum frequency with which a stretched string of length L, linear density m can vibrate under tension T?
Answer:
The minimum frequency with which a stretched string of length L, linear density m can vibrate under tension T is the fundamental frequency given by n = \(\frac{1}{2 L} \sqrt{\frac{T}{m}}\).

Question 58.
The mass per unit length of a wire is 1 × 10^-4 kg/m and the tension in the wire is 25 N. What is the speed of the transverse waves on the wire?
Answer:

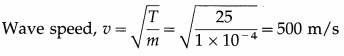
Question 59.
With neat labelled diagrams, explain the three lowest modes of vibration of a string stretched between rigid supports.
Answer:
Consider a string of linear density m stretched between two rigid supports a distance L apart. Let T be the tension in the string.

Stationary waves set up on the string are subjected to two boundary conditions : the displacement y = 0 at x = 0 and at x = L at all times. That is, there must be a node at each fixed end. These conditions limit the possible modes of vibration to only a discrete set of frequencies such that there are an integral number of loops p between the two fixed ends.

Since, the length of one loop (the distance between consecutive nodes) corresponds to half a wavelength (λ),

In the simplest mode of vibration, only one loop (p = 1) is formed. The corresponding lowest allowed frequency, n, given by
n = \(\frac{1}{2 L} \sqrt{\frac{T}{m}}\) … (4)
is called the fundamental frequency or the first harmonic. The possible modes of vibration with frequencies higher than the fundamental are called the overtones.

In the first overtone, two loops are formed (p = 2). Its frequency,
n₁ = \(\frac{2}{L} \sqrt{\frac{T}{m}}\) = 2n … (5)
is twice the fundamental and is, therefore, the second harmonic.

In the second overtone, three loops are formed (p = 3). Its frequency,
n₂ = \(\frac{3}{2 L} \sqrt{\frac{T}{m}}\) = 3n … (6)
is the third harmonic.

Question 60.
The speed of transverse waves on a vibrating string is 50 m/s. If the length of the string is 0.25 m, what is the fundamental frequency of vibration?
Answer:
Fundamental frequency, n = \(\frac{v}{(2 L)}\) = \(\frac{50}{(2 \times 0.25)}\)
= 100 Hz

Question 61.
State and explain the laws of vibrating strings.
Answer:
The fundamental frequency of vibration of a stretched string or wire of uniform cross section is 1
n = \(\frac{1}{2 L} \sqrt{\frac{T}{m}}\)
where L is the vibrating length, m the mass per unit length (linear density) of the string and T the tension in the string. From the above expression, we can state the following three laws of vibrating strings.

(1) Law of length : The fundamental frequency of vibrations of a stretched string is inversely proportional to its vibrating length, if the tension and mass per unit length are kept constant. If T and m are constant,
n ∝ \(\frac{1}{L}\) or nL = constant.

(2) Law of tension : The fundamental frequency of vibrations of a stretched string is directly proportional to the square root of the applied tension, if the length and mass per unit length are kept constant. If L and m are constant,
n ∝ or \(\sqrt{T}\) or n²/T = constant.

(3) Law of mass (or law of linear density) : The fundamental frequency of vibrations of a stretched string is inversely proportional to the square root of its mass per unit length, if the length and tension are kept constant. If L and T are constant,
n ∝ \(\frac{1}{\sqrt{m}}\) or n²m = constant.

Question 62.
How does the fundamental frequency of a vibrating string depend on the radius of cross section of the string and the mass density of the material of the string ?
Answer:
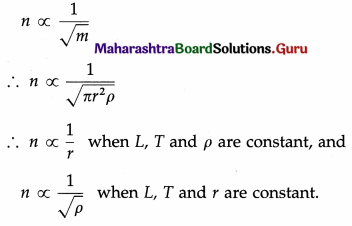
Consider a string stretched between two rigid supports a distance L apart. Let T be the tension in the string, r be its radius of cross section and p be the mass density of its material. Then, the mass of the string M = (πr²L)p, so that its linear density, i.e., mass per unit length, m = M/L = πr²p.

According to the law of mass of a vibrating string, the fundamental frequency (n) is inversely proportional to the square root of its linear density, when T and L are constant.

Question 63.
A string/wire is stretched between two rigid supports. State any two factors on which the fundamental frequency of the string/wire depends.
Answer:

1. Tension in the string/wire
2. length of the string/wire (or radius or mass per unit length or mass density of the material of the string/wire)

Question 64.
Why are strings of different thicknesses and materials used in a sitar or some other such instruments?
Answer:
The linear density of a string, m = πr²p, where r is the radius of cross section of the string and p is the mass density of its material. By the law of mass of a vibrating string, the frequency of vibrations n of the string is inversely proportional to \(\sqrt{m}\). Therefore, n ∝ \(\frac{1}{r}\) and n ∝\(\frac{1}{\sqrt{\rho}}\). Hence, the strings of different thicknesses and materials in a stringed musical instrument like sitar can be set to different scales.

Question 65.
If Y and ρ are Young’s modulus and mass density of the material of a stretched wire of length L, show that the fundamental frequency of vibration of the wire is n = \(\frac{1}{2 L} \sqrt{\frac{Y \Delta L}{\rho L}}\), where ∆L is the elastic extension of the wire.
Answer:
Consider a wire stretched between two rigid supports a distance L apart. Let T ≡ the tension in the wire, r ≡ the radius of cross section of the wire,
Y, ρ ≡ Young’s modulus and mass density of the material of the wire,
M,m ≡ the mass and linear density of the wire.
Then, M = (πr²L)ρ and m = \(\frac{M}{L}\) = πr²ρ … (1)
The stress in the wire = \(\frac{T}{\pi r^{2}}\)
∴ \(\frac{T}{m}\) = \(\frac{T}{\pi r^{2} \rho}\) = \(\frac{\text { stress }}{\rho}\) … (2)
The The fundamental frequency of vibration of the wire,

if ∆L is the elastic extension of the wire under tension T, strain = ∆L/L.

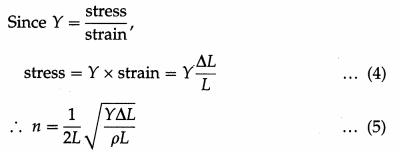
which is the required expression.

Question 66.
What is the linear density of a wire of mass density 8 g/cm³ and cross-sectional radius 0.05 mm ?
Answer:
Linear density of the wire = nr2p
= π(5 × 10^-3)² (8) = 2π × 10^-4 g/cm.

Question 67.
Stationary waves on a vibrating string of length 30 cm has three loops. What is the wavelength ?
Answer:

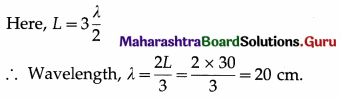
Question 68.
Write a short note on sonometer.
Answer:
A sonometer consists of a uniform wire stretched over a rectangular sounding box, and passes over

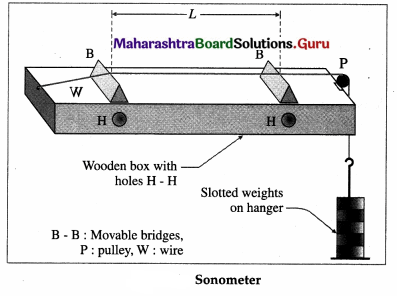
two movable bridges (or knife edges) and a pulley, in above figure. It works on the phenomenon of resonance. The tension in the wire is adjusted by adding weights to the hanger attached to the free end of the wire. The length of the wire between the movable bridges, L, is adjusted to vibrate in unison with a given timing fork either by beats method or by paper-rider method. L is called the vibrating length. First, the vibrating length is set to minimum and then gradually increased in small steps. In the beats method, the wire and the tuning fork are simultaneously set into vibrations for each vibrating length. Beats can be heard when the two frequencies are very close. Then, a finer adjustment of the wire is needed so that no beats are heard. This is when the two are in unison.

For the paper-rider method, a small light paper in the form of Λ is placed on the wire at its centre. The stem of the vibrating timing fork is gently pressed on the sonometer box. The vibrating length is gradually increased from minimum till the paper rider vibrates and thrown off. Because, when the wire resonates with the tuning fork at its lowest fundamental mode, the wire vibrates with maximum amplitude and the centre of the wire is an antinode. Hence, the paper rider is thrown off.

A sonometer is used to determine the frequency of a tuning fork and to verify the laws of vibrating strings.

Question 69.
Explain the use of a sonometer to verify
(i) the law of length
(ii) the law of tension
(iii) the law of linear density.
Answer:
(i) Verification of law of length : According to this law, n ∝ \(\frac{1}{L}\), if T and m are constant. To verify this
law, the sonometer wire of given linear density m is kept under constant tension T. The length of the wire is adjusted for the wire to vibrate in unison with tuning forks of different frequencies n₁, n₂, n₃,… . Let L₁, L₂, L₃, … be the corresponding resonating lengths of the wire. It is found that, within experimental errors, n₁L₁ = n₂L₂ = n₃L₃ = …. This implies that the product, nL = constant, which vertifies the law of length.

(ii) Verification of law of tension : According to this law, n ∝ \(\sqrt{T}\), if L and m are constant. To verify this law, the vibrating length L of the sonometer wire of given linear density m is kept constant.

A set of tuning forks of different frequencies is used. The tension in the wire is adjusted for the wire to vibrate in unison with tuning forks of different frequencies n₁, n₂, n₃,….. . T₁, T₂, T₃, ….. corresponding tensions. It is found that, within experimental errors, \(\frac{n_{1}}{\sqrt{T_{1}}}\) = \(\frac{n_{2}}{\sqrt{T_{2}}}\) = \(\frac{n_{3}}{\sqrt{T_{3}}}\) = ….. This implies \(\frac{n_{1}}{\sqrt{T}}\) = constant which verifies the law of tension.

(iii) Verification of linear density : According to this law, n ∝ \(\frac{1}{\sqrt{m}}\), if T and L are constant. To verify this law, two wires having different linear densities m₁ and m₂ are kept under constant tension T.
A tuning fork of frequency n is used. The lengths of the wires are adjusted for the wires to vibrate in unison with the tuning fork. Let L₁ and L₂ be the corresponding resonating lengths of the wires. It is found that, within experimental errors, \(L_{1} \sqrt{m_{1}}\) = \(L_{2} \sqrt{m_{2}}\). This implies \(L \sqrt{m}\) = constant. According to the law of length of a vibrating string, n ∝ \(\frac{1}{L}\).
∴ n ∝ \(\frac{1}{\sqrt{m}}\) which verifies the law of linear density.

Question 70.
A stretched sonometer wire vibrates at 256 Hz. If its length is increased by 10%, without changing the tension in the wire, what will be the frequency of the wire ?
Answer:
L₂ = 1.1 L₁ = \(\frac{n_{2}}{n_{1}}\) = \(\frac{L_{2}}{L_{1}}\) ∴ \(\frac{n_{2}}{256}\) = 1.1
∴ n₂ = 256 × 1.1 = 281.6 Hz

Question 71.
Solve the following :

Question 1.
Find the speed of a transverse wave along a string of linear density 3.6 × 10^-3 kg/m, when it is under a tension of 1.8 kg wt.
Solution :
Data : m = 3.6 × 10^-3 kg/m, g = 9.8 m/s²
∴ T = 1.8 kg wt = 1.8 × 9.8N
The speed of transverse waves along the string is

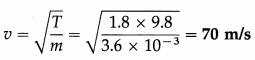
Question 2.
The speed of a transverse wave along a uniform metal wire, when it is under a tension of 1000 g wt, is 68 m/s. If the density of the metal is 7900 kg/m³, find the area of cross section of the wire.
Solution :
Data : g = 9.8 m/s², T = 1000 g wt = 1 kg wt = 9.8 N,
V = 68 m/s, ρ = 7900 kg/m³

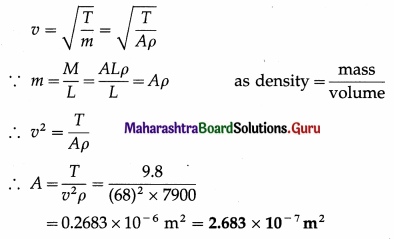
This gives the area of cross section of the wire.

Question 3.
A transverse wave is produced on a string 0.7 m long and fixed at its ends. Find the speed of the wave when it vibrates emitting the second overtone of frequency 300 Hz.
Solution :
Data : L = 0.7 m, n (second overtone) = 300 Hz
In this case, three loops are formed on the string.
∴ L = 3\(\frac{\lambda}{2}\)
∴ λ = \(\frac{2 L}{3}\) ∴ v = nλ = \(\frac{2}{3}\)nL
∴ v = \(\frac{2}{3}\) × 300 × 0.7 = 140 m/s
This gives the speed of the wave.

Question 4.
A uniform wire under tension is fixed at its ends. If the ratio of the tension in the wire to the square of its length is 360 dyn/cm² and the fundamental frequency of vibration of the wire is 300 Hz, find its linear density.
Solution :

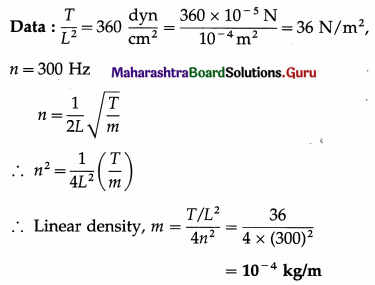
Question 5.
A metal wire of length 20 cm and diameter 0.2 mm is stretched by a load of 2 kg wt. If the density of the material of the wire is 7.8 g/cm³, find the fundamental frequency of vibration of the wire.
Solution :
Data : L = 20 cm = 0.2 m, d = 0.2 mm, g = 9.8 m/s², T = 2 kg wt = 2 × 9.8 N = 19.6 N, ρ = 7.8 g/cm³ = 7.8 × 10³ kg/m³
∴ r = \(\frac{d}{2}\) = 0.1 mm = 0.1 × 10^-3 m
n = \(\frac{1}{2 L} \sqrt{\frac{T}{m}}\) and m = πr²ρ

The fundamental frequency of vibration of the wire is 707 Hz.

Question 6.
Two wires, each 1 m long and of the same diameter, have densities 8 × 10³ kg/m³ and 2 × 10³ kg/m³ and are stretched by tensions 196 N and 49 N, respectively. Compare their fundamental frequencies.
Solution :
Data : L₁ = L₂ = 1 m, d₁ = d₂ (∴ r₁ = r₂), ρ₁ = 8 × 10³ kg/m³, ρ₂ = 2 × 10³ kg/m³, T₁ = 196 N, T₂ = 49N

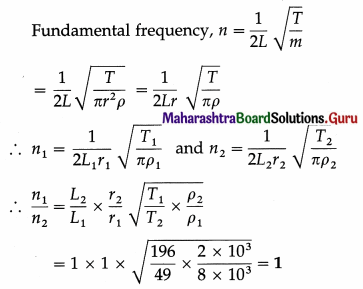
∴ Their fundamental frequencies are the same.

Question 7.
A uniform wire of length 49 cm and linear density 4 × 10^-4 kg/m is subjected to a tension of 28 N. Determine its frequency for

1. the fundamental mode
2. the second harmonic
3. the third overtone.

Solution :
Data : L = 49 cm = 0.49 m, T = 28 N, m = 4 × 10^-4 kg/m

1. Fundamental frequency :
   
2. Second harmonic : 2n = 2 × 270 = 540 Hz
3. Third overtone : 4n = 4 × 270 = 1080 Hz

Question 8.
Two wires of the same material, having lengths in the ratio 2 : 1 and diameters in the ratio 3 :1 are subjected to tensions in the ratio 1 : 4. Find the ratio of their fundamental frequencies.
Solution :
Let n₁, L₁, T₁, m₁, r₁ and ρ₁ be the fundamental frequency, vibrating length, tension, mass per unit length, radius and density of the first wire respectively and let n₂, L₂, T₂, m₂, r₂ and ρ₂ be the corresponding quantities of the second wire.

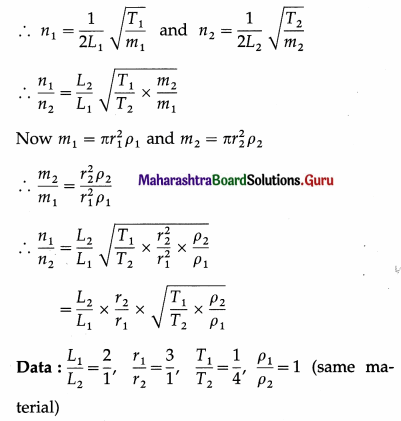
Substituting these values in the above relation,
\(\frac{n_{1}}{n_{2}}\) = \(\frac{1}{2}\) × \(\frac{1}{3}\) × \(\sqrt{\frac{1}{4} \times 1}\) = \(\frac{1}{12}\)

Question 9.
A wire under a certain tension, gives a note of fundamental frequency 320 Hz. When the tension is changed, the frequency of the fundamental note rises to 480 Hz. Compare the tensions in the wire.
Solution :
Data : n₁ = 320 Hz, n₂ = 480 Hz Fundamental frequency is n = \(\frac{1}{2 L} \sqrt{\frac{T}{m}}\)
As the length (L) of the wire and its mass per unit length (m) are kept constant,
n ∝ \(\sqrt{T}\)
∴\(\frac{n_{1}}{n_{2}}\) = \(\sqrt{\frac{T_{1}}{T_{2}}}\)
∴ The ratio of the tensions in the wire,
\(\frac{T_{1}}{T_{2}}\) = \(\left(\frac{n_{1}}{n_{2}}\right)^{2}\) = \(\left(\frac{320}{480}\right)^{2}\) = \(\left(\frac{2}{3}\right)^{2}\) = \(\frac{4}{9}\)

Question 10.
A transverse wave is produced on a stretched string 0.9 m long and fixed at its ends. Find the speed of the transverse wave, when the string vibrates while emitting second overtone of frequency 324 Hz.
Solution :
Data : L = 0.9 m, n (second overtone) = 324 Hz
For the second overtone of a vibrating string, λ = \(\frac{2}{3}\)L
The speed of the transverse wave formed on the string, v = nλ
∴ v = n × \(\frac{2}{3}\)L = 324 × \(\frac{2}{3}\) × 0.9
= 324 × 0.6 = 194.4 m/s

Question 11.
The string of a guitar is 80 cm long and has a fundamental frequency of 112 Hz. If a guitarist wishes to produce a frequency of 160 Hz, where should the person press the string ?
Solution :
Data : L₁ = 80 cm n₁ = 112 Hz, n₂ = 160 Hz
According to the law of length, n₁L₁ = n₂L₂.
∴ The vibrating length to produce the fundamental frequency of 160 Hz,
L₂ = \(\frac{n_{1} L_{1}}{n_{2}}\) = \(\frac{112(80)}{160}\) = 56 cm

Question 12.
What should be the tension applied to a wire of length 1 m and mass 10 grams, if it has to vibrate with the fundamental frequency of 50 Hz ?
Solution :
Data : L = 1 m, mass of the wire = 10 g = 0.01 kg, n = 50 Hz
∴ m = mass per unit length of the wire = \(\frac{0.01}{1}\)
= 0.01 kg/m
n = \(\frac{1}{2 L} \sqrt{\frac{T}{m}}\)
∴ Tension = T = 4n²L²m
= 40(50)²(1)²(0.01)
= 4 × 2500 × 0.01 = 100 N

Question 13.
A sonometer wire of length 1 m weighing 2 g is subjected to a suitable tension. The vibrating length of the wire in unison with a tuning fork of frequency 512 Hz is 12 cm. If the vibrating length of the wire in unison with another fork under the same conditions is 12.8 cm, find the frequency of this fork.
Solution :
Data : L₁ = 12 cm, n₁ = 512 Hz, L₂ = 12.8 cm n₁L₁ = n₂L₂
∴ The frequency of the second fork,
n₂ = \(\frac{n_{1} L_{1}}{L_{2}}\) = \(\frac{512(12)}{12.8}\) = 480 Hz

Question 14.
A stretched sonometer wire emits a fundamental note of frequency 256 Hz. Keeping the stretching force constant and reducing the length of the wire by 10 cm, the frequency becomes 320 Hz. Calculate the original length of the wire.
Solution:
Data : n₁ = 256 Hz, T and m constant, L₂ = L₁ – 10 cm, n₂ = 320 Hz

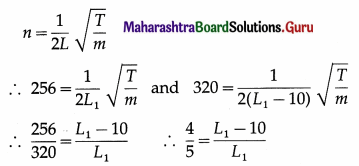
∴ 5L₁ – 50 = 4L₁
∴ L₁ = 50 cm = 0.5 m

Alternative method :
Since T and m are constant, nL = constant.
∴ n₁L₁ = n₂L₂ ∴ \(\frac{L_{1}}{L_{2}}\) = \(\frac{n_{2}}{n_{1}}\)
∴ \(\frac{L_{1}}{L_{1}-10}\) = \(\frac{320}{256}\) = \(\frac{20}{16}\) = \(\frac{5}{4}\)
∴ 4L₁ = 5L₁ – 50
∴ 5L₁ – 4L₁ = 50
∴L₁ = 50cm = 0.5 m

Question 15.
A sonometer wire, 36 cm long, vibrates with a frequency of 280 Hz in the fundamental mode when it is under a tension of 24.5 N. Calculate the linear density of the material of the wire.
Solution :
Data: L = 36 cm = 0.36 m, n = 280 Hz, T = 24.5 N,

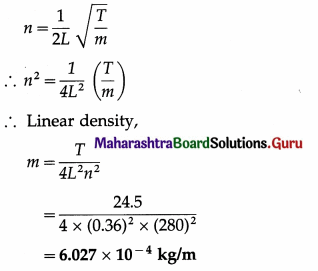
Question 16.
A sonometer wire 36 cm long, vibrates with a fundamental frequency of 280 Hz, when it is under tension of 24.5 N. Calculate mass per unit length of wire.
Solution :
Data : L = 36 cm = 0.36 m, n = 280 Hz, T = 24.5 N
n = \(\frac{1}{2 L} \sqrt{\frac{T}{m}}\)
∴ Linear density, m = \(\frac{T}{4 L^{2} n^{2}}\)
∴ m = \(\frac{24.5}{4(0.36)^{2}(280)^{2}}\)
= 6.0 × 10^-4 kg/m

Question 17.
The length of a sonometer wire between two fixed ends is 110 cm. Where should be the two bridges be placed so as to divide the wire into three segments, whose fundamental frequencies are in the ratio of 1: 2 : 3 ?
Solution :
Data : L₁ + L₂ + L₃ = 110 cm, n₁ : n₂ : n₃ = 1 : 2 : 3
According to the first law of length, n ∝ \(\frac{1}{L}\) if T and m are constant.
By given data, n₁ = 2n₂ = 3n₃
∴ \(\frac{1}{L_{1}}\) = \(\frac{2}{L_{2}}\) = \(\frac{3}{L_{3}}\)
∴ L₂ = 2L₁ and L₃ = 3L₁
L₁ + 2L₁ + 3L₁ = 110
∴ 6L₁ = 110
∴ L₁ = 18.3 cm
∴ L₂ = 2 × 18.3 = 36.6 cm
∴ L₃ = 3 × 18.3 = 54.9 cm
Therefore, the two bridge should be kept in such a way that the distance between them in 36.6 cm and distance of 1st bridge from the fixed end of the wire is 18.3 cm.

Question 72.
What are beats? Define
(1) the period of beats
(2) beat frequency (1 mark each)
Answer:
A periodic variation in loudness (or intensity) when two sound notes of slightly different frequencies are sounded
at the same time is called beats.

If two notes of slightly different frequencies n₁ and n₂ are played simultaneously, the resulting
note from their interference has a frequency of (n₁ + n₂)/2. However, the amplitude of this resulting note varies from the sum to the difference of the amplitudes of the two notes n₁ and n₂. An intensity maximum and an intensity minimum are respectively called waxing and waning. Thus, the resulting note will be heard as one of periodic loud (waxing) and faint (waning) sound. One waxing and one waning form one beat. Formation of beats is an example of interference in time.

The time interval between successive maxima or minima of sound at a given place is called the period of beats.
The number of beats produced per unit time is called the beat frequency.

Question 73.
Distinguish between stationary waves and beats. (Two points of distinction)
Answer:

Stationary waves	Beats
1. These are formed due to interference, under certain conditions, between two identical progressive waves travelling in opposite directions.	1. These are formed due to interference between two progressive waves which need not be travelling in opposite directions.
2. Interfering waves must have the same frequency.	2. Interfering waves must have slightly different frequencies.
3. At a given point, the amplitude is constant.	3. At a given point, the amplitude changes with time.
4. Nodes and antinodes are produced.	4. There is waxing and waning of resultant intensity.
5. The resultant wave does not travel in any direction.	5. The resultant wave travels in the forward direction.
6. There is no energy transport through the medium.	6. There is energy transport through the medium.

Question 74.
Discuss analytically the formation of beats and show that
(1) the beat frequency equals the difference in frequencies of two interfering waves
(2) the waxing and waning occur alternately and with the same period.
OR
Explain the production of beats and deduce analytically the expression for beat frequency.
Answer:
Consider two sound waves of equal amplitude (A) and slightly different frequencies n₁ and n₂ (with n₁ > n₂) propagating through the medium in the same direction and along the same line. These waves can be represented by the equations y₁ = A sin 2πn₁t and y₂ = A sin 2πn₂t at x = 0, where y denotes the displacement of the particle of the medium from its mean position.

By the principle of superposition of waves, the resultant displacement of the particle of the medium at the point at which the two waves arrive simultaneously is the algebraic sum
y = y₁ + y₂ = A sin 2πn₁t + A sin 2πn₂t
Now, sin C + sin D

∴y = R sin 2πnt

The above equation shows that the resultant motion has amplitude IRI which changes periodically with time. The period of beats is the period of waxing (maximum intensity of sound) or the period of waning (minimum intensity of sound). The intensity of sound is directly proportional to the square of the amplitude of the wave. It is maximum (waxing) when R becomes maximum;

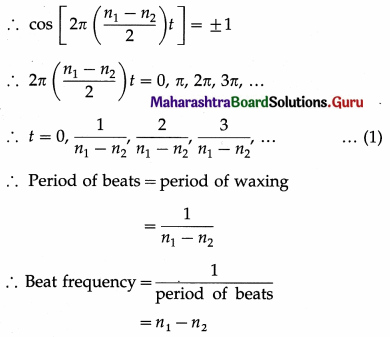
The intensity of sound is minimum (waning) when R = 0

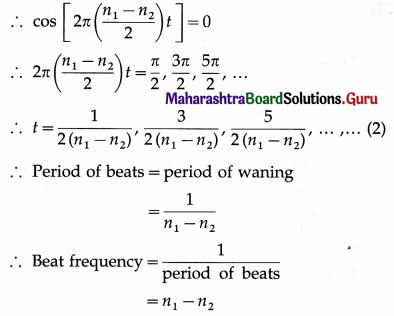
From Eqs. (1) and (2), it can be seen that the waxing and waning occur alternately and with the same period.

Question 75.
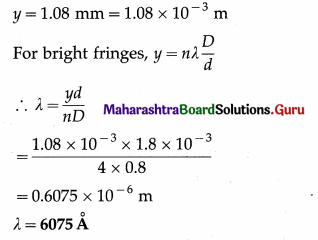
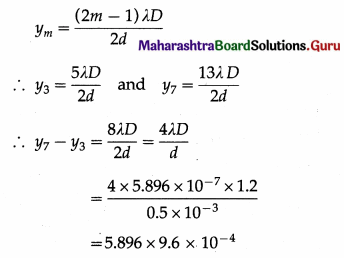
The speed of sound in air under certain conditions is 350 m/s. If two sound waves of wavelengths \(\) m and \(\) m arrive at a point at the same time, what will be the beat frequency?
Answer:
Beat frequency = |n₁ – n₂|

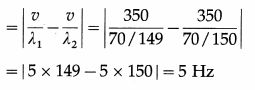
Question 76.
State the conditions for hearing beats.
Answer:
Conditions for hearing beats : For two sound waves to interfere and give rise to beats,

1. they should travel in the same medium and arrive at the listener at the same time
2. their frequencies should not differ by more than about 7 Hz for distinct beats
3. their amplitudes should be equal or nearly so.

Question 77.
When two tuning forks of slightly different frequencies are sounded together to produce beats, what is the effect on the beat frequency if the prongs of the tuning fork with higher frequency are waxed a little or filed a little?
Answer:
Filing the prongs of a tuning fork reduces its mass and thereby raises its frequency. Applying a little wax to the prongs increases its mass and thereby reduces its frequency. Therefore, filing the prongs of the tuning fork of the higher frequency increases the beat frequency while applying a little wax to its prongs decreases the beat frequency.

Question 78.
When two tuning forks of slightly different frequencies are sounded together to produce beats, what is the effect on beat frequency if the prongs of the tuning fork with lower frequency is waxed a little or filed a little?
Answer:
Filing the prongs of a tuning fork reduces its mass and thereby raises its frequency. Applying a little wax to the prongs increases its mass and thereby reduces its frequency. Therefore, filing the prongs of the tuning fork of the lower frequency decreases the beat frequency while applying a little wax to its prongs increases the beat frequency.

Question 79.
A tuning fork has frequency 512 Hz. What can you say about its frequency when

1. its prongs are filed
2. some wax is applied to its prongs ?

Answer:

1. Its frequency will be more than 512 Hz.
2. Its frequency will be less than 512 Hz.

Question 80.
Explain any two applications of beats.
Answer:
Applications of beats :

(1) Listening for beats – or rather, their absence-is the usual method of tuning musical instruments and in the determination of the frequency of a musical note.

(2) Ultrasonic vocal sounds made by bats and dolphins may be detected by superimposing a sound of different frequency to produce audible beats.

(3) In music, beats are used to produce a low frequency sound (a grave tone). Two notes whose difference in frequency is equal to the desired low frequency are used for this purpose. When two notes are nearly in tune, the beats are slow. But as the beat frequency increases to 20 Hz or more, the beats may ultimately merge into a continuous tone known as a difference tone.

(4) (i) Speed of a moving object can be determined using a Doppler RADAR. Radio waves from the RADAR are reflected off a moving object, such as an aeroplane. The superposition of the incident and reflected waves produces beats. The frequency of beats helps to determine the speed of the aeroplane.
The same principle is used in speed guns used by traffic police to determine the speed of cars on a highway.

(ii) In medicine, a Doppler ultrasound test (sonography) uses reflected sound waves to evaluate blood flow through the major arteries and veins of the arms, legs and neck. It can show blocked or reduced blood flow because of narrowing of the major arteries. Duplex (or 2D) Doppler, Colour Doppler and Power Doppler are different techniques of the same test.

Notes: Some other applications of beats are as follows :

1. Detection of toxic gases inside mines, especially collieries : Air from inside a mine and pure air are blown through two separate identical organ pipes. If beats are heard it would indicate that the composition of air inside the mines is different from that outside. This can serve as an early warning system.
2. In music, consonance and dissonance depend upon the beats produced when two notes are sounded simultaneously. A beat frequency between 10 Hz and 50 Hz (between the fundamental notes being played as well as any of their overtones) is unpleasant and results in dissonance.
3. Superheterodyne reception of radio waves in most radio, television and radar receivers : A low-frequency signal produced in the receiver is beat against an incoming high-frequency radio signal to produce an intermediate (beat) frequency (IF). This IF signal retains the information of the incoming signal. The receiver can be tuned to different broadcast frequencies by adjusting the frequency of the low-frequency signal. The IF signal though can be kept the same in every case and can therefore be amplified with higher gain.]

Question 81.
If beat frequency is 10 Hz, what is the time interval between
(i) successive waxings
(ii) a waxing and subsequent waning of sound.
Answer:
Beat period = \(\frac{1}{\text { beat frequency }}\) = \(\frac{1}{10 \mathrm{~Hz}}\) = 0.1 s
Hence, the time interval will be 0.1 s in case (i) and 0.05 s in case (ii).

Question 82.
A sonometer wire of length L₁ is in unison with a tuning fork of frequency n. When the vibrating length of the wire is reduced to L₂, it produces x beats per second with the fork. Show that n = x₂.\(\frac{L_{2}}{L_{1}-L_{2}}\).
Answer:
The fundamental frequency of vibration of a wire of length L₁, mass per unit length m and under tension T is
n₁ = \(\frac{1}{2 L_{1}} \sqrt{\frac{T}{m}}\) = n … (1)
since it is in unison with a tuning fork of frequency n. When the vibrating length of the wire is L₂, its fundamental frequency is
n₂ = \(\frac{1}{2 L_{2}} \sqrt{\frac{T}{m}}\) … (2)
T and m remaining constant.
∴ \(\frac{n_{2}}{n_{1}}\) = \(\frac{L_{1}}{L_{2}}\) … (3)
Since L₂ < L₁, n₂ > n₁ so that n₂ – n₁ = x
∴ n₂ = n₁ + x …. (4)
Substituting for n₂ in Eq. (3),
\(\frac{n_{1}+x}{n_{1}}\) = \(\frac{L_{1}}{L_{2}}\) or \(\frac{x}{n_{1}}\) = \(\frac{L_{1}-L_{2}}{L_{2}}\)
∴ n₁ = n = x.\(\frac{L_{2}}{L_{1}-L_{2}}\)
which is the required expression.

Question 83.
Solve the following :

Question 1.
A tuning fork C produces 6 beats/second- with another tuning fork D of frequency 320 Hz. When a little wax is put on the prongs of D, the number of beats reduces to 4 per second. Find the frequency of C.
Solution :

1. Initially 6 beats per second are heard. Hence, the difference between the frequencies of the tuning forks is 6 Hz. As the frequency of fork D is 320 Hz, the frequency of fork C = 320 + 6 Hz
   = 326 Hz or 314 Hz
2. When the prongs of fork D are loaded with a little wax, the frequency of fork D decreases and becomes less than 320 Hz.
3. If the frequency of fork C is 326 Hz, the number of beats heard per second must increase.
4. However, as the number of beats heard per second has decreased from 6 to 4, the frequency of fork C must be 314 Hz.

Question 2.
A tuning fork C produces 8 beats per second with another tuning fork D of frequency 340 Hz. When the prongs of tuning fork C are filed a little, the number of beats produced per second decreases to 4. Find the frequency of tuning fork C before filing its prongs.
Solution :
The frequency of timing fork D is 340 Hz. Let n be the frequency of tuning fork C. Since tuning forks C and D produce 8 beats per second when sounded together,
n – 340 = 8 or 340 – n = 8
∴ n = 348 Hz or 332 Hz
When the prongs of a tuning fork are filed a little, its frequency increases. Let n’ be its frequency after filing : n’ > n.

It is given that the beat frequency is reduced from 8 Hz to 4 Hz.
If n was 348 Hz, n’ will be more than 348 Hz. Hence, the beat frequency should increase. Hence, n ≠ 348 Hz.
∴ n = 332 Hz

Question 3.
A sonometer wire 100 cm long produces a resonance with a tuning fork. When its length is decreased by 10 cm, 8 beats per second are heard. Find the frequency of the tuning fork.
Solution :
Data : L₁ = 100 cm, L₂ = 100 – 10 = 90 cm, n₂ – n₁ = 8 beats per second

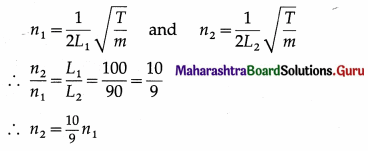
Now, n₂ – n₁ = 8 ∴ \(\frac{10}{9}\)n₁ – n₁ = 8
∴ 10n₁ – 9n₁ = 9 × 8 = 72
∴ n₁ = 72 Hz
This gives the frequency of the tuning fork as the wire of length 100 cm is in unison with the fork.

Question 4.
A stretched sonometer wire is in unison with a tuning fork. When its length is increased by 4 %, the number of beats heard per second is 6. Find the frequency of the fork.
Solution :
Data : \(\frac{L_{2}}{L_{1}}\) = 1.04, n₁ – n₂ = 6Hz
n₁ = \(\frac{1}{2 L_{1}} \sqrt{\frac{T}{m}}\) and n₂ = \(\frac{1}{2 L_{2}} \sqrt{\frac{T}{m}}\)
∴ \(\frac{n_{1}}{n_{2}}\) = \(\frac{L_{2}}{L_{1}}\) = 1.04
∴ n₁ = 1.04n₂
Now, n₁ – n₂ = 6
∴ 1.04 n₂ – n₂ = 6
∴ n₂ = \(\frac{6}{0.04}\) = 150 Hz
∴ n₁ = n₂ + 6 = 150 + 6 = 156 Hz
This gives the frequency of the tuning fork as initially the wire and the fork vibrate in unison.

Question 5.
The wavelengths of two notes in air are \(\frac{83}{170}\) m and \(\frac{83}{172}\) m. Each of these notes produces 4 beats per second with a third note of a fixed frequency. Find the speed of sound in air.
Solution :
Data : λ₁ = 83/170 m, λ₂ = 83/172 m
Let n₁ and n₂ be the corresponding frequencies.
∴ v = n₁λ₁ = n₂λ₂
where v is the speed of sound in air.
But λ₁ > λ₂
∴ n₁ < n₂
If n is the third frequency, n₁ < n < n₂
∴ n – n₁ = 4 and n₂ – n = 4
Method 1 :
∴ n₂ – n₁ = 8 ∴ \(\frac{v}{\lambda_{2}}\) – \(\frac{v}{\lambda_{1}}\) = 8
∴ v\(\left[\frac{172}{83}-\frac{170}{183}\right]\) = 8 ∴ v × \(\frac{2}{83}\) = 8
∴ v = 4 × 83 = 332 m/s
Method 2 :
∴ n₁ = n – 4 and n₂ = n + 4
∴ (n – 4) × \(\frac{83}{170}\) = (n + 4) × \(\frac{83}{172}\)
Simplifying, we get, 2n = 1368
∴ n = 684 Hz
∴ n₁ = 684 – 4 = 680 Hz
∴ v = n₁λ₁ = 680 × \(\frac{83}{170}\) = 4 × 83
∴ v = 332 m/s

Question 6.
Two sound notes have wavelengths \(\frac{83}{170}\) m and \(\frac{83}{172}\) m in air. These notes, when sounded together, produce 8 beats per second. Calculate the velocity of sound in air and frequencies of the two notes.
Solution :
Data : λ₁ = 83/170 m, λ₂ = 83/172 m
Let nλ₁ and nλ₂ be the corresponding frequencies.
∴ v = n₁λ₁ = n₂λ₂
where v is the velocity of sound in air.
∴ n₁ = \(\frac{v}{\lambda_{1}}\) and n₂ = \(\frac{v}{\lambda_{2}}\) … (1)
But λ₁ > λ₂ ∴ n₁ < n₂
∴ n₂ – n₁ = 8
∴ v\(\left(\frac{1}{\lambda_{2}}-\frac{1}{\lambda_{1}}\right)\) = 8 [From Eq. (1)] …(2)

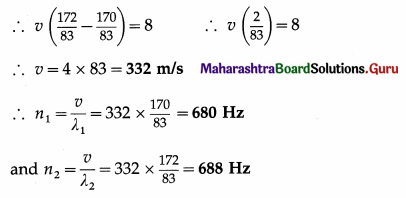
Question 7.
32 tuning forks are arranged in descending order of frequencies. If any two consecutive tuning forks are sounded together, the number of beats heard is eight per second. The frequency of the first tuning fork is octave of the last fork. Calculate the frequency of the first, last and the 21st fork.
Solution :
Data : n₁ = 2n₃₂, (n₁ is octave of n₃₂) beat frequency = 8 Hz
The set of tuning forks is arranged in descending order of their frequencies.
∴ n₂ = n₁ – 8
n₃ = n₂ – 8 = n₁ – 2 × 8
n₄ = n₃ – 8 = n₁ – 3 × 8
∴ n₃₂ = n₁ – 31 × 8 = n₁ – 248
Since n₁ = 2n₃₁, n₃₂ = 2n₃₂ – 248
∴ The frequency of the last fork, n₃₂ = 248 Hz
The frequency of the first fork, n₁ = 2n₃₂ = 2 × 248 = 496 Hz
∴ The frequency of the 21st fork, n₂₁ = n₁ – 20 × 8 = 496 -160 = 336 Hz

Question 8.
A set of 12 tuning forks is arranged in order of increasing frequencies. Each fork produces V beats per second with the previous one. The last is an octave of the first. The fifth fork has a frequency of 90 Hz. Find V and the frequency of the first and the last tuning forks.
Solution :
Data : n_i+1 – n_i = Y, n₁₂ = 2n₁, n₅ = 90 Hz n₂ – n₁ = Y beats/s
∴ n₂ = n₁ + Y beats/s
Similarly, n₃ = n₂ + Y = n₁ + Y + Y
∴ n₃ = n₁ + 2Y = n₁ + (3 – 1) Y
∴ n_x = n₁ + (x – 1) Y
Similarly, n₁₂ = n₁ + (12 – 1) Y = n₁ + 11Y
∴ n₁₂ = 2n₁ = n₁ + 11Y
∴ n₁ = 11Y
Also, n₅ = n₁ + (5 – 1) Y = n₁ + 4Y
∴ n₅ = 11Y + 4Y = 15Y
∵ n₅ = 90 Hz ∴ 15Y = 90 ∴ Y = 6
∴ n₁ = 11Y beats/s = 11 × 6 beats/s = 66 Hz and n₁₂ = 2n₁ = 2 (66) = 132 Hz

Question 9.
Two tuning forks when sounded together produce 5 beats per second. A sonometer wire of length 0.24 m is in unison with one of the forks. When the length of wire is increased by 1 cm, it is in unison with the other fork. Find the frequencies of the tuning forks.
Solution :
Data :L₁ = 0.24 m = 24 cm, L₂ = 24 + 1 = 25 cm, beat frequency = 5 Hz

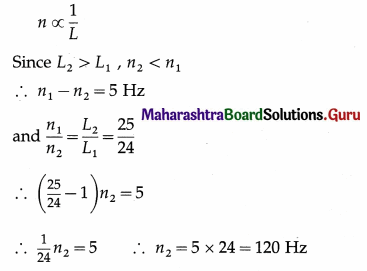
∴ n₁ = n₂ + 5 = 125 Hz
∴ The frequencies of the two tuning forks are 125 Hz and 120 Hz.

Question 10.
A closed pipe and an open pipe sounded together produce 5 beats/s. If the length of the open pipe is 30 cm, find by how much should the length of the closed pipe be changed to make the air columns in the two pipes vibrate in unison. [Speed of sound in air = 330 m/s]
Solution :
Data : Beat frequency = 5 s^-1, L_O = 0.3 m, v = 330 m/s
The fundamental frequencies of a closed pipe and open pipe are respectively
n_C = \(\frac{v}{4 L_{\mathrm{C}}}\) and n₀ = \(\frac{v}{2 L_{O}}\)
Let L’_C and n’_C be the changed length and frequency of the closed pipe,
n’_C = n_O

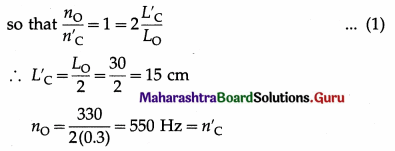
Method 1 :
Since the beat frequency = 5 Hz,
|n_C – n_O| = |n_C – n’_C| = 5

∴ The length of the given closed pipe should be changed by \(\frac{10}{11}\) % to bring it unison with the open pipe.

Method 2 :
Since the beat frequency = 5 Hz,
n_C – n_O = 5 or n_O – n_C = 5
i. e., n_C = 555 Hz or 545 Hz
∴ L_C = \(\frac{v}{4 n_{\mathrm{C}}}\) = \(\frac{330}{4(555)}\) or \(\frac{330}{4(545)}\)
= 0.1486 m or 0.1514 m
= 14.86 cm or 15.14 cm
∴ The length of the given closed pipe should be changed by 0.14 cm.

Question 11.
The forks, A and B, produce 4 beats/s when sounded together. Fork A is in unison with 30 cm length of a sonometer wire and fork B is in unison with 25 cm length of the same wire under the same tension. Calculate the frequencies of the forks.
Solution :
Data : L_A = 30 cm, L_B = 25 cm, beat frequency = 4 s^-1
n ∝ \(\frac{1}{L}\)
Since L_A > L_B, n_A < n_B
∴ n_B – n_A = 4 Hz
and, for the same tension and linear density,
\(\frac{n_{\mathrm{B}}}{n_{\mathrm{A}}}\) = \(\frac{L_{\mathrm{A}}}{L_{\mathrm{B}}}\) = \(\frac{30}{25}\) = \(\frac{6}{5}\)
∴ \(\left(\frac{6}{5}-1\right)\)n_A = 4
∴ \(\frac{1}{5}\)n_A = 4
∴ n_A = 20 Hz
∴ n_B = 24 Hz

Question 84.
Explain the following characteristics of sound :
(1) loudness
(2) pitch
(3) quality or timbre.
Ans.
(1) Loudness : The loudness of a note is the magnitude of the sensation produced by the sound waves on the ear. It depends upon
(a) the energy of the vibration
(b) the sensitiveness of the individual ear
(c) the pitch of the sound.

The loudness of a sound depends on the intensity of the sound wave, which is in turn proportional to the square of the amplitude of the wave itself. Loudness is a physiological (subjective) sensation, while intensity is an objectively measurable physical property of the wave. There is no direct relation between loudness and intensity. Near the middle of the audible range of frequencies, the ear is very sensitive to changes in intensity, which it interprets as changes in loudness.

The unit of loudness is the phon. It is equal to the loudness in decibel of any equally loud pure tone of frequency 1000 Hz.

(2) Pitch : By pitch we mean whether the note is high or low. The pitch of a note depends upon the frequency of the sound. But pitch is not determined by frequency alone. A physiological factor is involved and the sense of pitch is modified by the loudness and quality of the sound.

The average range of frequencies that the human ear detects as sound is approximately 20 Hz to 20000 Hz (the audible range). The human ear is capable of detecting a difference in pitch between two notes. The smallest difference in frequency that the ear can detect as a difference in pitch is approximately proportional to the frequency of one of the notes. That is, a given change in frequency of a low note will produce a greater change in pitch than it will in a high note.

(3) Quality : By quality or timbre is meant that characteristic of a sound by which it is possible to distinguish it from all other sounds of the same pitch and loudness. The same note played at the same loudness on two different musical instruments are easily distinguished from each other by their timbre.

[Note : A pure note, consisting of only one frequency, is different from a musical note, which may be a combination of many different frequencies. A musical note has a fundamental, or lowest frequency, and superimposed on it are higher frequencies, called overtones or partials. The number and relative strengths of the partials present determines the timbre of the note. The ear always recognizes the fundamental as determining the pitch of the note.]

Question 85.
Define intensity of sound. State its unit.
Answer:
Definition : The intensity of sound at a point is the time rate of flow of sound energy passing normally through a unit area at that point.
SI unit: the joule per second square metre (j/s.m²) or watt per square metre (W/m²).

Question 86.
What are the factors affecting the loudness of sound ? Is intensity the same as loudness ?
Answer:
(1) The factors affecting the loudness of sound are

1. the amplitude of the vibrations of the body
2. the distance of the listener from the vibrating body
3. the surface area of the vibrating body
4. the density of the medium
5. the presence of the resonating bodies
6. the sensitivity of the ear of the listener.

(2) Intensity and loudness are related, but not the same. Intensity is a measurable quantity whereas loudness is a sensation which is not measurable. Loudness depends on the intensity of sound as well as the sensitivity of the ear of the listener.

Question 87.
Explain the term decibel
Answer:
The intensity level of a sound wave, by definition, is β = log₁₀ \(\left(\frac{I}{I_{0}}\right)\)bels = 10 log₁₀ \(\left(\frac{I}{I_{0}}\right)\) decibels as one decibel is 0.1 bel. Here, I₀ (reference intensity) is taken as 10^-12 W/m².

Intensity level is expressed in decibel (dB). There is no direct relation between loudness and intensity. The decibel is not a unit of loudness.

[Note : The decibel, equal to 0.1 bel, is used for comparing two power levels, currents or voltages. The unit bel is named in honour of Alexander Graham Bell (1847-1922) British-American scientist, inventor of the telephone (1876).]

Question 88.
What is the difference between a musical sound and a noise ?
Answer:
A musical sound is pleasing to the listener while a noise is not. The pleasure derived from a musical note is because it strikes the ear as a perfectly undisturbed, uniform sound which remains unaltered as long as it exists. On the other hand, noise is accompanied by a rapid, irregular but distinct, alternations of various kinds of sounds.

A musical sound thus has a regularity or smoothness because the vibrations that cause the sound are periodic. But the converse, that if the vibrations are regular the sound is musical, is not always true. For example, a ticking clock does not produce a musical note, or the definite note produced by a card held against the teeth of a rotating toothed wheel is far from being pleasant to hear. Bearing such reservations in mind, the essential difference between music and noise is that the former is produced by periodic and continuous vibrations, while noise results from discontinuous sudden and sharp sounds with no marked periodicity.

Question 89.

1. Which quantity out of frequency and amplitude determines the pitch of the sound?
2. Which out of pitch and frequency is a measurable quantity ?

Answer:

1. The frequency of sound determines its pitch. A high pitched or shrill sound is produced by a body vibrating with a high frequency and a low pitched or flat sound is produced by a body vibrating with a low frequency.
2. Frequency is a measurable quantity whereas pitch is not a measurable quantity.

Question 90.
Write a note on the major diatonic scale.
OR
Explain what is a musical scale.
Answer:
A musical scale is constructed on the basis of certain groups of notes with simple intervals. A major chord or triad is a group of three notes with frequencies in the ratio 4:5:6 that produce a very pleasing effect when sounded together. The diatonic musical scale is composed of three sets of triads making eight notes.

Some note called the tonic, is chosen as the basis of the scale, and a triad is constructed using this note as the one of lowest frequency. Calling the tonic as the 1st, the major chords are 1st, 3rd and 5th, 4th, 6th and 8th, and 5th, 7th and 9th; 8th and 9th are respectively the octaves of the 1st and 2nd.

In addition to the eight notes of an octave, that form the major scale, five additional notes are also used. These are derived either by raising or lowering the pitch by the interval 25 / 24. If the pitch is raised the note is sharp, and when lowered, it is flat.

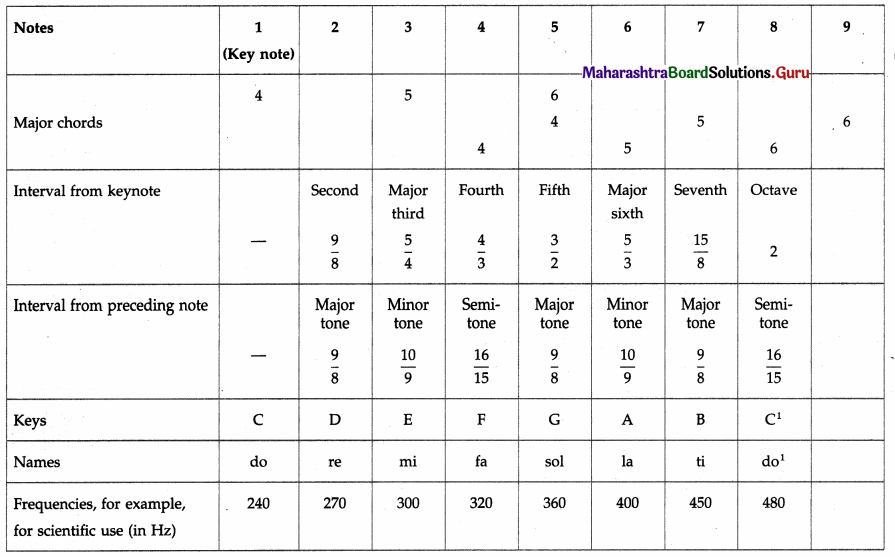
Question 91.
Write a short note on Indian musical scale.
Answer:
Indian music is chiefly based on melody, i.e., consonant notes in suitable succession. Besides this physiological sensation, there is a deep psychological involvement.
The notes or svaras (स्वर) used in an Indian musical scale have the same musical intervals as those of the major diatonic scale. The five additional notes in pure intonation, तीव्र (sharp) and कोमल (flat) are also used. Thus, the choice is usually made from the following twelve svaras : सा (shadja), रे(को) and रे (rishabha), ग(को) and ग g\(\bar{a}\)ndh\(\bar{a}\)ra), म and म(ती) (madhyam), प (pancham), ध (को) and ध (dhaivata), नी(को) and नी (nishad), सा. However, as compared to the fixed frequency of the tonic in western music, an Indian vocalist or musician has the freedom to set any frequency as the tonic. Besides, unlike western music, dissonant intervals are sometimes introduced to enhance the musical effect.

However, the whole structure of Indian music is based on r\(\bar{a}\)gas (राग), which are well-established melody types with a wide variety of emotional content. They can be courageous, amorous, melancholy, cheerful, soothing, or ecstatic. R\(\bar{a}\)gas are capable of conveying these emotions to the listener and different r\(\bar{a}\)gas are assigned to different seasons and different parts of the day.

Question 92.
Give reasons :
The notes of a sitar and a guitar sound different even if they have the same loudness and the pitch.
Answer:
The quality or timbre of the sound of a sitar is different from that of a guitar. The number of overtones or partials present and their relative intensities determine the quality or timbre of the sound of a musical instrument. Therefore, even if the pitch and the loudness are the same, the notes of a sitar and a guitar sound different.

Question 93.
Which are the three broad types of musical instruments ?
OR
Write a short note on types of musical instruments.
Musical instruments have been classified in various ways. One ancient system that was based on the primary vibrating medium distinguished three main types of instruments : stringed, wind and percussion.
Examples :
(1) Stringed instruments (stretched strings) :
(a) Plucked : Tanpura, sitar, veena, guitar, harp
(b) Bowed : Violin
(c) Struck : Santoor, pianoforte )

(2) Wind instruments :
(a) Free (air not confined) : Harmonica or mouth organ (without keyboard), harmonium (with keyboard). (Both are reed instruments in which free brass reeds are vibrated by air, blown or compressed.)
(b) Edge (air blown against an edge) : Flute
(c) Reedpipes : Saxophone (single reed), shehnai and bassoon (double reeds)

(3) Percussion instruments :
(a) Stretched skin heads : Tabla, mridangam, drums
(b) Metals (struck against each other or with a beater) : Cymbals, Xylophone

Question 94.
A simple harmonic wave of frequency 20 Hz is travelling in the positive direction of x-axis with a velocity of 30 m/s. Two particles in the path of the wave, 0.45 m apart, differ in phase by
(A) \(\frac{\pi}{3}\) rad
(B) \(\frac{\pi}{2}\) rad
(C) 0.6 π rad
(D) π rad.
Answer:
(C) 0.6 π rad

Question 95.
What is the period of the wave given by y = 0.003 sin (\(\frac{\pi}{0.08}\)t + \(\frac{\pi}{8}\)x ) (in SI units) ?
(A) 0.08 s
(B) 0.16 s
(C) 0.32 s
(D) 0.8 s.
Answer:
(B) 0.16 s

Question 96.
The equation of a progressive wave is y = 7 sin(4t – 0.02x), where x and y are in centimetre and time t in second. The maximum velocity of a particle is
(A) 28 cm/s
(B) 32 cm/s
(C) 49 cm/s
(D) 112 cm/s.
Answer:
(A) 28 cm/s

Question 97.
When a longitudinal wave is incident at the boundary of a denser medium, then
(A) a compression reflects as a compression
(B) a compression reflects as a rarefaction
(C) a rarefaction reflects as a compression
(D) a longitudinal wave reflects as a transverse wave.
Answer:
(A) a compression reflects as a compression

Question 98.
A transverse wave travelling in a denser medium is reflected from a rarer medium. Then,
(A) an incident crest is reflected as a crest
(B) an incident crest is reflected as a trough
(C) there is a phase change of 2π rad
(D) there is a phase change of π/2 rad.
Answer:
(A) an incident crest is reflected as a crest

Question 99.
Two simple harmonic waves of the same amplitude and frequency, but 90° out of phase, pass through the same region in a medium. The resultant wave has
(A) an amplitude greater than either of the component waves
(B) an amplitude smaller than either of the component waves
(C) zero amplitude
(D) an amplitude slowly varying with time.
Answer:
(A) an amplitude greater than either of the component waves

Question 100.
At a given instant two vibrating particles in the same loop of a stationary wave have
(A) the same phase
(B) opposite phases
(C) slightly different phases
(D) opposite velocities.
Answer:
(A) the same phase

Question 101.
Two vibrating particles in the adjacent loops of a stationary wave have ….. at a given instant.
(A) the same phase
(B) opposite phases
(C) slightly different phases
(D) the same velocity
Answer:
(B) opposite phases

Question 102.
A stretched string, 2 m long, vibrates in its third overtone. The distance between consecutive nodes is
(A) 40 cm
(B) 50 cm
(C) 66.7 cm
(D) 100 cm.
Answer:
(B) 50 cm

Question 103.
A stretched string of length l vibrates in the third overtone. The wavelength of stationary wave formed is
(A) \(\frac{l}{2}\)
(B) \(\frac{l}{4}\)
(C) l
(D) 21.
Answer:
(A) \(\frac{l}{2}\)

Question 104.
A stretched string tied between two rigid supports vibrates with a frequency double the fundamental frequency. The point midway between the supports is
(A) a node
(B) an antinode
(C) either a node or an antinode
(D) neither a node nor an antinode.
Answer:
(A) a node

Question 105.
A travelling wave of frequency 100 Hz along a string is reflected from a fixed end. The stationary wave formed has the nearest node at a distance of 10 cm from the fixed end. The speed of the travelling wave was
(A) 40 m/s
(B) 20 m/s
(C) 10 m/s
(D) 5m/s.
Answer:
(B) 20 m/s

Question 106.
Stationary waves are produced on a 10 m long stretched string fixed at both ends. If the string vibrates in 5 segments and the wave velocity is 20 m/s, the frequency of the waves is
(A) 10 Hz
(B) 5 Hz
(C) 4 Hz
(D) 2 Hz.
Answer:
(B) 5 Hz

Question 107.
The fundamental frequency of transverse vibrations of a stretched string of radius r is proportional to
(A) r^-2
(B) r^-1
(C) \(r^{-\frac{1}{2}}\)
(D) r².
Answer:
(B) r^-1

Question 108.
A stretched string of length 50 cm vibrates in five segments when stationary waves are formed on it. If the wave speed is 14 m/s, its frequency of vibration is
(A) 28 Hz
(B) 35 Hz
(C) 70 Hz
(D) 140 Hz.
Answer:
(C) 70 Hz

Question 109.
Two strings A and B are identical except that the diameter of A is twice the diameter of B. The ratio of the frequency of sound from A to that from B is
(A) 2 : 1
(B) \(\sqrt{2}\) : 1
(C) 1 : \(\sqrt{2}\)
(D) 1 : 2.
Answer:
(D) 1 : 2.

Question 110.
Two strings, A and B, have the same tension and length. The string A has a mass m while the string B has a mass Am. If the speed of the waves in string A is v, that on string B is
(A) \(\frac{1}{2}\)v
(B) v
(C) 2v
(D) v.
Answer:
(A) \(\frac{1}{2}\)v

Question 111.
An organ pipe is closed at one end. The pth overtone is the ….. th harmonic.
(A) 2p + 1
(B) 2p – 1
(C) p + 1
(D) P – 1
Answer:
(A) 2p + 1

Question 112.
Of two narrow organ pipes A and B, A is open at one end and B at both ends. Both the pipes have the same fundamental frequency. If A is 1.2 m long, how long is B?
(A) 0.8 m
(B) 1.8 m
(C) 2.4 m
(D) 3.0 m
Answer:
(C) 2.4 m

Question 113.
The value of end correction for an open organ pipe of radius r is
(A) 0.3 r
(B) 0.6 r
(C) 0.9 r
(D) 1.2 r.
Answer:
(D) 1.2 r.

Question 114.
Of two long narrow organ pipes A and B, A is open at one end and B at both ends. If both the pipes have the same fundamental frequency, the first overtone of A is ….. the first overtone of B.
(A) half of
(B) \(\frac{2}{3}\) of
(C) equal to
(D) twice
Answer:
(B) \(\frac{2}{3}\) of

Question 115.
In an open organ pipe, the first overtone produced is of such frequency that the length of the pipe is equal to
(A) \(\frac{\lambda}{4}\)
(B) \(\frac{\lambda}{3}\)
(C) \(\frac{\lambda}{2}\)
(D) λ
Answer:
(D) λ

Question 116.
A sonometer wire vibrates with three nodes and two antinodes. The corresponding mode of vibration is
(A) the first overtone
(B) the second overtone
(C) the third overtone
(D) the fourth overtone.
Answer:
(A) the first overtone

Question 117.
Velocity of a transverse wave along a stretched string is proportional to [T = tension in the string]
(A) \(\sqrt{T}\)
(B) T
(C) \(\frac{1}{\sqrt{T}}\)
(D) \(\frac{1}{T}\)
Answer:
(A) \(\sqrt{T}\)

Question 118.
The frequency of the second overtone of the vibration of a stretched string is
A. \(\frac{1}{l} \sqrt{\frac{T}{m}}\)
B. \(\frac{3}{2 l} \sqrt{\frac{T}{m}}\)
C. \(\frac{1}{2 l} \sqrt{\frac{T}{m}}\)
D. \(\frac{2}{3 l} \sqrt{\frac{T}{m}}\)
Answer:
B. \(\frac{3}{2 l} \sqrt{\frac{T}{m}}\)

Question 119.
When the air column in a pipe closed at one end vibrates such that three nodes are formed in it, the frequency of its vibrations is ….. times the fundamental frequency.
(A) 2
(B) 3
(C) 4
(D) 5
Answer:
(D) 5

Question 120.
One beat means that the intensity of sound should be
(A) once maximum
(B) once minimum
(C) once maximum and once minimum
(D) twice maximum and twice minimum.
Answer:
(C) once maximum and once minimum

Question 121.
Let n₁ and n₂ be two slightly different frequencies of sound waves. The time interval between a waxing and the immediate next waning is
(A) \(\frac{1}{n_{1}-n_{2}}\)
(B) \(\frac{2}{n_{1}-n_{2}}\)
(C) \(\frac{n_{1}-n_{2}}{2}\)
(D) \(\frac{1}{2\left(n_{1}-n_{2}\right)}\)
Answer:
(D) \(\frac{1}{2\left(n_{1}-n_{2}\right)}\)

Question 122.
In the formation of beats, the resultant amplitude varies with a frequency equal to
(A) the beat frequency
(B) the average frequency
(C) half the beat frequency
(D) double the beat frequency.
Answer:
(C) half the beat frequency

Question 123.
A tuning fork A of frequency 512 Hz produces 3 beats per second with another tuning fork B of frequency 515 Hz. If the prongs of B are filed a little, the number of beats produced per second will
(A) increase
(B) decrease
(C) remain the same
(D) increase or decrease.
Answer:
(A) increase

Question 124.
A tuning fork gives 1 beat in 2 seconds with a timing fork of frequency 341.3 Hz. If the beat frequency decreases when the first fork is filed a little, its original frequency was
(A) 336.3 Hz
(B) 340.8 Hz
(C) 341.8 Hz
(D) 346.3 Hz.
Answer:
(B) 340.8 Hz

Question 125.
In a set of 25 tuning forks, arranged in order of increasing frequency, each fork gives 3 beats per second with the succeeding one. If the frequency of the 10th fork is 127 Hz, the frequency of the 16th fork is
(A) 139 Hz
(B) 145 Hz
(C) 148 Hz
(D) 151 Hz.
Answer:
(B) 145 Hz

Question 126.
If two sound waves with the same amplitude but slightly different frequencies n₁ and n₂ superpose to produce beats, the resultant wave motion has frequency
(A) |n₁ – n₂|
(B) n₁ + n₂
(C) \(\frac{\left|n_{1}-n_{2}\right|}{2}\)
(D) \(\frac{n_{1}+n_{2}}{2}\)
Answer:
(D) \(\frac{n_{1}+n_{2}}{2}\)

Balbharti Maharashtra State Board Class 12 Secretarial Practice Important Questions Chapter 8 Correspondence with Depositors Important Questions and Answers.

Maharashtra State Board 12th Secretarial Practice Important Questions Chapter 8 Correspondence with Depositors

1A. Select the correct answer from the options given below and rewrite the statements.

Question 1.
_____________ capital is raised by accepting deposits.
(a) owned
(b) fixed
(c) borrowed
Answer:
(c) borrowed

Question 2.
Deposits are accepted to fulfil _____________ capital need of the company.
(a) working
(b) fixed
(c) owned
Answer:
(a) working

Question 3.
A company can accept deposit for minimum _____________ months.
(a) 12
(b) 36
(c) 6
Answer:
(c) 6

Question 4.
Person investing in company’s deposit is called _____________
(a) depositor
(b) debenture holder
(c) shareholder
Answer:
(a) depositor

Question 5.
Company makes payment of interest on deposits after deducting _____________
(a) ACS
(b) CGS
(c) TDS
Answer:
(c) TDS

Question 6.
Company can also make electronic payment of interest through _____________
(a) DGFT
(b) NEFT
(c) IEPF
Answer:
(b) NEFT

Question 7.
A government Company can accept maximum _____________ percent deposit.
(a) 25
(b) 35
(c) 30
Answer:
(b) 35

Question 8.
A private company can accept deposits from _____________
(a) directors
(b) government
(c) public
Answer:
(c) public

1B. Match the pairs.

Question 1.

Group ‘A’	Group ‘B’
1. Deposit maturity	(a) Letter of renewal
2. Accuracy	(b) Investment up to ₹ 25000
3. Deposit receipt	(c) 21 days
4. Small depositors	(d) Precaution
5. Renewal of deposit	(e) 36 months
	(f) Creditors

Answer:

Group ‘A’	Group ‘B’
1. Deposit maturity	(e) 36 months
2. Accuracy	(d) Precaution
3. Deposit receipt	(c) 21 days
4. Small depositors	(b) Investment up to ₹ 25000
5. Renewal of deposit	(a) Letter of renewal

1C. Write a word or a term or a phrase that can substitute each of the following statements.

Question 1.
A person who invests in a company’s deposits.
Answer:
Depositor

Question 2.
The status of the deposit holder in the company.
Answer:
Creditor

Question 3.
Amount deducted from gross interest exceeding ₹ 10,000.
Answer:
TDS

Question 4.
Avoiding rude and harsh words while writing letters to the depositor’s means.
Answer:
Courtesy

Question 5.
The way of writing letters from depositors’ point of view.
Answer:
You attitude

1D. State whether the following statements are true or false.

Question 1.
The deposit represents owned capital of the company.
Answer:
False

Question 2.
Fixed deposit is a long-term source of finance.
Answer:
False

Question 3.
Company Secretary has to follow legal provisions while corresponding with depositors.
Answer:
True

Question 4.
Correspondence with depositors helps to maintain the goodwill of the company.
Answer:
True

Question 5.
The deposit receipt should be issued within 21 days from the date of acceptance of the deposit.
Answer:
True

Question 6.
Government companies can raise deposits up to 35 percent.
Answer:
True

1E. Find the odd one.

Question 1.
Repayment of Deposit, Convertible Debentures, Renewal of Deposit
Answer:
Convertible Debentures

Question 2.
Depositor, Creditor, Evidence of Ownership
Answer:
Evidence of Ownership

Question 3.
6 months, 25 months, 36 months
Answer:
25 months

1F. Complete the sentences.

Question 1.
Company cannot accept deposits for a period more than _____________ months.
Answer:
36 months

Question 2.
The company is liable to pay regular _____________ on the deposits at a fixed rate.
Answer:
Interest

Question 3.
Company cannot accept deposits for period less than _____________ months.
Answer:
6 months

Question 4.
Unnecessary and irrelevant information in the letter should be _____________
Answer:
Avoided

Question 5.
Secretary conducts correspondence with the depositors as per the instructions of the _____________
Answer:
Board of Directors

1G. Answer in one sentence.

Question 1.
Who gives instructions to the company secretary for corresponding with the depositors?
Answer:
The Board of Directors gives instructions to the company secretary for corresponding with the depositors.

Question 2.
Who’s consent is necessary for the renewal of the deposit?
Answer:
Depositors’ consent is necessary for the renewal of the deposit.

Question 3.
What is the time limit for sending deposit receipts to the depositor from the date of acceptance?
Answer:
A deposit receipt is sent to the depositor within 21 days from the date of acceptance of a deposit.

1H. Correct the underlined words and rewrite the following sentences.

Question 1.
Deposits can be accepted for a minimum period of 12 months.
Answer:
Deposits can be accepted for a minimum period of 6 months.

Question 2.
Deposit receipt can be issued within a period of 15 days of acceptance.
Answer:
Deposit receipt can be issued within a period of 21 days of acceptance.

1I. Arrange in proper order.

Question 1.
Legal provision, Board Resolution, Acceptance of deposits
Answer:
Legal provision, Board Resolution, Acceptance of deposits

Question 2.
Appoint banker, Credit rating, Deposit insurance
Answer:
Appoint banker, Credit rating, Deposit insurance

Question 3.
Return with depositors, Entries in register of deposit, Issue of the deposit receipt
Answer:
Issue of deposit receipt, Entries in the register of deposit, Return with depositors

2. Justify the following statements.

Question 1.
Public deposits can be renewed after the maturity period.
Answer:

• Deposits are unsecured loans offered to the company.
• Deposits can be accepted for a minimum period of 6 months and a maximum period of 36 months.
• If the Depositor agrees, deposits can be renewed for a further period.
• Renewal may take place with the same terms and conditions or maybe with different terms and conditions.
• For renewal, the depositor has to submit the old deposit receipt with the renewal request.
• The company will cancel the old deposit receipt and issue the new deposit receipt.
• The depositor enjoys a fixed rate of interest till further maturity.
• Thus, it is rightly said that Public deposits can be renewed after the maturity period.

Question 2.
Deposits are repaid on maturity.
Answer:

• Deposit is a short-term source of finance of the company and it is used in order to satisfy the short-term working capital needs of the company.
• The company cannot accept deposits for a period less than 6 months or more than 36 months,
• The company is liable to pay regular interest on the deposits at a fixed rate along with the principal amount on maturity.
• On maturity of tenure of deposits, it is binding on the company to repay the deposit.
• Default in repayment of deposit results in levy of penalty.
• The letter for repayment of the deposit is to be sent to the depositor when the deposit is to be redeemed.
• Thus, it is rightly justified that deposits are repaid on maturity.

Question 3.
Correspondence with the depositors enhances the goodwill of the company.
Answer:

• The depositors of the company are the creditors of the company.
• They provide borrowed capital to the company to meet its working capital needs.
• proper correspondence with the depositors is helpful to convey proper information about their investments.
• It generates a bridge of good relations between company and depositors which ultimately helps to generate goodwill in the Corporate market.

Balbharti Maharashtra State Board Class 12 Secretarial Practice Important Questions Chapter 6 Correspondence with Members Important Questions and Answers.

Maharashtra State Board 12th Secretarial Practice Important Questions Chapter 6 Correspondence with Members

1A. Select the correct answer from the options given below and rewrite the statements.

Question 1.
Shareholders are ____________ of the company.
(a) Owners
(b) creditors
(c) loan givers
Answer:
Owners

Question 2.
Important decisions need approval of ____________ at the meeting.
(a) auditors
(b) shareholders
(c) directors
Answer:
shareholders

Question 3.
Secretary writes letter to members as ____________
(a) auditor
(b) Public Relations Officer
(c) Director
Answer:
Public Relations Officer

Question 4.
____________ shares are issued to existing equity shareholders.
(a) deferred
(b) preference
(c) bonus
Answer:
bonus

Question 5.
Polite and prompt correspondence by secretary creates ____________ of the company.
(a) goodwill
(b) prestige
(c) image
Answer:
goodwill

Question 6.
Secretary is a ____________ of confidential matter.
(a) caretaker
(b) custodian
(c) auditor
Answer:
custodian

1B. Match the pairs.

Question 1.

Group ‘A’	Group ‘B’
(1) Secretary	(a) Registered Document
(2) Electronic Mode	(b) Fluctuating rate of dividend
(3) Share Certificate	(c) Correspondence with members
(4) Reply Letter	(d) Dividend Warrant
(5) Bon as Share	(e) Payment of Dividend
	(f) Existing Equity Shareholder
	(g) Bearer Document
	(h) Low rate of dividend
	(i) Fluctuations in the market
	(j) Correspondence with Bank

Answer:

Group ‘A’	Group ‘B’
(1) Secretary	(c) Correspondence with members
(2) Electronic Mode	(e) Payment of Dividend
(3) Share Certificate	(a) Registered Document
(4) Reply Letter	(h) Low rate of dividend
(5) Bon as Share	(f) Existing Equity Shareholder

1C. Write a word or term or a phrase that can substitute each of the following statements.

Question 1.
Return of investment in shares.
Answer:
Dividend

Question 2.
Warrant attached with notice of dividend.
Answer:
Dividend warrant

Question 3.
Title to the shares issued by the company.
Answer:
Share Certificate

Question 4.
A portion of profits is distributed to the shareholders of the company.
Answer:
Dividend

Question 5.
The letter containing reasons for the low rate of dividends.
Answer:
Reply Letter

1D. State whether the following statements are True or False.

Question 1.
Secretary should always provide correct, updated, and tactful information to the members.
Answer:
True

Question 2.
Secretary should disclose confidential information to the members.
Answer:
False

Question 3.
Share certificates must be duly stamped and signed under the common seal of the company.
Answer:
True

Question 4.
The dividend is declared and approved in General Meeting.
Answer:
False

Question 5.
A dividend warrant is a written order given by the company to its banker to pay the amount mentioned in it to the shareholder whose name is specified therein.
Answer:
True

Question 6.
The dividend is to be paid to shareholders within 30 days from the date of declaration.
Answer:
False

1E. Find the odd one.

Question 1.
Allotment letter, Allotment slip, Refund order
Answer:
Refund order

Question 2.
Dividend Coupon, Dividend warrant, Transfer receipt
Answer:
Transfer receipt

1F. Complete the sentences.

Question 1.
Shareholders are the ____________ of the company.
Answer:
owners

Question 2.
The role of secretary while writing to members is of ____________
Answer:
Public Relations Officer

Question 3.
Share Certificate must be signed by at least ____________ directors.
Answer:
2

Question 4.
Dividend is the portion of ____________
Answer:
Profits

Question 10.
Bonus Shares issued are credited to Shareholders ____________ Account.
Answer:
Demat

1G. Select the correct option from the bracket.

Question 1.

Group ‘A’	Group ‘B’
(1) …………………..	(a) Directors and Members
(2) ………………….	(b) Registered Post
(3) Correspondence	(c) …………………….
(4) ………………….	(d) Payment of Dividend Electronically

(Written communication, Share Certificate, Secretary, ECS)
Answer:

Group ‘A’	Group ‘B’
(1) Secretary	(a) Directors and Members
(2) Share Certificate	(b) Registered Post
(3) Correspondence	(c) Written communication
(4) ECS	(d) Payment of Dividend Electronically

1H. Answer in one sentence.

Question 1.
What is politeness?
Answer:
Politeness means the use of courteous language. A courteous letter shows sympathy, respect, and mutual understanding.

Question 2.
What is the time period to dispatch the share certificate by the company?
Answer:
The company must dispatch a share certificate to the allottee within two months after the allotment of shares.

Question 3.
What is the time limit to pay the dividend?
Answer:
The dividend is to be paid to the shareholders within 30 days from the date of declaration.

1I. Correct the underlined word and rewrite the following sentences.

Question 1.
Secretary should follow relevant provisions of the Companies Act, 1986.
Answer:
Secretary should follow relevant provisions of the Board of Directors.

Question 2.
Share Certificate must be countersigned by the shareholder.
Answer:
Share Certificate must be countersigned by the Board of Directors.

Question 3.
The interim dividend is declared by shareholders.
Answer:
The interim dividend is declared by Equity.

Question 4.
Interim Dividend is paid through cash.
Answer:
Interim Dividend is paid through electronic mode.

Question 5.
Rights shares are the shares given to existing shareholders free of cost.
Answer:
Bonus shares are the shares given to existing shareholders free of cost.

1J. Arrange in proper order.

Question 1.
(a) Reply Letter
(b) Issue of Share Certificate
(c) Payment of Dividend
Answer:
(a) Issue of Share Certificate
(b) Payment of Dividend
(c) Reply Letter

2. Explain the following terms/concepts.

Question 1.
Correspondence with Members
Answer:
Correspondence with members means communicating the decisions and information of the management to the members through letters. The letter not only includes casual informing but also sending replies to the letters.

Question 2.
Share Certificate
Answer:
Share Certificate is a registered document. It is a title of shares issued by the company under the common seal of the company. It should be duly stamped and signed by two directors and countersigned by the secretary of the company.

3. Answer in brief.

Question 1.
Explain Secretary acts as a link between the Directors and the Members.
Answer:

• A secretary is an employee in the organization. The secretary is responsible for all the compliances with statutory and regulatory requirements.
• The Secretary communicates the decisions and information taken by the management to the members through correspondence.
• The secretary also deals with government agencies and private institutions.
• The secretary replies to the queries raised by the members. Complaint letters should be promptly attended without any delay by the secretary.
• The secretary should provide correct and updated information to the members as and when demanded.

Question 2.
Write a note on Payment of dividend.
Answer:

• The dividend is the portion of the company’s profits that is distributed among the shareholders of the company.
• The dividend is paid through the Dividend Warrants or through Electronic Mode i.e. ECS, NEFT, etc. to the registered shareholders.
• The rate of dividend is recommended by the Board of Directors.
• It is declared and approved by shareholders in the Annual General Meeting.
• The dividend is to be paid to the shareholders within 30 days from the date of declaration.
• The letter related to the payment of the dividend is sent to the concerned shareholders. The letter contains detailed information regarding the payment of dividends.

4. Justify the following statements.

Question 1.
Share Certificate is a registered document.
Answer:

• Share Certificate is the title to the shares issued by the company.
• It is duly stamped and signed by two directors and countersigned by the directors under the common seal of the company.
• It contains all the details regarding the issue of shares, shareholders’ names, number of shares issued, share certificate number, etc.
• It is issued or dispatched to the allottee within two months after allotment of shares.
• Thus, it is rightly said that a share certificate is a registered document.

Question 2.
Registered shareholders get dividends through dividend warrants or electronically.
Answer:

• The dividend is the portion of profits of the company which is distributed to the shareholders of the company.
• Dividend can be paid through dividend warrant or by means of electronic mode i.e. ECS or NEFT, etc only to the registered shareholders.
• The dividend is paid through dividend coupons to share warrant holders. Share warrant is a bearer document of title to shares.
• The letter contains a number of equity shares held, dividend warrant number, gross dividend, TDS if any, the net amount of dividend, etc.
• Thus, it is rightly said that the registered shareholders get dividends through dividend warrants or electronically.

Question 3.
Reply letter contains or specifies the reasons for the low rate of dividend.
Answer:

• Reply letter is the answer given to the query raised by the member.
• The letter is sent to the concerned member who is not satisfied with the payment of dividend made by the company.
• The letter contains reasons for the lowering down of the rate of dividend.
• The secretary has to convince the shareholders that the dividend is lowered due to void reasons and assure that company will overcome the situation.
• Thus, it is rightly said that a reply letter contains or specifies the reasons for the low rate of dividend.

5. Study the case/situation and express your opinion.

1. As a Secretary of Networks limited.

Question (a).
Give any 3 occasions where he will have to correspond with members.
Answer:
Secretary will correspond with members on the following occasions:

• Issue of Share Certificate;
• Payment of Dividend;
• Reply to query letter

Question (b).
3 points of precautions he will take while drafting a letter.
Answer:
Correct Information, Lucid language, and the image of the company have to be borne in mind.

Question (c).
Whether he should take Director’s approval before drafting any letter.
Answer:
Yes, Director’s approval is a must for major matters.

2. Comfort Motors Ltd. has allotted shares to Mr. Jayant Modi.

Question (a).
Will Comfort Motors Ltd. send a letter to Mr. Jayant Modi when demanded?
Answer:
Yes, when Mr. Jayant Modi demands, the company will send the letter for the issue of the share certificate.

Question (b).
How will the company send the share certificate?
Answer:
The company will send the share certificate through registered post.

Question (c).
What information should the letter of issue of share certificate contain?
Answer:
The letter will contain information such as folio no., share certificate no., no. of shares, etc.

3. Mr. Ashish Rai has applied for 100 shares of ₹ 10 each payable as ₹ 2 per share on application, ₹ 4 on the allotment, and ₹ 4 on 1st and final call.

Question (a).
Is there any compulsion that he get the number of shares he has applied for?
Answer:
No, there is no such compulsion to get the shares. he has applied for. In case of oversubscription he may or may not be allotted shares.

Question (b).
What is the amount he will have to pay at the time of application?
Answer:
Mr. Ashish will have to pay ₹ 200/- (₹ 2/- x 100 shares applied for) at the time of application.

Question (c).
What is the maximum amount he will have to pay for the above shares?
Answer:
₹ 1000/- (₹ 10 * 100 shares) is the maximum amount that Mr. Ashish will have to pay as a total amount for the shares.

4. Anmol Steel Industries Ltd. wants to pay dividends to the registered shareholders.

Question (a).
Who recommends and approves the rate of dividend?
Answer:
The Directors recommends and shareholders approve the rate of dividend.

Question (b).
How will the dividend be paid to the shareholders?
Answer:
The dividend can be paid through a dividend warrant or through electronic mode i.e. ECS, NEFT.

Question (c).
Is the letter sent to the registered shareholders before the payment of the dividend?
Answer:
Yes, the companies send the letter of payment of dividend.

5. Mr. Raj She seeks information about interim dividends as heard from one of his fellow shareholders.

Question (a).
What does the Secretary refer to?
Answer:
The Secretary will refer to the date of dividend notice sent to the members

Question (b).
Does he need to give the details of the interim dividend?
Answer:
Yes, he can give the date and details sent.

Question (c).
After getting the details does Mr. Raj have any document that he can refer to as proof?
Answer:
Mr. Rajat can verify with the Passbook/ statement as regards the amount credited.

6. Yash Industries Ltd. is issuing bonus shares.

Question (a).
Can the company issues bonus shares to all the shareholders?
Answer:
No, the company can issue bonus shares to only existing shareholders of the company.

Question (b).
Is it necessary for the shareholders to pay a certain amount for bonus shares?
Answer:
No, bonus shares are fully paid up shares given by the company as a gift.

Question (c).
How are the shareholders informed regarding the issue of bonus shares?
Answer:
Bonus Shares are credited to shareholders Demat Account or share certificate is issued if shares are held in physical form.

7. Mr.Anand Khot has complained of a low rate of dividends. As a Secretary,

Question (a).
Is it necessary to reply to the complaint letter?
Answer:
Yes, it is a must for the Secretary to send a reply letter.

Question (b).
Is it necessary to give reasons for the low rate of dividends?
Answer:
Yes, reasons, as discussed in the AGM, can be written in the letter in order to convince about the low rate of dividend.

Question (c).
Which points he has to keep in mind while drafting the above letter?
Answer:
Prompt response, correct information, politeness, and image of the company are some of the major points to be considered while drafting the letter.

Balbharti Maharashtra State Board Class 12 Secretarial Practice Important Questions Chapter 5 Deposits Important Questions and Answers.

Maharashtra State Board 12th Secretarial Practice Important Questions Chapter 5 Deposits

1A. Select the correct answer from the options given below and rewrite the statements.

Question 1.
No Deposits can be repaid before ___________ months.
(a) One
(b) Six
(c) Nine
Answer:
(b) Six

Question 2.
Premature deposits can be repaid after ___________ months.
(a) Two
(b) Three
(c) Four
Answer:
(b) Three

Question 3.
___________ company cannot collect deposits from the public.
(a) Private
(b) Public
(c) Government
Answer:
(a) Private

Question 4.
A copy of return of deposits must be sent to ___________
(a) Registrar of companies
(b) Govt of India
(c) State bank of India
Answer:
(a) Registrar of companies

Question 5.
On acceptance of deposits, companies required to keep register of deposits at ___________
(a) Office of the registrar
(b) Sebi office
(c) Registered office of the company
Answer:
(c) Registered office of the company

1B. Match the pairs.

Question 1.

Group ‘A’	Group ‘B’
(1) Return of deposits	(a) Cognisable criminal offense
(2) Deposits in contravention of the law	(b) 25% of paid-up capital
(3) Deposit Repayment Reserve Account	(c) Delivered to the registrar of companies
(4) Registrar of Companies	(d) Approved by the SEBI
(5) Eligible public company	(e) Filing of circular or advertisement
	(f) On or before 30th June every year
	(g) Not less than 15%
	(h) Net worth 100 crores
	(i) Net worth 10 crores
	(j) No punishment

Answer:

Group ‘A’	Group ‘B’
(1) Return of deposits	(f) On or before 30th June every year
(2) Deposits in contravention of the law	(a) Cognisable criminal offense
(3) Deposit Repayment Reserve Account	(g) Not less than 15%
(4) Registrar of Companies	(e) Filing of circular or advertisement
(5) Eligible public company	(h) Net worth 100 crores

Question 2.

Group ‘A’	Group ‘B’
(1) Private company	(a) Approval of secretary
(2) Period of deposit	(b) Can accept deposits from the public
(3) Validity of advertisement	(c) Can accept deposits from its members or relatives of directors or directors
(4) Board of directors	(d) Six months to three years
(5) Public company	(e) Approval of depositors
	(f) Right to accept deposits
	(g) Six months to forty-two months
	(h) Refund of deposit before maturity
	(i) Ten months after the expiry of the financial year
	(j) Six months after the expiry of the financial year

Answer:

Group ‘A’	Group ‘B’
(1) Private company	(c) Can accept deposits from its members or relatives of directors or directors
(2) Period of deposit	(d) Six months to three years
(3) Validity of advertisement	(j) Six months after the expiry of the financial year
(4) Board of directors	(f) Right to accept deposits
(5) Public company	(b) Can accept deposits from the public

1C. Write a word or a term or a phrase that can substitute each of the following statements.

Question 1.
An acknowledgment of deposit accepted by the company.
Answer:
Deposit Receipt

Question 2.
Type of company that can accept deposits from members as well as the public.
Answer:
Eligible Public Company

Question 3.
A record of details of public deposits maintained by the company.
Answer:
Deposit register

Question 4.
The authority which has the power to accept deposits.
Answer:
Board of Directors

Question 5.
Return on investments as deposits in the company.
Answer:
Interest

Question 6.
Time within which company has to issue deposit receipt.
Answer:
30 Days

1D. State whether the following statements are true or false.

Question 1.
A company can accept deposits payable on demand.
Answer:
False

Question 2.
A company can accept deposits to any extent.
Answer:
False

Question 3.
Acceptance of deposits is the same as a renewal.
Answer:
False

Question 4.
Deposits may be secured or unsecured.
Answer:
True

1E. Find the odd one.

Question 1.
Record of deposits registered office of the companies, Registrar of companies.
Answer:
Registrar of companies

Question 2.
Six months, thirty-six months, forty-two months.
Answer:
Forty-two months

Question 3.
Advertisements, Circular, Board meeting.
Answer:
Board meetings

Question 4.
English newspaper, application for, a regional newspaper.
Answer:
Application forms

Question 5.
Return of deposits, stock exchange, registrar of companies.
Answer:
Stock Exchange

1F. Answer in one sentence.

Question 1.
Are loans and deposits the same?
Answer:
Loans mean any sum borrowed by a company. It has to be repaid instantly or when demanded by the lender whereas deposits are sums borrowed by the company but repaid only on a fixed maturity date.

2. Explain the following terms/concepts.

Question 1.
Register of Deposit
Answer:

• Register for Deposits records all the deposits accepted or renewed by the company at its registered office.
• The details of the deposit along with the details of the depositors should be entered in the Register of Deposits within seven days from the date of issue of the Deposit Receipt.

Question 2.
Interest on Deposit
Answer:

• The return received on the deposit amount is called Interest on Deposit.
• The rate of interest on deposits is decided by the Reserve Bank of India from time to time.
• In case of premature repayment of deposits, the company can deduct 1% interest from the rate of interest stated at the time of acceptance of the deposit.

3. Answer in brief.

Question 1.
Explain the provisions related to the period/tenure of deposits.
Answer:
The provisions related to the period/tenure of deposits are as follows:

• No deposit can be accepted or renewed which is to be repaid within a period of six months or more than thirty-six months.
• In certain circumstances, a company may accept deposits repayable earlier than six months to meet its short-term needs.
• Such deposits must have a tenure of a minimum of three months and the number of such deposits cannot be more than 10% of the aggregate of the paid-up share capital and free reserves of the company.
• Under certain circumstances, at the request of the depositor, the company makes premature repayment of deposits.
• The company may also renew its deposits with the same terms of issue and it will be considered as fresh deposits.

4. Answer the following questions.

Question 1.
Explain terms and conditions for acceptance of deposits.
Answer:
The company raises funds by accepting deposits from the public and its own members. In other words, it is a short-term loan taken by the company. The company can collect deposits on certain terms and conditions laid down by the Companies Act 2013. They are as follows:
(i) Amount of Deposit:
Different types of companies have different terms and conditions for accepting deposits.
The following table shows the percentage of acceptance of deposits.

Companies	Acceptance of Deposits (%)
(i) Private Company	Up to 100% of the aggregate of paid-up share capital and free reserves
(ii) Public Company	Should not exceed 25% of the aggregate of paid-up capital and free resources
(iii) Eligible Public Company	Should not exceed 25% of the aggregate paid-up capital and free reserves in case of the public. Should not exceed 10% of the aggregate paid-up capital and free reserves in case of members.
(iv) Government Company	Should not exceed 35% of the paid-up share capital and free reserves.

(ii) Period/Tenure of Deposit:

• The tenure of deposit should be more than six months but less than thirty-six months.
• Under certain circumstances only, the company can accept deposits for 3 months.
• The company can make premature repayment of deposits after a minimum of 3 months.

(iii) No demand deposit:
The company cannot accept or renew deposits repayable on demand.

(iv) Secured or Unsecured Deposit:

• A company can accept secured or unsecured deposit if it is mentioned in the circular or advertisement.
• A company offering secured deposits has the right to create a charge on its tangible assets within 30 days of acceptance of deposits.

(v) Application Form:

• A prescribed application form is to be filled by the applicant.
• This application form is given by the company.
• It includes a declaration made by the applicant that the deposit he is making is not borrowed from any person.

(vi) Joint names:
The company can accept deposits in joint names of depositors. But there should not be more than three names.

(vii) Nomination:

• Every depositor has to nominate at least one person.
• Nominee enjoys all the benefits in the event of death of the depositor.

(viii) Circular or Advertisement:

• If a company invites deposits from its members, it issues a circular. But if it invites deposits from the public, the company has to issue an advertisement.
• A copy of the circular or advertisement signed by all directors must be filed with the Registrar of Companies.
• Issue of Circular or Advertisement:
• The company has to publish the advertisement in one English newspaper and one vernacular/regional newspaper.

(ix) Appointment of Deposit Trustee:

• Deposit Trustee is appointed by the public companies and eligible public companies.
• One of the more deposit trustees is appointed if secured deposits are issued.
• A contract ‘Trust Deed’ is signed between the company and deposit trustee.

(x) Create charge on assets:
The company secured deposits create a charge on its tangible assets within 30 days of acceptance.

(xi) Deposit Insurance:

• A company needs to take Deposit Insurance at least 30 days before the issue of circular or advertisement.
• Deposit insurance is necessary only when the deposit amount plus interest is up to ₹ 20,000.

(xii) To obtain a credit rating:

• The company obtains credit ratings for deposits on the basis of the net worth of the company, liquidating position, ability to repay deposits on time, etc.
• Credit rating for deposits is given by credit rating agencies.
• The rating is done every year during the tenure of deposits.

(xiii) Open Deposit Repayment Reserve Account:

• A Deposit Repayment Reserve Account is opened in a scheduled bank for accepting deposits.
• The company has to deposit an amount up to 15% of the number of deposits maturing during the current year and following the financial year on or before 30th April.
• This account is used only for repaying deposits only.

(xiv) Deposit Receipt:

• Deposit Receipt is to be issued to the depositors within 21 days from the date of receipt of money or realization of cheque.
• The receipt has to be signed by the officer duly authorized by the Board of Directors.
• The Receipt contains the name and address of the depositor, amount of deposit, rate of interest payable, and date on which it is repayable.

(xv) Register of Deposit:

• Register of Deposit is to be maintained by the company at its registered office.
• The details of the deposit accepted and renewal of the deposits are recorded and depositors are recorded in the Register of Deposits.
• Deposit Receipt should be issued within 7 days from the date of issue.

(xvi) Return of Deposit:

• A Return of Deposit should be recorded with the company on or before 30th June every year.
• The return includes details of deposit with the company as of 31st March of that year.

(xvii) Interest:

• Reserve Bank of India decides the rate of interest on deposits.
• The rate of interest changes from time to time.
• In case of premature repayment of deposits, the company can deduct 1% interest from the rate of interest.
• If the deposits matured and are claimed but remain unpaid by the company, the company will be liable to pay a penal rate of interest at the rate of 18%.

(xviii) Right to alter the Terms and Conditions:
A company cannot directly or indirectly alter the terms and conditions of the Deposit, Deposit Trust Deed, and Deposit Insurance, once the circular or advertisement is issued and deposits are accepted.

(xix) Disclosure in financial statements:
Deposit money received by the company from the Directors or the relatives of the Directors (in the case of a private company) should be disclosed in the company financial statement.

(xx) Punishment:

• As per the Companies Act, an officer of the company will be liable for punishment if found guilty / not fulfilling the provisions of the Act.
• The Maharashtra Protection of Interest of Depositors (in Financial establishments) Act, 1999 has the right to take action against companies and also punish if found guilty.
• Companies in Maharashtra will be punished for contravening the provisions of this Act also.

Question 2.
Explain the procedure for accepting deposits from the public.
Answer:
Only Eligible Public Companies can accept deposits from the public.
Following is the procedure to be followed for accepting deposits from the public:
(i) Hold Board Meeting:

• A resolution is passed in the Board Meeting for accepting the deposits from the public.
• The details of the amount of deposit, terms and conditions of issue, etc. are decided in this meeting.

(ii) Hold a General Meeting:

• A General Meeting is held by the company for seeking shareholders’ approval for accepting deposits.
• A Special resolution is passed and filed with the Registrar of Companies and if needed with RBI also.

(iii) Hold Board Meeting:

• The board meeting is held to verify and approve the draft of the advertisement.
• The draft should be signed by the majority of the Directors of the company.

(iv) Appoint Banker:
The company has to appoint a banker so that the applicants can submit their application form along with the deposit money.

(v) Obtain Credit Rating:

• The company has to obtain a Credit rating from a recognized Credit Rating Agency.
• This helps in the goodwill of the company.
• This credit rating should be mentioned in the advertisement.

(vi) Appoint Deposit Trustee:

• The company appoints Deposit Trustees when secured deposits are issued.
• It is a contract between the company and Trustee i.e. Deposit Trustee Deed.
• The Deposit Trust deed contains the terms and conditions.
• The deed has to be signed at least 7 days before issuing the advertisement.

(vii) Take Deposit Insurance:

• The company enters into an agreement with the Insurance Company 30 days before issuing the advertisement.
• Deposit Insurance is necessary only when the deposit amount plus interest exceeds ₹ 20,000.

(viii) File a copy of the advertisement with the Registrar of Companies:

• A copy of the advertisement has to be filed with the Registrar of Companies.
• The company can publish the advertisement after 30 days of filing.

(ix) Advertisement to the Public:

• Advertisement is published for the public after 30 days of filing with the Registrar of Companies.
• The advertisement has to be published in one English newspaper and one regional newspaper having wide circulation in the state where the company’s registered office is located.

(x) Upload the advertisement on the company’s website:
After releasing the advertisement to the public, it is also necessary to upload it on the company’s website.

(xi) Collect application form and money:
The banker collects the application forms along with the deposit money on behalf of the company.

(xii) Issue Deposit Receipt:
The company has to issue a Deposit Receipt within 21 days from the date of receipt of money or realization of cheque.

(xiii) Create charge on assets:

• The company issuing secured deposits can create charges on its assets.
• This charge of assets should be created within 30 days of acceptance of the deposit.

(xiv) Make entries in Register of Deposits:
Secretary has to record the details of deposits in the Register of Deposits within the 7 days after issuing the Deposit Receipt.
The entries have to be verified by an authorized officer.

(xv) File Return of Deposits with Registrar of Companies:

• The company has to file a Return of Deposit before 30th June every year.
• The return has details of Deposits with the company as of 31st March

Question 3.
Explain the procedure for accepting deposits from members.
Answer:
The following is the procedure to accept deposits from members:
(i) Hold Board Meeting:

• Secretary arranges for a Board Meeting.
• In the Board Meeting, decisions regarding the amount of deposit, terms of issue of deposit, etc. are decided.

(ii) Hold a General Meeting:

• To seek approval from shareholders for accepting deposits, the company holds a general meeting.
• A special resolution is passed in a general meeting and filed with the Registrar of Companies.

(iii) Hold Board Meeting:

• After getting shareholder’s approval, a Board meeting is held to approve the draft of the circular for inviting applications for deposits from members.
• The draft should be signed by a majority of the Directors of the company.

(iv) Appoint Banker:
A Banker is appointed for collecting the application forms and the Deposit money on behalf of the company.

(v) Obtain Credit Rating:

• Credit rating agencies rate the company’s deposit.
• The rating given by the Credit Rating Agency must be mentioned in the circular.

(vi) Appoint Deposit Trustee:

• Company appoints Deposit Trustees.
• The Trust deed is signed at least 7 days before issuing the circular.

(vii) Take Deposit Insurance:
The company takes Deposit Insurance at least 30 days before issuing the circular.

(viii) File a copy with Registrar of Companies:
A copy of the circular is filed with the Registrar of Companies and can be issued to the members-only after 30 days of filing.

(ix) Issue circular to members:

• The company sends the circulars to members after 30 days of filing them with the Registrar.
• It is sent by registered post, speed post, or email. The company may even publish the circular in newspapers.

(x) Upload circular on company’s website:

• An eligible public company has to upload the circular on the company’s website.
• Whereas, it is optional for a private company and other public companies.

(xi) Collect application form and money:
The company informs the bank to collect the application form along with the deposit money.

(xii) Issue of Deposit Receipt:

• A deposit Receipt is issued by the company.
• It should be issued within 21 days from the date of receipt of the money or realization of the cheque.

(xiii) Create charge on assets:
If a company accepts secured deposits, it has to create a charge on its assets within 30 days of acceptance of deposits.

(xiv) Make entries in Register of Deposits:

• Secretary has to enter the details of deposits in the Register of Depositors within 7 days from the date of issue of Deposit Receipt.
• It has to be signed by an authorized officer.

(xv) File Return of Deposits:

• The company has to file a return of deposit before 30th June every year.
• The return has details of deposits with the company as of 31st March.

Balbharti Maharashtra State Board 12th Physics Important Questions Chapter 7 Wave Optics Important Questions and Answers.

Maharashtra State Board 12th Physics Important Questions Chapter 7 Wave Optics

Question 1.
Name the various theories of the nature of light.
Answer:
The various theories of the nature of light from the 17th century to the modern times are

1. Descartes’ corpuscular theory of light (1637)
2. Newton’s corpuscular theory (1666)
3. Huygens’ wave theory of light propagation (1678), modified, verified and put on a firm mathematical base by Young, Fraunhofer, Fresnel and Kirchhoff (in the 1800s)
4. Maxwell’s electromagnetic theory (1865)
5. the light quantum, i.e., the photon model of the modern quantum theory by Planck (1900) and Einstein (1905).

Question 2.
State the postulates of Newton’s corpuscular theory of light.
Answer:
Sir Isaac Newton developed the corpuscular theory of light proposed by Rene’ Descartes (1596-1650), French philosopher and mathematician. The theory assumed that light consists of a stream of corpuscles emitted by a luminous source.
Postulates of Newton’s corpuscular theory of light:

1. Light corpuscles are minute, light and perfectly elastic particles.
2. A luminous source emits light corpuscles in all directions which then travel at high speed in straight lines in a given medium.
3. The constituent colours of white light are due to different sizes of the corpuscles.
4. The light corpuscles stimulate the sense of sight on their impact on the retina of the eye.
5. A reflective surface exerts a force of repulsion normal to the surface on the light corpuscles when they strike the surface.
6. A transparent medium exerts a force of attraction normal to the surface on the light corpuscles striking the surface. This force is different for different mediums.

Notes :

1. A consequence of the assumption (6) is that, according to the corpuscular theory, the speed of light in a denser medium is greater than that in air and has different values for different mediums.
2. It was known from earliest recorded times that when light is incident on the surface of glass or water, it is partly reflected and partly transmitted, simultaneously. To explain this, Newton postulated that the corpuscles must have fits of easy reflection and fits of easy transmission and must pass periodically from one state to the other.

Question 3.
State the drawbacks of Newton’s corpuscular theory of light.
Answer:
Drawbacks of Newton’s corpuscular theory of light:

1. The theory predicted that the speed of light in a
   denser medium should be greater than that in air. This was disproved when experiment showed that the speed of light in water is less than that in air (carried out in 1850 by French physicist Jean Bernard Leon Foucault). ,
2. The theory could not satisfactorily explain the phenomenon of polarization and the simultaneousness of reflection and refraction.
3. The corpuscular theory failed to explain the phenomena of diffraction and interference.
4. There was no basis for the hypothesis that the constituent colours of white light are due to different sized corpuscles.

Question 4.
What is a ray of light?
Answer:
A ray of light is the path along which light energy is transmitted from one point to another in an optical system.

Question 5.
What is meant by ray optics or geometrical optics?
Answer:
The formation of

1. shadows, and
2. images by mirrors and lenses can be explained by assuming that light propagates in a straight line in terms of rays. The study of optical phenomena under this assumption is called ray optics. It is also called geometrical optics as geometry is used in this study.

Question 6.
Give a brief account of Huygens’ wave theory of light. State its merits and demerits.
Answer:
Huygens’ wave theory of light [Christiaan Huygens (1629-95), Dutch physicist] :

1. Light emitted by a source propagates in the form of waves. Huygens’ original theory assumed them to be longitudinal waves.
2. In a homogeneous isotropic medium, light from a point source spreads by spherical waves.
3. It was presumed that a wave motion needed a medium for its propagation. Hence, the theory postulated a medium called luminiferous ether that exists everywhere, in vacuum as well as in transparent bodies. Ether had to be assigned some extraordinary properties, a high modulus of elasticity (to account for the high speed of light), zero density (so that it offers no resistance to planetary motion) and perfect transparency.
4. The different colours of light are due to different wavelengths.

Merits :

1. Huygens’ wave theory satisfactorily explains reflection and refraction as well as their simultaneity.
2. In explaining refraction, the theory concludes that the speed of light in a denser medium is less than that in a rarer medium, in agreement with experimental findings.
3. The theory was later used by Young in 1800-04, Fraunhofer and Fresnel in 1814 to satisfactorily explain interference, diffraction and rectilinear propagation of light. The phenomenon of polarization could also be explained considering the light waves to be transverse.

Demerits :

1. It was found much later that the hypothetical medium, luminiferous ether, has no experimental basis. Einstein discarded the idea of ether completely in 1905.
2. Phenomena like absorption and emission of light, photoelectric effect and Compton effect, cannot be explained on the basis of the wave theory.

[Note: To decide between the particle and wave theories of light, Dominique Francois Jean Arago (1786-1853), French physicist, suggested the measurement of the speed of light in air and water. The experiment was performed in 1850 by Leon Foucault (1819-68), French physicist, using Arago’s experimental equipment. He found that the speed of light in water is less than that in air.]

Question 7.
What is meant by wave optics?
Answer:
It is not possible to explain certain phenomena of light, such as interference, diffraction and polarization, with the help of ray optics (geometrical optics). The branch of optics which uses wave nature of light to explain these optical phenomena is called wave optics.

Question 8.
What was Maxwell’s concept of light?
Answer:
In 1865, James Clerk Maxwell developed a mathematical theory on the intimate relationship between electricity and magnetism. His theory predicted light to be a high-frequency transverse electromagnetic wave in ether. Electric and magnetic fields in the wave vary periodically in space and time at right angles to each other and to the direction of propagation of the wave.

The speed of the electromagnetic waves in a medium, as calculated on the basis of Maxwell’s theory, was experimentally found to be equal to the measured speed of light in that medium. Maxwell’s electromagnetic theory of light, with addition by others till 1896, could account for all the known phenomena regarding the propagation (or transmission) of light through space and through matter.

Question 9.
What is the photon model or quantum hypothesis of light ?
Answer:
To explain the interaction of light and matter (as in the emission or absorption of radiation), Max Planck, in 1900, and Einstein, in 1905, hypothesized light as concentrated or localized packets of energy. Such an energy packet is called a quantum of energy, which was given the name photon much later, in 1926, by Frithiof Wolfers and Gilbert Newton Lewis. For a radiation of frequency v, a quantum of energy is hv, where h is a universal constant, now called Planck’s constant.

[Note : ‘Localisation’ of energy in a region gives light its particle nature while frequency is a wave characteristic. The complementary properties of particle and wave of light quanta are reconciled as follows : light propagates as wave but interacts with matter as particle.]

Question 10.
Give a brief account of the wave nature of light.
Answer:

1. Light is a transverse, electromagnetic wave.
2. A light wave consists of oscillating electric and magnetic fields that are perpendicular to each other and also perpendicular to the direction of propagation of the wave.
3. Like all other electromagnetic waves, light waves do not require any material medium as they can travel even through vacuum.
4. In a material medium, the speed of light depends on the refractive index of the medium, which, in turn depends on the permeability and permittivity of the medium.

Question 11.
Define absolute refractive index of a medium.
Answer:
The absolute refractive index of a medium is defined as the ratio of the speed of light in vacuum to the speed of light in the medium.
[Note : Absolute refractive index of a medium (n) =

The absolute refractive index of vacuum is 1 (by definition) and that of air is greater than 1, but very nearly equal to 1.]

Question 12.
State the characteristics of the electromagnetic waves.
Answer:

1. The electromagnetic waves are transverse in nature as they propagate by oscillating electric and magnetic fields that are perpendicular to each other and also perpendicular to the direction of propagation of the wave.
2. These waves do not require any material medium for their propagation, i.e., they can travel even through vacuum.
3. The wavelength of the electromagnetic waves ranges from very small (< 1 fm) to very large (> 1 km). The waves are classified in the order of increasing wavelength as γ-rays, X rays, ultraviolet, visible, infrared, microwave and radio waves.
4. In vacuum, the speed of electromagnetic waves does not depend on the frequency of the wave. But, in a material medium, it depends on the frequency. For a given frequency, the speed is different in different mediums.
   [Note : 1 fm (femtometre) = 10^-15 m]

Question 13.
Define and explain :
(a) a wave normal
(b) a ray of light.
Answer:
(a) Wavenormal: A wave normal at a point on a wavefront is defined as a line drawn perpendicular to the wavefront in the direction of propagation of the wavefront.

In a homogeneous isotropic medium, a wavefront moves parallel to itself. Thus, at any point in the medium, the direction in which the wavefront moves is always perpendicular to the wavefront at that point. This direction is given by the wave normal at that point.

(b) Ray of light: The direction in which light is propagated is called a ray of light.

This term (ray of light) is also used to mean a narrow beam of light waves. Only in a homogeneous isotropic medium is a ray of light the same as a wave normal. For spherical wavefronts spreading out from a point source, the rays are radially divergent. The rays corresponding to a plane’ wavefront form a parallel beam.

Question 14.
What is a cylindrical wavefront? Draw the corresponding diagram.
Answer:
Cylindrical wavefront: An extended linear source, such as an aperture in the form of a narrow slit, gives rise to cylindrical wavefronts. All the points equidistant from the source lie on the curved surface of a cylinder. Thus, the shape of the wavefront is cylindrical.

Question 15.
What is a plane wavefront? Draw the corresponding diagram.
Answer:
Plane wavefront: It may be treated as a part of a spherical or cylindrical wavefront at a very great distance from the source, such that the wavefront has a negligible curvature.

Question 16.
Draw a neat labelled diagram illustrating spherical wavefronts corresponding to a diverging beam of light.
Answer:

Note : Lenses can be used to obtain

1. a converging beam of light
2. a diverging beam of light.

Question 17.
State Huygens’ principle.
Answer:
Huygens’ principle : Every point on a wavefront acts as a secondary source of light and sends out secondary wavelets in all directions. The secondary wavelets travel with the speed of light in the medium. These wavelets are effective only in the forward direction and not in the backward direction. At any instant, the forward-going envelope or the surface of tangency to these wavelets gives the position of the new wavefront at that instant.

Question 18.
Explain the construction and propagation of a plane wavefront using Huygens’ principle.
Answer:
Huygens’ construction nf a plane wavefront: A plane wavefront may be treated as a part of a spherical or cylindrical wave at a very great distance from a point source or an extended source, such that the wavefront has a negligible curvature. Let A, B, C, D, …, be points on a plane wavefront in a homogeneous isotropic medium in which the speed of light, taken to be monochromatic, is v.

In a time, t = T, secondary wavelets with points A, B, C, D,…, as secondary sources travel a distance vT. To find the position of the wavefront after a time t = T, we draw spheres of radii vT with A, B, C,…, as centres. The envelope or the surface of tangency to these spheres is a plane A’B’C’. This plane, the new wavefront, is at a perpendicular distance vT from the original wavefront in the direction of propagation of the wave. Thus, in an isotropic medium, plane wavefronts are propagated as planes.

Question 19.
Explain the construction and propagation of a spherical wavefront using Huygens’ principle.
Answer:
Huygens’ construction of a spherical wavefront: Consider a point source of monochromatic light S in a homogeneous isotropic medium. The light waves travel with the same speed v in all directions. After time f, the wave will reach all the points which are at a distance vt from S. This is spherical wavefront XY. Let, A, B, C, …,, be points on this wavefront.

To find the new wavefront after time T, we draw spheres of radius vT with A, B, C,…, as centres. The envelope or the surface of tangency of these spheres is the surface A’B’C’. This is the new spherical wavefront X’Y’. Thus, in an isotropic medium, spherical wavefronts are propagated as concentric spheres.

Question 20.
Suppose a parallel beam of monochromatic light is incident normally at a boundary separating two media. Explain what happens to the wavelength and frequency of the light as it propagates from medium 1 to medium 2. What happens when the medium 1 is vacuum ?
Answer:
Consider a parallel beam of monochromatic light incident normally on interface PQ separating a rarer medium (medium 1) and a denser medium (medium 2).

The three successive wavefronts AB, CD and EF are separated by a distance λ₁, the wavelength of light in first medium. The corresponding three wavefronts after refraction, are A’B’, C’D’ and E’F’. Due to the denser medium, the speed of light reduces and hence the wavefronts cover a less distance than that covered in the same time in the first medium. Thus, the wavefronts are comparitively more closely spaced than in the first medium. This distance between successive wavefronts is λ₂, the wavelength of light in the second medium. Thus, λ₂ is less than λ₁,. To find the relation between λ₁ and λ₂, let us consider the wavefront AB reaching

PQ at time t = 0. The next wavefront CD, separated from AB by distance λ₁, will reach PQ at time t = T. Let v₁ and v₂ be the speeds of light in medium 1 and medium 2 respectively. T is the time during which light covers distance λ₁ in medium 1 and λ₂ in medium 2.

This shows how the wavelength of light changes in refraction.

If the first medium is vacuum where the wavelength of light is λ₀ and n is the absolute refractive index of medium 2, then
λ₂ = λ₀\(\left(\frac{v_{2}}{c}\right)\) = \(\frac{\lambda_{0}}{n}\) (as v₁ = c) …(3)
Now, speed = frequency × wavelength.
Hence, the ratio of the frequencies v₁ and v₂, of the wave in the two mediums can be written using
EQ. (2) as, \(\frac{v_{1}}{v_{2}}\) = \(\frac{v_{1} / \lambda_{1}}{v_{2} / \lambda_{2}}\) = 1 …(4)

Thus, the frequency of a wave remains unchanged while going from one medium to another. Thus, v₀ = v₁ = v₂, where v₀ is the frequency of light in vacuum.

Question 21.
The refractive indices of diamond and water with respect to air are 2.4 and 4/3 respectively. What is the refractive index of diamond with respect to water ?
Answer:

is the refractive index of diamond with respect to water.

Question 22.
What is the refractive index of water with respect to diamond ? For data, see Question 21. above.
Answer:

is the refractive index of water with respect to diamond.

Question 23.
What happens to the frequency, wavelength and speed of light as it passes from one medium to another?
Answer:
The wavelength and speed of light change, but the frequency remains the same.

Question 24.
The refractive index of water with respect to air, for light of wavelength Aa in air, is 4/3. What is the wavelength of the light in water?
Answer:

as the wavelength of the light in water.

Question 25.
If the frequency of certain light is 6 × 10¹⁴ Hz, what is its wavelength in free space ? [c = 3 × 10⁸ m/s]
Answer:

is the required wavelength.

Question 26.
If the wavelength of certain light in air is 5000 Å and that in a certain medium is 4000 Å, what is the refractive index of the medium with respect to air ?
Answer:

is the refractive index of the medium with respect to air.

Data : c = 3 × 10⁸ m/s

Question 27.
Solve the following :

Question 1.
If the refractive index of glass is 3/2 and that of water is 4/3 respectively, find the speed of light in glass and in water.
Solution:
Let n_g and n_w be the refractive indices of glass and water respectively. Also let v_a, v_g and v_w be the speeds of light in air, glass and water, respectively.

Question 2.
The refractive indices of water and diamond are 4/3 and 2.42 respectively. Find the speed of light in water and diamond.
Solution :

This is the speed of light in diamond.

Question 3.
The refractive indices of glass and water with respect to air are \(\frac{3}{2}\) and \(\frac{4}{3}\), respectively. Determine the refractive index of glass with respect to water.
Solution :


This is the refractive index of glass with respect to water.

Question 4.
A diamond (refractive index = 2.42) is dipped into a liquid of refractive index 1.4. Find the refractive index of diamond with respect to the liquid.
Solution :
Data : n_d = 2.42, n₁ = 1.4
The refractive index of diamond with respect to liquid,

Question 5.
The refractive index of glycerine is 1.46. What is the speed of light in glycerine ? [Speed of light in vacuum = 3 × 10⁸ m/s]
Solution :
Data : n_g = 1.46, c = 3 × 10⁸ m/s c
n_g = \(\frac{c}{v}\)
v = \(\frac{c}{n}\) = \(\frac{3 \times 10^{8}}{1.46}\) = 2.055 × 10⁸ m/s
This is the speed of light in glycerine.

Question 6.
The refractive indices of glycerine and diamond with respect to air are 1.46 and 2.42 respectively. Calculate the speed of light in glycerine and in diamond. From these calculate the refractive index of diamond with respect to glycerine.
Solution:
Let n_g and n_d be the refractive indices of glycerine and diamond respectively. Also, let v_a, v_g and v_d be the speeds of light in air, glycerine and diamond respectively.
Data : v_a = 3 × 10⁸ m/s, n_g = 1.46, n_d = 2.42
(i) Refractive index of glycerine with respect to air,

(ii) Refractive index of diamond with respect to air,
n_d = \(\frac{v_{\mathrm{a}}}{v_{\mathrm{d}}}\)

(iii) Refractive index of diamond with respect to glycerine,

Question 7.
The wavelengths of a certain light in air and in a medium are 4560 Å and 3648 Å, respectively. Compare the speed of light in air with its speed in the medium.
Solution:
Let v_a and v_m be the speeds of light in air and in the medium respectively and let λ_a and λ_m be the wavelengths of light in air and in the medium respectively. Let v be the frequency of light in air.
When light passes from one medium to another, its frequency remains unchanged.

Question 8.
Monochromatic light of wavelength 632.8 nm is incident from air on a water surface. What are the wavelength, frequency and speed of (a) reflected and (b) refracted light ? [Given : Refractive index of water = 1.33]
Solution:
Let v be the frequency of the light, and λ₁ and λ₂ the wavelengths of reflected and refracted light respectively.
Data : λ₁ = 632.8 nm = 632.8 × 10^-9 m,
c = 3 × 10⁸ m/s, _an_w = 1.33
(a) For reflected light:
When the wave travels in air, its speed v₁ = c

(b) For refracted light:
Frequency does not change while going from one medium to other.
∴ V = 4.741 × 10¹⁴ Hz

This is the speed of the light in water.

Question 9.
The refractive indices of water for red and violet colours are 1.325 and 1.334, respectively. Find the difference between the speeds of the rays of these two colours in water. [c = 3 × 10⁸ m/s]
Solution :
Data : n_r = 1.325, n_v = 1.334, c = 3 × 10⁸ m/s

Question 10.
If the difference in speeds of light in glass and water is 2.505 × 10⁷ m/s, find the speed of light in air. [Refractive index of glass = 1.5, refractive index of water = 1.333]
Solution :


This is the speed of light in air.

Question 11.
If the difference in speeds of light in glass and water is 0.25 × 10⁸ m/s, find the speed of light in air. [n_g = 1.5 and n_w = \(\frac{4}{3}\)]
Solution :

∴ The speed of light in air, c = 12 (n_w – v_g)
= 12 × 0.25 × 10⁸
= 3 × 10⁸ m/s

Question 12.
Red light of wavelength 6400 Å in air has wavelength 4000 Å in glass. If the wavelength of violet light in air is 4400 Å, what is its wavelength in glass? Assume that the glass has the same refractive index for red and violet colours.
Solution :

[ Note : This problem was asked in Board examination in October 2014. The assumption n_r(glass) = n_v(glass) is manifestly gross. No glass can have the same refractive index for red and violet colours.]

Question 13.
A ray of light passes from air to glass. If the angle of incidence is 74° and the angle of refraction is 37°, find the refractive index of the glass.
Solution :
Data : i = 74°, r = 37°
n = \(\frac{\sin i}{\sin r}\) = \(\frac{\sin 74^{\circ}}{\sin 37^{\circ}}\) = \(\frac{0.9613}{0.6018}\)
∴ n = 1.597
This is the refractive index of the glass.

Question 14.
A ray of light is incident on a glass slab making an angle of 30° with the surface. Calculate the angle of refraction in glass and the speed of light in glass. The refractive index of glass and speed of light in air are 1.5 and 3 × 10⁸ m/s, respectively. Solution :
Data : v_air = 3 × 10⁸ m/s; n = 1.5
The angle of incidence (i) is the angle made by the incident ray with the normal drawn to the refracting surface.
∴ i = 90° – 30° = 60°
(a) Angle of refraction (r) in glass :

(b) Speed of light (v_glass) in glass :

Question 15.
A ray of light is incident on a water surface of refractive index \(\frac{4}{3}\) making an angle of 40° with the surface. Find the angle of refraction.
Solution :

∴ The angle of refraction, r = sin^-1(0.5745) = 35°4′

Question 16.
A ray of light travelling in air is incident on a glass slab making an angle of 30° with the surface. Calculate the angle by which the refracted ray in glass is deviated from its original path and the speed of light in glass [Refractive index of glass = 1.5].
Solution:
Solve for the speed (ug) of light in glass and the angle of refraction (r) in glass as in Solved Problem (14). ug = 2 × 10⁸ m/s and r = 35°16′
The angle of incidence (z), i.e., the angle between the incident ray and the normal to the glass surface, is i = 90° – 30° = 60°.
Hence, the angle by which the refracted ray is deviated from the original path is δ = i – r = 60° – 35°16′ = 24°44′

Question 17.
The wavelength of blue light in air is 4500 A. What is its frequency? If the refractive index of glass for blue light is 1.55, what will be the wavelength of blue light in glass ?
Solution :
Data : λ_a = 4500 Å = 4.5 × 10^-7 m, n_g = 1.55, v_a = 3 × 10⁸ m/s
v_a = v_aλ_a

This is the wavelength of blue light in glass.

Question 18.
White light consists of wavelengths from 400 nm to 700 nm. What will be the wavelength range seen when white light is passed through glass of refractive index 1.55 ?
Solution:
Let λ₁ and λ₂ be the wavelengths of light in water for 400 nm and 700 nm (wavelengths in vacuum) respectively. Let λ_a be the wavelength of light in vacuum.

The wavelength range seen when white light is passed through the glass would be 258.06 nm to 451.61 nm.

Question 19.
Determine the change in wavelength of electro-magnetic radiation as it passes from air to glass, if the refractive index of glass with respect to air for the radiation under consideration is 1.5 and the frequency of the radiation is 3.5 × 10¹⁴ Hz. [Speed of the radiation in air (c) = 3 × 10⁸ m/s]
Solution:
Let v be the frequency of the electromagnetic radiation and c its speed in air. Let λ_a and λ_b be the wavelengths of the radiation in air and glass, respectively.

Question 20.
Determine the change in wavelength of light during its passage from air to glass, if the refractive index of glass with respect to air is 1.5 and the frequency of light is 5 × 10¹⁴ Hz. [Speed of light in air = (c) = 3 × 10⁸ m/s] Solution:
Let v be the frequency of the electromagnetic radiation and c its speed in air. Let λ_a and λ_b be the wavelengths of the radiation in air and glass, respectively.

λ_a = 6000 Å, λ_g = 4000 Å ∴ λ_a – λ_g = 2000 Å

Question 21.
A light beam of wavelength 6400 Å is incident normally on the surface of a glass slab of thickness 5 cm. Its wavelength in glass is 4000 A. The beam of light takes the same time to travel from the source to the surface as it takes to travel through the glass slab. Calculate the distance of the source from the surface.
Solution :
Data : λ_a = 6400 Å, s_g = thickness of the glass slab = 5 cm, λ_g = 4000 Å
The speeds of light in glass and air are, respectively, v_g = λ_gv and v_a = λ_av
where the frequency of the light v remains un-changed with the change of medium.
The time taken to travel through the glass slab,
t_g = \(\frac{\mathrm{Sg}}{v_{\mathrm{g}}}\) = \(\frac{S_{g}}{\lambda_{g} v}\)
The time taken to travel through air,
t_a = \(\frac{S_{a}}{v_{a}}\) = \(\frac{d}{\lambda_{\mathrm{a}} v}\)
where s_a = d is the distance of the glass surface from the source.
Since t_a = t_g
\(\frac{d}{\lambda_{\mathrm{a}} v}\) = \(\frac{s_{\mathrm{g}}}{\lambda_{\mathrm{g}} v}\)
∴ d = \(\frac{\lambda_{\mathrm{a}}}{\lambda_{\mathrm{g}}} \mathrm{s}_{\mathrm{g}}\)S_g = \(\frac{6400}{4000}\) × 5 = 1.6 × 5 = 8 cm

Question 22.
A parallel beam of monochromatic light is incident on a glass slab at an angle of incidence 60°. Find the ratio of the width of the beam in glass to that in air if the refractive index of glass is \(\frac{3}{2}\).
Data: i = 60, _an_g = 1.5
By Snell’s law, _an_g = \(\frac{\sin i}{\sin r}\) ∴ sin r = \(\frac{\sin i}{\mathrm{a} n_{\mathrm{g}}}\)
Solution:

Question 23.
The width of a plane incident wavefront is found to be doubled in a denser medium if it makes an angle of 70° with the surface. Calculate the refractive index for the denser medium.
Solution :

Data : i = 70°, \(\frac{\mathrm{CD}}{\mathrm{AB}}\) = 2
\(\frac{\cos r}{\cos i}\) = \(\frac{\mathrm{CD}}{\mathrm{AB}}\) = 2
∴ cos r = 2 cos 70° = 2 × 0.3420 = 0.6840
∴ r = cos^-10.6840 = 46°51′
The refractive index of the denser medium relative to the rarer medium
= \(\frac{\sin i}{\sin r}\) = \(\frac{\sin 70^{\circ}}{\sin 46^{\circ} 51^{\prime}}\) = \(\frac{0.9397}{0.7296}\) = 1.288

Question 24.
A ray of light travelling through air falls on the surface of a glass slab at an angle i. It is found that the angle between the reflected and the refracted rays is 90°. If the speed of light in glass is 2 × 10⁸ m/s, find the angle of incidence.
Solution :
Data : c = 3 × 10⁸ m/s, v_g = 2 × 10⁸ m/s, angle between the reflected ray and the refracted ray = 90°
n_g = \(\frac{c}{v_{g}}\) = \(\frac{3 \times 10^{8}}{2 \times 10^{8}}\) = 1.5
The angle between the reflected and refracted rays = (90° – i) + (90° – r) = 180° – (i + r) = 90° (by the data)
i + r = 90° ∴ r = 90° – i
∴ sin r = sin(90° – i) = cos i
n_g = \(\frac{\sin i}{\sin r}\) = \(\frac{\sin i}{\cos i}\) = tan i
∴ The angle of incidence,
i = tan^-1 ng = tan^-1 1.5 = 56°19′

Question 28.
What is meant by polarized light ? How does it differ from unpolarized light?
Answer:
According to the electromagnetic theory of light, a light wave consists of electric and magnetic fields vibrating at right angles to each other and to the direction of propagation of the wave. If the vibrations of the electric field \(\vec{E}\) in a light wave are confined to a single plane containing the direction of propagation of the wave so that its electric field is restricted along one particular direction at right angles to the direction of propagation of the wave, the wave is said to be plane-polarized or linearly polarized.

If the vibrations of \(\vec{E}\) in a light wave are in all directions perpendicular to the direction of propagation of the light wave, the light wave is said to be unpolarized. Ordinary light, e.g. that emitted by a bulb, is unpolarized.

According to Biot, unpolarized light may be considered as a superposition of many linearly polarized waves, with random orientations. Also, these component waves are noncoherent, that is, irregular in their phase relationships.

[Note : Ordinary light consists of wave trains, each coming from a separate atom in the source. A beam of ordinary light in a single direction consists of millions of such wave trains from the very large number of atoms in the source radiating in that direction. Hence, the vibrations of \(\vec{E}\) are in all transverse directions with equal probability. Thus, light from an ordinary source is.un-polarized.]

Question 29.
How are polarized light and unpolarized light represented in a ray diagram ?
OR
How will you distinguish between polarized and unpolarized light in a ray diagram?
Answer:
Linearly polarized light is represented in a ray diagram by double-headed arrows or short lines drawn perpendicular to the direction of propagation of light, as shown in below figure.

An unpolarized light beam is represented by both dots and arrows, as shown in above figure. The dots are the ‘end views’ of arrows that are oriented normal to the plane of the diagram.

[Note : The length of an arrow or line represents the amplitude of the electric field (\(\vec{E}\)) in the plane of the diagram and the direction indicates the polarization axis of the beam, i.e., the direction of vibration of \(\vec{E}\). According to Jean Biot (1774-1862), French physicist, unpolarized light may be considered as a superposition of many linearly polarized waves, with random orientations in planes perpendicular to the direction of propagation. In an analytical treatment, the electric vectors may be resolved along two mutually perpendicular directions so that the multiplicity of vectors in can be replaced by the two mutually perpendicular vectors as in, both perpendicular to the direction of

propagation of light. Sir David Brewster (1781-1868), British physicist, conceived the ordinary unpolarized light to consist of two perpendicular, polarized components of equal intensity.]

Question 30.
What is a polarizer?
Answer:
When a beam of unpolarized light is passed through certain types of materials (or devices), these materials (or devices) allow only those light waves to pass through which have their electric field along a particular direction. All the other waves with the electric field in other directions are blocked. A material (or a device) which exhibits this special property is called a polarizer.

Question 31.
Explain the terms :

1. polarizing axis of a polarizer
2. plane of vibration
3. plane of polarization.

Answer:

1. Polarizing axis of a polarizer : When unpolarized light is incident on a polarizer, the particular direction along which the electric field of the emergent wave is oriented is called the polarizing axis of the polarizer.
2. Plane of vibration : The plane of vibration of an electromagnetic wave is the plane of vibration of the electric field vector containing the direction of propagation of the wave. Experiment shows that it is the electric field vector E which produces the optical polarization effects.
3. Plane of polarization : The plane of polarization of an electromagnetic wave is defined as the plane perpendicular to the plane of vibration. It is the plane containing the magnetic field vector and the direction of propagation of the wave.
   

Note :
For vision, photography, action of light on electrons and many other observed effects of light, it is the electric vector that is more important than the magnetic one. Hence, nowadays the plane of polarization is taken to be the plane of vibration. Most authoritative; modern books on Optics do not, therefore, explicitly define the plane of vibration. The above convention is obsolete and redundant.

Question 32.
What is a Polaroid? Explain its construction.
Answer:
A Polaroid is a synthetic dichroic sheet polarizer packed with tiny dichroic crystals oriented parallel to each other such that the transmitted light is plane polarized.

Construction : The first large polarizing sheet filter was made by US inventor Edwin H. Land (1909-91). He used the microscopic needlelike crystals of iodoquinine sulphate (known as herapathite) made into a thick colloidal dispersion in nitrocellulose. This material was squeezed through a long narrow slit which forced the needles to orient parallel to one another. The material was then dried to form a solid plastic sheet.

[Note : The modern version of Polaroid is made from long-chain polymer, polyvinyl alcohol. The transmission axis of a Polaroid is the plane of vibration of a plane polarized light which passes through with minimum absorption.]

If the vibrations of the electric field \(\vec{E}\) in a light wave are confined to a single plane containing the direction of propagation of the wave so that its electric field is restricted along one particular direction at right angles to the direction of propagation of the wave, the wave is said to be plane-polarized or linearly polarized.

This phenomenon of restricting the vibrations of light, i.e., of the electric field vector in a particular direction, which is perpendicular to the direction of the propagation of the wave is called polarization of light.

Consider an unpolarized light wave travelling along the x-direction. Let c, v and λ be the speed, frequency and wavelength, respectively, of the wave. The magnitude of its electric field (\(\vec{E}\)) is,
E = E₀ sin (kx – ωt), where E₀ = E_max = amplitude of the wave, ω = 2πv = angular frequency of the wave and k = \(\frac{2 \pi}{\lambda}\) = magnitude of the wave vector or propagation vector.
The intensity of the wave is proportional to |E₀|². The direction of the electric field can be anywhere in the y-z plane. This wave is passed through two identical polarizers as shown.

When a wave with its electric field inclined at an angle φ to the axis of the first polarizer is passed through the polarizer, the component E₀ cos φ will pass through it. The other component E₀ sin φ which is perpendicular to it will be blocked.

Now, after passing through this polarizer, the intensity of this wave will be proportional to the square of its amplitude, i.e., proportional to |E₀ cos φ|².

The intensity of the plane-polarized wave emerging from the first polarizer can be obtained by averaging E₀ cos φ|² over all values of φ between 0 and 180°. The intensity of the wave will be
proportional to \(\frac{1}{2}\) |E₀|² as the average value of cos² φ over this range is \(\frac{1}{2}\). Thus the intensity of an unpolarized wave reduces by half after passing through a polarizer.

When the plane-polarized wave emerges from the first polarizer, let us assume that its electric field (\(\overrightarrow{E_{1}}\)) is along the y-direction. Thus, this electric field is,
\(\overrightarrow{E_{1}}\) = \(\hat{i}\)E₁₀ sin (kx – ωt) … (1)
where, E₁₀ is the amplitude of this polarized wave. The intensity of the polarized wave,
I₁ ∝|E₁₀|² …(2)

Now this wave passes through the second polarizer whose polarization axis (transmission axis) makes an angle θ with the y-direction. This allows only the component E₁₀ cos θ to pass through it. Thus, the amplitude of the wave which passes through the second polarizer is E₂₀ = E₁₀ cos θ and its intensity,
I₂ ∝|E₂₀|²
∴ I₂ ∝ | E₁₀|² cos² θ
∴ I₂ = I₁ cos² θ … (3)

Thus, when plane-polarized light of intensity I₁ is incident on the second identical polarizer, the intensity of light transmitted by the second polarizer varies as cos² θ, i.e., I₂ = I₁ cos² θ, where θ is the angle between the transmission axes of the two polarizers. This is known as Malus’ law.

[Note : Etienne Louis Malus (1775-1812), French military engineer and physicist, discovered in 1809 that light can be polarized by reflection. He was the first to use the word polarization, but his arguments were based on Newton’s corpuscular theory.]

Question 33.
Unpolarized light is passed through two polarizers. Under what condition is the intensity of the emergent light
(i) maximum
(ii) zero?
Answer:
If the angle θ between the axes of polarization of the two polarizers, is 0°, i.e., the polarization axes of the two polarizers are parallel, the intensity of emergent light is maximum. If θ = 90°, i.e., the polarization axes of the two polarizers are perpendicular, no light emerges from the second polarizer, thus the intensity of emergent light is minimum.
[Note: θ = 0° and 90° are known as parallel and cross settings of the two polarizers.]

Question 34.
With a neat labelled diagram, explain the use of a pair of polarizers to vary the intensity of light. What are crossed polarizers?
Answer:
Consider an unpolarized light beam incident perpendicularly on the first polarizing sheet, called the polarizer, whose transmission axis is vertical, say. The light emerging from this sheet is polarized vertically, and the transmitted electric field is \(\overrightarrow{E_{0}}\).

The polarized light beam then passes through a second polarizing sheet, called the analyser, which is placed parallel to the polarizer with its transmission axis at an angle θ to the transmission axis of the polarizer. The component of \(\overrightarrow{E_{0}}\) which is perpendicular to the axis of the analyser is completely absorbed, and the component parallel to that axis is E₀ cos θ.

Since intensity varies as the square of the amplitude, the transmitted intensity varies as I = I₀ cos² θ

where I₀ is the incident light intensity on the analyser. This expression (known as Malus’s law) shows that the transmitted intensity is maximum when the transmission axes are parallel and zero when the transmission axes are perpendicular to each other.

Crossed polarizers are a pair of polarizers with their transmission axes perpendicular to each other so that the transmitted light intensity is zero.

Question 35.
If the angle made by the axis of polarization of the second polarizer to that of the first polarizer is 60°, what can you say about the intensity of the light transmitted by the second polarizer?
Answer:
If I₁ is the intensity of the light incident on the second polarizer and I₂ is the intensity of the light transmitted by the second polarizer,
I₂ = I₁ cos² θ = I₁ cos² 60°
= I₁\(\left(\frac{1}{2}\right)^{2}\)
= \(\frac{\boldsymbol{I}_{1}}{4}\)

Question 36.
If 75% of incident light is transmitted by the second polarizer, what is the angle made by the transmission axis of the second polarizer to the transmission axis of the first polarizer?
Answer:

Question 37.
Explain the phenomenon of polarization of light by reflection.
Answer:
Consider a ray of unpolarized monochromatic light incident at an angle θ_B on a boundary between two transparent media as shown. Medium 1 is a rarer medium with refractive index n₁ and medium 2 is a denser medium with refractive index n₂. Part of incident light gets refracted and the rest

gets reflected. The degree of polarization of the reflected ray varies with the angle of incidence.

The electric field of the incident wave is in the plane perpendicular to the direction of propagation of incident light. This electric field can be resolved into a component parallel to the plane of the paper, shown by double arrows, and a component perpendicular to the plane of the paper shown by dots, both having equal magnitude. Generally, the reflected and refracted rays are partially polarized, i.e., the two components do not have equal magnitude.

In 1812, Sir David Brewster discovered that for a particular angle of incidence. θ_B, the reflected wave is completely plane-polarized with its electric field perpendicular to the plane of the paper while the refracted wave is partially polarized. This particular angle of incidence (θ_B) is called the Brewster angle.

For this angle of incidence, the refracted and reflected rays are perpendicular to each other. For angle of refraction θ_r,
θ_B + θ_r = 90° …… (1)
From Snell’s law of refraction,
∴ n₁ sin θ_B = n₂ sin θ_r … (2)
From Eqs. (1) and (2), we have,

This is called Brewster’s law.

Question 38.
Define polarizing angle. At the polarizing angle, what is the plane of polarization of the reflected ray ?
Answer:
Polarizing angle : The polarizing angle for an interface is the angle of incidence for a ray of unpolarized light at which the reflected ray is completely polarized.

At the polarizing angle, the reflected ray is completely plane polarized in the plane of incidence.

Question 39.
Give one example in which polarization by reflection is used.
Answer:
Polarization by reflection is used to cut out glare from nonmetallic surfaces. Special sunglasses are used for this purpose. Sunglasses fitted with Polaroids reduce the intensity of partially or completely polarized / reflected light incident on the eyes from reflecting surfaces.

Question 40.
State any four uses of a Polaroid.
Answer:

1. A Polaroid lens filter makes use of polarization by reflection. This filter is used in photography to reduce or eliminate glare from reflective nonmetallic surfaces like glass, rock faces, water and foliage. It can also deepen the colour of the skies.
2. Polaroid sunglasses reduce the transmitted intensity by a factor of one half and also reduce or eliminate glare from nonmetallic surfaces such as asphalt roadways and snow fields.
3. Polaroid filters are used in liquid crystal display (LCD) screens.
4. Polaroids are used to produce and show 3-D movies to give the viewer a perception of depth.

[Note :Three-dimensional movies are filmed from two slightly different camera locations and shown at the same time through two projectors fitted with polarizers having different transmission axes. The audience wear glasses which have two Polaroid filters whose transmission axes are the same as those on the projectors.]

Question 41.
Explain the phenomenon of polarization by scattering.
Answer:
When a beam of sunlight strikes air molecules or dust particles whose size is of the order of wavelength of light, the beam gets scattered. The scattered light observed in a direction perpendicular to the direction of incidence, is plane polarized. This phenomenon is called polarization by scattering.

As shown in above figure, a beam of an un-polarized light is incident along the Z-axis on a molecule. Light waves being transverse in nature, all the possible directions of vibration of electric field vector in the unpolarized light are confined to the XY plane. The light incident on the molecule is scattered by the electromagnetic field of the molecule.

When observed along the X-axis, only the vibrations of electric field vector which are parallel to Y-axis can be seen. In the similar manner, when observed along the Y-axis, only the vibrations of electric field vector which are parallel to the X-axis can be seen. Thus, the light scattered in a direction perpendicular to the incident light is plane polarized.

Question 42.
Solve the following.

Question 1.
The angle between the transmission axes of two polarizers is 45°. What will be the ratio of the intensities of the original light and the transmitted light after passing through the second polarizer?
Solution :
Data : θ = 45°
According to Malus’ law,
I₂ = I₂ cos² θ

= 2
This is the required ratio.

Question 2.
Two polarizers are so oriented that the maximum amount of light is transmitted. To what fraction of its maximum value is the intensity of the transmitted light reduced when the second polarizer is rotated through
(a) 300
(b) 600?
Solution:
Data : θ = 30°, 60°,
I₂ = I₂ cos²θ
where I₁ is the intensity of the incident light and I₂ is that of the transmitted light.
(i) For θ = 30°

I₂ = 0.75 I₁
= 75% of I₁

(ii) For θ = 60°
I₂ = I₁ (cos 60°)²
= I₁\(\left(\frac{1}{2}\right)^{2}\) = \(\frac{1}{4}\)I₁
= 0.25 I₁ = 25% of I₁

Question 3.
For a glass plate as a polarizer with refractive index 1.633, calculate the angle of incidence at which reflected light is completely polarized.
Solution :
Data : n = 1.633
tan θ_B = n = 1.633
∴ The polarizing angle,
θ_B = tan^-1 1.633 = 58°31′

Question 4.
Find the refractive index of glass if the angle of incidence at which the light reflected from the surface of the glass is completely polarized is 58°.
Solution :
Data : θ_B = 58°
n_g = tan θ_B = tan 58° .
= 1.6003
This is the refractive index of the glass.

Question 5.
The critical angle for a glass-air interface is sin^-1\(\frac{5}{8}\). A ray of unpolarized monochromatic light in air is incident on the glass. What is the polarizing angle?
Solution :
Data : θ_c = sin^-1 \(\frac{5}{8}\)
∴ sin θ_c = \(\frac{5}{8}\)
∴ n = \(\frac{1}{\sin \theta_{\mathrm{c}}}\) = \(\frac{8}{5}\) = 1.6
Also, n = tan θ_B
∴ θ_B = tan^-1 n
∴ The polarizing angle, θ_B = tan^-1 1.6 = 58°

Question 6.
The refractive index of a medium is \(\sqrt{3}\). What is the angle of refraction, if the unpolarized light is incident on it at the polarizing angle of the medium?
Solution :

This is the angle of refraction.

Question 7.
For a given medium, the polarizing angle is 60°. What is the critical angle for this medium ?
Solution :

This is the critical angle for the medium.

Question 8.
Unpolarized light is incident on a plane glass surface. What should be the angle of incidence so that the reflected and refracted rays are perpendicular to each other? [Given n = 1.5]
Solution :
Data : Refractive index, n = 1.5
The reflected and refracted rays will be perpendicular to each other, when the angle of incidence = the polarizing angle θ_B,
tan θ_B = n = 1.5
∴ θ_B = tan^-1 (1.5) = 56°19′

Question 9.
If a glass plate of refractive index 1.732 is to be used as a polarizer, what would be the
(i) polarizing angle and
(ii) angle of refraction?
Solution :
Data : n_g = 1.732
n_g = tan θ_B
0B = tan^-1 (1.732) = 60°
This is the polarizing angle,

∴ θ_r = sin^-1\(\left(\frac{0.8660}{1.732}\right)\)
= sin^-1 (0.5) = 30°
This is the angle of refraction.

Question 10.
For a certain unpolarized monochromatic light incident on glass and water, the polarizing angles are 59°32′ and 53°4′, respectively. What would be the polarizing angle for the light if it is incident from water on to the glass?
Solution:

Question 11.
The wavelengths of a certain blue light in air and in water are 4800 Å and 3600 Å, respectively. Find the corresponding Brewster angle.
Solution:

This is the Brewster angle for the given light incident on water surface.

Question 12.
A ray of light is incident on a glass slab at the polarizing angle of 58°. Calculate the change in the wavelength of light in glass.
Solution :


∴ The change in the wavelength of the light in glass, λ_a – λ_b = 0.37514λ_a, i.e., 37.51% of its wavelength in air.

Question 13.
For what angle of incidence will light incident on a bucket filled with liquid having refractive index 1.3 be completely polarized after reflection ?
Solution:
The reflected light will be completely polarized when the angle of incidence is equal to the Brewster’s angle which is given by θ_B = tan^-1 \(\frac{n_{2}}{n_{1}}\), where n₁ and n₂ are refractive indices of the first and the second medium respectively. In this case, n₁ = 1 and n₂ = 1.3.
Thus, the required angle of incidence = Brewster’s angle = tan^-1\(\frac{1.3}{1}\) = 52.26°

Question 43.
State and explain the principle of superposition of waves.
Answer:
Principle of superposition of waves : The dis-placement at a point due to the combined effect of a number of waves arriving simultaneously at the point is the vector sum of the displacements due to the individual waves arriving at the point.

Explanation : This is a general principle of linear systems applied to wave phenomena. When two or more wave trains arrive at a point simultaneously, they interpenetrate without disturbing each other. The resultant displacement at that point is the vector sum of the displacements due to the individual waves arriving at the point. The amplitude and phase angle of the resulting disturbance are functions of the individual amplitudes and phases.

Notes :

1. In the case of mechanical waves, e.g., sound, the displacement is that of a vibrating particle of the medium.
2. When we consider superposition of electromagnetic waves, light in this chapter, the term displacement refers to the electric field component of the electromagnetic wave.

Question 44.
Explain what you understand by interference of light.
Answer:
The phenomenon in which the superposition of two or more light waves produces a resultant disturbance of redistributed light intensity or energy is called the interference of light.

Light waves are transverse in nature. If two monochromatic light waves of the same frequency arrive in phase at a point, the crest of one wave coincides with the crest of the other and the trough of one wave coincides with the trough of the other. Therefore, the resultant amplitude and hence the resultant intensity of light at that point is maximum and the point is bright. This phenomenon is called constructive interference. If two light waves having the same amplitude are in opposite phase, the crest of one wave coincides with the trough of the other. Therefore, the resultant amplitude, and hence the intensity, at that point is minimum (zero) and the point is dark. This phenomenon is called destructive interference. If the amplitudes are unequal, the resultant amplitude is minimum, but not zero. At other points, the intensity of light lies between the maximum and zero.

Question 45.
Explain how the phenomenon of interference can be demonstrated in a ripple tank.
Answer:

1. ‘Two pins, a small distance d apart, are attached to the electrical vibrator or an electrically maintained tuning fork of a ripple tank. The pins are kept vertical with their tips in contact with the surface of water in the ripple tank.
2. When the vibrator is switched on, the two pins vibrate together in phase with the same frequency and the same amplitude. Their tips form the sources S₁ and S₂ of circular waves which spread outward along the water surface.
   
3. The waves from the two sources interfere constructively at points where they meet in phase. Thus, where the crest of a wave from S₁ is superposed on the crest of a wave from S₂ (such as point A), and where the trough of a wave from S₁ is superposed on the trough of a wave from S₂ (such as point B), the water molecules have maximum amplitude of vibration.
4. The waves from the two sources interfere destructively at points where they meet in opposite phase. Thus, where the crest of a wave from S₁ is superposed on the trough of a wave from S₂ (such as point C), and where the trough of a wave from S₁ is superposed on the crest of a wave from S₂ (such as point D), the water molecules have minimum amplitude of vibration.

Question 46.
What are coherent sources? Is it possible to observe interference pattern with light from any two different sources ? Why ?
Answer:
Coherent sources : Two sources of light are said to be coherent if the phase difference between the emitted waves remains constant.

It is not possible to observe interference pattern with light from any two different sources. This is because, no observable interference phenomenon occurs by superposing light from two different sources. This happens due to the fact that different sources emit waves of different frequencies. Even if the two sources emit light of the same frequency, the phase difference between the wave trains from them fluctuates randomly and rapidly, i.e., they are not coherent.

Consequently, the interference pattern will change randomly and rapidly, and steady interference pattern would not be observed.

Question 47.
State the conditions for constructive and destructive interference of light.
Answer:
(1) Constructive interference (brightness) : There is constructive interference at a point and the brightness or intensity is maximum there, if the two waves of light of the same frequency arrive at the point in phase, i.e., with a phase difference of zero or an integral multiple of 2π radians.
A phase difference of 2π radians corresponds to a path difference λ, where λ is the wavelength of light. Since

for constructive interference with maximum intensity of light, phase difference = 0, 2π, 4π, 6π, … rad
= n(2π) rad
or path difference = 0, λ, 2λ, 3λ, …, etc.
= nλ
where n = 0, 1, 2, 3, …, etc.

(2) Destructive interference (darkness) : There is destructive interference at a point and the point is the darkest, i.e., the intensity of light is minimum, if the two waves of light of the same frequency and intensity arrive at the point in opposite phase, i.e., with a phase difference of an odd-integral multiple of π radians. A phase difference 2π radians corresponds to a path difference λ, where λ is the wavelength of light.
∴ For destructive interference with minimum intensity of light, phase difference = π, 3π, 5π, … rad
= (2m – 1)π rad
or path difference = λ/2, 3λ/2, 5λ/2, …, etc.
= (2m – 1)\(\frac{\lambda}{2}\)
where m = 1, 2, 3, …, etc.

Question 48.
In Young’s double-slit experiment using light of wavelength 5000Å, what phase difference corresponds to the 11th dark fringe from the centre of the interference pattern?
Answer:
The required phase difference is (2m – 1)π rad = (2 × 11 – 1)π rad = 21π rad.

Question 49.
In Young’s double-slit experiment, if the path difference at a certain point on the screen is 2.999 λ, what can you say about the intensity of light at that point ?
Answer:
The intensity of light at that point will be close to the maximum intensity and the point will be nearly bright as the path difference = 2.999 λ \(\approx\) 3λ (integral multiple of λ).

Question 50.
In Young’s double-slit experiment, if the path difference at a certain point on the screen is 7.4999 λ, what can you say about the nature of the illumination at that point ?
Answer:
The point will be nearly dark as the path difference = 7.4999 λ \(\approx\) (8 – 0.5) λ, which is of the form
(m – \(\frac{1}{2}\))λ, where m = 1, 2, … …. 8

Question 51.
How is Young’s interference experiment performed using a single source of light?
Answer:
When a narrow slit is placed in front of an intense source of monochromatic light, cylindrical wave-fronts propagate from the slit. In Young’s experiment, two coherent sources are then obtained by wavefront splitting by placing a second screen with two narrow slits at a small distance from the first slit.

Question 52.
State any two points of importance of Young’s experiment to observe the interference of light.
Answer:
Importance of Young’s experiment observe the interference of light:

1. It was the first experiment (1800-04) in which the interference of light was observed.
2. This experiment showed that light is propagated in the form of waves.
3. From this experiment, the wavelength of monochromatic light can be determined.

Question 53.
What is the nature of the interference pattern obtained using white light?
Answer:
With white light, one gets a white central fringe at the point of zero path difference along with a few coloured fringes on both the sides, the colours soon fade off to white.

The central fringe is white because waves of all wavelengths constructively interfere here. For a path difference of \(\frac{1}{2}\)λ_violet, complete destructive interference occurs only for the violet colour; for waves of other wavelengths, there is only partial destructive interference. Consequently, we have a line devoid of violet colour and thus reddish in appearance. A point for which the path difference = \(\frac{1}{2}\)λ_red is similarly devoid of red colour, and appears violettish. Thus, following the white central fringe we have coloured fringes, from reddish to violettish. Beyond this, the fringes disappear because there are so many wavelengths in the visible region which constructively interfere that we observe practically uniform white illumination.

Question 54.
In Young’s double-slit experiment, the second minimum in the interference pattern is exactly in front of one slit. The distance between the two slits is d and that between the source and screen is D. What is the wavelength of the light used ?
Answer:
The distance of the mth minimum from the central fringe is,

This is the wavelength of the light used.

Question 55.
In Young’s double-slit experiment, a glass slide of refractive index n_g and thickness b is placed in front of one of the slits. What happens to the interference pattern and fringe width ? Derive an expression for the positions of the bright fringes in the interference pattern.
Answer:
Suppose, in Young’s double-slit experiment, a glass slide of refractive index n_g and thickness b is placed in front of the slit S₁.

If the experiment is set up in free space, this introduces an additional optical path length (n_g – 1)b in the path S₁P.
Then, the optical path difference to the point P from S₁ and S₂ is,
∆l = S₂P – [S₁P + (n_g – 1)b] = (S₂P – S₁P) – (n_g – 1)b = y\(\frac{d}{D}\) – (n_g – 1)b … (1)
where y = PO’, d is the distance between S₁ and S₂, and D is the distance of the screen from S₁ and S₂. Thus, point P will be bright (maximum intensity) if ∆l = nλ, where n = 0, 1, 2, … . The central bright fringe, corresponding to n = 0, will be obtained at a distance y₀ from O’ such that,
∆l = y_o\(\frac{d}{D}\) – (n_g – 1)b = 0
∴ y_o\(\frac{d}{D}\) = (n_g – 1)b ∴ y₀ = \(\frac{D}{d}\)(ng-l) b …(2)
Therefore, the central bright fringe and the interference pattern will shift up (towards P) by a distance y₀ given by EQ. (2).
The distance of the nth bright fringe from O’ towards P is,

The distance of the (n + l)th bright fringe from O’ towards P is

Therefore, the fringe width,
w = y_n+1 – y_n = \(\frac{\lambda D}{d}\) ….(5)
Thus, the fringe width remains unchanged.

Question 56.
What happens to the interference pattern when the phase difference between the two sources of light changes with time ?
Answer:
If the two sources do not maintain their phase relation during the time required for observation, the intensity of light at any point on the screen and consequently the interference pattern changes rapidly, and hence steady interference pattern is not observed.

Question 57.
Obtain expressions in terms of electric field, for the resultant amplitude and the intensity for the interference pattern produced by monochromatic light waves from two coherent sources.
Answer:
Consider a two-source interference pattern produced by superposition of monochromatic light waves of angular frequency ω, wavelength λ and constant phase difference, φ. At the centre of a bright fringe, there is constructive interference. Here, the amplitude of the resultant wave is double the amplitude of the wave incident on AB. Now, the intensity is proportional to the square of the amplitude of the wave. Hence, the resultant intensity is, I = 4I₀, where I₀ is the intensity of the incident wave. At the centre of a dark fringe, there is destructive interference. Here, the amplitude of the resultant wave and hence the intensity is zero. At other points the intensity is between 4I₀ and zero, depending on the phase difference.

Let the equations of the two waves coming from S₁ and S₂ at some point in the interference pattern be, E₁ = E₂ sin ωt and E₂ = E₀ sin (ωt + φ) respectively, where E₀ is the amplitude of the electric field vector.

By the principle of superposition of waves, the resultant electric field at that point is the algebraic sum, E = E₁ + E₂
∴ E = E₀ sin ωt + E₀ sin (ωt + φ)
= E₀ [sin ωt + sin (ωt + φ)]
= 2E₀ sin (ωt + φ/ 2) cos (φ/2)
The amplitude of the resultant wave is 2 E₀ cos (φ/2).
Therefore, the intensity at that point is I ∝ |2 E₀ cos (φ/2) |²
∴ I = 4I₀ cos² (φ/2)
as I₀ ∝ |E₀|².

Question 58.
Monochromatic light waves of amplitudes E₁₀ and E₂₀ and a constant phase difference φ produce an interference pattern. State an expression for the resultant amplitude at a point in the pattern. Hence, deduce the conditions for
(i) constructive interference with maximum intensity
(ii) destructive interference with minimum intensity. Also show that the ratio of the maximum and minimum intensities is
\(\frac{I_{\max }}{I_{\min }}\) = \(\left(\frac{\boldsymbol{E}_{10}+\boldsymbol{E}_{20}}{\boldsymbol{E}_{10}-\boldsymbol{E}_{20}}\right)^{2}\)
Answer:
Consider a two-source interference pattern produced by monochromatic light waves of angular frequency ω, wavelength λ, amplitudes E₁₀ and E₂₀ and a constant phase difference φ.

Let the individual electric fields along the same line due to the waves at some point in the interference pattern be
E₁ = E₁₀ sin ωt and E₂ = E₂₀ sin (ωt + φ)
By the principle of superposition of waves, the resultant electric field (displacement) at that point is the algebraic sum

Since, the intensity of a wave is proportional to the square of its amplitude, the resultant intensity at P,
I ∝ |R|²

Thus, the intensity depends on cos φ.
The condition for constructive interference with maximum intensity is cos φ is maximum, equal to 1, i.e., φ = 2nπ (n = 0, 1, 2, 3…) … (3)
The condition for destructive interference with minimum intensity is cos φ is minimum equal to

Question 59.
If the amplitude ratio (E₂₀/E₁₀) of two interfering coherent waves producing an interference pattern is 0.5, what is the ratio of the minimum intensity to maximum intensity?
Answer:

Question 60.
If the amplitude ratio (E₂₀/E₁₀) of two interfering coherent waves producing an interference pattern is 0.8, what is the ratio of the maximum intensity to the minimum intensity?
Answer:

Question 61.
Two slits in Young’s experiment have widths in the ratio 2 : 3. What is the ratio of the intensities of light waves coming from them?
Answer:
The required ratio is \(\frac{I_{1}}{I_{2}}\) = \(\frac{w_{1}}{w_{2}}\) = \(\frac{2}{3}\)

Question 62.
Monochromatic light waves of intensities I₁ and I₂, and a constant phase difference φ produce an interference pattern. State an expression for the resultant intensity at a point in the pattern. Hence deduce the expressions for the resultant intensity, maximum intensity and minimum intensity if I₁ = I₂ = I₀.
Answer:
Consider a two-source interference pattern produced by monochromatic light waves of intensities I₁ and I₂, and a constant phase difference φ. The resultant intensity at a point in the pattern is

At a point of constructive interference with maximum intensity, cos φ = 1.
∴ I_max = 2I₀(1 + 1) = 4I₀ …(3)
At point of destructive interference, with minimum intensity, cos φ = – 1.
∴ I_min = 2I₀(1 – 1) = 0… (4)
Notes :

(1) Since 1 + cos φ = 2 cos²φ, EQ. (2) above can be expressed as I = 4I₀ cos²\(\frac{\phi}{2}\) = I_max cos²\(\frac{\phi}{2}\).

(2) The average of cos² \(\frac{\phi}{2}\), averaged over one cycle, is \(\frac{1}{2}\).
Therefore, the average intensity of a bright and dark fringe in an interference pattern is I_av = 4I₀ × \(\frac{1}{2}\) = 2I₀. The graph of l versus φ is shown, in which the dotted line shows I_av.

In the absence of interference phenomenon, the intensity at a point due to two waves each of intensity I₀ is 2I₀, which is the same as the average intensity in an interference pattern. Thus, interference of light is consistent with the law of conservation of energy. Interference produces a redistribution of energy out of regions where it is destructive into the regions where it is constructive.

Question 63.
At a point in an interference pattern, the two interfering coherent waves of equal intensity I₀ have phase difference 60°. What will be the resultant intensity at that point ?
Answer:
Resultant intensity, I₀ = 2I₀ (1 + cos φ)
= 2I₀(1 + cos 60°) = 2I₀(1 + \(\frac{1}{2}\)) = 3I₀.

Question 64.
Solve the following :

Question 1.
Find the ratio of intensities at two points X and Y on a screen in Young’s double-slit experiment where waves from the slits S₁, and S₂ have path difference of 0 and \(\frac{\lambda}{4}\) respectively.
Solution :

Question 2.
Two coherent sources, whose intensity ratio is 81 : 1, produce interference fringes. Calculate the ratio of the intensities of maxima and minima in the fringe system.
Solution :
Data : I₁ : I₂ = 81 : 1
If E₁₀ and E₂₀ are the amplitudes of the interfering waves, the ratio of the maximum intensity to the minimum intensity in the fringe system is

Question 3.
In Young’s double-slit experiment, the ratio of the intensities at the maxima and minima in the interference pattern is 36 : 16. What is the ratio of the widths of the two slits?
Answer:

Question 4.
In Young’s double-slit experiment, the ratio of the intensities of the maxima and minima in an interference pattern is 36 : 9. What is the ratio of the intensities of the two interfering waves?
Answer:

∴ The ratio of the intensities of the two interfering waves is 9 : 1.

Question 5.
Two slits in Young’s double-slit experiment have widths in the ratio 81 : 1. What is the ratio of the amplitudes of light waves coming from them?
Solution:
Data : w₁ : w₂ = 81 : 1
Since the intensity of a wave is directly proportional to the square of its amplitude,
\(\frac{I_{1}}{I_{2}}\) = \(\left(\frac{E_{10}}{E_{20}}\right)\) …. (1)
Also, the intensity of a wave coming out of a slit is directly propotional to the slit width.
∴ \(\frac{I_{1}}{I_{2}}\) = \(\frac{w_{1}}{w_{2}}\) …. (2)
From Eqs. (1) and (2),

The ratio of the amplitudes of light waves from the slits is 9: 1.

Question 6.
Two monochromatic light waves of equal intensities produce an interference pattern. At a point in the pattern, the phase difference between the interfering waves is π/3 rad. Express the intensity at this point as a fraction of the maximum intensity in the pattern.
Solution :
Data : I₁ = I₂ = I₀, φ = π/3 rad
The resultant intensity at the point in the pattern is

Question 7.
In Young’s double-slit experiment using monochromatic light of wavelength λ, the intensity of light at a point on the screen where the path difference is λ is I. What is the intensity of light at a point where the path difference is λ/3 ?
Solution :
Data : ∆l₁ = λ, I₁ = I, ∆l₂ = λ/3
We assume that light waves coming out of the two slits are of equal intensity I₀.
Then, at a point in the interference pattern where the phase difference between the interfering waves is φ, the resultant intensity is,
I = 2I₀ (1 + cos φ)
Phase difference (φ) = \(\frac{2 \pi}{\lambda}\) × path difference (∆l)


∴ A The intensity of light at a point where the path difference is λ/3 is λ/4.

Question 8.
The optical path difference between identical waves from two coherent sources and arriving at a point is 87λ. What can you say about the resultant intensity at the point? If the path difference is 49.19 μm, calculate the wavelength of light.
Solution :
Data : Path difference = 87λ = 49.19 /rm.
(i) Path difference = 87λ = nλ, where n = 87. As the path difference is an integral multiple of the wavelength λ, the point where the waves interfere is a bright point with maximum intensity.

(ii) 87 λ = 49.19 μn = 49.19 × 10^-6 m .
∴ λ = \(\frac{49.19 \times 10^{-6}}{87}\) = 5.654 × 10^-7 m = 5654 Å

Question 9.
The optical path difference between identical waves from two coherent sources and arriving at a point is 172. What can you say about the resultant intensity at the point? If the path difference is 9.18 μm, calculate the wavelength of light.
Solution :
Data : Path difference = 17λ = 9.18 μm
(i) Path difference = 17λ = nλ, where n = 17
As the path difference is an integral multiple of the wavelength λ, the point where the waves interfere is a bright point with maximum intensity.

(ii) 17λ = 9.18 μm = 9.18 × 10^-6 m

Question 10.
At a point on the two-slit interference pattern obtained using a source of green light of wavelength 5500 Å, the path difference is 4.125 pm. Is the point at the centre of a bright or dark fringe ? Hence, find the order of the fringe.
Solution :
Data : Path difference, ∆l = 4.125 × 10^-6 λ = 5500 Å = 5.5 × 10^-7 m

As the path difference is an odd integral multiple of \(\frac{\lambda}{2}\) the point is at the centre of a dark fringe.
∴ p = 2m – 1 (m = 1, 2, 3…)
∴ 2m – 1 = 15 ∴ m = 8
∴ The order of the fringe is 8 (i.e., the point lies at the centre of the 8th dark fringe.)

Question 11.
The two slits in an interference experiment are illuminated by light of wavelength 5600 A. Deter-mine the path difference and phase difference between the waves arriving at the centre of the eighth dark fringe on the screen.
Solution :
Data : λ = 5600 A = 5.6 × 10^-7 m
Order of the dark fringe is 8, ∴ m = 8
For the fringe to be dark, path difference
= (2m – 1)\(\frac{\lambda}{2}\)
∴ Path difference, ∆l = (2 × 8 – 1)\(\frac{\lambda}{2}\) = 7.5λ
= 7.5 × 5.6 × 10^-7 m
= 4.2 × 10^-6 m
Phase difference, φ = \(\frac{2 \pi}{\lambda}\) × ∆l
= \(\frac{2 \pi}{5.6 \times 10^{-7}}\) × 4.2 × 10^-6 = 15π

Question 12.
In Young’s double-slit experiment, interference fringes are observed on a screen 1 m away from the two slits which are 2 mm apart. A point P on the screen is 1.8 mm from the central bright fringe,
(i) Find the path difference at P.
(ii) If the wavelength of the light used in 4800 Å, what can you say about the illumination at P?
Solution :
Data : D = 1 m, d = 2 mm = 2 × 10^-3 m, y = 1.8 mm = 1.8 × 10^-3 m, λ = 4800 Å = 4.8 × 10^-7 m

∴ Path difference = 15\(\frac{\lambda}{2}\)
As the path difference is an odd integral multiple of \(\frac{\lambda}{2}\), point P is a dark point with minimum intensity.

Question 13.
Two slits 1.25 mm apart are illuminated by light of wavelength 4500 Å. The screen is 1 m away from the plane of the slits. Find the separation between the 2nd bright fringe on one side and the 2nd bright fringe on the other side of the central maximum.
Solution :
Data : D = 1 m, d = 1.25 mm = 1.25 × 10^-3 m,
λ = 4500 Å = 4500 × 10^-10 m = 4.5 × 10^-7 m
Since, it is a second bright fringe, n = 2. If s is the distance between the 2nd bright fringe on one side and the 2nd bright fringe on the other side of the central maximum, then
s = 2y = 2nλ\(\frac{D}{d}\)

= 14.4 × 10^-4 m
W = 1.44 mm

Question 14.
A plane wavefront of light of wavelength 5000 Å is incident on two slits in a screen perpendicular to the direction of light rays. If the total separation of 10 bright fringes on a screen 2 m away is 4 mm, find the distance between the slits.
Solution:
Given : λ = 5000 Å = 5 × 10^-7 m,
D = 2 m and the total separation of 10 fringes = 4 mm = 4 × 10^-3 m

Question 15.
In Young’s double-slit experiment with slits of equal width, a point P on the screen is at a distance equal to one-fourth of the fringe width from the central maximum. If the intensity at the central maximum is I_c, find the intensity at P.
Solution :

Since, the slits have equal width, the intensities of the two interfering waves are equal, say I₀. Then the intensity at a point on the screen is
I = 4I₀ cos² \(\frac{\phi}{2}\)
At the central maximum, φ = 0.

The intensity at P is half that at the central maximum.

Question 16.
In a double-slit experiment, the optical path difference between the waves coming from two coherent sources at a point P on one side of the central bright band is 7.5 × 10^-6 m and that at a point Q on the other side of the central bright band is 1.8 × 10^-6 m. How many bright and dark bands are observed between points P and Q if the wavelength of light used is 6 × 10^-7 m ?
Solution :
Data : ∆l₁ = 7.5 × 10^-6 m, ∆l₂ = 1.8 × 10^-6 m λ = 6 × 10^-7 m
For point P : Let p\(\frac{\lambda}{2}\) = ∆l₁

The path difference ∆l₁ is an odd integral multiple of λ/2 : ∆l₁ = (2m – 1)\(\frac{\lambda}{2}\), where m is an integer,
∴ 2m – 1 = 25 ∴ m = 13
∴ Point P is at the centre of the 13th dark band.
For point Q :
Let q\(\frac{\lambda}{2}\) = ∆l₂

The path difference ∆l₂ is an even integral multiple of \(\frac{\lambda}{2}\) : ∆l₂ = (2n)\(\frac{\lambda}{2}\), where n is an integer
∴ 2n = 6 ∴ n = 3
∴ Point Q is at the centre of the 3rd bright band. Between points P and Q, excluding the respective bands at P and Q, the number of dark bands = 12 + 3 = 15 and the number of bright bands (including the central bright band) = 12 + 2 + 1 = 15

Question 17.
In Young’s double-slit experiment, light waves of wavelength 5.2 × 10^-7 m and 6.5 × 10^-7 m are used in turn keeping the same geometry. Compare the fringe widths in the two cases.
Solution :
Data : λ₁ = 5.2 × 10^-7 m, λ₂ = 6.5 × 10^-7 m
As, W = \(\frac{\lambda D}{d}\) and the geometry is the same, i.e., D and d remain the same,

Question 18.
Two coherent sources are 1.8 mm apart and the fringes are observed on a screen 80 cm away from them. It is found that with a certain source of light, the fourth bright fringe is situated at a distance of 1.08 mm from the central fringe. Calculate the wavelength of light.
Solution :
Data : d = 1.8 mm = 1.8 × 10^-3 m
D = 80 cm = 0.8 m
For fourth bright fringe, n = 4

This is the wavelength of light.

Question 19.
Green light of wavelength 5100 Å from a narrow slit is incident on a double-slit. If the overall separation of 10 fringes on a screen 200 cm away from it is 2 cm, find the slit-separation.
Solution :
Data : λ = 5100 Å = 5.1 × 10^-7 m,
W = \(\frac{2}{10}\) cm = 2 × 10^-3 m,
D = 200 cm = 2 m
W = \(\frac{\lambda D}{d}\)
∴ d = \(\frac{\lambda D}{W}\) = \(\frac{5.1 \times 10^{-7} \times 2}{2 \times 10^{-3}}\)
= 5.1 × 10^-4 m
This is the slit-separation.

Question 20.
Sodium light of wavelength 5.896 × 10^-7 m is passed through two pinholes 0.5 mm apart, and an interference pattern is formed on a screen kept parallel to the plane of the pinholes and 1.2 m from them. Find the distance between
(i) the second and the fifth bright fringes
(ii) the third and the seventh dark fringes on the same side of the central bright point.
Solution :
Data : λ = 5.896 × 10^-7 m, d = 0.5 mm= 0.5 × 10^-3 m, D = 1.2 m
(i) The distance of the nth bright fringe from the central bright point is

The distance between the second and the fifth bright fringes on the same side of the central bright point is 4.245 × 10^-3 m.

(ii) The distance of the mth dark fringe from the central bright point is

= 5.66 × 10^-3 m
The distance between the third and the seventh dark fringes on the same side of the central bright point is 5.66 × 10^-3 m.

Question 21.
In Young’s double-slit experiment, the two slits are 2 mm apart. The interference fringes for light of wavelength 6000Å are formed on a screen 80 cm away from them.
(i) How far is the second bright fringe from the central bright point?
(ii) How far is the second dark fringe from the central bright point?
Solution:
Data: D = 80 cm = 0.8 m,
d = 2 mm = 2 × 10^-3 m, λ = 6000Å = 6 × 10^-7 m
(i) For the second bright fringe from the central bright point, n = 2

This is the required distance.

(ii) For the second dark fringe from the central bright point, m = 2

This is the required distance.

Question 21.
In Young’s double-slit experiment, the distance between two consecutive bright fringes on a screen placed at 1.5 m from the two slits is 0.6 mm. What would be the fringe width, if the screen is brought towards the slits by 50 cm, keeping rest of the setting the same?
Solution:
Let λ be the wavelengths of light used and d the distance between the two sources (i.e., slits), if D is the distance between the sources and the screen, the fringe width is
w = \(\frac{\lambda D}{d}\)
For the same λ and d, W ∝ D.
∴ \(\frac{W_{2}}{W_{1}}\) = \(\frac{D_{2}}{D_{1}}\)
Data:
W₁ = 0.6 mm = 6 × 10^-4 m,
D₁ = 1.5 m, D₂ = 1 m

This is the required fringe width.

Question 22.
On passing light of wavelength 5000 Å through two pinholes 2 mm apart, an interference pattern is formed on a screen kept parallel to the plane of the pinholes and 100 cm from them. Find the distance between the fifth bright band on one side of the central bright band and the sixth dark band on the other side.
Solution :
Data : λ = 5000 Å = 5 × 10^-7 m, d = 2 mm = 2 × 10^-3 m, D = 100 cm = 1 m

∴ y_6d = (6 – \(\frac{1}{2}\)) × 2.5 × 10^-4m = 1.375 × 10^-3 m
∴ y_5b + y_6d = 1.25 × 10^-3m + 1.375 × 10^-3m
= 2.625 × 10^-3 m = 2.625 mm
This is the required distance.

Question 23.
Monochromatic light from a narrow slit illuminates two narrow slits 3 mm apart, producing an interference pattern with bright fringes 0.15 mm apart on a screen 75 cm away from the slits. Find the wavelength of the light. How will the fringe width be altered if
(a) the distance of the screen from the slits is doubled
(b) the separation between the slits is doubled ?
Solution :
Data : d = 3 mm = 3 × 10^-3 m,
W = 0.15 mm = 1.5 × 10^-4 m, D = 75 cm = 0.75 m

Question 24.
In Young’s double-slit experiment the slits are 2 mm apart and interference is observed on a screen placed at a distance of 100 cm from the slits. It is found that the ninth bright fringe is at a distance of 2.208 mm from the second dark fringe from the centre of the fringe pattern. Find the wavelength of light used.
Solution :
Data : d = 2 mm = 2 × 10^-3 m,

Question 25.
In Young’s double-slit experiment, the two slits separated by 4 mm are illuminated by light of wavelength 6400 Å. Interference fringes are obtained on a screen placed at a distance of 60 cm from the slits. Find the change in the fringe width if the separation between the slits is
(i) increased by 1 mm
(ii) decreased by 1 mm.
Solution :
Data : d = 4 mm = 4 × 10^-3 m, λ = 6.4 × 10^-7m, D = 0.6 m, d’ = 5 mm = 5 × 10^-3 m, d” = 3 mm = 3 × 10^-3m
Fringe width, W = \(\frac{\lambda D}{d}\) ∴ W ∝ \(\frac{1}{d}\)
(i) Since d’ > d, W’ < W, i.e., the fringe width decreases.
Decrease in the fringe width = W – W

(ii) Since d” < d, W” > W, i.e., the fringe width increases.
Increase in the fringe width = W” – W

Question 26.
In Young’s double-slit experiment, the separation between the slits is 3 mm and the distance between the slits and the screen is 1 m. If the wavelength of light used is 6000 Å, calculate the fringe width. What will be the change in the fringe width if the entire apparatus is immersed in a liquid of refractive index \(\frac{4}{3}\)?
Solution :
Data : d = 3 × 10^-3 m, D = 1 m, λ = 6 × 10^-7 m, n = \(\frac{4}{3}\)

Question 27.
In Young’s double-slit experiment using monochromatic light, the fringe pattern shifts by certain distance on the screen when a mica sheet of refractive index 1.6 and thickness 1.964 microns is introduced in the path of one of the interfering waves. The mica sheet is then removed and the slits-to-screen distance is doubled. It is found that the distance between successive maxima now is the same as the observed fringe shift with the mica sheet. Calculate the wavelength of the monochromatic light used.
Solution :
Data : n_m = 1.6, b = 1.964 microns
= 1.964 × 10^-6 m,
D₂ = 2D₁, W₂ = y₀
The fringe shift with the mica sheet,
y₀ = \(\frac{D_{1}}{d}\)(n_m – 1)b
Subsequent to the removal of the mica sheet and doubling the slits-to-screen distance, the new fringe width is,

Question 28.
What must be the thickness of a thin film which, when kept near one of the slits shifts the central fringe by 5 mm for incident light of wavelength 5890 Å in Young’s double-slit interference experiment ? The refractive index of the material of the film is 1.1 and the distance between the slits is 0.5 mm.
Solution :
Data : λ = 5890 Å, nm = 1.1, the shift of the central bright fringe = 5 mm

Let t be the thickness of the film and P the point on the screen where the central fringe has shifted. Suppose the film is kept in front of slit S1. Due to the film, the optical path travelled by the light passing through it increases by (1.1 – 1)f = 0.1t. Thus, the optical paths between the two beams passing through the two slits are not equal at the midpoint of the screen but are equal at P, 5 mm away from the centre. At this point the distance travelled by light from the other slit S₂ to the screen is larger than that travelled by light from S₁ by 0.1t.

The difference in distances, S₂P – S₁P = yλ/d, where y is the distance along the screen = 5 mm = 5 × 10^-3m and d = 0.5 mm = 5 × 10^-4 m.

This has to be equal to the difference in optical paths introduced by the film.
Thus, 0.1t = 5 × 10^-3 × 5890 × 10^-10/5 × 10^-4.
∴ t = 5890 × 10^-8m = 5.89 × 10^-5 m = 0.0589 mm

Question 29.
In a biprism experiment, the eyepiece is placed at a distance of 1.2 metres from the source. The distance between the virtual sources was found to be 7.5 × 10^-4 m. Find the wavelength of light if the eyepiece is to be moved transversely through a distance of 1.888 cm for 20 fringes.
Solution :
Data : D = 1.2 m, d = 7.5 × 10^-4 m,
20 W = 1.888 cm = 1.888 × 10^-2 m

Question 30.
A biprism is placed 5 cm from the slit illuminated by sodium light of wavelength 5890 Å. The width of the fringes obtained on a screen 75 cm from the biprism is 9.424 × 10^-2 cm. What is the distance between the two coherent sources?
Solution :
Data : D = 5 cm + 75 cm = 80 cm = 0.8 m,
λ = 5890 Å = 5.890 × 10^-7 m,
W = 9.424 × 10^-2 cm = 9.424 × 10^-4 m
Fringe width, W = \(\frac{\lambda D}{d}\)
∴ The distance between the two coherent sources,

= 5 × 10^-4 m = 0.5 mm

Question 31.
In a biprism experiment, the distance of the 20th bright band from the centre of the interference pattern is 8 mm. Calculate the distance of the 30th bright band from the centre.
Solution :
Data : y₂₀ = 8 mm (bright band)
The distance of the nth bright band from the centre of the interference pattern,

The distance of the 30th bright band from the centre of the interference pattern is 12 mm.

Question 32.
In a biprism experiment, a source of light having wavelength 6500 Å is replaced by a source of light having wavelength 5500 Å. Calculate the change in the fringe width, if the screen is at a distance of 1 m from the sources which are 1 mm apart.
Solution :

The fringe width decreases by 1 × 10^-4 m = 0.1 mm.

Question 33.
In a biprism experiment, light of wavelength 5200 Å is used to get an interference pattern on the screen. The fringe width changes by 1.3 mm when the screen is moved towards the biprism by 50 cm. Find the distance between the two virtual images of the slit.
Solution :
Data : λ = 5200 Å = 5.2 × 10^-7 m,
W₁ – W₂ = 1.3 mm = 1.3 × 10^-3 m,
D₁ – D₂ = 50 cm = 0.5 m

This is the distance between the two virtual sources.

Question 34.
In a biprism experiment, the 10th dark band is observed at 2.09 mm from the central bright point on the screen with red light of wavelength 6400 Å. By how much will the fringe width change if blue light of wavelength 4800 A is used with the same setting?
Solution :

Question 35.
In a biprism experiment, the slit is illuminated by red light of wavelength 6400 Å, and the cross wire of the eyepiece is adjusted at the centre of the 3rd bright band. On using blue light, it is found that the 4th bright band is on the cross wire. Find the wavelength of blue light.
Solution :
Data : λ_r = 6400 Å, y₃ (red, bright)

This is the wavelength of blue light.

Question 36.
In a biprism experiment, the wavelength of red light used is 6000 Å and the nth bright band is obtained at a point P on the screen. Keeping the same setting, the source is replaced by a source of green light of wavelength 5000 Å and the (n + 1)th bright band of green light coincides with point P. Find n.
Solution :

Question 37.
In a biprism experiment, the slit is illuminated by light of wavelength 4800 Å. The distance between the slit and the biprism is 15 cm and that between the biprism and the eyepiece is 85 cm. If the distance between the virtual sources is 3 mm, determine the distance between the 4th bright band on one side and the 4th dark band on the other side of the central band.
Solution :
Data : λ = 4800 Å = 4.8 × 10^-7 m,
d = 3 mm = 3 × 10^-3 m,
D = distance between the slit and the biprism + distance between the biprism and the eyepiece = 15 + 85 = 100 cm = 1 m The distance of the nth bright band from the central band is

This is the required distance.

Question 38.
An isosceles prism of refracting angle 179° and refractive index 1.6 is used as a biprism by keeping it 10 cm away from a slit, the edge of the biprism being parallel to the slit. The slit is illuminated by a light of wavelength 600 nm and the screen is 90 cm away from the biprism. Calculate the location of the centre of the 10th dark band from the centre of the interference pattern and the path difference at this location.
Solution :

Question 39.
In a biprism experiment, the distance between the second and tenth dark bands on the same side of the central bright band is 0.12 cm, that between the slit and the biprism is 20 cm and that between the biprism and the eyepiece is 80 cm. If the slit images given by the lens in the two positions are 4.5 mm and 2 mm apart, find the wavelength of light used.
Solution :
The distance between the second and tenth dark bands on the same side of the central band is equal to 8 times the fringe width (W).
∴ 8 W = 0.12 cm (by the data)
∴ W = \(\frac{0.12}{8}\) cm = 0.015 cm = 0.015 × 10^-2 m
The distance (D) between the slit and the eyepiece is equal to the sum of the distance between the slit and the biprism and the distance between the biprism and the eyepiece.
∴ D = 20 + 80 = 100 cm = 1 m (by the data)
Also, d₁ = 4.5 mm and d₂ = 2 mm
∴ The distance (d) between the virtual images of the slit is
d = \(\sqrt{d_{1} d_{2}}\) = \(\) mm = 3 mm
= 3 × 10^-3 m
∴ The wavelength of light,

Question 40.
In a biprism experiment, the slit and the eyepiece. are 10 cm and 80 cm away from the biprism. When a convex lens was interposed at 30 cm from the slit, the separation of the two magnified images of the slit was found to be 4.5 mm. If the wavelength of the source is 4500 Å, calculate the fringe width.
Solution:
Data : d₁ = 4.5 mm = 4.5 × 10^-3 m,
λ = 4500 Å = 4.5 × 10^-7 m,
distance between the slit and the eyepiece (D) = distance between the slit and the biprism + distance between the biprism and the eyepiece = 10 cm + 80 cm = 90 cm = 0.9 m, u₁ = 30 cm = 0.3 m v₁ = D – u₁ = 0.9m – 0.3m = 0.6 m
Linear magnification of a lens,

Question 65.
Describe with a neat labelled ray diagram the Fraunhofer diffraction pattern due to a single slit. Obtain the expressions for the positions of the intensity minima and maxima. Also obtain the expression for the width of the central maximum.
Answer:
When a parallel beam of monochromatic light of wavelength λ illuminates a single slit of finite width a, we observe on a screen some distance from the slit, a broad pattern of alternate dark and bright fringes. The pattern consists of a central bright fringe, with successive dark and bright fringes of diminishing intensity on both sides. This is called ‘ the diffraction pattern of a single slit.

Consider a single slit illuminated with a parallel beam of monochromatic light perpendicular to the plane of the slit. The diffraction pattern is obtained on a screen at a distance D (» a) from the slit and at the focal plane of the convex lens.

We can imagine the single slit as being made up of a large number of Huygens’ sources evenly distributed over the width of the slit. Then the maxima and minima of the pattern arise from the interference of the various Huygens’ wavelets.
Now, imagine the single slit as made up of two adjacent slits, each of width a/2. Since, the incident plane wavefronts are parallel to the plane of the slit, all the Huygens sources at the slit will be in phase. They will therefore also in phase at the point P₀ on the screen, where P₀ is equidistant from all the Huygens sources. At P₀, then, we get the central maximum.

For the first minimum of intensity on the screen, the path difference between the waves from the Huygens sources A and O (or O and B) is λ/2, which is the condition for destructive interference. Suppose, the nodal line OP for the first minimum subtends an angle θ at the slit; θ is very small. With P as the centre and PA as radius, strike an arc intersecting PB at C. Since, D » a, the arc AC can be considered a straight line at right angles to PB. Then, ∆ ABC is a right-angled triangle similar to A OP₀P.
This means that, ∠BAC = θ
∴ BC = a sin θ
∴ Difference in path length,
BC = PB – PA = (PB – PO) + (PO – PA)

(∵ θ is very small and in radian)
The other nodal lines of intensity minima can be understood in a similar way. In general, then, for the with minimum (wi = +1, ±2, ±3, …).
θ_m = \(\frac{m \lambda}{a}\) (with minimum) … (2)
as θ_m is very small and in radian.
Between the successive minima, the intensity rises to secondary maxima when the path difference is an odd-integral multiple of \(\frac{\lambda}{2}\) :

(mth secondary maximum) … (3)
Width of the central maximum :
Equation (1) gives the angular half width of the central maximum. Therefore, the angular width of the central maximum is,
2θ = \(\frac{2 \lambda}{a}\) … (4)
From ∆OP₀P, P₀P = D tan θ \(\simeq\) D sin θ
(∵ θ is very small and in radian)
∴ y₁ = P₀P = \(\frac{D \lambda}{a}\) [from Eq. (1)] … (5)
This is the distance of the first minimum from the centre of the central maximum.
∴ Width of the central bright fringe :
W_c = 2y_1d = 2W = 2\(\left(\frac{\lambda D}{a}\right)\) …(6)

The central bright fringe is spread between the first dark fringes on either side. Thus, the width of the central bright fringe is the distance between the centres of the first dark fringe on either side.

If the lens is very close to the slit, D is very nearly equal to f, where f is the focal length of the lens. Then W_c = 2\(\left(\frac{\lambda D}{a}\right)\) = 2\(\left(\frac{\lambda f}{a}\right)\) … (7)

Question 66.
Represent graphically intensity distribution in
(a) Young’s double-slit interference
(b) single- slit diffraction and
(c) double-slit diffraction
Answer:

Question 67.
State the characteristics of a single-slit diffraction pattern.
Answer:
Characteristics of a single-slit diffraction pattern :

1. The image cast by a single-slit is not the expected purely geometrical image.
2. For a given wavelength, the width of the diffraction pattern is inversely proportional to the slit width.
3. For a given slit width a, the width of the diffraction pattern is proportional to the wavelength.
4. The intensities of the non-central, i.e., secondary, maxima are much less than the intensity of the central maximum.
5. The minima and the non-central maxima are of the same width, Dλ/a.
6. The width of the central maximum is 2Dλ/a. It is twice the width of the non-central maxima or minima.

Question 68.
Explain briefly the double-slit diffraction pattern.
Answer:
The double-slit diffraction pattern is determined by the diffraction patterns due to the individual slits, and by the interference between them.

We can see narrow interference fringes similar to those obtained in Young’s double-slit experiment. These fringes vary in brightness and the shape of their envelope as that of the single-slit diffraction pattern.

Question 69.
What should be the order of the size of an obstacle or aperture to produce diffraction of light?
Answer:
For pronounced diffraction, the size of an obstacle or aperture should be of the order of the wavelength of light or greater.
[Note : For diffraction from a single slit of width a with monochromatic light of wavelength λ, the condition for first minimum (dark fringe) is
sin θ₁ = \(\frac{\lambda}{a}\)
When a = λ, θ₁ = 90° and the central maximum spreads over 180°; then, while the diffraction is maximum, no fringe pattern is seen at all.

When a » X (say, a is of the order of a centimetre or more), θ₁ is so small that there is practically no diffraction and the illuminated region on the screen is almost as given by geometrical optics. However, diffraction pattern due to a straight-edge will always be seen at the edge of the illuminated region. Hence, for an observable fringe pattern due to a single slit, a should be of the order of X with a > λ..]

Question 70.
Solve the following.

Question 1.
Plane waves of light from a sodium lamp are incident on a slit of width 2 μm. A screen is located 2 m from the slit. Find the spacing between the first secondary maxima of two sodium lines as measured on the screen.
(Given : λ₁ = 5890 Å and ₂ = 5896 Å)
Solution:


This is the required spacing.

Question 2.
The diffraction pattern of a single slit of width 0. 5 cm is formed by a lens of focal length 40 cm. Calculate the distance between the first dark and the next bright fringe from the axis. The wavelength of light used is 4890 Å.
Solution :
Data : a = 0.5 cm = 0.5 × 10^-2 m,
D \(\simeq\) f = 40 cm = 0.4 m, λ = 4890 Å = 4.890 × 10^-7 m
The distance between the first dark fringe and the next bright fringe = \(\frac{\lambda D}{2 a}\)

Question 3.
Light of wavelength 6000 Å is incident on a slit of width 0.3 mm. A screen is placed parallel to the slit 2 m away from the slit. Find the position of the first dark fringe from the centre of the central maximum. Also, find the width of the central maximum.
Solution :
Data : λ = 6 × 10^-7 m, a = 0.3 mm = 3 × 10^-4 m,
D = 2 m
The distance y_m of the m th minimum from the centre of the central maximum is

The first dark fringe is 4 mm from the centre of the central fringe.
∴ Half-width of the central maximum = 4 mm
∴ The width of the central maximum = 2 × 4 mm = 8 mm

Question 4.
In a single-slit diffraction pattern, the distance between the first minimum on the right and the first minimum on the left is 5.2 mm. The screen on which the pattern is displayed is 80 cm from the slit and the wavelength of light is 5460 Å. Calculate the slit width.
Solution :
Data : y₁ (minimum, right) + y₁ (minimum, left)

Question 5.
In a single-slit diffraction pattern, the distance between the first minimum on the right and the first minimum on the left of the central maximum is 4 mm. The screen is 2 m from the slit and the wavelength of light used is 6000 Å. Calculate the width of the slit and the width of the central maximum.
Solution :
Data : y₁ (minimum, right) + y₁ (minimum, left)
= 4 mm = 4 × 10^-3 m, D = 2 m, λ = 6 × 10^-7 m

Question 6.
Determine the angular spread between the central maximum and first order maximum of the diffraction pattern due to a single slit of width 0.25 mm, when light of 6650 Å is incident on it normally.
Solution:
Data : λ = 6650 Å = 6650 × 10^-10m, a = 0.25 mm

This is the required angular spread.

Question 71.
Explain and define the resolving power of an optical instrument.
Answer:
Resolving power of an optical instrument:
The primary aim of using an optical instrument is to see fine details, whether observing a star system through a telescope or a living cell through a microscope. After passing through an optical system, light from two adjacent parts of the object should produce sharp, distinct (separate) images of those parts. The objective lens or mirror of a telescope or microscope acts like a circular aperture. The diffraction pattern of a circular aperture consists of a central bright spot (called the Airy disc and corresponds to the central maximum) and concentric dark and bright rings.

Light from two close objects or parts of an object after passing through the aperture of an optical system produces overlapping diffraction patterns that tend to obscure the image. If these diffraction patterns are so broad that their central maxima overlap substantially, it is difficult to decide if the intensity distribution is produced by two separate objects or by one.

The resolving power of an optical instrument, e.g., a telescope or microscope, is a measure of its ability to produce detectably separate images of objects that are close together.

Definition : The smallest linear or angular separation between two point objects which appear just resolved when viewed through an optical instrument is called the limit of resolution of the instrument and its reciprocal is called the resolving power of the instrument.

Question 72.
State and explain Rayleigh’s criterion for minimum resolution.
Answer:
Rayleigh’s criterion for minimum resolution : Two overlapping diffraction patterns due to two point sources are acceptably or just resolved if the centre of the central peak of one diffraction pattern is as far as the first minimum of the other pattern.

The ‘sharpness’ of the central maximum of a diffraction pattern is measured by the angular separation between the centre of the peak and the first minimum. It gives the limit of resolution.

Two overlapping diffraction patterns due to two point sources are not resolved if the angular separation between the central peaks is less than the limit of resolution. They are said to be just separate, or resolved, if the angular separation between the central peaks is equal to the limit of resolution. They are said to be well resolved if the angular separation between the central peaks is more than the limit of resolution.

Question 73.
Explain the Rayleigh criterion for the limit of resolution for
(i) two linear objects
(ii) a pair of point objects.
Answer:
(i) The Rayleigh criterion for the limit of resolution for two linear objects : Consider, two self luminous objects or slits separated by some distance. Let λ be the wavelength of the light and a the width of the slits. As per the Rayleigh criterion, the first minimum of the diffraction pattern of one of the sources should coincide with the central maximum of the other. Thus, it is at the just resolved condition.
The angular separation dθ (position) of the first principal minimum is,
dθ = \(\frac{\lambda}{a}\) …(1)
This angular separation between the two objects must be minimum as this minimum coincides with the central maximum of the other. This is called the limit of resolution of that instrument. It is written as,
limit of resolution, dθ = \(\frac{\lambda}{a}\)
Minimum separation between the two linear objects that are just resolved, at distance D from the instrument is, ni
y = D(dθ) = \(\frac{D \lambda}{a}\) …(2)
It is the distance of the first minimum from the centre.

(ii) The Rayleigh criterion for the limit of resolution for a pair of point objects : The objects to be viewed through a microscope are often of the point-size. The diffraction pattern of such objects consists of a central bright spot called the Airy disc and corresponds to the central maximum surrounded by concentric dark and bright rings called Airy rings.

If a red laser beam passes through a 90 µm pinhole aperture, the Airy disc and several orders (rings) of diffraction are as shown.

According to Lord Rayleigh, for such objects to be just resolved, the first dark ring of the diffraction pattern of the first object should be formed at the centre of the diffraction pattern of the second object and vice versa. Thus, the minimum separation between the images on the screen should be equal to the radius of the first dark ring.
This is applicable to the eye, microscope, telescope, etc.

Question 74.
Define and explain the resolving power of a microscope. State the expressions for the resolving power of
(i) a microscope with a pair of non-luminous objects
(ii) a microscope with self luminous point objects.
OR
What is meant by the limit of resolution and the resolving power of a microscope?
Answer:
Definition : The limit of resolution of a microscope is the least separation between two-closely spaced points on an object which are just resolved when viewed through the microscope.

Definition : The resolving power of a microscope is defined to be the reciprocal of its limit of resolution.

In a compound microscope, the objective lens forms a real, magnified image of an object placed just beyond the focal length of the lens. The objective has a short focal length (for greater magnification) and is held close to the object so that it gathers as much of the light scattered by the object as possible.

Let a be the least separation between two point objects O and O’ viewed through an objective AB of a compound microscope. The medium between the object and the objective has a refractive index n. The images of the objects O and O’ are I and I’ respectively. In this case, the angular separation between the objects, at the objective is 2α. D is the diameter of the objective AB.

According to the Rayleigh criterion, the first dark ring due to O’ should coincide with I and that of O should coincide with I’. The nature of illumination at a point on the screen is determined by the effective path difference at that point. Let us consider point I to be symmetric with respect to O. Paths of the extreme rays reaching I from O’ are O’AI and O’BI. The paths AI and BI are equal. Thus, the actual path difference is O’B – O’A.

The enlarged view of the region around O and O’ is as shown.
From this,
path difference = DO’ + O’C
= 2a sin α

(i) Microscope with a pair of non-luminous objects (dark objects):
In actual practice, the objects O and O’ viewed through a microscope are illuminated by the same source. Often the eyepiece of the microscope is filled with some transparent material of refractive index n. Then the wavelength of light in this material is
λ_n = \(\frac{\lambda}{n}\) where λ is the wavelength of light in air.

In such a set up the path difference at the first dark ring in λ_n. Thus, from eq (1),

The factor n sin a is called numerical aperture (NA). The resolving power of the microscope is
R = \(\frac{1}{a}\) = \(\frac{2 \mathrm{NA}}{\lambda}\) …. (3)

(ii) Microscope with self luminous point objects : Applying Abbe’s theory of Airy discs and rings to Fraunhofer diffraction due to a pair of self luminous point objects, the path difference between the extreme rays, at the first dark ring is 1.22 λ, thus, for the requirement of just resolution,

The resolving power of the microscope is,
R = \(\frac{1}{a}\) = \(\frac{\mathrm{NA}}{0.61 \lambda}\) …. (5)
When the value of a is minimum, the quality of resolution is high.
Notes:

1. If f and D are the focal length and the diameter of the microscope objective.
   sin i_max \(\simeq \frac{D / 2}{f}\) so that Eq. (3) can be written as resolving power = \(\frac{n D}{f \lambda}\)
2. Ernst Abbe(1840 -1905), German physicist and developer of optical instruments.

Question 75.
Explain why microscopes of high magnifying power have oil filled (oil-immersion) objectives.
Answer:
Higher angular magnification of a high magnifying power microscope is of little use if the finer details in a tiny object are obscured by diffraction effects. Hence, a microscope of high magnifying power must also have a high resolving power.
Resolving power ot a microscope = \(\frac{2 n \sin \alpha}{\lambda}\)
Where α ≡ the half angle of the angular separation between the objects, at the objective lens. n ≡ the refractive index of the medium between the object and the objective, λ ≡ the wavelength of the light used to illuminate the object.

The factor n sin α is called the numerical aperture of the objective and the resolving power increases with increase in the numerical aperture. To increase α the diameter of the objective would have to be increased. But this increase in aperture would degrade the image by decreasing the resolving power. Hence, in microscopes of high magnifying power, the object is immersed in oil that is in contact with the objective. Usually cedarwood oil having a refractive index 1.5 (close to that of the objective glass) is used. Closeness of the refractive indices also reduces loss of light by reflection at the objective lens.

Question 76.
On what factors does the resolving power of a microscope depend? How can it be increased?
Answer:
Resolving power of a microscope
= \(\frac{2 n \sin \alpha}{\lambda}\) = \(\frac{2 \mathrm{NA}}{\lambda}\)

where, α ≡ the half angle of the angular separation between the objects at the objective lens. n ≡ the refractive index of the medium between the object and the objective, λ ≡ the wavelength of the light used to illuminate the object, NA = n sin α = the numerical aperture of the objective.

Thus, the resolving power of a microscope depends directly on the NA and inversely on λ.
The resolving power is increased by,

1. increasing the numerical aperture using oil-immersion objective.
2. illuminating the object with smaller wavelength radiation. But our eyes are not very sensitive to the shorter wavelength blue end of the visible spectrum. Hence, ultraviolet radiation is used for illumination with quartz lenses, but then photographs must be taken to examine the image.

Question 77.
With a neat ray diagram, explain the resolving power of a telescope. On what factors does it depend ?
OR
What is meant by the angular limit of resolution and resolving power of a telescope?
Answer:
The resolving power of a telescope is defined as the reciprocal of the angular limit of resolution between two closely-spaced distant objects so that they are just resolved when seen through the telescope.

Consider two stars seen through a telescope. The diameter (D) of the objective lens or mirror corresponds to the diffracting aperture. For a distant point source, the first diffraction minimum is at an angle θ away from the centre such that

Dsin θ = 1.22 λ
where λ is the wavelength of light. The angle 9 is usually so small that we can substitute sin θ \(\approx\) θ (θ in radian). Thus, the Airy disc for each star will be spread out over an angular half-width θ = 1.22 λ/D about its geometrical image point. The radius of the Airy disc at the focal plane of the objective lens is r = fθ = 1.22fλ/D, where f is the focal length of the objective.

When observing two closely-spaced stars, the Rayleigh criterion for just resolving the images as that of two point sources (instead of one) is met when the centre of one Airy disc falls on the first minimum of the other pattern. Thus, the angular limit (or angular separation) of resolution is
θ = \(\frac{1.22 \lambda}{D}\) … (1)

and the linear separation between the images at the focal plane of the objective lens is
y = fθ … (2)
∴ Resolving power of a telescope.
R = \(\frac{1}{\theta}\) = \(\frac{D}{1.22 \lambda}\) … (3)

It depends

1. directly on the diameter of the objective lens or mirror,
2. inversely on the wavelength of the radiation.

Question 78.
How can the resolving power of a telescope be increased?
Answer:
The resolving power of an astronomical telescope depends directly on the diameter of the objective lens or mirror, and inversely on the wavelength of radiation. Hence, the resolving power can be increased by

1. using an objective lens/mirror of larger diameter
2. observing a celestial object at smaller wavelengths.

Question 79.
Define the resolving power of a telescope and state its formula. What are the advantages of using a large objective lens in an astronomical telescope:
Answer:
Definition: The resolving power of a telescope is defined as the reciprocal of the angular limit of resolution between two closely-spaced distant objects so that they are just resolved when seen through the telescope.
Formula : Resolving power of a telescope
R = \(\frac{1}{\theta}\) = \(\frac{D}{1.22 \lambda}\)
where θ ≡ the minimum angular separation of two closely-spaced celestial objects or the angular limit of resolution, D ≡ the diameter of the objective lens of the telescope, λ ≡ the wavelengh of light.

Advantages of a large objective lens in an astronomical telescope :

1. The resolving power is directly proportional to the diameter of the objective lens. Hence, a large objective lens results in a smaller Airy disc and a sharper image.
2. It collects more of the incident radiation from a distant object which results in a brighter image.

Question 80.
What is the resolving power of a telescope if the diameter of the objective of the telescope is 1.22 m and the wavelength of light is 5000 Å ?
Answer:
Resolving power of the telescope = \(\frac{D}{1.22 \lambda}\)
= \(\frac{1.22}{1.22 \times 5 \times 10^{-7}}\)
= \(\frac{10 \times 10^{6}}{5}\) = 2 × 10⁶ rad^-1

Question 81.
Briefly explain about radio telescope.
Answer:
A radio telescope is an astronomical instrument consisting of a radio receiver and an antenna system used to detect radio-frequency radiation emitted by extraterrestrial sources. The wavelength of radio-frequency radiation is very long. Hence, it is expressed in metre. In order to attain the resolution of an optical telescope, the radio telescope should be very large. A single disc of such large diameters is impracticable. In such cases arrays of antennae spread over several kilometres are used. Giant Metrewave Radio Telescope (GMRT) located at Narayangaon, Pune, Maharashtra uses such an array consisting of 30 dishes of diameter 45 m each. It spreads over 25 km. It is the largest distance between two of its antennae. The highest angular resolution achievable ranges from about 60 arcsec at the lowest frequency of 50 MHz to about 2 arcsec at 1.4 GHz.

Question 82.
Solve the following :

Question 1.
Light of wavelength 650 nm falls on the objective of a microscope having total angle of angular w separation as 60°. Calculate the resolving power of the microscope. [Refractive index of air = 1]
Solution :
Data : λ = 650 nm = 6.5 × 10^-7m, n = 1,
2α = 60°
∴ α = 30°
Resolving power of the microscope = \(\frac{2 n \sin \alpha}{\lambda}\)
= \(\frac{2 \times 1 \times \sin 30^{\circ}}{6.5 \times 10^{-7}}\) = \(\frac{10}{6.5}\) × 10⁶

Question 2.
The semi vertical angle of the cone of the rays incident on the objective of a microscope is 20°. If the wavelength of incident light is 6600 Å, calculate the smallest distance between two points which can be just resolved.
Solution :
Data : α = 20°, λ = 6600 Å = 6.6 × 10^-7 m, n = 1 (air) resolution between two closely-spaced distant objects so that they are just resolved when seen through the telescope.
Formula : Resolving power of a telescope
R = \(\frac{1}{\theta}\) = \(\frac{D}{1.22 \lambda}\)
where θ ≡ the minimum angular separation of two closely-spaced celestial objects or the angular limit of resolution, D ≡ the diameter of the objective lens of the telescope, λ ≡ the wavelengh of light.

Advantages of a large objective lens in an astronomical telescope :

1. The resolving power is directly proportional to the diameter of the objective lens. Hence, a large objective lens results in a smaller Airy disc and a sharper image.
2. It collects more of the incident radiation from a distant object which results in a brighter image.

Question 83.
What is the resolving power of a telescope if the diameter of the objective of the telescope is 1.22 m and the wavelength of light is 5000 Å ?.
Answer:
Resolving power of the telescope = \(\frac{D}{1.22 \lambda}\)

Question 84.
Briefly explain about radio telescope.
Answer:
A radio telescope is an astronomical instrument consisting of a radio receiver and an antenna system used to detect radio-frequency radiation emitted by extraterrestrial sources. The wavelength of radio-frequency radiation is very long. Hence, it is expressed in metre. In order to attain the resolution of an optical telescope, the radio telescope should be very large. A single disc of such large diameters is impracticable. In such cases arrays of antennae spread over several kilometres are used. Giant Metrewave Radio Telescope (GMRT) located at Narayangaon, Pune, Maharashtra uses such an array consisting of 30 dishes of diameter 45 m each. It spreads over 25 km. It is the largest distance between two of its antennae. The highest angular resolution achievable ranges from about 60 arcsec at the lowest frequency of 50 MHz to about 2 arcsec at 1.4 GHz.

Question 85.
Solve the following :

Question 1.
Light of wavelength 650 nm falls on the objective of a microscope having total angle of angular separation as 60°. Calculate the resolving power of the microscope. [Refractive index of air = 1]
Solution :
Data : λ = 650 nm = 6.5 × 10^-7m, n = 1, 2α = 60°
∴ α = 30°
Resolving power of the microscope = \(\frac{2 n \sin \alpha}{\lambda}\)

= 1.538 × 10⁶ m^-1

Question 2.
The semi vertical angle of the cone of the rays incident on the objective of a microscope is 20°. If the wavelength of incident light is 6600 Å, calculate the smallest distance between two points which can be just resolved.
Solution :
Data : α = 20°, λ = 6600 Å = 6.6 × 10^-7 m, n = 1 (air)
The numerical aperture, NA = n sin α
= 1 × sin 20° = 0.3420
The limit of resolution for an illuminated object,

Question 3.
What is the minimum distance between two objects which can be resolved by a microscope having the visual angle of 30° when light of wavelength 600 nm is used ?
Solution :

Question 4.
The two headlights of an approaching automobile are 1.22 m apart. At what maximum distance will eye resolve them? Assume a pupil diameter of 5.0 mm and λ = 5500 Å. Assume also that this distance is determined only by the diffraction effect at the circular aperture.
Solution:
Data : y = 1.22 m, diameter D = 5 × 10^-3 m,

This is the required distance.

Question 5.
What is the minimum angular separation between two stars if a telescope is used to observe them with an objective of aperture 20 cm? The wavelength of light is 5900 Å.
Solution :
Data : D = 20 cm = 0.2 m,
λ = 5900 Å = 5.9 × 10^-7 m

This is the required angular separation.

Question 6.
The diameter of the objective of a telescope is 10 cm. Find the resolving power of the telescope is 10 cm. Find the resolving power of the telescope if the wavelength of light is 5000 Å.
Solution :
Data : D = 10 cm = 0.1 m, λ = 5000 Å = 5 × 10^-7 m
The resolving power of the telescope D 0.1

Question 7.
A telescope has an objective of diameter 2.44 m. What is its angular resolution when it observes at 5500 A?
Solution :
Data : λ = 5500A = 5.5 × 10^-7 m,
D = 2.44 m
Angular resolution. ∆θ = 1.22λ/D, D being the diameter of the aperture.
∴ ∆θ = 1.22 × 5.5 × \(\frac{10^{-7}}{2.44}\)= 2.75 × 10^-7 rad
= 0.0567 arcsec

Question 8.
The minimum angular separation between two stars is 4 × 10^-6 rad when a telescope is used to observe them with an objective of aperture 16 cm. Find the wavelength of the light.
Solution :
Data : θ = 4 × 10^-6 rad, D = 16 cm = 0.16 m
θ = \(\frac{1.22 \lambda}{D}\)
∴ The wavelength of the light used,

Question 9.
Estimate the smallest angular separation of two stars which can be just resolved by the telescope having objective of diameter 25 cm. The mean wavelength of light is 555 nm.
Solution :
Data : λ = 555 nm 555 × 10^-9 m
D = 25 cm = 25 × 10^-2 m

= 2.708 × 10^-6 rad
This is the required angular separation.

Question 86.
Huygens’ wave theory could not explain
(A) interference
(B) reflection
(C) photoelectric effect
(D) refraction.
Answer:
(C) photoelectric effect

Question 87.
The wavefront originating from a point source of light at finite distance is a wavefront.
(A) circular
(B) spherical
(C) plane
(D) cylindrical
Answer:
(B) spherical

Question 88.
In an isotropic medium, the secondary wavelets centred on every point of a given wavefront are all
(A) spherical
(B) cylindrical
(C) oval
(D) of arbitrary shape.
Answer:
(A) spherical

Question 89.
Consider a medium through which light is propagating with a speed v. Given a wavefront, in order to determine the wavefront after a time interval ∆t, the secondary wavelets are drawn with
a radius.
(A) of unit length
(B) v ∆ t
(C) \(\frac{\Delta t}{v}\)
(D) \(\frac{v}{\Delta t}\)
Answer:
(B) v ∆ t

Question 90.
Two points, equidistant from a point source of light, are situated at diametrically opposite positions in an isotropic medium. The phase difference between the light waves passing through the two points is
(A) zero
(B) π/2 rad
(C) π rad
(D) finite, but not these.
Answer:
(A) zero

Question 91.
Wavenormals to spherical wavefronts can be
(A) only diverging
(B) only converging
(C) parallel to each other
(D) diverging or converging.
Answer:
(D) diverging or converging.

Question 92.
Huygens’ principle is used to
(A) obtain the new position of wavefront geometrically
(B) explain the principle of superposition of waves
(C) explain the phenomenon of interference
(D) explain the phenomenon of polarization.
Answer:
(A) obtain the new position of wavefront geometrically

Question 93.
When a ray of light enters into water from air,
(A) its wavelength decreases
(B) its wavelength increases
(C) its frequency increases
(D) its frequency decreases.
Answer:
(A) its wavelength decreases

Question 94.
A parallel beam of light travelling in water is incident obliquely on a glass surface. After refraction, its width
(A) decreases
(B) increases
(C) remains the same
(D) becomes zero.
Answer:
(B) increases

Question 95.
Light of a certain colour has 1800 waves to the millimetre in air. What is its frequency in water?
[n = \(\frac{4}{3}\) for water]
(A) 1.67 × 10⁶ Hz
(B) 4.05 × 10¹⁴ Hz
(C) 5.4 × 10¹⁴ Hz
(D) 7.2 × 10¹⁴ Hz.
Answer:
(C) 5.4 × 10¹⁴ Hz

Question 96.
A ray of light, in passing from vacuum into a medium of refractive index n, suffers a deviation d equal to half the angle of incidence. Then, the refractive index is
(A) sin δ
(B) 2 sin δ
(C) cos δ
(D) 2 cos δ.
Answer:
(D) 2 cos δ.

Question 97.
A ray of light passes from vacuum to a medium of refractive index n. The angle of incidence is found to be twice the angle of refraction. The angle of incidence is
(A) cos^-1 \(\left(\frac{n}{2}\right)\)
(B) cos^-1 (n)
(C) 2cos^-1 \(\left(\frac{n}{2}\right)\)
(D) 2sin^-1 \(\left(\frac{n}{2}\right)\).
Answer:
(C) 2cos^-1 \(\left(\frac{n}{2}\right)\)

Question 98.
If the polarizing angle for a given medium is 60°, then the refractive index of the medium is
(A) \(\frac{1}{\sqrt{3}}\)
(B) \(\frac{\sqrt{3}}{2}\)
(C) 1
(D) \(\sqrt{3}\)
Answer:
(D) \(\sqrt{3}\)

Question 99.
A narrow beam of light in air is incident on glass at an angle of incidence of 58°. If the reflected beam is completely plane polarized, the refractive index of the glass is
(A) 1.9
(B) 1.8
(C) 1.7
(D) 1.6.
Answer:
(D) 1.6.

Question 100.
Polarization of light CANNOT be produced by
(A) reflection
(B) double refraction
(C) dichroism
(D) diffraction.
Answer:
(D) diffraction.

Question 101.
A glass plate of refractive index 1.732 is to be used as a polarizer. Its polarizing angle is
(A) 30°
(B) 45°
(C) 60°
(D) 90°.
Answer:
(C) 60°

Question 102.
Light transmitted through a Polaroid P₁ has an intensity I and is incident on a crossed Polaroid P₂. The intensity of the light transmitted by P₂ is
(A) zero
(B) \(\frac{1}{2}\)I
(C) I
(D) 2I.
Answer:
(A) zero

Question 103.
At points of constructive interference with maximum intensity of two coherent monochromatic waves (wavelength λ), the path difference between them is
(A) zero or an integral multiple of λ
(B) zero or an integral multiple of λ/2
(C) zero or an even integral multiple of λ/2
(D) an odd integral multiple of λ/2.
Answer:
(A) zero or an integral multiple of λ

Question 104.
Two sources of light are said to be coherent if light from them have
(A) the same speed and the same phase
(B) the same phase and the same or nearly the same amplitude
(C) constant phase difference and nearly the same frequency
(D) zero, or some constant, phase difference.
Answer:
(D) zero, or some constant, phase difference.

Question 105.
In a two-source interference pattern, the phase difference between the waves reaching a dark point in radian is (m = 1, 2, 3, …)
(A) 0
(B) mπ
(C) (2m – 1)\(\frac{\pi}{2}\)
(D) (2m – 1)π
Answer:
(D) (2m – 1)π

Question 106.
For destructive interference, the phase difference (in radian) between the two waves should be
(A) 0, 2π, π, …
(B) 0, 2π, 4π, …
(C) π, 3π, 5π, …
(D) \(\frac{\pi}{2}\), \(\frac{3 \pi}{2}\), \(\frac{5 \pi}{2}\), …..
Answer:
(C) π, 3π, 5π, …

Question 107.
For constructive interference, the phase difference (in radian) between the two waves should be
(A) 0, \(\frac{\pi}{2}\), π, …
(B) 0, 2π, 4π, …
(C) π, 3π, 5π, …
(D) \(\frac{\pi}{4}\), \(\frac{\pi}{2}\), \(\frac{3 \pi}{4}\), …..
Answer:
(B) 0, 2π, 4π, …

Question 108.
If λ is the wavelength of light used in Young’s double-slit experiment, the path difference for a phase difference of 11π rad is
(A) 23 λ
(B) 11 λ
(C) 11\(\frac{\lambda}{2}\)
(D) 23\(\frac{\lambda}{2}\)
Answer:
(C) 11\(\frac{\lambda}{2}\)

Question 109.
The fringe width in an interference pattern is W. The distance between the 6th dark fringe and the 4th bright fringe on the same side of the central bright fringe is
(A) 1.5 W
(B) 2 W
(C) 2.5 W
(D) 10.5 W.
Answer:
(A) 1.5 W

Question 110.
Which of the following graphs shows the variation of the fringe width with the frequency of light in a two-source interference pattern ?

Answer:

Question 111.
In an interference pattern using two coherent sources of light, the fringe width is
(A) directly proportional to the wavelength
(B) inversely proportional to the square of the wavelength
(C) inversely proportional to the wavelength
(D) directly proportional to the square of the wavelength.
Answer:
(A) directly proportional to the wavelength

Question 112.
Two slits, 2 mm apart, are placed 300 cm from a screen. When light of wavelength 6000 Å is used, the separation (in mm) between the successive bright lines of the interference pattern is
(A) 0.9
(B) 4.5
(C) 6
(D) 9.
Answer:
(A) 0.9

Question 113.
In two separate setups of Young’s double-slit experiment, the wavelengths of the lights used are in the ratio 1 : 2 while the separation between the slits are in the ratio 2 : 1. If the fringe widths are equal, the ratio of the distances between the slit and the screen is
(A) 1 : 4
(B) 1 : 2
(C) 2 : 1
(D) 4 : 1.
Answer:
(D) 4 : 1.

Question 114.
In Young’s double-slit experiment, the slit separation is reduced to half while the distance of the screen from the slits is increased by 50%. In terms of the initial fringe width, W, the new fringe width is,
(A) \(\frac{1}{4}\) W
(B) \(\frac{3}{4}\) W
(C) \(\frac{3}{2}\) W
(D) 3 W.
Answer:
(D) 3 W.

Question 115.
A pair of slits 1.5 mm apart is illuminated with monochromatic light of wavelength 5500 Å and the interference pattern is obtained on a screen 1.5 m from the slits. The least distance of a point from the central maximum where the intensity is minimum is
(A) 0.275 mm
(B) 0.55 mm
(C) 2.75 mm
(D) 5.5 mm.
Answer:
(A) 0.275 mm

Question 116.
In Young’s double-slit experiment, if a thin transparent sheet covers one of the slits, the optical path of the wave from that slit
(A) increases
(B) decreases
(C) changes by \(\frac{D}{d}\) (n_m – 1)t
(D) is not affected.
Answer:
(A) increases

Question 117.
In Young’s double-slit experiment, if a thin mica sheet of thickness t and refractive index n_m covers one of the slits, the optical path of the wave from that slit
(A) increases by (n_m – 1 )t
(B) decreases by (n_m – 1) t
(C) changes by \(\frac{D}{d}\) (n_m – 1) t
(D) is not affected.
Answer:
(A) increases by (n_m – 1 )t

Question 118.
In a two-slit intereference experiment, if a thin transparent sheet of thickness f and refractive index n_m covers both the slits, the optical path difference between the two interfering waves
(A) increases by (n_m – 1)t
(B) decreases by (n_m – 1)t
(C) changes by \(\frac{D}{d}\)(n_m – 1)t
(D) is not affected.
Answer:
(D) is not affected.

Question 119.
In a biprism experiment two interfering waves are produced by division of
(A) amplitude
(B) wavefront
(C) amplitude and wavefront
(D) neither wavefront nor amplitude.
Answer:
(B) wavefront

Question 120.
In Fresnel’s biprism experiment, with the eyepiece 1 m from the two coherent sources, the fringe width obtained is 0.4 mm. If just the eyepiece is moved towards the biprism by 25 cm, then the fringe width
(A) decreases by 0.01 mm
(B) decreases by 0.1 mm
(C) increases by 0.01 mm
(D) increases by 0.1 mm.
Answer:
(B) decreases by 0.1 mm

Question 121.
In a biprism experiment, keeping the experimental setup unchanged, the fringe width
(A) increases with increase in wavelength
(B) decreases with increase in wavelength
(C) increases with decrease in wavelength
(D) remains unchanged with change in wavelength.
Answer:
(A) increases with increase in wavelength

Question 122.
In finding the distance between the two coherent sources in Fresnel’s biprism experiment by the conjugate foci method, one uses
(A) a long focus convex lens that forms real images of the virtual sources
(B) a short focus concave lens that forms real images of the virtual sources
(C) a short focus convex lens that forms virtual images of the virtual sources
(D) a short focus convex lens that forms real images of the virtual sources.
Answer:
(D) a short focus convex lens that forms real images of the virtual sources.

Question 123.
Using a light of wavelength 4800 Å in Fresnel’s biprism experiment, 21 fringes are obtained in a given region. If light of wavelength 5600 Å is used, the number of fringes in the same region will be
(A) 14
(B) 18
(C) 21
(D) 24.
Answer:
(B) 18

Question 124.
To obtain pronounced diffraction with a single slit illuminated by light of wavelength λ, the slit width should be
(A) of the same order as λ
(B) considerably larger than λ
(C) considerably smaller than λ
(D) exactly equal to λ/2.
Answer:
(B) considerably larger than λ

Question 125.
In single-slit diffraction, which of the following are equal ?
(A) Widths of all bright and dark fringes
(B) Intensities of non-central bright fringes
(C) Widths of non-central bright fringes
(D) Both widths and intensities of noncentral bright fringes.
Answer:
(C) Widths of non-central bright fringes

Question 126.
In single slit diffraction (at a narrow slit of width a), the intensity of the central maximum is
(A) independent of a
(B) proportional to a
(C) proportional to a²
(D) inversely proportional to a.
Answer:
(D) inversely proportional to a.

Question 127.
For a single slit of width a, the diffraction pattern minima are located at angles θ_m, where m is a positive, non-zero integer. Which of the following expressions is most correct ?
(A) a sin θ_m = mλ
(B) a sin θ_m = \(\frac{m \lambda}{2}\)
(C) a sin θ_m = (2m + 1)\(\frac{\lambda}{2}\)
(D) a sin θ_m = (2m – 1)\(\frac{\lambda}{2}\)
Answer:
(A) a sin θ_m = mλ

Question 128.
In a diffraction pattern due to a single slit of width a with incident light of wavelength λ, at an angle of diffraction θ, the condition for the first minimum is
(A) λ sin θ = a
(B) a cos θ = λ
(C) a sin θ = λ
(D) λ cos θ = a.
Answer:
(C) a sin θ = λ

Question 129.
The fringes produced in a diffraction pattern are of
(A) equal width with the same intensity
(B) unequal width with varying intensity
(C) equal intensity
(D) equal width with varying intensity.
Answer:
(B) unequal width with varying intensity

Question 130.
For a single slit of width a, the first diffraction maximum with light of wavelength λ subtends an angle θ such that sin θ is equal to
(A) \(\frac{\lambda}{2 a}\)
(B) \(\frac{\lambda}{a}\)
(C) \(\frac{1.5 \lambda}{a}\)
(D) \(\frac{2 \lambda}{a} .\)
Answer:
(C) \(\frac{1.5 \lambda}{a}\)

Question 131.
Fraunhofer diffraction pattern of a parallel beam of light (wavelength λ) passing through a narrow slit (width a) is observed on a screen using a convex lens (focal length f). The angular half-width of the central fringe is
(A) \(\frac{2 \lambda f}{a}\)
(B) \(\frac{\lambda f}{a}\)
(C) \(\frac{2 \lambda}{a}\)
(D) \(\frac{\lambda}{a}\)
Answer:
(D) \(\frac{\lambda}{a}\)

Question 132.
A parallel beam of light (λ = 5000 Å) is incident normally on a narrow slit of width 0.2 mm. The angular separation between the first two minima is
(A) 2.5 × 10^-3 degree
(B) 2.5 × 10^-3 rad
(C) 5 × 10^-3 degree
(D) 5 × 10^-3 rad.
Answer:
(B) 2.5 × 10^-3 rad

Question 133.
A parallel beam of light (λ = 5000 Å) is incident normally on a narrow slit of width 0.2 mm. The Fraunhofer diffraction pattern is observed on a screen placed at the focal plane of a convex lens (f = 20 cm). The first two minima are separated by
(A) 0.005 cm
(B) 0.05 cm
(C) 2.5 mm
(D) 5 mm.
Answer:
(B) 0.05 cm

Question 134.
A plane wave of wavelength 5500 Å is incident normally on a slit of width 2 × 10^-2 cm. The width of the central maximum on a screen 50 cm away is
(A) 2.50 × 10^-3 cm
(B) 2.75 × 10^-3 cm
(C) 2.75 × 10^-3 m
(D) 5.50 × 10^-3 m.
Answer:
(D) 5.50 × 10^-3 m.

Question 135.
If NA is the numerical aperture of a microscope objective, then its resolving power with an illumination of wavelength λ is
(A) \(\frac{0.5 \lambda}{\mathrm{NA}}\)
(B) \(\frac{0.61 \lambda}{\mathrm{NA}}\)
(C) \(\frac{1.22 \mathrm{NA}}{\lambda}\)
(D) \(\frac{2 \mathrm{NA}}{\lambda}\)
Answer:
(A) \(\frac{0.5 \lambda}{\mathrm{NA}}\)

Question 136.
A microscope with numerical aperture 0.122 is used with light of wavelength 6000 A. The limit of resolution is
(A) 3.33 × 10⁶ m
(B) 3.33 mm
(C) 3 × 10^-6 m
(D) 3 × 10^-7 m.
Answer:
(C) 3 × 10^-6 m

Question 137.
The objective lens of a telescope has a diameter D. The angular limit of resolution of the telescope for light of wavelength λ is
(A) \(\frac{D}{1.22 \lambda}\)
(B) \(\frac{1.22 \lambda}{D}\)
(C) \(\frac{D}{0.61 \lambda}\)
(D) \(\frac{0.61 \lambda}{D} .\)
Answer:
(B) \(\frac{1.22 \lambda}{D}\)

Question 138.
Two closely-spaced distant stars are just resolved when seen through a telescope with an objective lens of diameter D and focal length f. The separation between their images is given by.
(A) \(\frac{D}{1.22 \lambda f}\)
(B) \(\frac{f \mathcal{D}}{1.22 \lambda}\)
(C) \(\frac{1.22 \lambda f}{D}\)
(D) \(\frac{1.22 D \lambda}{f}\)
Answer:
(C) \(\frac{1.22 \lambda f}{D}\)

Question 139.
High magnifying power microscopes have oil-immersion objectives
(A) to increase the fringe width
(B) to increase the numerical aperture of the objective
(C) to decrease the wavelength of light
(D) because oil does not damage the observed sample.
Answer:
(B) to increase the numerical aperture of the objective

Question 140.
If the numerical aperture of a microscope is increased, then its
(A) resolving power decreases
(B) limit of resolution decreases
(C) resolving power remains constant
(D) limit of resolution increases
Answer:
(B) limit of resolution decreases

Question 141.
The numerical aperture of the objective of a microscope is 0.12. The limit of resolution, when light of wavelength 6000 A is used to view an object, is
(A) 0.25 × 10^-7 m
(B) 2.5 × 10^-7m
(C) 25 × 10^-7 m
(D) 250 × 10^-7 m.
Answer:
(C) 25 × 10^-7 m

Question 142.
The resolving power of a refracting telescope is increased by
(A) using oil-immersion objective
(B) increasing the diameter D of the objective lens
(C) resorting to short-wavelength radiation
(D) increasing D and using smaller λ.
Answer:
(D) increasing D and using smaller λ.

Question 143.
The resolving power of a telescope of aperture 100 cm, for light of wavelength 5.5 × 10^-7 m, is
(A) 0.149 × 10⁷
(B) 1.49 × 10⁷
(C) 14.9 × 10⁷
(D) 149 × 10⁷
Answer:
(A) 0.149 × 10⁷

Question 144.
The resolving power of a telescope depends upon the
(A) length of the telescope
(B) focal length of the objective
(C) diameter of the objective
(D) focal length of the eyepiece.
Answer:
(C) diameter of the objective

Question 145.
If a is the aperture of a telescope and 2 is the wavelength of light then the resolving power of the telescope is
(A) \(\frac{\lambda}{1.22 a}\)
(B) \(\frac{1.22 a}{\lambda}\)
(C) \(\frac{1.22 \lambda}{a}\)
(D) \(\frac{a}{1.22 \lambda}\)
Answer:
(D) \(\frac{a}{1.22 \lambda}\)

Question 146.
Using a monochromatic light of wavelength 2 in Young’s double-slit experiment, the eleventh dark fringe is obtained on the screen for a phase difference of
(A) \(\frac{11}{2}\) π rad
(B) \(\frac{21}{2}\) π rad
(C) 13 π rad
(D) 21 π rad
Answer:
(D) 21 π rad

Balbharti Maharashtra State Board 12th Physics Important Questions Chapter 15 Structure of Atoms and Nuclei Important Questions and Answers.

Maharashtra State Board 12th Physics Important Questions Chapter 15 Structure of Atoms and Nuclei

Question 1.
What was Dalton’s atomic theory of chemistry?
Answer:
John Dalton (1766-1844), British meteorologist, in his atomic theory of chemistry (1808-1810) proposed the following postulates :
(1) Matter consists of very small indivisible particles called atoms.
(2) Each element consists of a characteristic kind of identical atoms. There are consequently as many different kinds of atoms as there are elements.
(3) When different elements combine to form a compound, the smallest unit of the compound consists of a definite number of atoms of each element. These ‘compound atoms’ are now called molecules.
(4) In chemical reactions, atoms are neither created nor destroyed, but only rearranged.

Question 2.
Explain Thomson’s model of the atom. What are its drawbacks?
Answer:
The first model of the atom with a sub-structure was put forward in 1898 by Sir J.J. Thomson (1856-1940), a British physicist. According to this model, an atom consists of a sphere with a uniform distribution of positive charge and electrons embedded in it such that the atom is electrically neutral and stable.

Drawbacks : This model, known as the plumpudding model, failed to account for the observed scattering of α-particles and spectra of various elements.
[Note : It can be shown that the Thomson atom cannot be stable.]

Question 3.
Who suggested the famous a-particle scattering experiment? Why?
Answer:
Sir Ernest Rutherford (1871-1937), New Zealand- British physicist, following his pioneering work on radioactivity and properties of a-particles, had noted that a narrow stream of α-particles gets somewhat broadened or scattered on passing through a thin metal foil or mica sheet. Since most of the α-particles remained undeviated, this suggested that atoms could not be solid spheres as proposed in Thomson’s model and kinetic theory of gases. To probe into the effects of the distribution of an atom’s mass and charge on the a-particles, he suggested his collaborator Geiger and the latter’s student Marsden to see if any α-particles are scattered through a large angle.

Question 4.
With the help of a neat labelled diagram, describe the Geiger-Marsden experiment.
Answer:
The Geiger-Marsden a-scattering (or gold foil) experiment (1908) : Geiger and Marsden made a stream of a-particles strike a very thin gold foil about 40 jum thick. Their apparatus is shown schematically in figure.

Apparatus: A radium compound, an intense source of a-particles, was placed in the lead enclosure B, provided with a small hole. The stream of α-particles was collimated by lead bricks. The number of particles scattered through each angle θ were counted by a rotatable detector. The detector consisted of a small zinc sulphide screen S at the focus of a low power microscope M. Each incidence produced a scintillation-a momentary pinpoint of fluorescence. These scintillations were observed and counted using the microscope.

Geiger-Marsden experiment of scattering of a-particles by a gold foil

Observations: Most of the α-particles passed through the foil almost undeviated, with less than 0.2% deflected by more than 1°. Still smaller fractions were found to be deflected by 90° or more, sometimes almost straight back towards the source.

Rutherford quantitatively accounted for the distribution of small and large angle scattering by considering each scattering to be a single collision of an a-particle with a positive ‘central charge’ Ne concentrated at a point. Since the probability of an a-particle coming very close to such a point charge was small, this explained the very small number of a-particles deflecting through large angles.

Scattering of a-particles by a gold foil

[Notes : (1) Hans Wilhelm Geiger (1882-1945), German physicist and Sir Ernest Marsden (1889-1970), English-New Zealand physicist. (2) In 1908 and 1909, they conducted a series of a-scattering experiments with gold and silver foils of different thicknesses and a thick platinum plate. Rutherford reported (in 1911) that “about 1 in 20000 were turned through 90° on passing through a gold foil about 40 nm thick.” The number ‘1 in 8000’ was reported (in 1909) by Geiger and Marsden for reflection off a thick platinum plate ‘at large angle.]

Question 5.
Explain Rutherford’s model of the atom.
Answer:
Rutherford’s model of the nuclear atom (1911) :
(1) An atom has a very small nucleus which contains all the positive charge and almost all the mass of the atom.
(2) The nuclear size (radius about 10^-14 m) is very small compared to the atomic size (radius about 10^-10m), about 10000 times smaller.
(3) Electrons revolve in circular orbits around the nucleus. The electrostatic force (Coulomb force) of attraction between the positively charged nucleus and the negatively charged electron is the centripetal force required for the orbital motion of the electron.
(4) Since an atom as a whole is electrically neutral, the positive charge on the nucleus must be equal to the total negative charge of all the orbiting electrons.
As this model resembles the solar system, it is known as the planetary atom model.

Question 6.
Solve the following :
(1) An a-particle having a kinetic energy of 8 MeV is projected directly toward the nucleus of an atom of \(\begin{gathered}
208 \\
82
\end{gathered}\)Pb. Find the distance of closest approach.
(e = 1.6 × 10^-19 C, ε₀ = 8.85 × 10^-12 C²/N∙m²)
Solution :
Data : e = 1.6 × 10^-19 C, Z(Pb) = 82, ε₀ = 8.85 × 10^-12 C²/N∙m², (KE)_α = 8 MeV
If d is the distance of closest approach, we must have, by the principle of conservation of energy, initial kinetic energy of the a-particle = potential energy of the a-particle when it is at the distance d from the centre of the nucleus of
\(\begin{gathered}
208 \\
82
\end{gathered}\) Pb.

(2) An α-particle projected directly toward the nucleus of an atom of \(\begin{gathered}
208 \\
82
\end{gathered}\)Pb comes to rest momentarily at the surface of the nucleus. Find the initial kinetic energy and speed of the α-particle.
[m(α) = 6.68 × 10^-27 kg, r₁(α) = 1.8 × 10^-15 m, r₂(Pb) = 7.11 × ^-15 m, e = 1.6 × 10^-19 C, \(\frac{1}{4 \pi \varepsilon_{0}}\) = 9 × 109 N-m2/C2]
Solution:
For reference, see the solved problem (1) above.

This is the initial speed of the α-particle.
[Note : 3.564 × 10⁷ m/s is about 12% of the speed of light in vacuum.]

Question 7.
What is the emission spectrum of a substance? Explain in brief.
Answer:
The emission spectrum of a substance is the distribution of electromagnetic radiations emitted by the substance when it is heated, or bombarded by electrons, or ions or photons. The distribution is arranged in order of increasing (or decreasing) frequency (or wavelength) and is characteristic of the substance unless the temperature of the substance is very high when the distribution is continuous. The spectrum may be a line spectrum or band spectrum. The intensities corresponding to different frequencies are different.
[Note : The absorption spectrum is formed by absorption of electromagnetic radiation when the substance is exposed to radiation of all frequencies.]

Question 8.
State and explain the formula that gives wavelengths of lines in the hydrogen spectrum.
Answer:
Formula : \(\frac{1}{\lambda}=R\left[\frac{1}{n^{2}}-\frac{1}{m^{2}}\right]\), where λ is the wavelength of a line in the hydrogen spectrum, R is a constant, now called the Rydberg constant, and n and m are integers with n = 1,2,3,… and m = n + 1, n + 2, n + 3,
For a fixed value of n, λ decreases as m increases and has minimum value as m → ∞ λ = \(\frac{n^{2}}{R}\) as

Lyman, Balmer and Paschen series in the hydrogen spectrum (For reference only)
[Note : A line spectrum is atomic in origin. It is a signature of the element, i.e., we can determine the elements present in a mixture of elements by studying the line spectrum of the mixture.]

Question 9.
State the equations corresponding to Bohr’s atomic model.
Answer:

Here, m_e is the mass of the electron, e is the electron charge, Z is the atomic number of the atom, r_n is the radius of the nth stable orbit, v_n is the speed of the electron in the n^th orbit and ε₀ is the absolute premittivity of free space. In hydrogen, Z = 1.
m_e v_n r_n = n\(\frac{h}{2 \pi}\) …………… (2)
[Angular momentum of the electron]

where n ( = 1, 2, 3, …) is the positive integer, called the principal quantum number, and h is Planck’s constant, n denotes the number of the orbit.
E_m – E_n = hv …………. (3)

Here, E_m is the energy of the electron in the m^th orbit, E_n is the energy of the electron in the nth orbit (m > n), hv is the energy of the photon emitted and v is the frequency of the electromagnetic radiation emitted.
[Note : Niels Bohr (1885-1962), Danish theoretical physicist, made significant contribution to atomic and
nuclear physics.]

Question 10.
What is the minimum angular momentum of the electron in an hydrogen atom?
Answer:
\(\frac{h}{2 \pi}\).

Question 11.
Which physical quantity of an atomic electron has the dimensions same as that of h?
Ans.
Angular momentum.

Question 12.
What is meant by a stationary orbit?
Answer:
In the Bohr model of the hydrogen atom, a stationary orbit refers to any of the discrete allowed orbits such that the electron does not radiate energy while it is in such orbits.

Question 13.
Derive an expression for the linear speed of an electron in a Bohr orbit. Hence, show that it is inversely proportional to the principal quantum number.
Answer:
Consider an electron revolving in the nth Bohr orbit around the nucleus of an atom with the atomic number Z. Let m and – e be the mass and charge of the electron, r the radius of the orbit and v the linear speed of the electron.

According to Bohr’s first postulate, centripetal force on the electron = electrostatic force of attraction exerted on the electron by the nucleus.
∴ \(\frac{m v^{2}}{r}=\frac{Z e^{2}}{4 \pi \varepsilon_{0} r^{2}}\) ………….. (1)
where ε₀ is the permittivity of free space.
∴mv² = \(\frac{Z e^{2}}{4 \pi \varepsilon_{0} r}\) ………….. (2)
According to Bohr’s second postulate, the orbital angular momentum of the electron is quantized :
mvr = \(\frac{n h}{2 \pi}\) ………… (3)
where h is Planck’s constant and n is the principal quantum number which takes integral values 1, 2, 3, …, etc.
∴ r = \(\frac{n h}{2 \pi m v}\)
Substituting this expression for r in Eqn (2), we get,

as Z, e, ε₀ and h are constants.
[Note : In this topic, unless stated otherwise, m = m_e, r = r_n, and v = v_n.]

Question 14.
What is the angular momentum of the electron in the third Bohr orbit in the hydrogen atom ?
[\(\frac{h}{2 \pi}\) = 1.055 × 10^-34 kg∙m²/s
Answer:
Angular momentum, L = mvr = \(\frac{nh}{2 \pi}\)
For n = 3, L = 3(\(\frac{h}{2 \pi}\)) = 3 (1.055 × 10^-34)
= 3.165 × 10^-34 kg∙m²/s

Question 15.
Derive an expression for the radius of the nth Bohr orbit in an atom. Hence, show that the radius of the orbit is directly proportional to the square of the principal quantum number.
Answer:
Consider an electron revolving in the nth orbit around the nucleus of an atom with the atomic number Z. Let m and – e be the mass and charge of the electron, r the radius of the orbit and v the linear speed of the electron.

According to Bohr’s first postulate, centripetal force on the electron = electrostatic force of attraction exerted on the electron by the nucleus.

where h is Planck’s constant and n is the principal quantum number which takes integral values 1, 2, 3, …… etc.

Since, ε₀, h, Z, in and e are constants, it follows that r ∝ n², i.e., the radius of a Bohr orbit of the electron in an atom is directly proportional to the square of the principal quantum number.

Question 16.
The radius of the first Bohr orbit in the hydro gen atom is 0.5315 Å. What is the radius of the second Bohr orbit in the hydrogen atom?
Ans.
In the usual notation,

∴ r₂ = 4r₁ = 4 × 0.5315 = 2.125 Å is the required radius.
[Note : r₁ is also denoted by a₀.]

Question 17. Show that the angular speed of an electron in the nth Bohr model is ω = \(\frac{\pi m e^{4}}{2 \varepsilon_{0}^{2} h^{3} n^{3}}\) and the corresponding frequency of the revolution of the electron is f = \(\) .
Answer:
The radius of the nth Bohr orbit is
r = \(\frac{\varepsilon_{0} h^{2} n^{2}}{\pi m Z e^{2}}\) ………….. (1)
and the linear speed of an electron in this orbit is
v = \(\frac{Z e^{2}}{2 \varepsilon_{0} n h}\) ………….. (2)
where ε₀ ≡ permittivity of free space, h ≡ Planck’s constant, n ≡ principal quantum number, m ≡ electron mass, e ≡ electronic charge and Z ≡ atomic number of the atom.

Since angular speed ω = \(\frac{v}{r}\), then from Eqs. (1) and (2) we get,

which gives the required expression for the angular speed of an electron in the nth Bohr orbit.
From Equestion (3), the frequency of revolution of the electron,

Question 18.
The angular speed of an electron in the first orbit in H-atom is 4.105 × 10¹⁶ rad/s. Find the angular speed of the electron in the second orbit.
Answer:

= 5.131 × 10¹⁵ rad/s This is the required quantity.

Question 19.
The frequency of revolution of the electron in the second Bohr orbit in the hydrogen atom is 8.158 × 10¹⁴ Hz. What is the frequency of revolution of the electron in the fourth Bohr orbit in the hydrogen atom ?
Ans.
In the usual notation,

Question 20.
Show that the energy of the electron in the nth stationary orbit in the hydrogen atom is
E_n = -Rch/n².
Answer:
The energy of the electron in the nth stationary orbit in the hydrogen atom is
E_n = \(-\frac{m e^{4}}{8 \varepsilon_{0}^{2} h^{2} n^{2}}\)
and the Rydberg constant is
R = \(\frac{m e^{4}}{8 \varepsilon_{0}^{2} h^{3} c}\)
where m = mass of electron, e = electronic charge, h = Planck’s constant, c = speed of light in free space and i, = permittivity of free space.

Question 21.
State the limitations of Bohr’s atomic model.
Answer:
Limitations of Bohr’s atomic model:

1. The model cannot explain the relative intensities of spectral lines even in the hydrogen spectrum.
2. The model cannot explain the atomic spectra of many-electron atoms of higher elements.
3. The model cannot account for the Zeeman effect and Stark effect (fine structure of spectral lines as revealed in the presence of strong magnetic field and electric field, respectively).

[Note : In 1896, Pieter Zeeman (1865-1943), Dutch physicist, discovered the splitting of spectral lines by magnetic field. In 1913, Johannes Stark (1874-1957), German physicist, discovered the splitting of spectral lines by electric field.]

Question 22.
Draw a neat, labelled energy level diagram for the hydrogen atom. Hence explain the different series of spectral lines for hydrogen.
Answer:
According to Bohr’s model of the hydrogen atom, an atom exists most of the time in one of a number of stable and discrete energy states. The various states arranged in order of increasing energy constitute the energy level diagram of the atom, as shown in below figure for the hydrogen atom. Here, the higher (less negative) energies are at the top while the lower (more negative) energies are toward the bottom.

According to Bohr’s theory, electromagnetic radiation of a particular wavelength is emitted when there is a transition of the electron from a higher energy state to a lower energy state. Let the quantum number n = m represent a higher energy state and n = n represent a lower energy state (m > n). The formation of the different series of spectral lines is explained from the energy level diagram.

(1) Lyman series : This series in the far UV region of the spectrum arises due to the transitions of the electron to n = 1 from m = 2, 3, 4, …, etc. The wavelengths (λ) of the spectral lines in this series are given by
\(\frac{1}{\lambda}=R\left(\frac{1}{1^{2}}-\frac{1}{m^{2}}\right)\) (m = 2, 3, 4, ……….,∞)
where R is the Rydberg constant.

(2) Balmer series : This series in the visible region of the spectrum arises due to the transitions to n = 2 from m = 3, 4, 5, …, etc. The wavelengths (λ) of the spectral lines in this series are given by
\(\frac{1}{\lambda}=R\left(\frac{1}{2^{2}}-\frac{1}{m^{2}}\right)\) (m = 3, 4, 5, …. ∞)

(3) Paschen, Brackett and Pfiind series : These three series in the infrared region of the spectrum arise due to the transitions to n = 3, 4 and 5, respectively from m=n + 1, n + 2, etc. The wavelengths (λ) of the lines are given by

In each series, the smallest quantum of radiation (smallest frequency, longest wavelength) arises from the transition from m = n +1 to n, and the largest quantum (highest frequency, shortest wavelength — short wavelength limit or series limit) is for the transition from m = ∞ to n.
[Notes :
(1) The lines in the Lyman and Balmer series, beginning with the longest wavelength, are labelled with the Greek letters α, β, γ, δ, ε, …. Thus, the lines in the Lyman series are called L_α, L_β, L_γ, L_δ … lines while those in the Balmer series are called H_α, H_β, H_γ, H_δ, … lines. Thus, the L_α, line has a wavelength λ, where

(2) The first four prominent hydrogen lines (H_α, H_β, H_γ, H_δ) lie in the visible region and were discovered in the solar spectrum by Anders Angstrom (the first three in 1862 and the fourth in 1871); he also measured their wavelengths to high accuracy.

Johann Jakob Balmer (1825 – 98), Swiss mathema-tician, discovered in 1884 that their wavelengths fitted
the relation λ = \(\frac{B m^{2}}{m^{2}-4}\), where m has integral values 3, 4, 5 and 6 for successive lines and B here is a constant equal to 3645.6 Å. This is Balmer’s formula; originally empirical, it pointed to the need to find an explanation. This led through Rydberg’s work to Bohr’s theory.

(3) Lyman series was discovered between 1906-14, Paschen series in 1908, Brackett series in 1922 and Pfiind series in 1924. A sixth, and the last of the named series in the hydrogen spectrum, is the Humphreys series which results from transitions to n = 6 from m = 7, 8, 9, … etc. The Paschen series lies in the near-infrared region while Pfiind and Humphreys series lie in the far-infrared region.

Further series are unnamed but follow the same pattern. Their lines are increasingly faint showing that they correspond to increasingly rare atomic events.]

Question 23.
Obtain the expressions for longest and shortest wavelengths of spectral lines in ultraviolet region for hydrogen atom.
Answer:
For hydrogen,

where R is the Rydberg constant, and m and n are the principal quantum numbers of the initial and final energy states. The Lyman series in the far UV region of the spectrum arises due to the transitions of the electron to n = 1 from,m = 2, 3,4, …, etc.
For the longest wavelength λ_Lα in the Lyman series, m = 2.

Question 24.
How many spectral series are possible in the hydrogen spectrum?
Answer:
Infinite. The last (sixth) of the named series in the hydrogen spectrum is the Humphreys series which results from transitions to n_f = 6 from n_i = 7, 8, 9, … etc. in the far-infrared region. Further series are unnamed but follow the same pattern. Their lines are increasingly faint showing that they correspond to increasingly rare atomic events.
[Note : There are infinite number of lines in each series. The spacing between adjacent lines decreases with decreasing wavelength, converging to the so-called series limit or short-wavelength limit.]

Question 25.
The energy of the electron in the first Bohr orbit in the hydrogen atom is -13.6 eV. What is its energy in the second and third Bohr orbit?
Answer:

Question 26.
The potential energy of the electron in the first Bohr orbit in the hydrogen atom is -27.2 eV. What is its kinetic energy and binding energy in the same orbit?
Answer:
Kinetic energy = –\(\frac{\text { potential energy }}{2}=-\frac{27.2}{2}\)
= 13.6 eV and
binding energy = – total energy – (potential energy + kinetic energy)
= – (-27.2 + 13.6) = 13.6 eV

Question 27.
Obtain the ratio of the longest wavelength of spectral line in the Paschen series to the longest wavelength of spectral line in the Brackett series.
Answer:
The Bohr formula for the wave number of a spectral line in the hydrogen spectrum is
\(\bar{v}=\frac{1}{\lambda}=R\left(\frac{1}{n^{2}}-\frac{1}{m^{2}}\right)\)
where is the wavelength of the emitted radiation when the electron jumps from a higher energy state of principal quantum number m to a lower energy state of principal quantum number n. R is the Rydberg constant.

The Paschen series and Brackett series of spectral lines arise due to the transitions to n = 3 and m = 4, respectively. The longest wavelength lines (λ_Pα and λ_Bx) in these series arise due to the transitions from m =4 and m = 5, respectively.

Question 28.
Obtain the ratio of the wavelength of the H_α line to the wavelength of the H_γ. line in the Balmer series.
Ans.
The Bohr formula for the wave number of a spectral line in the hydrogen spectrum is
\(\bar{v}=\frac{1}{\lambda}=R\left(\frac{1}{n^{2}}-\frac{1}{m^{2}}\right)\)
where λ is the wavelength of the emitted radiation when the electron jumps from a higher energy state of principal quantum number m to a lower energy state of principal quantum number n. R is the Rydberg constant.

The Balmer series of spectral lines arises due to the transitions to n = 2. The H_α and H_γ lines in this series arise due to the transitions from m = 3 and 5, respectively.

Question 29.
On the basis of the de Broglie hypothesis, obtain Bohr’s condition of quantization of angular momentum.
Answer:
By the de Broglie equation, the wavelength associated with an electron having momentum p = mv is
λ = \(\frac{h}{p}=\frac{h}{m v}\) ……….. (1)
In a hydrogen atom in its ground state, the de Broglie wavelength associated with the electron is the same as the circumference of the first Bohr orbit. Therefore, the electron orbit in a hydrogen atom in its ground state corresponds to one complete electron wave joined on itself.

Thus, a stationary orbit can be intepreted as one which can accommodate an integral number of de Broglie wavelength so that the associated matter wave will be in phase with itself and constructive interference will allow a standing wave along the orbit.

Therefore, for a stationary Bohr orbit of circumference 2πr,
2πr = nλ
where n is a positive integer.
∴ 2πr = \(\frac{n h}{m v}\) …………. [From Eqn (1)]
∴ Angular momentum, L = mvr = n(\(\frac{h}{2 \pi}\))
which is just the Bohr condition of angular momentum quantization for stable or allowed orbits.

30. Solve the following :
Data: e = 1.6 × 10^-19 C, m_e = 9.1 × 10^-31 kg, h = 6.63 × 10^-34 J∙s, c = 3 × 10⁸ m/s. I eV = 1.6 × 10^-19 J, ε₀ = 8.85 × 10^-12 F/m, R = 1.097 × 10⁷ m 1, 1/4πε₀ = 9 × 10⁹ N∙m²/C²

Question 1.
The radius of the first orbit of the electron in a hydrogen atom is 0.53 Å. Find the centripetal force acting on the electron.
Solution:
Data : r = 5.3 × 10^-11 m
The centripetal force on the electron = the electrostatic force between the proton and electron

Question 2.
Calculate the angular momentum of the electron in the third Bohr orbit of hydrogen atom.
Solution:
Data: n = 3
The angular momentum, L = \(\frac{n h}{2 \pi}\)
= \(\frac{3\left(6.63 \times 10^{-34}\right)}{2(3.142)}\) = 3.165 × 10^-34 kg.m²/s

Question 3.
Calculate the potential energy of the electron in the second Bohr orbit of hydrogen atom in electron volt. The radius of the Bohr orbit is 2.12 Å.
Answer:
Data : r = 2.12 × 10^-10 m
The potential energy of the electron

Question 4.
Calculate the radius of the first Bohr orbit in the hydrogen atom. [ε₀ = 8.85 × 10^-12 C²/N∙m² e = 1.6 × 10^-19 C, m(electron) = 9.1 × 10^-31 kg, h = 6.63 × 10^-34 J∙s]
Solution:
Data : ε₀ = 8.85 × 10^-12 C²/N∙m² e = 1.6 × 10^-19 C,
h = 6.63 × 10^-34 J∙s, m = 9.1 × 10^-31 kg
The radius of a Bohr orbit,

This is the radius of the third Bohr orbit in a hydrogen atom.

Question 5.
Find the ratio of the diameter of the first Bohr orbit to that of the fourth Bohr orbit in a hydrogen atom.
Solution:
The radius of a Bohr orbit,

Question 6.
Calculate the frequency of revolution of the electron in the second Bohr orbit of the hydrogen atom. The radius of the orbit is 2.14 Å and the speed of the electron in the orbit is 1.09 × 10⁶ m/s.
Solution:
Data: r = 2.14Å = 2.14 × 10^-10 m, v = 1.09 × 10⁶ m/s
v = ωr = (2πf)r
∴ The frequency of revolution of the electron in the second Bohr orbit,

Question 7.
The speed of the electron in the first Bohr orbit (in H atom) of radius 0.5 Å is 2.3 × 10⁶ m/s. Calculate the period of revolution of the electron in this orbit.
Solution:
Data: r = 0.5 Å = 5 × 10¹¹ m, v = 2.3 x 10⁶ m/s
Period of revolution of the electron,

Question 8.
The energy of the electron in the ground state of the hydrogen atom is -13.6 eV. Find its KE and PE in the same state.
Solution:

Question 9.
An electron is orbiting in the 3rd Bohr orbit in H atom. Calculate the corresponding ionization energy, if the ground state energy is – 13.6 eV.
Solution:
Data : E₁ = -13.6 eV, n₁ = 1, n₃ = 3, E_∞ = 0 eV
The energy of the electron in the nth Bohr orbit,

Question 10.
Find the energy of the electron in the fifth Bohr orbit of the hydrogen atom. [Energy of the electron in the first Bohr orbit -13.6 eVI
Solution:
Data : E₁ = -13.6 eV

Question 11.
Calculate the energy of the electron in the ground state of the hydrogen atom. Express it in joule and in eV.
[m_electron = 9.11 × 10^-31 kg, e = 1.6 × 10^-19 C, h = 6.63 × 10^-34 J∙s, ε₀ = 8.85 × 10^-12 C²/N∙m²]
(3 marks)
Solution:
Data: m = 9.11 × 10^-31 kg, e = 1.6 × 10^-19 C, h = 6.63 × 10^-34 J∙s, ε₀ = 8.85 × 10^-12 C²/N∙m²
The energy of electron in nth Bohr orbit is

Question 12.
The energy of the electron in an excited hydrogen atom is – 0.85 eV. Find the corresponding angular momentum of the electron.
[h = 6.63 × 10^-34 J∙s, π = 3.142, E₁ = -13.6 eV]
Solution :
Data : E_n = – 0.85 eV, h = 6.63 × 10^-34 J∙ s, π = 3.142, E₁ = -13.6 eV

Question 13.
A photon of energy 12.75 eV is absorbed by an electron in the ground state of a hydrogen atom and raises it to an excited state. Find the quantum number of this state.
Solution:
Data : hv = 12.75 eV
The energy of the electron in the ground state of the hydrogen atom, E₁ = -13.6 eV
On absorbing a photon of energy hv = 12.75 eV,
its final energy state is
E₁ = E_i + hv = – 13.6.+ 12.75 = -0.85 eV
E_n = \(-\frac{13.6}{n^{2}}\) eV
∴ E_f = -0.85 = \(-\frac{13.6}{n^{2}}\)
∴ n = \(\sqrt{\frac{13.6}{0.85}}\) = 4
∴ The principal quantum number of the final state of the electron = 4

Question 14.
Determine the linear momentum of the electron in the second Bohr orbit in a hydrogen atom. Hence determine the linear momentum in the third Bohr orbit.
Solution:
The linear speed of the electron in the nth Bohr orbit,
v_n = \(\frac{e^{2}}{2 \varepsilon_{0} n h}\)
∴ The linear momentum of the electron in the second orbit,

Question 15.
A quantum of monochromatic radiation of wavelength λ is incident on an hydrogen atom that takes it from the ground state to the n = 3 state. Find λ and the frequency of the radiation.
[E₁ = -13.6 eV, E₃ = -1.51 eV]
Solution :
Data : n_i = 3, n_f = 1, E₁ = -13.6 eV, E₃ = 1.51 eV, h = 6.63 × 10^-34 J∙s, e = 1.602 × 10^-19 C, c = 3 × 10⁸ m/s
The energy of the incident radiation,
hv = E₃ – E₁
∴ The frequency of the incident radiation,

Question 16.
A hydrogen atom undergoes a transition from a state with n = 4 to a state with n = 1. Calculate the change in the angular momentum of the electron and the wavelength of the emitted radiation.
[h = 6.63 × 10^-34 J∙s, R = 1.097 × 10⁷ m^-1]
Solution :
Data : h = 6.63 × 10^-34 J∙s, R = 1.097 × 10⁷ m^-1
(i) The angular momentum of the electron in the nth orbit of the hydrogen atom is
L_n = \(\frac{n h}{2 \pi}\)
The change in the angular momentum when the electron jumps from the 4th orbit to the 1st orbit is

Question 17.
Find the Rydberg constant given the energy of the electron in the second orbit in hydrogen atom is -3.4 eV.
Solution:
Data: E₂ = -3.4 eV = -3.4 × 1.6 × 10^-19 J, h = 6.63 × 10^-34 J∙s, c = 3 × 10⁸ m/s

Question 18.
Find the energy of the electron in eV in the third Bohr orbit of the hydrogen atom.
[R = 1.097 × 10⁷ m ^-1, c = 3 × 10⁸ m/s, h = 6.63 × 10^-19 J∙s, e = 1.6 × 10^-19 C]
Solution:
Data : n = 3, R = 1.097 × 10⁷ m ^-1, c = 3 × 10⁸ m/s, h = 6.63 × 10^-19 J∙s, e = 1.6 × 10^-19 C

Question 19.
Calculate the wavelength of the first two lines of the Balmer series in the hydrogen spectrum.
Solution :

∴ The wavelength of the second Balmer line,
λ_Hβ = \(\frac{16}{3.291}\) × 10^-7 = 4.862 × 10^-7 m = 4862 Å

Question 20.
The wavelength of H line of the Balmer series is 4860Å. Calculate the wavelength of the Balmer H_x line.
Solution:
Data : λ_β = 4860 Å

Question 21.
The short wavelength limit of the Lyman series is 911.3 Å. Compute the short wavelength limit of the Balmer series.
Solution:
Data : λ_∞L = 911.3 Å
The wavelengths of the lines in the Lyman series are given by
\(\frac{1}{\lambda}=R\left(\frac{1}{1^{2}}-\frac{1}{m^{2}}\right)\) (m = 2, 3, 4, ……..)
For the shortest wavelength line, m = ∞. Therefore, the short wavelength limit of the Lyman series is given by

Question 22.
The second member of the Balmer series for the hydrogen atom has wavelength 4860 Å. Calculate Rydberg’s constant.
Solution:

Question 23.
Find the shortest wavelength of the Paschen series, given that the longest wavelength of the Balmer series in the hydrogen spectrum is 6563 Å.
Solution:
Let λ_Bα and λ_P∞ be the wavelength of the first line, i.e., the longest wavelength line, of the Balmer series and the shortest wavelength of the Paschen series, respectively.
Data : λ_Bα = 6560 Å

Question 24.
Find the longest wavelength in the Paschen series. [R = 1.097 × 10⁷ m^-1]
Solution:
Data: R = 1.097 × 10⁷ m^-1
\(\frac{1}{\lambda}=R\left(\frac{1}{n^{2}}-\frac{1}{m^{2}}\right)\)
For the longest wavelength line in the Paschen series, m = 4 and n = 3.

Question 25.
Find the ratio of the longest to shortest wavelengths in the Paschen series.
Solution:

Question 26.
Find the ratio of the longest wavelength in the Paschen series to the shortest wavelength in the Balmer series.
Solution:

Question 27.
Compute the shortest and the longest wavelength in the Lyman series of hydrogen atom.
Solution:

Question 28.
The wavelength of the first line of the Balmer series is 6563 Å. Calculate the wavelength of the first line of (i) the Lyman series (ii) the Paschen series.
Solution:

∴ The wavelength of the first line of the Lyman series,

Question 31.
Name the constituents of an atomic nucleus. What is a nucleon?
Answer:
The constituents of an atomic nucleus are (1) the proton, a positively charged particle (2) the neutron, a neutral (uncharged) particle. The term nucleon (nuclear constituent) refers to a proton as well as a neutron.

[Note : The electron was discovered by J. J. Thomson in 1897. The proton was discovered by Ernest Rutherford in 1919. The neutron was discovered in 1932 by James Chadwick (1891-1974), British physicist. The existence of the neutron and the deuteron was predicted by Rutherford in 1920. The proton has a mass about 1836 times that of the electron, but the magnitude of the electric charge is the same for both. The mass of the neutron is slightly more than that of the proton.]

Question 32.
Define (i) atomic number (ii) mass number. Give their symbols.
Answer:

1. The number of protons in the nucleus of an atom of an element is called the atomic number of the element. It is also known as the proton number.
   It is denoted by Z.
2. The number of nucleons (protons and neutrons) in the nucleus of an atom is called the mass number or the atomic mass number. It is denoted by A.

[Notes : (i) The number of neutrons in the nucleus of an atom is known as the neutron number, denoted by N. It is usually greater than Z, with the exceptions of helium (2 protons, 2 neutrons) and hydrogen (1 proton, no neutron) (ii) A = Z + N.]

Question 33.
Write the atomic symbol for an element giving the atomic number and mass number. Give two examples. Which of the two numbers is characteristic of the element? Why?
Answer:
An atom is represented as \(\frac{A}{Z}\)X, where X is the chemical symbol for the element, Z is the atomic number and A is the mass number.
Examples : Fluorine, \(\begin{aligned}
&19 \\
&9
\end{aligned}\)F; Phosphorus, \(\begin{aligned}
&31 \\
&15
\end{aligned}\)P; Gold, \(\begin{aligned}
&197 \\
&79
\end{aligned}\)Au.

The atomic number of an element, which is the number of protons in the nucleus of an atom of the element, is characteristic of the element. It equals the number of electrons in the atom and hence determines the chemical properties of the element and its place in the modern periodic table.

Question 34.
What are isotopes? Give an example.
Answer:
Atoms of an element having the same atomic number (Z) but different mass numbers (A) are called the isotopes of that element.

Isotopes of an element have different neutron numbers but the same chemical properties.

Example : Hydrogen has three isotopes, namely, hydrogen \( (\begin{aligned}
&1 \\
&1
\end{aligned}\) H), deuterium \( (\begin{aligned}
&2 \\
&1
\end{aligned}\) D) and tritium \( (\begin{aligned}
&3 \\
&1
\end{aligned}\) T). Deuterium and tritium have one and two neutrons in their nuclei, respectively, in addition to the single proton (Z = 1).

Question 35.
What are isobars? Give an example.
Answer:
Atoms of different elements that have the same mass number (A) but different atomic numbers (Z) are called isobars.

Although isobars have the same mass number, they are different elements because the chemical nature of an element is determined by its atomic number. Isobars have different neutron numbers.

Example : \(\begin{array}{r}
13 \\
6
\end{array}\)C and \(\begin{array}{r}
13 \\
7
\end{array}\)N are isobars. They have
the same mass number, A (viz., 13), but their different proton numbers, Z (6 and 7) make them different elements.

Question 36.
What are isotones? Give an example.
Answer:
Atoms of different elements that have the same neutron number (N) but different atomic numbers (Z) are called isotones.

Although isotones have the same neutron number, they are different elements because the chemical nature of an element is determined by its atomic number.

Question 37.
What is unified atomic mass unit? Express it in J/c² and MeV/c².
Answer:
The unified atomic mass unit is an accepted but non-SI unit of mass. It is defined to be equal to \(\frac{1}{12}\) of the mass of a free atom of the isotope of carbon with mass number 12 which is at rest and in its ground state. It is denoted by u.

Its value in SI unit is obtained experimentally. 1 u = 1.660538782 (83) × 10^-27 kg, with the standard uncertainty in the last two digits given in v parenthesis.
Taking, 1 u = 1.660538782 × 10^-27 kg
c = 2.99792458 × 10⁸ m/s,
e = 1.602176462 × 10^-19 C and
using the relation E = me² { ≡ m ≡ E / c²),
we get, 1 u = 1.49241783 × 10^-10 J/c²
≅ 1.492 × 10^-10 J/c²
and 1 u = 931.494042 MeV / c²
≅ 931.5 MeV/c²

Question 38.
How is the nuclear size determined ? State the relation between nuclear size (radius) and mass number.
Answer:
The nuclear size is determined from particle scattering experiments using fast electrons or neutrons. The de Broglie wavelength of the bombarding electrons or neutrons should be less than the radius of the nucleus under study.

It is found that the volume of a nucleus is directly proportional to the mass number A, i.e., to the number of nucleons in the nucleus. For many purposes, nuclei may be assumed to be spherical.
Thus, a nucleus of radius R has a volume \(\frac{4}{3}\) πR³
∴ R³ ∝ A or R ∝ A^{\(\frac{1}{3}\)}
∴ R = R₀A^{\(\frac{1}{3}\)}
where R₀ ≈ 1.2 × 10^-15 m = 1.2 fm.
∴ R ≈ 1.2 AA^{\(\frac{1}{3}\)} fm

[Note : A nucleus does not have a sharp boundary. Also, electron scattering and neutron scattering yield slightly different values of R₀. Hence, the relation above is only representative of effective nuclear size. 1 femtometre or 1 fm = 10^-15 m; an earlier non-SI unit of the same value called fermi, in honour of Enrico Fermi (1901-54), Italian-US nuclear physicist, is no longer accepted in SI.]

Question 39.
Nuclear density is essentially the same for all nuclei. Justify.
Given 1 u = 1.66 × 10^-27 kg and R₀ ≈ 1.2 fm, estimate the nuclear density.
Answer:
Relative atomic mass rounded to the nearest integral value equals the atomic mass number A. Thus, ignoring the masses of the atomic electrons and binding energies, nuclear mass expressed in unified atomic mass unit = A u. Also, it is experimentally found that the volume of a nucleus is directly proportional to the mass number A. As both nuclear mass and volume are proportional to the mass number, nuclear density is essentially the same for all nuclei.

The order of nuclear density is 10¹⁷ kg/m³.

[Note : Some stars, with masses between 1.4\(M_{\odot}\) and 3\(M_{\odot}\), where \(M_{\odot}\) denotes the mass of our Sun, undergo supernova at the end of their active life and collapse into neutron stars of densities comparable with nuclear v density.]

Question 40.
Define mass defect and state an expression for it.
Answer:
The difference between the sum of the masses of all the individual nucleons in a nucleus and the mass of the nucleus is known as the mass defect.

Mass defect, ∆m = (Zm_p + Nm_n) – M where mp is the proton mass, mn is the neutron mass, M is the mass of the nucleus, Z is the atomic number and N = A – Z is the neutron number.

Question 41.
Explain the term nuclear binding energy and express it in terms of mass defect. What is binding energy per nucleon? Write the expression for it.
Answer:
In the atomic nucleus, the protons and the neutrons are bound together by a strong, short range and charge independent, attractive force called the nuclear force. It is necessary to supply energy to break the nucleus. The minimum energy required to separate a nucleus into its free constituents, i.e., protons and neutrons, is known as the nuclear binding energy. It is the mass energy of the nucleons minus the mass energy of the nucleus.

The mass defect of a nucleus, of mass M, mass number A, proton number Z and neutron number N = A – Z, is
∆m = (Zm_p + Nm_n) – M
where, mp is the proton mass and mn is the neutron mass. Then, from Einstein’s mass-energy relation,
nuclear binding energy = ∆mc²
= [(Zm_p -(- Nm_n) – M] c² (in joule)
= [(Zm_p + Nm_n) – M] \(\frac{c^{2}}{e}\) (in eV)

where c is the speed of light in free space and e is the elementary charge. In calculations using the above equations, mp is replaced by mH (mass of hydrogen atom). M is taken to be the atomic mass and not nuclear mass because the electron masses cancel out and the difference in electronic binding energies can be ignored as these are 106 times smaller than the nuclear binding energy.
∴ Nuclear binding energy
≅ [(Zm_H + Nm_n) – M_atom] c² (in joule)
The minimum energy required on the average to separate a nucleon from a given nucleus is called the binding energy per nucleon for that nucleus. It is the nuclear binding energy for a nucleus divided by its mass number.
∴ Binding energy per nucleon = \(\frac{\Delta m c^{2}}{A}\)
= [(Zm_p + Nm_n) – M] \(\frac{c^{2}}{A}\)
≅ [(Zm_H + Nm_n) – M_atom] \(\frac{c^{2}}{A}\)

Question 42.
What is the significance of binding energy per nucleon?
Answer:
The greater the binding energy per nucleon in a nucleus, the greater is the minimum energy needed to remove a nucleon from the nucleus. Thus, binding energy per nucleon indicates the stability of a nucleus.

[Note : The binding energy per nucleon is high when both Z and N are even numbers, and such nuclei are most common. Nuclei with both Z and N odd are very rare.]

Question 43.
What are stable nuclei? What decides nuclear stability? What are the properties of nuclear force?
Answer:
Those nuclei which for certain combinations of neutrons and protons do not spontaneously disintegrate are called stable nuclei. There are two aspects that decide the stability of a nucleus. Firstly, the existence of nuclear energy levels implies certain configurations to achieve potential energy minimum, and secondly, the balance of forces.

Just like energy levels in atoms, nuclear energy levels are filled in sequence obeying the exclusion principle. Thus, there is a tendency for N to equal Z, or to have both even Z and even N.
Properties of the nuclear force :
(1) The nucleons in a nucleus are held .together by the attractive strong nuclear force. This force is much stronger than gravitational force and electromagnetic force.

(2) Nucleons interact strongly only with their nearest neighbours because the nuclear force has an extremely short range. Gravitational force and electromagnetic force are long range forces. They tend to zero only when the separation between two particles tends to infinity.

(3) Inside a nucleus, this force appears to be the same between two protons, a proton and a neutron, and two neutrons. However, between two protons there is also Coulomb repulsion which has a much longer range and, therefore, has appreciable magnitude throughout the entire nucleus. In nuclei having 2 ≤ Z ≤ 83, with neutrons present, the nuclear force is strong enough to overcome the Coulomb repulsion.

For light nuclei (A < 20), N ≥ Z, but is never smaller (except in \(\begin{aligned} &1 \\ &1 \end{aligned}\)H and \(\begin{aligned} &3 \\ &2 \end{aligned}\)H). However, with more than about 10 protons, an excess of neutrons is required to form a stable nucleus; for high atomic numbers, N/Z = 1.6. For Z > 83, even an excess of neutrons cannot prevent spontaneous disintegration and there are no stable nuclei.
[Note : The strength of the nuclear force is evident from the nuclear binding energy.]

Question 44.
Draw a neat labelled graph showing the variation of binding energy per nucleon as a function of mass number. What can we infer from the
graph?
Answer:
Figure shows the plot of the binding energy per nucleon (BE/A, in MeV per nucleon) against mass number A.

From Figure, we can draw the following inferences :
(1) The greater the binding energy per nucleon, the more stable is the nucleus because greater is the minimum energy needed to remove a nucleon. Thus, the nuclei appearing high on the plot are more tightly bound.

(2) BE/A has a maximum value of about 8.8 MeV per nucleon at A = 56 (⁵⁶Fe nuclide) and then decreases to 7.6 MeV per nucleon at A = 238 (²³⁸U nuclide). Thus, the iron nuclide ⁵⁶Fe has the maximum binding energy per nucleon and is the most stable nuclide. Also, the peak at A = 4 shows that the 4He nucleus (α particle) has higher binding energy per nucleon compared to its neighbours in the periodic table and is exceptionally stable.

(3) The increase in BE /A as A decreases from 240 to 60 shows that if a heavy nucleus splits into two medium-sized fragments, each of the new nuclei will have more BE / A than the original nucleus. The binding energy difference, which can be very large, will then be released. The process of splitting a heavy nucleus is called nuclear fission. The energy released in a fission of ²³⁵U nucleus is about 200 MeV.

(4) Joining together, or fusing, two very light nuclei to form a single nucleus will also lead to larger BE/A in the new heavier nucleus. Again, the binding energy difference will be released. This process,which is called nuclear fusion, is also a very effective way of obtaining energy.

45. Solve the following :
Question 1.
Find the nuclear radii of ²⁰⁶Pb and ²⁰⁸Pb.
Solution:
Data : R₀ = 1.2 × 10^-15 m
Nuclear radius, R=R₀A^{\(\frac{1}{3}\)}
(i) For ²⁰⁶Pb, A = 206
∴ R = (1.2 × 10^-15) (206)^{\(\frac{1}{3}\)}
= 1.2 × 10^-15 × 5.906
= 7.087 × 10^-15 m = 7.087 fm

(ii) For ²⁰⁸ Pb, A = 208
∴ R = (1.2 × 10^-15)(208)^{\(\frac{1}{3}\)}
= 1.2 × 10^-15 × 5.925
= 7.11 × 10^-15 m = 7.11 fm

Question 2.
Given the atomic mass of the isotope of iron 56Fe is 55.93 u, find its nuclear density.
Solution :
Data : A = 56, m = 55.93 u = 55.93 × 1.66 × 10^-27 kg, R₀ = 1.2 × 10^-15 m .

Question 3.
Given the nuclear radius of ¹⁶O is 3.024 fm, find that of ²³⁵U.
Solution:
Data : A₁ = 16 and R₁ = 3.024 fm (for ¹⁶O),
A₂ = 235 (for ²³⁵U)

Question 4.
The mass defect of He nucleus is 0.0304 u. Calculate its binding energy.
Solution:
Data: ∆m = 0.0304 u, 1 u = 931.5 \(\frac{\mathrm{MeV}}{c^{2}}\)
The binding energy of He nucleus
= ∆mc²
= 0.0304 × 931.5
= 28.32 MeV

Question 5.
Calculate the mass defect and binding energy of \(\begin{aligned}
&59 \\
&27
\end{aligned}\) Co which has a nucleus of mass 58.933 u. [Take m^p = 1.0078 u, mⁿ = 1.0087 u]
Solution:
Data: m^p = 1.0078 u, mⁿ = 1.0087 u, m^Co = 58.933 u,
1 u = 931.5 \(\frac{\mathrm{MeV}}{c^{2}}\)
For \(\begin{aligned}
&59 \\
&27
\end{aligned}\)Co, A = 59, Z = 27
∴ N = A – Z = 59 – 27 = 32
The mass defect,
∆m=(Zm_p + N_n) – m_Co
= (27 × 1.0078 + 32 × 1.0087) – 58.933
= (27.2106 + 32.2784) – 58.933
= 59.4890 – 58.933 = 0.556 u
∴ The binding energy
= ∆mc²
= 0.556 uc² × 931.5 \(\frac{\mathrm{MeV}}{uc^{2}}\) = 517.8 MeV

Question 6.
Find the mass energy of a particle of mass 1 u in joule and electronvolt.
Solution:
Data : m = 1 u = 1.66 × 10^-27 kg, c = 3 × 10⁸ m/s,
1 eV = 1.602 × 10^-19 J
The mass energy,
E = mc²
= (1.66 × 10^-27) (3 × 10⁸)⁸
= 1.66 × 9 × 10^-11 n
= 1.494 × 10^-10 J
= \(\frac{1.494 \times 10^{-10}}{1.602 \times 10^{-19}}\) eV
= 9.326 × 10⁸ eV or 932.6 MeV
[Note : The answer differs from the accepted value of about 931.5 MeV because of the rounding off errors in the data used.]

Question 7.
Find the mass energy of a proton at rest in MeV. [m_p = 1.673 × 10^-27kg]
Solution:
Data : m_p = 1.673 × 10^-27 kg, c = 3 × 10⁸ m/s,
1 eV = 1.602 × 10^-19 J
∴ 1 MeV = 10⁶ × 1.602 × 10^-19 J = 1.602 × 10^-13 J
The mass energy of a proton at rest,

Question 8.
Find the mass energy of a particle of mass 1 g in joule.
Solution:
Data : m = 1 g = 1 × 10^-3 kg, c = 3 × 10⁸ m/s,
1 eV = 1.602 × 10^-19 J
The mass energy,
E = mc²
= (1 × 10^-3)(3 × 10⁸)²
= 9 × 10¹³ J

Question 46.
What is radioactivity? OR Define radioactivity.
Answer:
Radioactivity is the phenomenon in which unstable nuclei of an element spontaneously distintegrate into nuclei of another element by emitting a or β particles accompanied by γ-rays. Such transformation is known as radioactive transformation or radioactive decay.

Question 47.
Who discovered radioactivity? Give a brief account of the first observation of radioactivity.
Answer:
Antoine Henri Becquerel (1852-1908), French physicist, discovered radioactivity in 1896.

He had kept photographic plates wrapped in a thick black paper in a drawer of his desk. Later, he also kept uranium salts near the photographic plates. After some days he developed the photographic plates and was surprised to find that they were fogged although he had protected them from light. Becquerel concluded that uranium salts must be emitting some invisible rays which affected the photographic plates. In this way, radioactivity was discovered by Becquerel.

Question 48.
What is a radioactive element? Give two examples of radioactive elements.
Answer:
The element which exhibits the property of radioactivity, i.e., spontaneous disintegration of unstable nuclei of the element by emission of α or β particles accompanied by γ-rays is called a radioactive element. Examples : Uranium, thorium, polonium, radium, actinium.

Question 49.
Name the three radioactive decay processes. State the nature of particle/radiation emitted in each process. What is meant by the Q value or Q factor of the decay?
Answer:
The processes by which a radioactive element can decay are :
(1) α-decay, by emission of an α-particle (\(\begin{aligned}
&4 \\
&2
\end{aligned}\) He nucleus),
(2) β-decay, by emission of an electron (\(\begin{aligned}
&0 \\
&-1
\end{aligned}\)e) or a positron \(\begin{aligned}
&0 \\
&1
\end{aligned}\)e), and

(3) γ-decay, by emitting electromagnetic radiations (γ-rays) of very short wavelength of about 10^-12 m to 10^-14 m.

In a radioactive decay, the difference in the energy equivalent of the mass of the parent atom and that of the sum of the masses of the products is called the Q value or Q factor of the decay. It is also called disintegration energy.

Question 50.
State the observations which lead to the conclusion that radioactivity is a nuclear phenomenon.
Answer:
The rate of disintegration of a radioactive material is not affected by changes in physical and chemical conditions such as (1) temperature and pressure (2) action of electric and magnetic fields (3) chemical composition of the material. The above changes affect the orbital electrons, but not the nucleus. Therefore, we conclude that radioactivity is a nuclear phenomenon.

Question 51.
State the nature and properties of a-particles.
Answer:
Nature of α-particles :
(1) An alpha particle is a helium nucleus, i.e., a doubly ionized helium atom. It consists of two protons and two neutrons.
(2) Mass of the α-particle ≅ 4u
Charge on the α-particle = 2 × charge on the proton
Properties of α-particles :
(1) Of the three types of radioactive radiations, α- particles have the maximum ionizing power. It is about 100 times that of β-particles and 104 times that of γ-rays.

(2) They have the least penetrating power, about 100 times less than that of β-particles and 10⁴ times less than that of γ-rays. They can pass through very thin sheets of paper but are scattered by metal foils and mica. Since α-particles produce intense ionization in a medium, they lose their kinetic energy quickly. As a result, they do not penetrate more than a few centimetres (about 2.7 cm to 8.6 cm) in air under normal conditions.

(3) They are deflected by electric and magnetic fields since they are charged particles. Their deflection is less than that of β-particles in the same field.

(4) They affect photographic plates.
(5) They cause fluorescence in fluorescent materials such as zinc sulphide.
(6) They emerge from the nuclei with tremendous speeds in the range of \(\frac{1}{100}\)th to \(\frac{1}{10}\)th of the speed of light in free space.
(7) They destroy living cells.
[Note : α-rays and β-rays were discovered by Henri BecquereL]

Question 52.
State the nature and properties of β-particles.
Answer:
Nature of β-particles : A β-particle is an electron or a positron.
Properties of β-particles :

1. β-particles have a moderate ionizing power. It is about 100 times less than that of α-particles, but 100 times more than that of γ-rays.
2. They have a moderate penetrating power. It is about 100 times more than that of α-particles, but 100 times less than that of γ-rays.
3. They are deflected by electric and magnetic fields. Their deflection is more than the deflection of α-particles in the same field but in the opposite direction.
4. They affect photographic plates.
5. They cause fluorescence in a fluorescent material such as zinc sulphide.
6. Their energies and speeds are very high. Their speed is of the order of 10⁸ m/s. Some β-particles have speeds of the order of 0.99 c, where c is the speed of light in free space.
7. They cause more biological damage than α-particles, because they have more penetrating power.

[Note : When a nucleus emits an electron, one of its neutrons changes to a proton; the electron is accompanied by a neutral and almost massless particle called antineutrino \(\bar{v}_{\mathrm{e}}\) with which the electron shares its energy and momentum. Hence, β-particles are emitted with speeds ranging from about 0 to 0.99 c.

The properties of a positron are identical to those of an electron except that it carries a positive charge of the same magnitude as an electron. A positron emission in a β⁺ decay is accompanied by a neutrino v_e. A positron is an anti-particle of an electron, and an antineutrino is an antiparticle of neutrino.

The existence of a small neutral particle, emitted simultaneously with the electron in β^–-decay, was proposed in 1931 by Wolfgang Pauli (1900 -1958), Austrian-US theoretical physicist. It was confirmed experimentally in 1956 by Frederic Reines and Clyde Lorrain Cowan, Jr., US physicists. This neutral particle, that appears in β⁺ -decay, was called the neu-trino. It travels with a speed very close to that of light in free space. The existence of positron (in 1928) and other antiparticles was predicted by Paul Adrien Maurice Dirac (1902 – 84), British theoretical physicist. All these predictions were eventually confirmed experimentally; the positron was discovered in 1932 by Carl David Anderson, US physicist.]

Question 53.
State the nature and properties of γ-rays.
Answer:
Nature of γ-rays :

1. γ-rays are electromagnetic waves of very short wavelength (about 10^-12 m to 10^-14 m).
2. They are uncharged.

Properties of γ-rays :

1. γ-rays produce feeble ionization. Their ionizing power is 104 times less than that of a-particles and 100 times less than that of β-particles.
2. They have the maximum penetrating power. It is about 100 times that of β-particles and 10⁴ times that of α-particles.
3. They are not deflected by electric and magnetic fields as they are uncharged.
4. They affect photographic plates.
5. They cause fluorescence in a fluorescent material such as zinc sulphide.
6. Their speed in free space is 3 × 10⁸ m/s( the same as that of light waves and X-rays in free space).
7. γ-rays can be diffracted by crystals. In recent times, γ-ray diffraction has emerged as a powerful tool in structural and defect studies of crystals.
8. They destroy living cells and tissues and are used for destroying cancer cells.

[Note : γ-rays (not established clearly in Berquerel’s work) were discovered in 1900 by Paul Villard (1860-1934), French physicist.]

Question 54.
(a) What is α-decay? What is the consequence of an α-decay on a radioactive element? What is the Q value or Q factor in this case ?
Q = [m_U – m_Th – m_α]c²
(b) What is β-decay ? What is the consequence of a β-decay on a radioactive element? What is the Q value or Q factor in this case ?
Answer:
(a) A radioactive transformation in which an a-particle is emitted is called α-decay.
In an α-decay, the atomic number of the nucleus decreases by 2 and the mass number decreases by 4.
Example : \({ }_{92}^{38} \mathrm{U} \rightarrow{ }_{90}^{234} \mathrm{Th}+{ }_{2}^{4} \alpha\)
Q = [m_U – m_Th – m_α]c²

(b) A radioactive transformation in which a β-particle is emitted is called β-decay.
In a β -decay, the atomic number of the nucleus increases by 1 and the mass number remains unchanged.
Example : \({ }_{90}^{23} \mathrm{Th} \rightarrow{ }_{91}^{234} \mathrm{~Pa}+{ }_{-1}^{0} e+\bar{v}_{\mathrm{e}}\)
where \(\bar{v}_{\mathrm{e}}\) is the antineutrino emitted to conserve the momentum, energy and spin.
Q = [m_Th – m_Pa – m_e]c²
In a β⁺-decay, the atomic number of the nucleus decreases by 1 and the mass number remains unchanged.
Example : \({ }_{15}^{30} \mathrm{P} \rightarrow{ }_{14}^{30} \mathrm{Si}+{ }_{+1}^{0} e+v_{\mathrm{e}}\)
where v_e is the neutrino emitted to conserve the momentum, energy and spin.
Q = [m_P – m_Si – m_e]c²
[Note : The term fi particle refers to the electron (or positron) emitted by a nucleus.]

Question 55.
Why are α- and β-particle emissions often accompanied by γ-rays?
Answer:
A given nucleus does not emit α- and βparticles simultaneously. However, on emission of α or β-particles, most nuclei are left in an excited state. A nucleus in an excited state emits a γ-ray photon in a transition to the lower energy state. Hence, α- and β-particle emissions are often accompanied by γ-rays.

Question 56.
State the law of radioactive decay and express it in the exponential form.
OR
State the law of radioactive decay. Hence derive the relation N = N₀e^-λt, where the symbols have their usual meanings.
OR
Show that the number of nuclei of a radioactive material decreases exponentially with time.
Answer:
Law of radioactive decay : At any instant, the rate of radioactive distintegration is directly proportional to the number of nuclei of the radioactive element present at that instant.

Derivation : Let N₀ be the number of nuclei present at time t = 0, and N the number of nuclei present at time t.

where λ is a constant of proportionality called the radioactive decay constant or the distintegration constant. It is a constant for a particular radioactive element. The minus sign indicates that N decreases as t increases.
Integrating Eqn (1),

This is the exponential form of the law of radioactive decay. It shows that the number of nuclei present decreases exponentially with time.
[Note : This equation is also written in the form
N(t) = N₀e^-λt]

Question 57.
If the number of nuclei of a radioactive substance becomes \(\frac{1}{e}\) times the initial number in 10 days, what is the decay constant of the substance ?
Answer:

Question 58.
What is meant by the activity of a sample of radioactive element? State the expression for it and also the units.
Answer:
The rate of disintegration of a sample of a radioactive element is called its activity. Let A denote the activity at time t and A₀ the initial activity. Then,

The SI unit of activity is called the becquerel (Bq) in honour of Henri Becquerel. 1 Bq = 1 disintegration per second.

The earlier unit, the curie (Ci) was based on the activity of 1 gram of ²²⁶Ra. 1 Ci = 3.7 × 10¹⁰ Bqn It was named after Marie Curie, Polish-bom French chemist.
[Note : Eqn (1) is also written in the form A (t) = A₀e^-λt]

Question 59.
Define half-life a radioactive element and obtain the relation between half-life and decay constant.
Answer:
The half-life of a radioactive element is defined as the average time interval during which half of the initial number of nuclei of the element disintegrate.

Let N₀ be the number of nuclei of a radioactive element present at time t = 0 and N, the number of nuclei present at time t. From the law of radioactive decay,
N = N₀e^-λt
where λ is the decay constant of the element.
If T is the half-life of the element, then, N = \(\frac{N_{0}}{2}\) when t = T.

This is the relation between the half-life and the decay constant of a radioactive element.

[Note : Radioactive decay law is statistical in nature and applicable only when the number of nuclei in the sample under consideration is very large. λ gives the probability that a nucleus of the element will decay in one second. We cannot know the exact number of nuclei that would decay in a given time interval. Hence, the use of the term average in the definition of half-time.]

Question 60.
What is meant by average life or mean life of a radioactive species ? How is it related to the half-life?
Answer:
Let N₀ = number of nuclei present at time t = 0 and λ = decay constant of a radioactive species.
| dN | = | λNdt |. ∴ The number of nuclei decaying between time tand t + dt is λN₀e^-λtdt. The life time of these nuclei is t. The average life or mean life of a radioactive species is denoted by t and is, by definition,

Question 61.
Define decay constant or distintegration constant of a radioactive element. If λ is the decay constant of a radioactive element, show that about 37% of the original nuclei remains undecayed after a time interval of λ^-1.
Answer:
The decay constant or disintegration constant of a radioactive element is defined as the ratio of the disintegration rate at an instant to the number of undecayed nuclei of the element present at that instant.

Let N₀ be the number of nuclei of a radioactive element present at time t = 0 and N, the number of undecayed nuclei at time t. From the radioactive law,
N = N₀e^-λt
where λ is the decay constant. At t = λ^-1, the fraction of undecayed nuclei is
\(\frac{N}{N_{0}}\) = e^{-λ × λ-1} = e^-1 = \(\frac{1}{e}\)
Since e ≅ 2.718,
\(\frac{N}{N_{0}}=\frac{1}{2.718}\) = 0.3679
Therefore, about 36.79% ≈ 37% of the original nuclei remains undecayed after a time λ^-1. Since λ is the probability that a nucleus of the element will decay in one second, λ^-1 gives the mean-life or the mean life time τ of the radioactive element measured in second; τ = λ^-1.

Question 62.
Show graphically how the number of nuclei (N) of a radioactive element varies with time (t).
Answer:

Question 63.
The half-life of a radioactive material is 4 days. Find the time required for 1/4 of the initial number of radioactive nuclei of the element to remain undisintegrated.
Answer:
For t = nT, N = N₀/ 2ⁿ. In this case, n = 2.
∴ f = 2T = 2 × 4 = 8 days is the required time.

Question 64.
A radioactive sample with half-life 2 days has initial activity 32 μCi. What will be its activity after 8 days?
Answer:
Here, t = 8d and T = 2d
∴ t =4T
For t = nT, N = N₀/ 2ⁿ and activity ∝ N
∴ A = A₀/2⁴ = A₀/16 = 32 /16
= 2 μCi is the required activity.

Question 65.
In successive radioactive decay if the decrease in mass number is 32 and the decrease in atomic number is 8, how many (i) α particles (ii) β particles are emitted in the process ?
Answer:

1. Number of α-particles emitted = 32/4 = 8
2. Number of β-particles emitted = 16 – 8 = 8.

66. Solve the following :
Question 1.
The decay constant of a radioactive substance is 4.33 × 10^-4 per year. Calculate its half-life and average life.
Solution:
Data : λ = 4.33 × 10^-4 per year
The half-life period of the radioactive substance.

Question 2.
The half-life of \(\begin{array}{r}
226 \\
88
\end{array}\)Ra is 1620 y. Find its decay constant in SI unit.
Solution:
Data: T = 1620 y = 1620 × 365 × 8.64 × 10⁴ s
= 5.109 × 10¹⁰ s
The decay constant of \(\begin{array}{r}
226 \\
88
\end{array}\)Ra is
λ = \(\frac{0.693}{T}=\frac{0.693}{5.109 \times 10^{10}}\) = 1.356 × 10^-11 s^-1

Question 3.
The half-life of \(\begin{array}{r}
210 \\
84
\end{array}\)Po is 138 d. Find the time required for 75% of the initial number of radio active nuclei of \(\begin{array}{r}
210 \\
84
\end{array}\)Po to disintegrate.
Solution:
Data: T = 138 d, = \(\frac{N}{N_{0}}=\frac{1}{4}\) (since only 25% of the
initial number of nuclei remains undisintegrated)
In one half-life (t = T), \(\frac{N}{N_{0}}=\frac{1}{2}\). In two half-lives (t = 2T), \(\frac{N}{N_{0}}=\frac{1}{4}\)
∴ The time for 75% of \(\begin{array}{r}
210 \\
84
\end{array}\)Po nuclei to disintegrate is 2T = 2 × 138 = 276 d.

Question 4.
Protactinium \(\begin{array}{r}
233 \\
91
\end{array}\)Pa decays to \(\frac{1}{5}\)th of its initial quantity in 62.7 days. Calculate its decay constant, mean-life and half-life.
Solution:
Data : \(\frac{N}{N_{0}}=\frac{1}{5}\), t = 62.7d

Question 5.
The half-life of \(\begin{array}{r}
210 \\
82
\end{array}\)Pb is 2.2.3 y. How long will it take for its activity to reduce to 30% of the initial activity?
Solution:
Data : T = 22.3 y, A = 0.3 A₀
By the radioactive decay law,
N = N₀e^-λt
∴ λN = λN₀e^-λt
∴ λ = A₀e^-λt
where A₀ = AN₀ is the initial activity and A = λN is the activity at time t.

∴It will take 38.75 y for the activity of \(\begin{array}{r}
210 \\
82
\end{array}\)Pb to reduce to 30% of the initial activity.

Question 6.
Radioactive sodium \(\begin{aligned}
&24 \\
&11
\end{aligned}\)Na has half-life of 15 h. Find its decay constant and mean-life. How much of 10 g of \(\begin{aligned}
&24 \\
&11
\end{aligned}\)Na will be left after 24 h?
Answer:
Data : T = 15 h, m₀ = 10 g, t = 24 h

since mass of a sample is directly proportional to the number of atoms or nuclei present.

Question 7.
Thorium \(\begin{gathered}
232 \\
90
\end{gathered}\)Th disintegrates into lead \(\begin{gathered}
200 \\
82
\end{gathered}\)Pb. Find the number of α and β particles emitted in the disintegration.
Solution:
An α-particle is a helium nucleus with mass number 4 and atomic number 2. In an α-decay, the mass number of the disintegrating nucleus decreases by 4 and its atomic number decreases by 2.

A β^–-particle is an electron with mass number 0 and atomic number – 1. In a β^–-decay, the mass number of the disintegrating nucleus remains unchanged and its atomic number increases by 1.

Let x α-particles and y ß-particles be emitted in the disintegration of \(\begin{gathered}
232 \\
90
\end{gathered}\)Th into \(\begin{gathered}
200 \\
82
\end{gathered}\)Pb.
\({ }_{90}^{232} \mathrm{Th} \stackrel{x \alpha+y \beta^{-}}{\longrightarrow}{ }_{82}^{200} \mathrm{~Pb}\)
∴ 232 – 4x – 0(y) = 200
∴ 4x = 232 – 200 = 32
∴ x = 8
Also, 90 – 2x + 1(y) = 82
∴ 90 – 2(8) + y = 82
∴ y = 82 + 16 – 90 = 8
∴ 8 α-particles and 8 ß^–-particles are emitted in the decay series of ²³²Th to ²⁰⁰Pb.

Question 67.
What is nuclear energy?
Answer:
Energy released in a nuclear reaction such as a spontaneous or induced nuclear fission, or nuclear fusion, or in interaction of two nuclei, is called nuclear energy.

[Note : It is far greater from that released in a chemical reaction.]

Question 68.
What is a nuclear reaction? Give one example.
Answer:
A reaction between the nucleus of an atom and a bombarding particle leading to the production of a new nucleus and, in general, the ejection of one or more particles is known as a nuclear reaction.

Question 69.
What are the quantities conserved in a nuclear reaction?
Answer:
The total momentum, energy, spin, charge and number of nucleons are conserved in a nuclear reaction.

Question 70.
What is fission? Who discovered nuclear fission?
Answer:
Nuclear fission is a nuclear reaction in which a heavy nucleus of an atom, such as that of uranium, splits into two or more fragments of comparable size, either spontaneously or as a result of bombardment of a neutron on the nucleus (induced fission). It is followed by emission of two or three neutrons.

The mass of the original nucleus is more than the sum of the masses of the fragments. This mass difference is released as energy, which can be enormous as in the fission of ²³⁵U.

Nuclear fission was discovered by Lise Meitner, Otto Frisch, Otto Hahn and Fritz Strassmann in 1938.

[Notes : (1) Lise Meitner (1878-1968), Austrian- Swedish physicist and radiochemist. Otto Frisch (1904-79), Austrian-British physicist and Meitner’s nephew. Otto Hahn (1879-1968), German radiochemist. Friedrich Wilhelm “Fritz” Strassman (1902-1980), German chemist. (2) In 1934, Enrico Fermi bombarded uranium with neutrons to produce nuclear reactions leading to formation of transuranic elements (Z > 92). In the process, he had carried out nuclear fission, but he misinterpreted the results. The experiments and analysis carried out by Meitner, Hahn and Strassman, and the theoretical work by Meitner and Frisch led to the discovery of fission in 1938-39.

Frisch named the phenomenon fission. Fermi, for his work in the area of nuclear science, was awarded the 1938 Nobel Prize for physics. Hahn was awarded the 1944 Nobel Prize for chemistry; it was not shared by Meitner as her important role in the discovery of fission came to light much later. (3) The most abundant urnanium isotope ²³⁸U (abundance 99.28%) can be fissioned by neutrons with high kinetic energy (called fast neutrons), at least 1.3 MeV. ²³⁵U (abundance 0.72%) can be fissioned by thermal (or low energy) neutrons having kinetic energy about 0.025 eV. Some types of nuclear reactors require the natural uranium to be enriched to increase its ²³⁵U content to about 3%.]

Question 71.
What are the products of the fission of uranium 235 by thermal neutrons?
Answer:
The products of the fission of ²³⁵U by thermal neutrons are not unique. A variety of fission fragments are produced with mass number A ranging from about 72 to about 138, subject to the conservation of mass-energy, momentum, number of protons (Z) and number of neutrons (N). A few typical fission equations are

Question 72.
What is nuclear fusion? Give one example with an equation.
Answer:
A type of nuclear reaction in which lighter atomic nuclei (of low atomic number) fuse to form a heavier nucleus (of higher atomic number) with the’ release of enormous amount of energy is called nuclear fusion.

Very high temperatures, of about 10⁷ K to 10⁸ K, are required to carry out nuclear fusion. Hence, such a reaction is also called a thermonuclear reaction.

Example : The D-T reaction, being used in experimental fusion reactors, fuses a deuteron and a triton nuclei at temperatures of about 10⁸ K.

(2) The value of the energy released in the fusion of two deuteron nuclei and the temperature at which the reaction occurs mentioned in the textbook are probably misprints.]

Question 73.
Give one area of application of (i) nuclear fission (ii) nuclear fusion.
Answer:
(i) Nuclear fission is used in (1) a nuclear reactor as very efficient and the least-polluting source of energy to generate electricity (2) atomic bombs.

(ii) Nuclear fusion is used in (experimental) fusion reactors to generate electricity without the hazards of radioactive radiations and radioactive pollution which happens with fission reactors. Nuclear fusion reactions in the interior of stars are the source of their energy output and the means of synthesis of higher elements like carbon, nitrogen and silicon from hydrogen and helium.

Question 74.
Explain the basic exothermic reaction in stars.
Answer:
The fusion of hydrogen nuclei into helium nuclei results in release of energy. It is the basic exothermic reaction in stars.
(1) The proton-proton cycle :


The total energy released is 24.7 MeV.

Question 75.
What is a nuclear reactor?
Answer:
A nuclear reactor is a device in which a nuclear fission chain reaction is used in a controlled manner (i) to produce energy in form of heat which is then converted into electricity or (ii) to produce radioisotopes or (iii) to produce new nuclides using a suitable fissionable material such as uranium or plutonium.
In a uranium reactor, \(\begin{gathered}
235 \\
92
\end{gathered}\)U is bombarded by slow neutrons to produce \(\begin{gathered}
235 \\
92
\end{gathered}\)U which undergoes fission.

Question 76.
What is a chain reaction? How is it produced?
Answer:
A chain reaction is a self-multiplying process in which neutrons ejected in a nuclear fission strike neighbouring nuclei of fissionable material and cause more fissions.
A fission of \(\begin{gathered}
235 \\
92
\end{gathered}\)U nucleus by a thermal neutron leads to ejection of two or three neutrons (2.7 neutrons on an average) having high kinetic energy of about 2 MeV. The kinetic energy of at least one of these neutrons is lowered to about 0.025 eV by a suitable moderator and the neutron is used to cause further fission. The process continues and hence it is called a chain reaction.

Multiple Choice Questions

Question 1.
The linear momentum of an electron in a Bohr orbit of an H-atom (principal quantum number n) is proportional to
(A) \(\frac{1}{n^{2}}\)
(B) \(\frac{1}{n}\)
(C) n
(D) n².
Answer:
(B) \(\frac{1}{n}\)

Question 2.
The angular momenta of the electron in successive Bohr orbits differ by
(A) \(\frac{n h}{2 \pi}\)
(B) h
(C) \(\frac{h}{2 \pi}\)
(D) (n – 1)\(\frac{h}{2 \pi}\)
Answer:
(C) \(\frac{h}{2 \pi}\)

Question 3.
The angular momentum of the electron in the second Bohr orbit of hydrogen atom is l. Its angular moementum in the third Bohr orbit is
(A) \(\frac{2}{3}\) l
(B) \(\frac{3}{2}\) l
(C) 3l
(D) \(\frac{4}{3}\) l.
Answer:
(B) \(\frac{3}{2}\) l

Question 4.
The time taken by an electron moving with a speed of 2.18 × 10⁶ m/s to complete one revolution in the first orbit (radius 0.53 A) of hydrogen atom is
(A) 1.527 × 10^-15 s
(B) 1.527 × 10^-16 s
(C) 1.527 × 10^-17 s
(D) 1.527 × 10^-18 s.
Answer:
(B) 1.527 × 10^-16 s

Question 5.
If the electron in a hydrogen atom is raised to one of its excited energy states, the electron’s
(A) potential energy increases and kinetic energy decreases
(B) potential energy decreases and kinetic energy increases
(C) potential energy increases with no change in kinetic energy
(D) potential energy decreases but kinetic energy remains constant.
Answer:
(A) potential energy increases and kinetic energy decreases

Question 6.
The potential energy of the electron in a hydrogen atom in its ground state is
(A) – 6.8 eV
(B) – 13.6 eV
(C) – 27.2 eV
(D) 13.6 eV.
Answer:
(C) – 27.2 eV

Question 7.
The kinetic energy of the orbital electron in a hydrogen atom in the excited state corresponding to n = 2 is
(A) 3.4 eV
(B) 6.8 eV
(C) 13.6 eV
(D) 27.2 eV.
Answer:
(A) 3.4 eV

Question 8.
The ratio of the kinetic energy of an electron in a Bohr orbit to its total energy in the same orbit is
(A) -1
(B) 2
(C) \(\frac{1}{2}\)
(D) -0.5.
Answer:
(A) -1

Question 9.
The energy of an electron in the nth Bohr orbit is proportional to
(A) n²
(B) n
(C) \(\frac{1}{n}\)
(D) \(\frac{1}{n^{2}}\)
Answer:
(D) \(\frac{1}{n^{2}}\)

Question 10.
The radius of the first Bohr orbit is 0.53 Å and that of nth orbit is 212 Å. The value of n is
(A) 2
(B) 12
(C) 20
(D) 400.
Answer:
(C) 20

Question 11.
The radius and the energy of the first Bohr orbit in a hydrogen atom are r₁ and E₁. If the orbital electron makes a transition to a orbit of radius 4rv the energy of the electron changes to
(A) \(\frac{E_{1}}{4}\)
(B) \(\frac{E_{1}}{2}\)
(C) 2E₁
(D) 4E₁
Answer:
(A) \(\frac{E_{1}}{4}\)

Question 12.
A hydrogen atom in its ground state is excited to the state of energy E₃ by an electron colliding with it. The minimum energy that the colliding electron must have is
(A) 10.2 eV
(B) 12.09 eV
(C) 12.5 eV
(D) 13.6 eV.
Answer:
(B) 12.09 eV

Question 13.
The energy of the electron in a hydrogen atom is raised from a state of energy E2 to that of energy E4. In the process, its
(A) energy doubles
(B) angular momentum doubles
(C) velocity doubles
(D) linear momentum doubles.
Answer:
(B) angular momentum doubles

Question 14.
Given that R is the Rydberg constant for hydrogen, the H_α line in the hydrogen spectrum has a wavelength
(A) \(\frac{1}{6 R}\)
(B) 6R
(C) \(\frac{5 R}{36}\)
(D) \(\frac{36}{5 R}\)
Answer:
(D) \(\frac{36}{5 R}\)

Question 15.
When the electron in a hydrogen atom jumps from the second orbit to the first orbit, the wavelength of the emitted rediation is λ. When the electron jumps from the third orbit to the first orbit, the wavelength of the emitted radiation would be
(A) \(\frac{27}{32}\) λ
(B) \(\frac{32}{27}\) λ
(C) \(\frac{2}{3}\) λ
(D) \(\frac{3}{2}\) λ.
Answer:
(A) \(\frac{27}{32}\) λ

Question 16.
In hydrogen atom, the Balmer series is obtained when the electron jumps from
(A) a higher orbit to the first orbit
(B) the first orbit to a higher orbit
(C) a higher orbit to the second orbit
(D) the second orbit to a higher orbit.
Answer:
(C) a higher orbit to the second orbit

Question 17.
The nuclei having the same number of protons but different number of neutrons are called
(A) isobars
(B) α-particles
(C) isotopes
(D) γ-particles.
Answer:
(C) isotopes

Question 18.
The nuclear volume of \(\begin{aligned}
&8 \\
&4
\end{aligned}\) Be is ………. that of \(\begin{aligned}
&1 \\
&1
\end{aligned}\) H.
(A) equal to
(B) two times
(C) four times
(D) eight times.
Answer:
(D) eight times.

Question 19.
The nuclear radius of the tungsten nuclide ₇₄W is twice that of the sodium nuclide \(\begin{aligned}
&23 \\
&11
\end{aligned}\) Na. The neutron number of the tungsten nuclide is
(A) 82
(B) 100
(C) 110
(D) 184.
Answer:
(C) 110

Question 20.
When a β-particle is emitted by a nucleus, its mass number
(A) decreases
(B) remains the same
(C) increases
(D) may decrease or increase.
Answer:
(B) remains the same

Question 21.
When an α-particle is emitted by a nucleus, its mass number
(A) increases by 4
(B) decreases by 4
(C) increases by 2
(D) decreases by 2.
Answer:
(B) decreases by 4

Question 22.
When a γ-ray photon is emitted by an unstable nucleus,
(A) Z increases
(B) Z decreases
(C) A increases
(D) Z and A remain the same.
Answer:
(D) Z and A remain the same.

Question 23.
In a radioactive transformation, a change in the mass number occurs with
(A) α- or β-decay
(B) β-decay
(C) γ-decay
(D) α-decay.
Answer:
(D) α-decay.

Question 24.
The half-life of radium is 1600 y. How much of 1 μg of radium will remain undistintegrated after 8000 y?
(A) \(\frac{1}{8}\) μg
(B) \(\frac{1}{16}\) μg
(C) \(\frac{1}{32}\) μg
(D) \(\frac{1}{64}\) μg.
Answer:
(C) \(\frac{1}{32}\) μg

Question 25.
In one mean lifetime of a radioactive element, the fraction of the nuclei that has disintegrated is [e is the base of natral logarithm.]
(A) \(\frac{1}{e}\)
(B) 1 – \(\frac{1}{e}\)
(C) e
(D) e – 1.
Answer:
(B) 1 – \(\frac{1}{e}\)

Question 26.
The decay constant of a radioactive element is λ After a time 2λ^-1 of the original number of radioactive nuclei about ……………….. remains undecayed.
(A) 37%
(B) 27%
(C) 25%
(D) 13.7%
Answer:
(D) 13.7%

Question 27.
Complete the following fission reaction :

Answer:
(D) \({ }_{35}^{85} \mathrm{Br}+3{ }_{0}^{1} \mathrm{n}\)

Question 28.
The energy generated in the stars is because of
(A) radioactivity
(B) nuclear fission
(C) nuclear fusion
(D) photoelectric phenomenon.
Answer:
(C) nuclear fusion