Class 6

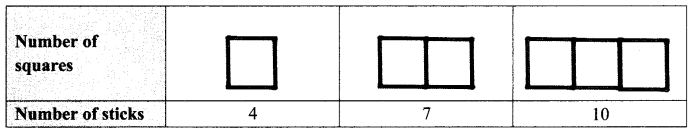
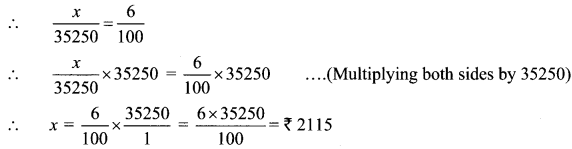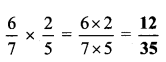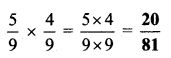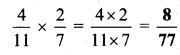Balbharti Maharashtra State Board Class 6 Maths Solutions covers the Std 6 Maths Chapter 11 Ratio-Proportion Class 6 Practice Set 29 Answers Solutions.

6th Standard Maths Practice Set 29 Answers Chapter 11 Ratio-Proportion

Question 1.
If 20 metres of cloth costs Rs 3600, find the cost of 16 m of cloth.
Solution:
Cost of 20 metres of cloth = Rs 3600
∴ Cost of 1 metre of cloth = \(\frac{\text { cost of } 20 \text { metres of cloth }}{20}=\frac{3600}{20}\)
= Rs 180
∴ Cost of 16 metres of cloth = Cost of 1 metre of a cloth × 16
= 180 x 16 = Rs 2880
∴ The cost of 16 metres of cloth is Rs 2880.

Question 2.
Find the cost of 8 kg of rice, if the cost of 10 kg is Rs 325.
Solution:
Cost of 10 kg rice = Rs 325
∴ Cost of 10 kg rice

Cost of 8 kg rice = Cost of 1 kg rice x 8

∴ The cost of 8 kg rice is Rs 260.

Question 3.
If 14 chairs cost Rs 5992, how much will have to be paid for 12 chairs?
Solution:
Cost of 14 chairs = Rs 5992
∴ Cost of 1 chairs

= Rs 428
∴ Cost of 12 chairs = Cost of 1 chair x 12
= 428 x 12 = Rs 5136
∴ The amount to be paid for 12 chairs is Rs 5136.

Question 4.
The weight of 30 boxes is 6 kg. What is the weight of 1080 such boxes?
Solution:
Weight of 30 boxes = 6 kg
∴ Weight of 1 box

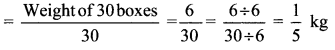
∴ Weight of 1080 boxes = Weight of 1 box x 1080

∴ The weight of 1080 boxes is 216 kg.

Question 5.
A car travelling at a uniform speed covers a distance of 165 km in 3 hours. At that same speed,
a. How long will it take to cover a distance of 330 km?
b. How far will it travel in 8 hours?
Solution:
Distance covered in 3 hours = 165 km
Distance covered in 1 hour

= 55 km
a. Time required to covered a distance of 330 km

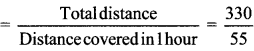
= 6 hours
∴ The time required to cover a distance of 330 km is 6 hours.

b. Distance traveled in 8 hours = Distance covered in 1 hour x 8
= 55 x 8 = 440 km
∴ The distance traveled in 8 hours is 440 km.

Question 6.
A tractor uses up 12 litres of diesel while ploughing 3 acres of land. How much diesel will be needed to plough 19 acres of land?
Solution:
Diesel required to plough 3 acres of land =12 litres
∴ Diesel required to plough 1 acre of land

= 4 liters
∴ Diesel required to plough 19 acres of land = Diesel required to plough 1 acre of land x 19
= 4 x 19 = 76 litres
∴ Diesel needed to plough 19 acres of land is 76 litres.

Question 7.
At a sugar factory, 5376 kg of sugar can be obtained from 48 tonnes of sugarcane. If Savitatai has grown 50 tonnes of sugarcanes, how much sugar will it yield?
Solution:
Sugar obtained from 48 tonnes of sugarcane = 5376 kg
∴ Sugar obtained from 48 tonnes of sugarcane

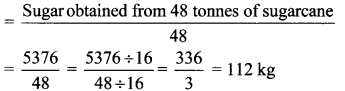
∴ Sugar obtained from 50 tonnes of sugarcane = Sugar obtained from 1 tonne of sugarcane x 50
= 112 x 50 = 5600 kg
∴ 50 tonnes of sugarcane will yield 5600 kg of sugar.

Question 8.
In an orchard, there are 128 mango trees in 8 rows. If all the rows have an equal number of trees, how many trees would there be in 13 rows?
Solution:
Number of mango trees in 8 rows =128
Number of mango trees in 1 row

∴ Number of mango trees in 13 rows = Number of mango trees in 1 row x 13
= 16 x 13 = 208
∴ The number of mango trees in 13 rows are 208.

Question 9.
A pond in a field holds 120000 litres of water. It costs Rs 18000 to make such a pond. How many ponds will be required to store 480000 litres of water, and what would be the expense?
Solution:
Capacity of 1 pond = 1,20,000 litres
Total quantity of water = 4,80,000 litres
∴ Number of ponds required

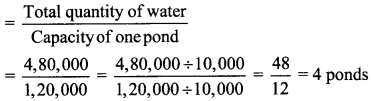
Amount required to make 1 pond = Rs 18,000
∴ Amount required to make 4 ponds = Amount required to make 1 pond x 4
= 18,000 x 4 = Rs 72,000
∴ The number of ponds required to store 4,80,000 litres of water is 4, and the expense incurred in making the ponds is Rs 72,000.

Maharashtra Board Class 6 Maths Chapter 11 Ratio-Proportion Practice Set 29 Intext Questions and Activities

Question 1.
Vijaya wanted to gift pens to seven of her friends on her birthday. When she went to a shop to buy them, the shopkeeper told her the rate for a dozen pens.
i. Can you help Vijaya to find the cost of 7 pens?
ii. If you find the cost of one pen, you can also find the cost of 7, right? (Textbook pg. no. 59)

Solution:
Cost of 12 pens = Rs 84.
∴ Cost of 12 pens

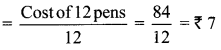
∴ Cost of 7 pens = Cost of one pen x Number of pens = 7 × 7
∴ Cost of 7 pens = Rs 49
∴ The cost of 7 pens (Rs 49) can be found by unitary method.

Balbharti Maharashtra State Board Class 6 Maths Solutions covers the Std 6 Maths Chapter 10 Equations Class 6 Practice Set 26 Answers Solutions.

6th Standard Maths Practice Set 26 Answers Chapter 10 Equations

Question 1.
Different mathematical operations are given in the two rows below. Find out the number you get in each operation and make equations.

1. 1. 16 ÷ 2,
   2. 5 × 2,
   3. 9 + 4,
   4. 72 ÷ 3,
   5. 4 + 5,

1. 8 × 3,
2. 19 – 10,
3. 10 – 2,
4. 37 – 27,
5. 6 + 7

Solution:

1. 16 ÷ 2 = 8
2. 5 × 2 = 10
3. 9 + 4 = 13
4. 72 ÷ 3 = 24
5. 4 + 5 = 9
6. 8 × 3 = 24
7. 19 – 10 = 9
8. 10 – 2 = 8
9. 37 – 27 = 10
10. 6 + 7 = 13

∴ The equations are

1. 16 ÷ 2 = 10 – 2
2. 5 × 2 = 37 – 27
3. 9 + 4 = 6 + 7
4. 72 ÷ 3 = 8 x 3
5. 4 + 5 = 19 – 10

Balbharti Maharashtra State Board Class 6 Maths Solutions covers the Std 6 Maths Chapter 1 Basic Concepts in Geometry Class 6 Practice Set 1 Answers Solutions.

6th Standard Maths Practice Set 1 Answers Chapter 1 Basic Concepts in Geometry

Question 1.
Look at the figure alongside and name the following:
i. Collinear points
ii. Rays
iii. Line segments
iv. Lines

Solution:

Question 2.
Write the different names of the line.

Solution:
The different names of the given line are line l, line AB, line AC, line AD, line BC, line BD and line CD.

Question 3.

Solution:
(i – Line),
(ii – Line Segment),
(iii – Plane),
(iv – Ray)

Question 4.
Observe the given figure. Name the parallel lines, the concurrent lines and the points of concurrence in the figure.

Solution:

Maharashtra Board Class 6 Maths Chapter 1 Basic Concepts in Geometry Intext Questions and Activities

Question 1.
Complete the rangoli. Then, have a class discussion with the help of the following questions:

1. What kind of surface do you need for making a rangoli?
2. How do you start making a rangoli?
3. What did you do in order to complete the rangoli?
4. Name the different shapes you see in the rangoli.
5. Would it be possible to make a rangoli on a scooter or on an elephant’s back?
6. When making a rangoli on paper, what do you use to make the dots?

Solution:

1. For making a rangoli, I need a flat surface.
2. I can start making a rangoli by drawing equally spaced dots on the flat surface using a chalk.
3. In order to complete the rangoli, I joined the dots by straight lines to make a design.
4. In the rangoli, I find various shapes such as square, rectangle and triangles of two different size.
5. No. It won’t be possible to make a rangoli on a scooter or on an elephant’s back as they do not have a flat surface.
6. When making a rangoli on paper, I made use of scale and pencil to make equally spaced dots.

Question 2.
Write the proper term, ‘intersecting lines’ or ‘parallel lines’ in each of the empty boxes. (Textbook pg. no. 4)

Solution:
i. Intersecting Lines
ii. Parallel Lines
iii. Intersecting Lines

Question 3.
Draw a point on the blackboard. Every student now draws a line that passes through that point. How many such lines can be drawn? (Textbook pg. no. 2)
Solution:
An infinite number of lines can be drawn through one point.

Question 4.
Draw a point on a paper and use your ruler to draw lines that pass through it. How many such lines can you draw? (Textbook pg. no. 2)
Solution:
An infinite number of lines can be drawn through one point.

Question 5.
There are 9 points in the figure. Name them. (Textbook pg. no. 3)
i. If you choose any two points, how many lines can pass through the pair?
ii. Which three or more of these nine points lie on a straight line?
iii. Of these nine points, name any three or more points which do not lie on the same line.

Solution:
i. One and only one line can be drawn through two distinct , points.
ii. Points A, B, C and D lie on the same line. Points F, G and C lie on the same line.
iii. Points E, F, G, H and I do not lie on the same line, points A, B, E, H and I do not lie on the same line.

Question 6.
Observe the picture of the game being played. Identify the collinear players, non-collinear players, parallel lines and the plane. (Textbook pg. no. 4)

Solution:

Question 7.
In January, we can see the constellation of Orion in the eastern sky after seven in the evening. Then it moves up slowly in the sky. Can you see the three collinear stars in this constellation? Do you also see a bright star on the same line some distance away? (Textbook pg. no. 4)

Solution:

1. The three stars shown by points C, D and E are collinear.
2. The star shown by point H lies on the same line as the stars C, D and E.

Question 8.
Maths is fun! (Textbook pg. no. 5)
Take a flat piece of thermocol or cardboard, a needle and thread. Tie a big knot or button or bead at one end of the thread. Thread the needle with the other end. Pass the needle up through any convenient point P. Pull the thread up, leaving the knot or the button below. Remove the needle and put it aside. Now hold the free end of the thread and gently pull it straight. Which figure do you see? Now, holding the thread straight, turn it in different directions. See how a countless number of lines can pass through a single point P.

Solution:

1. The pulled thread forms a straight line.
2. An infinite number of lines can be drawn through one point.

Question 9.
Choose the correct option for each of the following questions:
i. ______ is used to name a point.
(A) Capital letter
(B) Small letter
(C) Number
(D) Roman numeral
Solution :
(A) Capital letter

ii. A line segment has two points showing its limits. They are called_____
(A) origin
(B) end points
(C) arrow heads
(D) infinite points
Solution :
(B) end points

iii. An arrow head is drawn at one end of the ray to show that it is _____ on that side.
(A) finite
(B) ending
(C) infinite
(D) broken
Solution :
(C) infinite

iv. Lines which lie in the same plane but do not intersect are said to be ____ to each other.
(A) intersecting
(B) collinear
(C) parallel
(D) non-collinear
Solution :
(C) parallel

Question 10.
Determine the collinear and non-collinear points in the figure alongside:

Solution:
Collinear points:

1. Points A, E, H and C.
2. Points B, E, I and D.

Non-collinear points:
Points B, G, F and I

Question 11.
Look at the figure alongside and answer the questions given below:
i. Name the parallel lines.
ii. Name the concurrent lines and the point of concurrence.
iii. Write the different names of line PV.

Solution:
i. Parallel lines:
a. line l and line n
b. line p, line q, line r and line s

ii. Concurrent Lines: line q, line m, line n
Point of Concurrence: point S

iii. line l, line PT, line PR, line PV, line RT, line RV and line TV.

Question 12.
Name the different line segments and rays in the given figure:

Solution:
Line Segments:
seg UV, seg OY, seg OX, seg OV and seg OU

Rays:
ray OV, ray OX, ray OY and ray UV.

Balbharti Maharashtra State Board Class 6 Maths Solutions covers the Std 6 Maths Chapter 4 Operations on Fractions Class 6 Practice Set 13 Answers Solutions.

6th Standard Maths Practice Set 13 Answers Chapter 4 Operations on Fractions

Question 1.
Write the reciprocals of the following numbers:

1. 7
2. \(\frac { 11 }{ 3 }\)
3. \(\frac { 5 }{ 13 }\)
4. 2
5. \(\frac { 6 }{ 7 }\)

Solution:

1. \(\frac { 1 }{ 7 }\)
2. \(\frac { 3 }{ 11 }\)
3. \(\frac { 13 }{ 5 }\)
4. \(\frac { 1 }{ 2 }\)
5. \(\frac { 7 }{ 6 }\)

Question 2.
Carry out the following Divisions:
i. \(\frac{2}{3} \div \frac{1}{4}\)
ii. \(\frac{5}{9} \div \frac{3}{2}\)
iii. \(\frac{3}{7} \div \frac{5}{11}\)
iv. \(\frac{11}{12} \div \frac{4}{7}\)
Solution:
i. \(\frac{2}{3} \div \frac{1}{4}\)

ii. \(\frac{5}{9} \div \frac{3}{2}\)

iii. \(\frac{3}{7} \div \frac{5}{11}\)

iv. \(\frac{11}{12} \div \frac{4}{7}\)

Question 3.
There were 420 students participating in the Swachh Bharat Campaign. They cleaned \(\frac { 42 }{ 75 }\) part of the town, Sevagram. What part of Sevagram did each student clean if the work was equally shared by all?
Solution:
Total number of students = 420
Part of town cleaned by all the students = \(\frac { 42 }{ 75 }\)
∴ Part of town cleaned by one student

∴ Part of town cleaned bv one student is \(\frac { 1 }{ 750 }\)

Maharashtra Board Class 6 Maths Chapter 4 Operations on Fractions Practice Set 13 Intext Questions and Activities

Question 1.
Ramanujan’s Magic square. (Textbook pg. no. 28)

• Add the four numbers in the rows, the columns and along the diagonals of this square.
• What is the sum?
• Is it the same every time?
• What is the peculiarity?
• Look at the numbers in the first row, 22 – 12 – 1887. Find out why this date is special.

Obtain and read a biography of the great Indian mathematician Srinivasa Ramanujan.
Solution:
Sum of the numbers in each row:
i. 22 + 12 + 18 + 87 = 139
ii. 88 + 17 + 9 + 25 = 139
iii. 10 + 24 + 89 + 16 = 139
iv. 19 + 86 + 23 + 11 = 139

Sum of the numbers along the diagonals:
i. 22 + 17 + 89 + 11 = 139
ii. 87 + 9 + 24 + 19 = 139

Sum of the numbers in each column:
i. 22 + 88 + 10 + 19 = 139
ii. 12 + 17 + 24 + 86 = 139
iii. 18 + 9 + 89 + 23 = 139
iv. 87 + 25 + 16 + 11 = 139

∴ We observe that the sum of the numbers in each of the rows, the columns and along each diagonal remains the same every time. The numbers in the first row 22 – 12 – 1887 is the birth date of Srinivasa Ramanujan.

Balbharti Maharashtra State Board Class 6 Maths Solutions covers the Std 6 Maths Chapter 14 Banks and Simple Interest Class 6 Practice Set 35 Answers Solutions.

6th Standard Maths Practice Set 35 Answers Chapter 14 Banks and Simple Interest

Question 1.
At a rate of 10 p.c.p.a., what would be the interest for one year on Rs 6000?
Solution:
Principal Amount = Rs 6000
Rate of Interest = 10 p.c.p.a.
Let interest on principal Rs 6000 be Rs x.
By taking ratio of the interest to the principal for both, we obtain an equation x 10

∴ The interest for one year is Rs 600.

Question 2.
Mahesh deposited Rs 8650 in a bank at a rate of 6 p.c.p.a. How much money will he get at the end of the year in all?
Solution:
Principal Amount = Rs 8650
Rate of interest = 6 p.c.p.a.
Let interest on principal Rs 8650 be Rs x
By taking ratio of the interest to the principal for both, we obtain an equation

∴ Amount received at the end of the year = Principal amount + Interest
= Rs 8650 + Rs 519
= Rs 9169
∴ Mahesh will get Rs 9169 at the end of the year.

Question 3.
Ahmad Chacha borrowed Rs 25,000 at 12 p.c.p.a. for a year. What amount will he have to return to the bank at the end of the year?
Solution:
Principal Amount = Rs 25,000
Rate of interest = 12 p.c.p.a.
Let interest on principal Rs 25,000 be Rs x
By taking ratio of the interest to the principal for both, we obtain an equation

Amount to be returned to the bank at the end of the year = Principal Amount + Interest
= Rs 25,000 + Rs 3,000
= Rs 28,000
Ahmad Chacha has to return Rs 28,000 to the bank at the end of the year.

Question 4.
Kisanrao wanted to make a pond in his field. He borrowed Rs 35,250 from a bank at an interest rate of 6 p.c.p.a. How much interest will he have to pay to the bank at the end of the year?
Solution:
Principal Amount = Rs 35,250
Rate of interest = 6 p.c.p.a.
Let interest on principal Rs 35,250 be Rs x
By taking ratio of the interest to the principal for both, we obtain an equation

∴ Kisanrao will have to pay an interest of Rs 2115 to the bank at the end of the year.

Maharashtra Board Class 6 Maths Chapter 14 Banks and Simple Interest Practice Set 35 Intext Questions and Activities

Question 1.
Study the figure given below and answer the following questions (Textbook pg. no. 74)

1. In the above picture, who are the people shown to be using bank services?
2. What does the symbol on the bag in the centre stand for?
3. What do the arrows in the given picture tell you?

Solution:

1. Students, farmers, Women’s savings groups, industrialists / professionals and traders / businessmen are shown to be using bank services.
2. The symbol on the bag in the center stands for rupees.
3. The arrows tell us about the monetary transactions taking place. In simple words, it explains the give and take relationship.

Question 2.
Visit the Bank (Textbook pg. no. 74)

• Teachers should organise a visit to a bank. Encourage the children to obtain some preliminary information about banks.
• Help them to fill some bank forms and slips for withdrawals and deposits.
• If there is no bank nearby, teachers could obtain specimen forms and get the children to fill them in class.
• Give a demonstration of banking transactions by setting up a mock bank in the school.
• Invite participation of parents who work in banks or other bank employees to give the children more detailed information about banking.

Solution:
(Students should attempt this activity with the help of their teacher/parents.)

Balbharti Maharashtra State Board Class 6 Maths Solutions covers the Std 6 Maths Chapter 13 Profit-Loss Class 6 Practice Set 34 Answers Solutions.

6th Standard Maths Practice Set 34 Answers Chapter 13 Profit-Loss

Question 1.
Cost price Rs 1600, selling price Rs 2800.
Solution:
Sanju bought goods worth Rs 1600 and sold them for Rs 2800. What was his profit in percentage?
Cost price = Rs 1600, Selling price = Rs 2800
Profit = Selling price – Cost price
= 2800 – 1600
= Rs 1200
Let Sanju make profit of x%.

∴ x = 75%
∴ Sanju made a profit of 75%.

Question 2.
Cost price Rs 2000, selling price Rs 1900.
Solution:
Rakhi bought books worth Rs 2000 and sold them for Rs 1900. What was her loss in percentage?
Cost price = Rs 2000, Selling price = Rs 1900
Loss = Cost price – Selling price .
= 2000 – 1900
= Rs 100
Let Rakhi incur a loss of x%.

∴ x = 5%
∴ Rakhi suffered a loss of 5%.

Question 3.
Cost price of 8 articles is Rs 1200 each, selling price Rs 1400 each.
Solution:
Pallavi bought 8 tables for Rs 1200 each and sold them for Rs 1400 each. What was the percentage of her profit or loss?
Cost price of 1 table = Rs 1200
∴ Cost price of 8 tables = 1200 x 8 = Rs 9600
Selling price of 1 table = Rs 1400
∴ Selling price of 8 tables = 1400 x8 = Rs 11200
Selling price is greater than the cost price.
∴ Pallavi made a profit.
∴ Profit = Selling price – Cost price
= 11200 – 9600
= Rs 1600
Let Pallavi make a profit of x%.

∴ Pallavi made a profit of \(16\frac { 2 }{ 3 }\) %.

Question 4.
Cost price of 50 kg grain Rs 2000, Selling price Rs 43 per kg.
Solution:
Ramesh bought 50 kg grains for Rs 2000 and sold it at the rate of Rs 43 per kg. Find the percentage of profit or loss.
Cost price of 50 kg grains = Rs 2000
Selling price of 1 kg grains = Rs 43
∴ Selling price of 50 kg grains= 43 x 50
= Rs 2150
Selling price is greater than the cost price.
∴ Ramesh made a profit.
∴ Profit = Selling price – Cost price
= 2150 – 2000
= Rs 150
Let Ramesh make profit of x%.

∴ Ramesh made a profit of \(7\frac { 1 }{ 2 }\) %.

Question 5.
Cost price Rs 8600, Transport charges Rs 250, Portage Rs 150, Selling price Rs 10000.
Solution:
Faruk bought a fridge for Rs 8600. He spent Rs 250 on transport and Rs 150 on portage.
If he sold the fridge for Rs 10,000, what was his percent profit or loss?
Total cost price of a fridge = Cost of fridge + Transportation cost + Portage
= 8600 + 250 + 150
= Rs 9000
∴ Selling price = Rs 10,000
Selling price is greater than the total cost price.
∴ Faruk made a profit.
Profit = Selling price – Total cost price
= 10000 – 9000
= Rs 1000
Let Faruk make a profit of x% on cost price.

∴ Faruk made a profit of \(11\frac { 1 }{ 9 }\) %.

Question 6.
Seeds worth Rs 20500, Labour Rs 9700, Chemicals and fertilizers Rs 5600, selling price Rs 28640.
Solution:
Ramchandra bought sunflower seeds worth Rs 20500. He spent Rs 9700 on labour and Rs 5600 on chemicals and fertilizers. He sold it for Rs 28640. What is the percentage of profit or loss?
Total cost price = Cost of seeds + Labour cost + Cost of chemicals and Fertilizers
= 20500 + 9700 + 5600
= Rs 35800
Selling price = Rs 28,640
The total cost price is greater than selling price.
∴ Ramchandra suffered a loss.
Loss = Total cost price – Selling price
= 35800- 28640
= Rs 7160
Let Ramchandra incur a loss of x%.

∴ x = 20%
∴ Ramchandra incurred a loss of 20%.

Maharashtra Board Class 6 Maths Chapter 13 Profit-Loss Practice Set 34 Intext Questions and Activities

Question 1.
Maths is fun! (Textbook pg. no. 72)

Arpita used 4 matchsticks to make a square. Then she took 3 more sticks and arranged them to make 2 squares. Another 3 sticks helped her to make 3 squares. How many sticks are needed to make 7 such squares in the same way? How many sticks are needed to make 50 squares?
Solution:
Matchsticks needed to make 7 squares = 4 + (6 × 3)
= 22
Matchsticks needed to make 50 squares= 4 + (49 × 3)
= 151

Question 2.
Project (Textbook pg. no. 72)
i. Relate instances of profit and loss that you have experienced. Express them as problems and solve the problems.
ii. Organize a fair. Gain the experience of selling things/trading. What was the expenditure on preparing or obtaining the good to be sold? How much were the sales worth? Write a composition about it or enact this entire transaction.
Solution:
(Students should attempt the activities on their own.)

Balbharti Maharashtra State Board Class 6 Maths Solutions covers the Std 6 Maths Chapter 4 Operations on Fractions Class 6 Practice Set 12 Answers Solutions.

6th Standard Maths Practice Set 12 Answers Chapter 4 Operations on Fractions

Question 1.
Multiply:
i. \(\frac{7}{5} \times \frac{1}{4}\)
ii. \(\frac{6}{7} \times \frac{2}{5}\)
iii. \(\frac{5}{9} \times \frac{4}{9}\)
iv. \(\frac{4}{11} \times \frac{2}{7}\)
v. \(\frac{1}{5} \times \frac{7}{2}\)
vi. \(\frac{9}{7} \times \frac{7}{8}\)
vii. \(\frac{5}{6} \times \frac{6}{5}\)
viii. \(\frac{6}{17} \times \frac{3}{2}\)
Solution:
i. \(\frac{7}{5} \times \frac{1}{4}\)

ii. \(\frac{6}{7} \times \frac{2}{5}\)

iii. \(\frac{5}{9} \times \frac{4}{9}\)

iv. \(\frac{4}{11} \times \frac{2}{7}\)

v. \(\frac{1}{5} \times \frac{7}{2}\)

vi. \(\frac{9}{7} \times \frac{7}{8}\)

vii. \(\frac{5}{6} \times \frac{6}{5}\)

viii. \(\frac{6}{17} \times \frac{3}{2}\)

Question 2.
Ashokrao planted bananas on \(\frac { 2 }{ 7 }\) of his field of 21 acres. What is the area of the banana plantation?
Solution:
Area of banana plantation is \(\frac { 2 }{ 7 }\) of 21
∴ Area of banana plantation

∴ Area of banana plantation is 6 acres

Question 3.
Of the total number of soldiers in our army, \(\frac { 4 }{ 9 }\) are posted on the northern border and one-third of them on the north-eastern border. If the number of soldiers in the north is 5,40,000, how many are posted in the north-east?
Solution:
Number of soldiers posted on northern border = 5,40,000
Since, number of soldiers in north-east = one third of the soldiers on northern border
∴ Number of soldiers in the north-east

∴ The number of soldiers in the north-east is 1,80,000.

Balbharti Maharashtra State Board Class 6 Maths Solutions covers the Std 6 Maths Chapter 9 HCF-LCM Class 6 Practice Set 25 Answers Solutions.

6th Standard Maths Practice Set 25 Answers Chapter 9 HCF-LCM

Question 1.
Find out the LCM of the following numbers.
i. 9,15
ii. 2,3,5
iii. 12,28
iv. 15,20
v. 8,11
Solution:
i. Multiples of 9 = 9, 18, 27, 36, 45, 54, 63, 72, 81, 90
Multiples of 15 = 15, 30, 45
∴ LCM of 9 and 15 = 45

ii. Multiples of 2 = 2, 4,6, 8, 10, 12, 14, 16, 18, 20, 22, 24, 26, 28, 30
Multiples of 3 = 3, 6, 9, 12, 15, 18, 21, 24, 27, 30
Multiples of 5 = 5, 10, 15, 20, 25, 30
∴ LCM of 2,3 and 5 = 30

iii. Multiples of 12 = 12, 24, 36, 48, 60, 72, 84, 96, 108, 120
Multiples of 28 = 28, 56, 84
∴ LCM of 12 and 28 = 84

iv. Multiples of 15 = 15, 30, 45, 60, 75, 90, 105, 120
Multiples of 20 = 20, 40, 60
∴ LCM of 15 and 20 = 60

v. Multiples of 8 = 8, 16, 24, 32, 40, 48, 56, 64, 72, 80, 88, 96
Multiples of 11 = 11, 22, 33, 44, 55, 66, 77, 88
∴ LCM of 8 and 11 = 88

Question 2.
Solve the following problems:
i. On the playground, if the children are made to stand for drill either 20 to a row or 25 to a row, all rows are complete and no child is left out. What is the lowest possible number of children in that school?

ii. Veena has some beads. She wants to make necklaces with an equal number of beads in each. If She makes necklaces of 16 or 24 or 40 beads, there is no bead left over. What is the least number of beads with her?

iii. An equal number of laddoos have been placed in 3 different boxes. The laddoos in the first box were distributed among 20 children equally, the laddoos in the second box among 24 children and those in the third box among 12 children. Not a single laddoo was left over. What was the minimum number of laddoos in the three boxes altogether?

iv. We observed the traffic lights at three different squares on the same big road. They turn green every 60 seconds, 120 seconds and 24 seconds. When the signals are switched on at 8 o’clock in the morning, all the lights were green. How long after that will all three signals turn green simultaneously again?

v. Given the fractions \(\frac { 13 }{ 45 }\) and \(\frac { 22 }{ 75 }\). Write their equivalent fractions with same denominators and add the fractions.
Solution:
i. The lowest possible number of children is equal to the lowest common multiple of 20 and 25.
Multiples of 20 = 20, 40, 60, 80, 100, 120, 140, 160, 180, 200
Multiples of 25 = 25, 50, 75, 100
∴ LCM of 20 and 25 = 100
∴ The least number of students in the school is 100.

ii. The least number of beads with Veena is equal to the lowest common multiple of 16,24 and 40.
Multiples of 16 = 16, 32, 48, 64, 80, 96, 112, 128, 144, 160, 176, 192, 208, 224, 240, 256, 272, 288
Multiples of 24 = 24, 48, 72, 96, 120, 144, 168, 192, 216, 240
Multiples of 40 = 40, 80, 120, 160, 200, 240
∴ LCM of 16, 24 and 40 = 240
∴ The least number of beads with Veena are 240.

iii. The lowest common multiple of 20,24 and 12 gives the minimum number of laddoos in one box.
Multiples of 20 = 20, 40, 60, 80, 100,120, 140, 160, 180, 200
Multiples of 24 = 24, 48, 72, 96, 120
Multiples of 12 = 12, 24, 36, 48, 60, 72, 84, 96, 108, 120
∴ LCM of 20, 24 and 12 = 120
∴ Minimum number of ladoos in 1 boxes =120
∴ Minimum number of ladoos in 3 boxes = 3 x 120 = 360
∴ The minimum number of ladoos in 3 boxes are 360.

iv. All three signals will turn green for lowest common multiple of 60 seconds, 120 seconds and 24 seconds.
Multiples of 60 = 60, 120, 180, 240, 300, 360, 420, 480
Multiples of 120 = 120, 240, 360
Multiples of 24 = 24, 48, 72, 96, 120
LCM of 60, 120 and 24 = 120
Since, 60 seconds = 1 minute
∴ 120 seconds = 2 minutes
∴ The signals will turn green simultaneously again after 120 seconds i.e. 2 minutes.

v. The lowest common multiple of 45 and 75 gives the same denominator.
Multiples of 45 = 45, 90, 135, 180, 225, 270, 315, 360, 405, 450
Multiples of 75 = 75, 150, 336
∴ LCM of 45 and 75 = 225

Maharashtra Board Class 6 Maths Chapter 9 HCF-LCM Practice Set 25 Intext Questions and Activities

Question 1.
Pravin, Bageshri and Yash are cousins who live in the same house. Pravin is an Army Officer. Bageshri is studying in a Medical College in another city. Yash lives in a nearby town in a hostel. Pravin can come home every 120 days.
Bageshri comes home every 45 days and Yash, every 30 days. All three of them left home at the same time on the 15th of June 2016. Their parents said, “We shall celebrate like a festival the day you all come home together.” Mother asked Yash, “What day will that be?”
Yash said, “The number of days after which we come back together must be divisible by 30, 120. That means we shall be back together on the 10th of June next year. That will certainly be a for us!”
How did Yash find the answer? (Textbook pg. no. 49)

Solution:
The day when Pravin, Bageshri and Yash come back together is lowest common multiple of 30, 45 and 120.
Multiples of 30: 30, 60, 90, 120, 150, 180,210, 240, 270, 300, 330, 360
Multiples of 45: 45, 90, 135, 180, 225, 270, 315, 360
Multiples of 120: 120, 240, 360
∴ They will come together after 360 days
Day when they left home = 15th June
∴ Day when they come back together = 15th June + 360 days
= 10th June next year
∴ Pravin, Bageshri and Yash will come back together on 10th June next year.

Question 2.
A Maths Riddle! (Textbook pg. no. 50)
We have four papers. On each of them there is a number on one side and some information on the other. The numbers on the papers are 7, 2, 15, 5. The information on the papers is given below in random order.
i. A number divisible by 7
ii. A prime number
iii. An odd number
iv. A number greater than 100
If the number on every paper is mismatched with the information on its other side, what is the number on the paper which says ‘A number greater than 100?
Solution:

Balbharti Maharashtra State Board Class 6 Maths Solutions covers the Std 6 Maths Chapter 4 Operations on Fractions Class 6 Practice Set 11 Answers Solutions.

6th Standard Maths Practice Set 11 Answers Chapter 4 Operations on Fractions

Question 11.
What fractions do the points A and B show on the number lines below?

Solution:
(1) Each unit is divided in 6 parts
A is 5th division from 0
∴ \(A=\frac { 5 }{ 6 }\)

B is 10th division from 0
∴ \(B=\frac { 10 }{ 6 }\)

(2) Each unit is divided in 5 parts
A is 3rd division from 0
∴ \(A=\frac { 3 }{ 5 }\)

B is 7th division from 0
∴ \(B=\frac { 7 }{ 5 }\)

(3) Each unit is divided in 7 parts
A is 10th division from 0
∴ \(A=\frac { 10 }{ 7 }\)

B is 3rd division from 0
∴ \(B=\frac { 3 }{ 7 }\)

Question 2.
Show the following fractions on the number line:
i. \(\frac{3}{5}, \frac{6}{5}, 2 \frac{3}{5}\)
ii. \(\frac{3}{4}, \frac{5}{4}, 2 \frac{1}{4}\)
Solution:
i. \(\frac{3}{5}, \frac{6}{5}, 2 \frac{3}{5}\)

ii. \(\frac{3}{4}, \frac{5}{4}, 2 \frac{1}{4}\)

Maharashtra Board Class 6 Maths Chapter 4 Operations on Fractions Practice Set 11 Intext Questions and Activities

Question 1.
If we want to show the fractions \(\frac{3}{10}, \frac{9}{20}, \frac{19}{40}\) on the number line, how big should the unit be? (Textbook pg. no. 24)
Solution:
The denominators of the given fractions are not equal.
The numbers in the denominators 10, 20 and 40 have common multiple 40.
∴ Making the denominators equal, we get

∴ To represent these fractions on the numbers line, each main unit should be divided into 40 equal sub-units.

Therefore,
\(\frac{3}{10}=\frac{12}{40}\) is represented on 12th mark from 0.
\(\frac{9}{20}=\frac{18}{40}\) is represented on 18th mark from 0 and
\(\frac { 19 }{ 40 }\) is represented on 19 mark from 0.