Class 12

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 2 Matrices Ex 2.2 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 2 Matrices Ex 2.2

Question 1.
If A = \(\left[\begin{array}{cc}
2 & -3 \\
5 & -4 \\
-6 & 1
\end{array}\right]\), B = \(\left[\begin{array}{cc}
-1 & 2 \\
2 & 2 \\
0 & 3
\end{array}\right]\) and C = \(\left[\begin{array}{cc}
4 & 3 \\
-1 & 4 \\
-2 & 1
\end{array}\right]\) show that
(i) A + B = B + A
(ii) (A + B) + C = A + (B + C)
Solution:


From (1) and (2), we get
(A + B) + C = A + (B + C).

Question 2.
If A = \(\left[\begin{array}{cc}
1 & -2 \\
5 & 3
\end{array}\right]\), B = \(\left[\begin{array}{ll}
1 & -3 \\
4 & -7
\end{array}\right]\), then find the matrix A – 2B + 6I, where I is the unit matrix of order 2.
Solution:

Question 3.
If A = \(\left[\begin{array}{ccc}
1 & 2 & -3 \\
-3 & 7 & -8 \\
0 & -6 & 1
\end{array}\right]\), B = \(\left[\begin{array}{ccc}
9 & -1 & 2 \\
-4 & 2 & 5 \\
4 & 0 & -3
\end{array}\right]\), then find the matrix C such that A + B + C is a zero matrix.
Solution:
A + B + C = 0
∴ C = -A – B

Question 4.
If A = \(\left[\begin{array}{cc}
1 & -2 \\
3 & -5 \\
-6 & 0
\end{array}\right]\), B = \(\left[\begin{array}{cc}
-1 & -2 \\
4 & 2 \\
1 & 5
\end{array}\right]\) and C = \(\left[\begin{array}{cc}
2 & 4 \\
-1 & -4 \\
-3 & 6
\end{array}\right]\), find the matrix X such that 3A – 4B + 5X = C.
Solution:
3A – 4B + 5X = C
∴ 5X = C – 3A + 4B

Question 5.
If A = \(\left[\begin{array}{ccc}
5 & 1 & -4 \\
3 & 2 & 0
\end{array}\right]\), find (A^T)^T.
Solution:
A = \(\left[\begin{array}{ccc}
5 & 1 & -4 \\
3 & 2 & 0
\end{array}\right]\)
∴ A^T = \(\left[\begin{array}{rr}
5 & 3 \\
1 & 2 \\
-4 & 0
\end{array}\right]\)
∴ (A^T)^T = \(\left[\begin{array}{ccc}
5 & 1 & -4 \\
3 & 2 & 0
\end{array}\right]\) = A

Question 6.
If A = \(\left[\begin{array}{ccc}
7 & 3 & 1 \\
-2 & -4 & 1 \\
5 & 9 & 1
\end{array}\right]\), find (A^T)^T.
Solution:
A = \(\left[\begin{array}{ccc}
7 & 3 & 1 \\
-2 & -4 & 1 \\
5 & 9 & 1
\end{array}\right]\)
∴ A^T = \(\left[\begin{array}{rrr}
7 & -2 & 5 \\
3 & -4 & 9 \\
1 & 1 & 1
\end{array}\right]\)
∴ (A^T)^T = \(\left[\begin{array}{ccc}
7 & 3 & 1 \\
-2 & -4 & 1 \\
5 & 9 & 1
\end{array}\right]\) = A

Question 7.
Find a, b, c if \(\left[\begin{array}{ccc}
1 & \frac{3}{5} & a \\
b & -5 & -7 \\
-4 & c & 0
\end{array}\right]\) is a symetric matrix.
Solution:
Let A = \(\left[\begin{array}{ccc}
1 & \frac{3}{5} & a \\
b & -5 & -7 \\
-4 & c & 0
\end{array}\right]\)
Since, A is a symmetric matrix, a_ij = a_ji for all i and j

Question 8.
Find x, y, z if \(\left[\begin{array}{ccc}
0 & -5 i & x \\
y & 0 & z \\
\frac{3}{2} & -\sqrt{2} & 0
\end{array}\right]\) is a skew symmetric matrix.
Solution:
Let A = \(\left[\begin{array}{ccc}
0 & -5 i & x \\
y & 0 & z \\
\frac{3}{2} & -\sqrt{2} & 0
\end{array}\right]\)
Since, A is skew-symmetric matrix,

Question 9.
For each of the following matrices, find its transpose and state whether it is symmetric, skew-symmetric or neither:
(i) \(\left[\begin{array}{ccc}
1 & 2 & -5 \\
2 & -3 & 4 \\
-5 & 4 & 9
\end{array}\right]\)
Solution:
Let A = \(\left[\begin{array}{ccc}
1 & 2 & -5 \\
2 & -3 & 4 \\
-5 & 4 & 9
\end{array}\right]\)
Then A^T = \(\left[\begin{array}{rrr}
1 & 2 & -5 \\
2 & -3 & 4 \\
-5 & 4 & 9
\end{array}\right]\)
Since, A = A^T, A is a symmetric matrix.

(ii) \(\left[\begin{array}{ccc}
2 & 5 & 1 \\
-5 & 4 & 6 \\
-1 & -6 & 3
\end{array}\right]\)
Solution:
Let B = \(\left[\begin{array}{ccc}
2 & 5 & 1 \\
-5 & 4 & 6 \\
-1 & -6 & 3
\end{array}\right]\)
Then B^T = \(\left(\begin{array}{rrr}
2 & -5 & -1 \\
5 & 4 & -6 \\
1 & 6 & 3
\end{array}\right)\)
∴ B ≠ B^T
Also,
-B^T = \(\left(\begin{array}{rrr}
2 & -5 & -1 \\
5 & 4 & -6 \\
1 & 6 & 3
\end{array}\right)=\left(\begin{array}{rrr}
-2 & 5 & 1 \\
-5 & -4 & 6 \\
-1 & -6 & -3
\end{array}\right)\)
∴ B ≠ -B^T
Hence, B is neither symmetric nor skew-symmetric matrix.

(iii) \(\left[\begin{array}{ccc}
0 & 1+2 i & i-2 \\
-1-2 i & 0 & -7 \\
2-i & 7 & 0
\end{array}\right]\)
Solution:

Hence, C is a skew-symmetric matrix.

Question 10.
Construct the matrix A = [a_ij]_3×3, where a_ij = i – j. State whether A is symmetric or skew-symmetric.
Solution:

Question 11.
Solve the following equations for X and Y, if 3X – Y = \(\left[\begin{array}{cc}
1 & -1 \\
-1 & 1
\end{array}\right]\) and X – 3Y = \(\left[\begin{array}{ll}
0 & -1 \\
0 & -1
\end{array}\right]\)
Solution:

Question 12.
Find matrices A and B, if 2A – B = \(\left[\begin{array}{ccc}
6 & -6 & 0 \\
-4 & 2 & 1
\end{array}\right]\) and A – 2B = \(\left[\begin{array}{ccc}
3 & 2 & 8 \\
-2 & 1 & -7
\end{array}\right]\)
Solution:

Question 13.
Find x and y, if \(\left[\begin{array}{ccc}
2 x+y & -1 & 1 \\
3 & 4 y & 4
\end{array}\right]+\left[\begin{array}{ccc}
-1 & 6 & 4 \\
3 & 0 & 3
\end{array}\right]=\left[\begin{array}{ccc}
3 & 5 & 5 \\
6 & 18 & 7
\end{array}\right]\)
Solution:

By equality of matrices, we get
2x + y – 1 = 3 ……..(1)
and 4y = 18 ……….(2)
From (2), y = \(\frac{9}{2}\)
Substituting y = \(\frac{9}{2}\) in (1), we get
2x + \(\frac{9}{2}\) – 1 = 3
∴ 2x = 3 – \(\frac{7}{2}\) = \(\frac{-1}{2}\)
∴ x = \(\frac{-1}{4}\)
Hence, x = \(\frac{-1}{4}\) and y = \(\frac{9}{2}\).

Question 14.
If \(\left[\begin{array}{cc}
2 a+b & 3 a-b \\
c+2 d & 2 c-d
\end{array}\right]=\left[\begin{array}{cc}
2 & 3 \\
4 & -1
\end{array}\right]\), find a, b, c and d.
Solution:
\(\left[\begin{array}{cc}
2 a+b & 3 a-b \\
c+2 d & 2 c-d
\end{array}\right]=\left[\begin{array}{cc}
2 & 3 \\
4 & -1
\end{array}\right]\)
By equality of matrices,
2a + b = 2 ….. (1)
3a – b = 3 …… (2)
c + 2d = 4 …… (3)
2c – d = -1 …… (4)
Adding (1) and (2), we get
5a = 5
∴ a = 1
Substituting a = 1 in (1), we get
2(1) + b = 2
∴ b = 0
Multiplying equation (4) by 2, we get
4c – 2d = -2 …… (5)
Adding (3) and (5), we get
5c = 2
∴ c = \(\frac{2}{5}\)
Substituting c = \(\frac{2}{5}\) in (4), we get
2(\(\frac{2}{5}\)) – d = -1
∴ d = \(\frac{4}{5}\) + 1 = \(\frac{9}{5}\)
Hence, a = 1, b = 0, c = \(\frac{2}{5}\) and d = \(\frac{9}{5}\).

Question 15.
There are two book shops owned by Suresh and Ganesh. Their sales (in Rupees) for books in three subjects – Physics, Chemistry and Mathematics for two months, July and August 2017 are given by two matrices A and B:
July sales (in Rupees), Physics, Chemistry, Mathematics
A = \(\left[\begin{array}{lll}
5600 & 6750 & 8500 \\
6650 & 7055 & 8905
\end{array}\right]\) First Row Suresh / Second Row Ganesh
August Sales (in Rupees), Physics, Chemistry, Mathematics
B = \(\left[\begin{array}{ccc}
6650 & 7055 & 8905 \\
7000 & 7500 & 10200
\end{array}\right]\) First Row Suresh / Second Row Ganesh
(i) Find the increase in sales in Z from July to August 2017.
(ii) If both book shops get 10% profit in the month of August 2017,
find the profit for each bookseller in each subject in that month.
Solution:
The sales for July and August 2017 for Suresh and Ganesh are given by the matrices A and B as:

(i) The increase in sales (in ₹) from July to August 2017 is obtained by subtracting the matrix A from B.


Hence, the increase in sales (in ₹) from July to August 2017 for:
Suresh book shop: ₹ 1050 in Physics, ₹ 305 in Chemistry, and ₹ 405 in Mathematics.
Ganesh book shop: ₹ 350 in Physics, ₹ 445 in Chemistry, and ₹ 1295 in Mathematics.
(ii) Both the book shops get 10% profit in August 2017,
the profit for each bookseller in each subject in August 2017 is obtained by the scalar multiplication of matrix B by 10%,
i.e. \(\frac{10}{100}=\frac{1}{10}\)

Hence, the profit for Suresh book shop are ₹ 665 in Physics, ₹ 705.50 in Chemistry and ₹ 890.50 in Mathematics and for Ganesh book shop are ₹ 700 in Physics, ₹ 750 in Chemistry and ₹ 1020 in Mathematics.

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 2 Matrices Miscellaneous Exercise 2 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 2 Matrices Miscellaneous Exercise 2

(I) Choose the correct alternative.

Question 1.
If AX = B, where A = \(\left[\begin{array}{cc}
-1 & 2 \\
2 & -1
\end{array}\right]\), B = \(\left[\begin{array}{l}
1 \\
1
\end{array}\right]\) then X = ___________
(a) \(\left[\begin{array}{l}
\frac{3}{5} \\
\frac{3}{7}
\end{array}\right]\)
(b) \(\left[\begin{array}{l}
\frac{7}{3} \\
\frac{5}{3}
\end{array}\right]\)
(c) \(\left[\begin{array}{l}
1 \\
1
\end{array}\right]\)
(d) \(\left[\begin{array}{l}
1 \\
2
\end{array}\right]\)
Answer:
(c) \(\left[\begin{array}{l}
1 \\
1
\end{array}\right]\)

Question 2.
The matrix \(\left[\begin{array}{lll}
8 & 0 & 0 \\
0 & 8 & 0 \\
0 & 0 & 8
\end{array}\right]\) is ___________
(a) identity matrix
(b) scalar matrix
(c) null matrix
(d) diagonal matrix
Answer:
(b) scalar matrix

Question 3.
The matrix \(\left[\begin{array}{lll}
0 & 0 & 0 \\
0 & 0 & 0
\end{array}\right]\) is ___________
(a) identity matrix
(b) diagonal matrix
(c) scalar matix
(d) null matrix
Answer:
(d) null matrix

Question 4.
If A = \(\left[\begin{array}{lll}
a & 0 & 0 \\
0 & a & 0 \\
0 & 0 & a
\end{array}\right]\), then |adj A| = ___________
(a) a¹²
(b) a⁹
(c) a⁶
(d) a^-3
Answer:
(c) a6
Hint:
adj A = \(\left[\begin{array}{ccc}
a^{2} & 0 & 0 \\
0 & a^{2} & 0 \\
0 & 0 & a^{2}
\end{array}\right]\)
∴ |adj A| = a²(a⁴ – 0) = a⁶

Question 5.
Adjoint of \(\left[\begin{array}{ll}
2 & -3 \\
4 & -6
\end{array}\right]\) is ___________
(a) \(\left[\begin{array}{ll}
-6 & 3 \\
-4 & 2
\end{array}\right]\)
(b) \(\left[\begin{array}{cc}
6 & 3 \\
-4 & 2
\end{array}\right]\)
(c) \(\left[\begin{array}{cc}
-6 & -3 \\
4 & 2
\end{array}\right]\)
(d) \(\left[\begin{array}{cc}
-6 & 3 \\
4 & -2
\end{array}\right]\)
Answer:
(a) \(\left[\begin{array}{ll}
-6 & 3 \\
-4 & 2
\end{array}\right]\)

Question 6.
If A = diag. [d₁, d₂, d₃, …, d_n], where d₁ ≠ 0, for i = 1, 2, 3, …….., n, then A^-1 = ___________
(a) diag [1/d₁, 1/d₂, 1/d₃, …, 1/d_n]
(b) D
(c) I
(d) O
Answer:
(a) diag [1/d₁, 1/d₂, 1/d₃, …, 1/d_n]

Question 7.
If A² + mA + nI = O and n ≠ 0, |A| ≠ 0, then A^-1 = ___________
(a) \(\frac{-1}{m}\)(A + nI)
(b) \(\frac{-1}{n}\)(A + mI)
(c) \(\frac{-1}{m}\)(I + mA)
(d) (A + mnI)
Answer:
(b) \(\frac{-1}{n}\)(A + mI)
Hint:
A² + mA + nI = 0
∴ (A² + mA + nI) . A^-1 = 0 . A^-1
∴ A(AA^-1) + m(AA^-1) + nIA^-1 = 0
∴ AI + mI + nA^-1 = 0
∴ nA^-1 = -A – mI
∴ A^-1 = \(\frac{-1}{n}\)(A + mI)

Question 8.
If a 3 × 3 matrix B has its inverse equal to B, then B² = ___________
(a) \(\left[\begin{array}{lll}
0 & 1 & 1 \\
0 & 1 & 0 \\
1 & 0 & 1
\end{array}\right]\)
(b) \(\left[\begin{array}{lll}
1 & 1 & 1 \\
1 & 1 & 1 \\
1 & 0 & 1
\end{array}\right]\)
(c) \(\left[\begin{array}{lll}
1 & 0 & 1 \\
0 & 1 & 0 \\
0 & 0 & 0
\end{array}\right]\)
(d) \(\left[\begin{array}{lll}
1 & 0 & 0 \\
0 & 1 & 0 \\
0 & 0 & 1
\end{array}\right]\)
Answer:
(d) \(\left[\begin{array}{lll}
1 & 0 & 0 \\
0 & 1 & 0 \\
0 & 0 & 1
\end{array}\right]\)
Hint:
B^-1 = B
∴ B² = B.B^-1 = I

Question 9.
If A = \(\left[\begin{array}{cc}
\alpha & 4 \\
4 & \alpha
\end{array}\right]\) and |A³| = 729 then α = ___________
(a) ±3
(b) ±4
(c ) ±5
(d) ±6
Answer:
(c ) ±5
Hint:
|A|= \(\left|\begin{array}{ll}
\alpha & 4 \\
4 & \alpha
\end{array}\right|\) = α² – 16
∴ |A³| = |A|³ = (α² – 16)³ = 729
∴ α² – 16 = 9
∴ α² = 25
∴ α = ±5

Question 10.
If A and B square matrices of order n × n such that A² – B² = (A – B)(A + B), then which of the following will be always true?
(a) AB = BA
(b) either A or B is a zero matrix
(c) either of A and B is an identity matrix
(d) A = B
Answer:
(a) AB = BA
Hint:
A² – B² = (A – B)(A + B)
∴ A² – B² = A² + AB – BA – B²
∴ 0 = AB – BA
∴ AB = BA

Question 11.
If A = \(\left[\begin{array}{ll}
2 & 5 \\
1 & 3
\end{array}\right]\) then A^-1 = ___________
(a) \(\left[\begin{array}{rr}
3 & -5 \\
1 & 2
\end{array}\right]\)
(b) \(\left[\begin{array}{rr}
3 & -5 \\
-1 & 2
\end{array}\right]\)
(c) \(\left[\begin{array}{rr}
3 & 5 \\
-1 & 2
\end{array}\right]\)
(d) \(\left[\begin{array}{ll}
3 & -5 \\
1 & -2
\end{array}\right]\)
Answer:
(b) \(\left[\begin{array}{rr}
3 & -5 \\
-1 & 2
\end{array}\right]\)

Question 12.
If A is a 2 × 2 matrix such that A(adj A) = \(\left[\begin{array}{ll}
5 & 0 \\
0 & 5
\end{array}\right]\), then |A| = ___________
(a) 0
(b) 5
(c) 10
(d) 25
Answer:
(b) 5
Hint:
A(adj A) = |A|.I

Question 13.
If A is a non-singular matrix, then det(A^-1) = ___________
(a) 1
(b) 0
(c) det(A)
(d) 1/det(A)
Answer:
(d) 1/det(A)
Hint:
AA^-1 = I
∴ |A|.|A^-1| = 1
∴ |A^-1| = \(\frac{1}{|\mathrm{~A}|}\)

Question 14.
If A = \(\left[\begin{array}{cc}
1 & 2 \\
-3 & -1
\end{array}\right]\), B = \(\left[\begin{array}{rr}
-1 & 0 \\
1 & 5
\end{array}\right]\) then AB = ___________
(a) \(\left[\begin{array}{rr}
1 & -10 \\
1 & 20
\end{array}\right]\)
(b) \(\left[\begin{array}{rr}
1 & 10 \\
-1 & 20
\end{array}\right]\)
(c) \(\left[\begin{array}{ll}
1 & 10 \\
1 & 20
\end{array}\right]\)
(d) \(\left[\begin{array}{rr}
1 & 10 \\
-1 & -20
\end{array}\right]\)
Answer:
(c) \(\left[\begin{array}{ll}
1 & 10 \\
1 & 20
\end{array}\right]\)

Question 15.
If x + y + z = 3, x + 2y + 3z = 4, x + 4y + 9z = 6, then (y, z) = ___________
(a) (-1, 0)
(b) (1, 0)
(c) (1, -1)
(d) (-1, 1)
Answer:
(b) (1, 0)

(II) Fill in the blanks:

Question 1.
A = \(\left[\begin{array}{l}
3 \\
1
\end{array}\right]\) is ___________ matrix.
Answer:
column

Question 2.
Order of matrix \(\left[\begin{array}{lll}
2 & 1 & 1 \\
5 & 1 & 8
\end{array}\right]\) is ___________
Answer:
2 × 3

Question 3.
If A = \(\left[\begin{array}{ll}
4 & x \\
6 & 3
\end{array}\right]\) is a singular matrix, then x is ___________
Answer:
2

Question 4.
Matrix B = \(\left[\begin{array}{ccc}
0 & 3 & 1 \\
-3 & 0 & -4 \\
p & 4 & 0
\end{array}\right]\) is a skew-symmetric, then value of p is ___________
Answer:
-1

Question 5.
If A = [a_ij]_2×3 and B = [b_ij]_m×1, and AB is defined, then m = ___________
Answer:
3

Question 6.
If A = \(\left[\begin{array}{cc}
3 & -5 \\
2 & 5
\end{array}\right]\), then cofactor of a₁₂ is ___________
Answer:
-2

Question 7.
If A = [a_ij]_m×m is non-singular matrix, then A^-1 = \(\frac{1}{\ldots \ldots}\) adj (A).
Answer:
|A|

Question 8.
(A^T)^T = ___________
Answer:
A

Question 9.
If A = \(\left[\begin{array}{ll}
2 & 1 \\
1 & 1
\end{array}\right]\) and A^-1 = \(\left[\begin{array}{cc}
1 & -1 \\
x & 2
\end{array}\right]\), then x = ___________
Answer:
-1

Question 10.
If a₁x+ b₁y = c₁ and a₂x + b₂y = c₂, then matrix form is \(\left[\begin{array}{cc}
\cdots & \ldots \\
\cdots & \ldots
\end{array}\right]\left[\begin{array}{l}
x \\
y
\end{array}\right]=\left[\begin{array}{l}
\ldots \\
\cdots
\end{array}\right]\)
Answer:
\(\left[\begin{array}{ll}
a_{1} & b_{1} \\
a_{2} & b_{2}
\end{array}\right]\left[\begin{array}{l}
x \\
y
\end{array}\right]=\left[\begin{array}{l}
c_{1} \\
c_{2}
\end{array}\right]\)

(III) State whether each of the following is True or False:

Question 1.
Single element matrix is row as well as a column matrix.
Answer:
True

Question 2.
Every scalar matrix is unit matrix.
Answer:
False

Question 3.
A = \(\left[\begin{array}{ll}
4 & 5 \\
6 & 1
\end{array}\right]\) is non-singular matrix.
Answer:
True

Question 4.
If A is symmetric, then A = -A^T.
Answer:
False

Question 5.
If AB and BA both exist, then AB = BA.
Answer:
False

Question 6.
If A and B are square matrices of same order, then (A + B)² = A² + 2AB + B².
Answer:
False

Question 7.
If A and B are conformable for the product AB, then (AB)^T = A^TB^T.
Answer:
False

Question 8.
Singleton matrix is only row matrix.
Answer:
False

Question 9.
A = \(\left[\begin{array}{cc}
2 & 1 \\
10 & 5
\end{array}\right]\) is invertible matrix.
Answer:
False

Question 10.
A(adj A) = |A| I, where I is the unit matrix.
Answer:
True.

(IV) Solve the following:

Question 1.
Find k, if \(\left[\begin{array}{ll}
7 & 3 \\
5 & k
\end{array}\right]\) is a singular matrix.
Solution:
Let A = \(\left[\begin{array}{ll}
7 & 3 \\
5 & k
\end{array}\right]\)
Since, A is singular matrix, |A| = 0
∴ \(\left|\begin{array}{ll}
7 & 3 \\
5 & k
\end{array}\right|\) = 0
∴ 7k – 15 = 0
∴ k = \(\frac{15}{7}\)

Question 2.
Find x, y, z if \(\left[\begin{array}{lll}
2 & x & 5 \\
3 & 1 & z \\
y & 5 & 8
\end{array}\right]\) is a symmetric matrix.
Solution:

By equality of matrices,
x = 3, y = 5 and z = 5.

Question 3.
If A = \(\left[\begin{array}{ll}
1 & 5 \\
7 & 8 \\
9 & 5
\end{array}\right]\), B = \(\left[\begin{array}{cc}
2 & 4 \\
1 & 5 \\
-8 & 6
\end{array}\right]\), C = \(\left[\begin{array}{cc}
-2 & 3 \\
1 & -5 \\
7 & 8
\end{array}\right]\) then show that (A + B) + C = A + (B + C).
Solution:


From (1) and (2),
(A + B) + C = A + (B + C).

Question 4.
If A = \(\left[\begin{array}{ll}
2 & 5 \\
3 & 7
\end{array}\right]\), B = \(\left[\begin{array}{cc}
1 & 7 \\
-3 & 0
\end{array}\right]\), find the matrix A – 4B + 7I, where I is the unit matrix of order 2.
Solution:

Question 5.
If A = \(\left[\begin{array}{cc}
2 & -3 \\
3 & -2 \\
-1 & 4
\end{array}\right]\), B = \(\left[\begin{array}{ccc}
-3 & 4 & 1 \\
2 & -1 & -3
\end{array}\right]\) verify
(i) (A + 2B^T)^T = A^T + 2B
(ii) (3A – 5B^T)^T = 3A^T – 5B
Solution:

Question 6.
If A = \(\left[\begin{array}{lll}
1 & 2 & 3 \\
2 & 4 & 6 \\
1 & 2 & 3
\end{array}\right]\), B = \(\left[\begin{array}{ccc}
1 & -1 & 1 \\
-3 & 2 & -1 \\
-2 & 1 & 0
\end{array}\right]\) thenshow that AB and BA are both singular matrices.
Solution:


∴ BA is also a singular matrix.
Hence, AB and BA are both singular matrices.

Question 7.
If A = \(\left[\begin{array}{ll}
3 & 1 \\
1 & 5
\end{array}\right]\), B = \(\left[\begin{array}{cc}
1 & 2 \\
5 & -2
\end{array}\right]\), verify |AB| = |A| |B|.
Solution:

Question 8.
If A = \(\left[\begin{array}{cc}
2 & -1 \\
-1 & 2
\end{array}\right]\), then show that A² – 4A + 3I = 0.
Solution:

Question 9.
If A = \(\left[\begin{array}{cc}
-3 & 2 \\
2 & 4
\end{array}\right]\), B = \(\left[\begin{array}{ll}
1 & a \\
b & 0
\end{array}\right]\) and (A + B)(A – B) = A² – B², find a and b.
Solution:
(A + B)(A – B) = A² – B²
∴ A² – AB + BA – B² = A² – B²
∴ -AB + BA = 0
∴ AB = BA


By equality of matrices,
-3 + 2b = -3 + 2a ……..(1)
-3a = 2 + 4a ……..(2)
2 + 4b = -3b ……..(3)
2a = 2b ……..(4)
From (2), 7a = -2
∴ a = \(\frac{-2}{7}\)
From (3), 7b = -2
∴ b = \(\frac{-2}{7}\)
These values of a and b also satisfy equations (1) and (4).
Hence, a = \(\frac{-2}{7}\) and b = \(\frac{-2}{7}\)

Question 10.
If A = \(\left[\begin{array}{cc}
1 & 2 \\
-1 & 3
\end{array}\right]\), then find A³.
Solution:

Question 11.
Find x, y, z if \(\left\{5\left[\begin{array}{ll}
0 & 1 \\
1 & 0 \\
1 & 1
\end{array}\right]-\left[\begin{array}{cc}
2 & 1 \\
3 & -2 \\
1 & 3
\end{array}\right]\right\}\left[\begin{array}{l}
2 \\
1
\end{array}\right]=\left[\begin{array}{c}
x-1 \\
y+1 \\
2 z
\end{array}\right]\)
Solution:

∴ By equality of matrices, we get
x – 1 = 0 ∴ x = 1
y + 1 = 6 ∴ y = 5
2z = 10 ∴ z = 5

Question 12.
If A = \(\left[\begin{array}{cc}
2 & -4 \\
3 & -2 \\
0 & 1
\end{array}\right]\), B = \(\left[\begin{array}{ccc}
1 & -1 & 2 \\
-2 & 1 & 0
\end{array}\right]\) then showthat(AB)^T = B^TA^T.
Solution:

Question 13.
If A = \(\left[\begin{array}{lll}
1 & 0 & 0 \\
2 & 1 & 0 \\
3 & 3 & 1
\end{array}\right]\), then reduce it to unit matrix by row tranformation.
Solution:

Question 14.
Two farmers Shantaram and Kantaram cultivate three crops rice, wheat, and groundnut. The sale (in ₹) of these crops by both the farmers for the month of April and May 2016 is given below:

Find (i) the total sale in rupees for two months of each farmer for each crop.
(ii) the increase in sales from April to May for every crop of each farmer.
Solution:
The given information can be written in matrix form as:

(i) The total sale in ₹ for two months of each farmer for each crop can be obtained by the addition A + B.
Now, A + B

∴ total sale in ₹ for two months of each farmer for each crop is given by

Hence, the total sale for Shantaram are ₹ 33000 for Rice, ₹ 28000 for Wheat, ₹ 24000 for Groundnut, and for Kantaram are ₹ 39000 for Rice, ₹ 31500 for Wheat, ₹ 24000 for Groundnut.
(ii) The increase in sales from April to May for every crop of each farmer can be obtained by the subtraction of A from B.
Now, B – A

Hence, the increase in sales from April to May of Shantam is ₹ 3000 in Rice, ₹ 2000 in Wheat, nothing in Groundnut and of Kantaram are ₹ 3000 in Rice, ₹ 1500 in Wheat, ₹ 8000 in Groundnut.

Question 15.
Check whether following matrices are invertible or not:
(i) \(\left[\begin{array}{ll}
1 & 0 \\
0 & 1
\end{array}\right]\)
Solution:
Let A = \(\left[\begin{array}{ll}
1 & 0 \\
0 & 1
\end{array}\right]\)
Then |A| = \(\left|\begin{array}{ll}
1 & 0 \\
0 & 1
\end{array}\right|\)
= 1 – 0
= 1 ≠ 0
∴ A is a non-singular matrix.
Hence, A^-1 exists.

(ii) \(\left[\begin{array}{ll}
1 & 1 \\
1 & 1
\end{array}\right]\)
Solution:
Let A = \(\left[\begin{array}{ll}
1 & 1 \\
1 & 1
\end{array}\right]\)
Then |A| = \(\left|\begin{array}{ll}
1 & 1 \\
1 & 1
\end{array}\right|\)
= 1 – 1
= 0
∴ A is a singular matrix.
Hence, A^-1 does not exist.

(iii) \(\left[\begin{array}{lll}
3 & 4 & 3 \\
1 & 1 & 0 \\
1 & 4 & 5
\end{array}\right]\)
Solution:
Let A = \(\left[\begin{array}{lll}
3 & 4 & 3 \\
1 & 1 & 0 \\
1 & 4 & 5
\end{array}\right]\)
Then |A| = \(\left|\begin{array}{lll}
3 & 4 & 3 \\
1 & 1 & 0 \\
1 & 4 & 5
\end{array}\right|\)
= 3(5 – 0) – 4(5 – 0) + 3(4 – 1)
= 15 – 20 + 9
= 4 ≠ 0
∴ A is a non-singular matrix.
Hence, A^-1 exists.

(iv) \(\left[\begin{array}{lll}
1 & 2 & 3 \\
2 & 4 & 5 \\
2 & 4 & 6
\end{array}\right]\)
Solution:
Let A = \(\left[\begin{array}{lll}
1 & 2 & 3 \\
2 & 4 & 5 \\
2 & 4 & 6
\end{array}\right]\)
Then |A| = \(\left|\begin{array}{lll}
1 & 2 & 3 \\
2 & 4 & 5 \\
2 & 4 & 6
\end{array}\right|\)
= 1(24 – 20) – 2(12 – 10) + 3(8 – 8)
= 4 – 4 + 0
= 0
∴ A is a singular matrix.
Hence, A^-1 does not exist.

Question 16.
Find inverse of the following matrices (if they exist) by elementary transformation:
(i) \(\left[\begin{array}{cc}
1 & -1 \\
2 & 3
\end{array}\right]\)
Solution:

(ii) \(\left[\begin{array}{ll}
2 & 1 \\
7 & 4
\end{array}\right]\)
Solution:

(iii) \(\left[\begin{array}{ccc}
2 & -3 & 3 \\
2 & 2 & 3 \\
3 & -2 & 2
\end{array}\right]\)
Solution:

(iv) \(\left[\begin{array}{ccc}
2 & 0 & -1 \\
5 & 1 & 0 \\
0 & 1 & 3
\end{array}\right]\)
Solution:

Question 17.
Find the inverse of \(\left[\begin{array}{lll}
3 & 1 & 5 \\
2 & 7 & 8 \\
1 & 2 & 5
\end{array}\right]\) by adjoint method.
Solution:

Question 18.
Solve the following equations by method of inversion:
(i) 4x – 3y – 2 = 0, 3x – 4y + 6 = 0
Solution:
The given equations are
4x – 3y = 2
3x – 4y = -6
These equations can be written in matrix form as:




By equality of matrices,
x = \(\frac{26}{7}\), y = \(\frac{30}{7}\) is the required solution.

(ii) x + y – z = 2, x – 2y + z = 3 and 2x – y – 3z = -1
Solution:
The given equations can be written in matrix form as:




By equality of matrices,
x = 3, y = 1, z = 2 is the required solution.

(iii) x – y + z = 4, 2x + y – 3z = 0 and x + y + z = 2
Solution:

Question 19.
Solve the following equations by method of reduction:
(i) 2x + y = 5, 3x – 5y = -3
Solution:
The given equation can be written in matrix form as:
\(\left[\begin{array}{ll}
2 & 1 \\
3 & 5
\end{array}\right]\left[\begin{array}{l}
x \\
y
\end{array}\right]=\left[\begin{array}{r}
5 \\
-3
\end{array}\right]\)
By R₂ – 5R₁, we get

By equality of matrices,
2x + y = 5 …….(1)
-7x = -28 ……(2)
From (2), x = 4
Substituting x = 4 in (1), we get
2(4) + y = 5
∴ y = -3
Hence, x = 4 and y = -3 is the required solution.

(ii) x + 2y + z = 3, 3x – y + 2z = 1 and 2x – 3y + 3z = 2
Solution:
The given equations can be written in matrix form as:

By equality of matrices,
x + 2y + z = 3 …….(1)
-7y – z = -8 …….(2)
2z = 4 …….(3)
From (3), z = 2
Substituting z = 2 in (2), we get
-7y – 2 = -8
∴ -7y = -6
∴ y = \(\frac{6}{7}\)
Substituting y = \(\frac{6}{7}\), z = 2 in (1), we get
x + 2(\(\frac{6}{7}\)) + 2 = 3
x = 3 – 2 – \(\frac{12}{7}\) = \(\frac{-5}{7}\)
Hence, x = \(\frac{-5}{7}\), y = \(\frac{6}{7}\) and z = 2 is the required solution.

(iii) x – 3y + z = 2, 3x + y + z = 1 and 5x + y + 3z = 3.
Solution:

Question 20.
The sum of three numbers is 6. If we multiply the third number by 3 and add it to the second number, we get 11. By adding first and third numbers we get a number that is double the second number. Use this information and find a system of linear equations. Find the three numbers using matrices.
Solution:
Let the three numbers be x, y, and z.
According to the given condition,
x + y + z = 6
3z + y = 11, i.e. y + 3z = 11
and x + z = 2y, i.e. x – 2y + z = 0
Hence, the system of linear equations is
x + y + z = 6
y + 3z = 11
x – 2y + z = 0
These equations can be written in matrix form as:

By equality of matrices,
x + y + z = 6 …….(1)
y + 3z = 11 ………(2)
-3y = -6 ………(3)
From (3), y = 2
Substituting y = 2 in (2), we get
2 + 3z = 11
∴ 3z = 9
∴ z = 3
Substituting y = 2, z = 3 in (1), we get
x + 2 + 3 = 6
∴ x = 1
∴ x = 1, y = 2, z = 3
Hence, the required numbers are 1, 2 and 3.

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 2 Matrices Ex 2.1 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 2 Matrices Ex 2.1

Question 1.
Construct a matrix A = [a_ij]_3×2 whose elements aij isgiven by
(i) a_ij = \(\frac{(i-j)^{2}}{5-i}\)
Solution:

(ii) a_ij = i – 3j
Solution:
a_ij = i – 3j
∴ a₁₁ = 1 – 3(1) = 1 – 3 = -2
a₁₂ = 1 – 3(2) = 1 – 6 = -5
a₂₁ = 2 – 3(1) = 2 – 3 = -1
a₂₂ = 2 – 3(2) = 2 – 6 = -4
a₃₁ = 3 – 3(1) = 3 – 3 = 0
a₃₂ = 3 – 3(2) = 3 – 6 = -3
∴ A = \(\left[\begin{array}{cc}
-2 & -5 \\
-1 & -4 \\
0 & -3
\end{array}\right]\)

(iii) a_ij = \(\frac{(i+j)^{3}}{5}\)
Solution:

Question 2.
Classify each of the following matrices as a row, a column, a square, a diagonal, a scalar, a unit, an upper triangular, a lower triangular matrix:

Solution:
(i) Since, all the elements below the diagonal are zero, it is an upper triangular matrix.
(ii) This matrix has only one column, it is a column matrix.
(iii) This matrix has only one row, it is a row matrix.
(iv) Since, diagonal elements are equal and non-diagonal elements are zero, it is a scalar matrix.
(v) Since, all the elements above the diagonal are zero, it is a lower triangular matrix.
(vi) Since, all the non-diagonal elements are zero, it is a diagonal matrix.
(vii) Since, diagonal elements are 1 and non-diagonal elements are 0, it is an identity (or unit) matrix.

Question 3.
Which of the following matrices are singular or non-singular:
(i) \(\left[\begin{array}{ccc}
a & b & c \\
p & q & r \\
2 a-p & 2 b-q & 2 c-r
\end{array}\right]\)
Solution:

(ii) \(\left[\begin{array}{ccc}
5 & 0 & 5 \\
1 & 99 & 100 \\
6 & 99 & 105
\end{array}\right]\)
Solution:

(iii) \(\left[\begin{array}{ccc}
3 & 5 & 7 \\
-2 & 1 & 4 \\
3 & 2 & 5
\end{array}\right]\)
Solution:
Let C = \(\left[\begin{array}{ccc}
3 & 5 & 7 \\
-2 & 1 & 4 \\
3 & 2 & 5
\end{array}\right]\)
∴ |C| = \(\left|\begin{array}{rrr}
3 & 5 & 7 \\
-2 & 1 & 4 \\
3 & 2 & 5
\end{array}\right|\)
= 3(5 – 8) – 5(-10 – 12) + 7(-4 – 3)
= -9 + 110 – 49
= 52 ≠ 0
∴ C is a non-singular matrix.

(iv) \(\left[\begin{array}{cc}
7 & 5 \\
-4 & 7
\end{array}\right]\)
Solution:
Let D = \(\left[\begin{array}{cc}
7 & 5 \\
-4 & 7
\end{array}\right]\)
∴ |D| = \(\left|\begin{array}{rr}
7 & 5 \\
-4 & 7
\end{array}\right|\)
= 49 – (-20)
= 69 ≠ 0
∴ D is a non-singular matrix.

Question 4.
Find k, if the following matrices are singular:
(i) \(\left[\begin{array}{cc}
7 & 3 \\
-2 & K
\end{array}\right]\)
Solution:
Let A = \(\left[\begin{array}{cc}
7 & 3 \\
-2 & K
\end{array}\right]\)
Since, A is a singular matrix, |A| = 0
∴ \(\left|\begin{array}{rr}
7 & 3 \\
-2 & k
\end{array}\right|\) = 0
∴ 7k – (-6) = 0
∴ 7k = -6
∴ k = \(-\frac{6}{7}\)

(ii) \(\left[\begin{array}{ccc}
4 & 3 & 1 \\
7 & \mathrm{~K} & 1 \\
10 & 9 & 1
\end{array}\right]\)
Solution:
Let B = \(\left[\begin{array}{ccc}
4 & 3 & 1 \\
7 & \mathrm{~K} & 1 \\
10 & 9 & 1
\end{array}\right]\)
Since, B is a singular matrix, |B| = 0
∴ \(\left|\begin{array}{rrr}
4 & 3 & 1 \\
7 & k & 1 \\
10 & 9 & 1
\end{array}\right|\) = 0
∴ 4(k – 9) – 3(7 – 10) + 1(63 – 10k) = 0
∴ 4k – 36 + 9 + 63 – 10k = 0
∴ -6k + 36 = 0
∴ 6k = 36
∴ k = 6.

(iii) \(\left[\begin{array}{ccc}
K-1 & 2 & 3 \\
3 & 1 & 2 \\
1 & -2 & 4
\end{array}\right]\)
Solution:
Let C = \(\left[\begin{array}{ccc}
K-1 & 2 & 3 \\
3 & 1 & 2 \\
1 & -2 & 4
\end{array}\right]\)
Since, C is a singular matrix, |C| = 0
∴ \(\left|\begin{array}{crr}
k-1 & 2 & 3 \\
3 & 1 & 2 \\
1 & -2 & 4
\end{array}\right|\) = 0
∴ (k – 1)(4 + 4) – 2(12 – 2) + 3(-6 – 1) = 0
∴ 8k – 8 – 20 – 21 = 0
∴ 8k = 49
∴ k = \(\frac{49}{8}\)

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 2 Matrices Ex 2.6 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 2 Matrices Ex 2.6

Question 1.
Solve the following equations by the method of inversion:
(i) x + 2y = 2, 2x + 3y = 3
Solution:
The given equations can be written in the matrix form as:



By equality of matrices,
x = 0, y = 1 is the required solution.

(ii) 2x + y = 5, 3x + 5y = -3
Solution:

(iii) 2x – y + z = 1, x + 2y + 3z = 8 and 3x + y – 4z = 1
Solution:
The given equations can be written in the matrix form as:






By equality of matrices,
x = 1, y = 2, z = 1 is the required solution.

(iv) x + y + z = 1, x – y + z = 2 and x + y – z = 3
Solution:

Question 2.
Express the following equations in matrix form and solve them by method of reduction:
(i) x + 3y = 2, 3x + 5y = 4.
Solution:
The given equations can be written in the matrix form as:


Hence, x = \(\frac{1}{2}\), y = \(\frac{1}{2}\) is the required solution.

(ii) 3x – y = 1, 4x + y = 6.
Solution:
The given equations can be written in the matrix form as:

By equality of matrices,
12x – 4y = 4 …..(1)
7y = 14 …..(2)
From (2), y = 2
Substituting y = 2 in (1), we get
12x – 8 = 4
∴ 12x = 12
∴ x = 1
Hence, x = 1, y = 2 is the required solution.

(iii) x + 2y + z = 8, 2x + 3y – z = 11 and 3x – y – 2z = 5.
Solution:
The given equations can be written in the matrix form as:


By equality of matrices,
x + 2y + z = 8 ……(1)
-y – 3z = -5 …….(2)
16z = 16 ……….(3)
From (3), z = 1
Substituting z = 1 in (2), we get
-y – 3= -5
∴ y = 2
Substituting y = 2, z = 1 in (1), we get
x + 4 + 1 = 8
∴ x = 3
Hence, x = 3, y = 2, z = 1 is the required solution.

(iv) x + y + z = 1, 2x + 3y + 2z = 2 and x + y + 2z = 4.
Solution:
The given equations can be written in the matrix form as:

By equality of matrices,
x + y + z = 1 ……(1)
y = 0
z = 3
Substituting y = 0, z = 3 in (1), we get
x + 0 + 3 = 1
∴ x = -2
Hence, x = -2, y = 0, z = 3 is the required solution.

Question 3.
The total cost of 3 T.V. and 2 V.C.R. is ₹ 35000. The shopkeeper wants a profit of ₹ 1000 per T.V. and ₹ 500 per V.C.R. He sells 2 T.V. and 1 V.C.R. and he gets total revenue of ₹ 21500. Find the cost and selling price of T.V. and V.C.R.
Solution:
Let the cost of each T.V. be ₹ x and each V.C.R. be ₹ y.
Then the total cost of 3 T.V. and 2 V.C.R. is ₹ (3x + 2y) which is given to be ₹ 35000.
∴ 3x + 2y = 35000
The shopkeeper wants a profit of ₹ 1000 per T.V. and ₹ 500 per V.C.R.
The selling price of each T.V. is ₹ (x + 1000) and of each V.C.R. is ₹ (y + 500).
∴ selling price of 2 T.V. and 1 V.C.R is
₹ [2(x + 1000) + (y + 500)] which is given to be ₹ 21500.
∴ 2(x + 1000) + (y + 500) = 21500
∴ 2x + 2000 + y + 500 = 21500
∴ 2x + y = 19000
Hence, the system of linear equations is
3x + 2y = 35000
2x + y = 19000
The equations can be written in matrix form as:

By equality of matrices,
-x = -3000 …….(1)
2x + y = 19000 ……….(2)
From (1), x = 3000
Substituting x = 3000 in (2), we get
2(3000) + y = 19000
∴ y = 19000 – 6000 = 13000
Hence, the cost price of one T.V. is ₹ 3000 and of one V.C.R. is ₹ 13000 and the selling price of one T.V. is ₹ 4000 and of one V.C.R. is ₹ 13500.

Question 4.
The sum of the cost of one Economics book, one Cooperation book, and one Account book is ₹ 420. The total cost of an Economic book, 2 Cooperation books, and an Account book is ₹ 480. Also, the total cost of an Economic book, 3 Cooperation books, and 2 Account books is ₹ 600. Find the cost of each book.
Solution:
Let the cost of 1 Economic book, 1 Cooperation book and 1 Account book be ₹ x, ₹ y and ₹ z respectively.
Then, from the given information
x + y + z = 420
x + 2y + z = 480
x + 3y + 2z = 600
These equations can be written in matrix form as:

By equality of matrices,
x + y + z = 420 …….(1)
y = 60
2y + z = 180 ………(2)
Substituting y = 60 in (2), we get
2(60) + z = 180
∴ z = 180 – 120 = 60
Substituting y = 60, z = 60 in (1), we get
x + 60 + 60 = 420
∴ x = 420 – 120 = 300
Hence, the cost of each Economic book is ₹ 300, each Cooperation book is ₹ 60 and each Account book is ₹ 60.

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 2 Matrices Ex 2.5 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 2 Matrices Ex 2.5

Question 1.
Apply the given elementary transformation on each of the following matrices:
(i) \(\left[\begin{array}{cc}
3 & -4 \\
2 & 2
\end{array}\right]\), R₁ ↔ R₂
(ii) \(\left[\begin{array}{cc}
2 & 4 \\
1 & -5
\end{array}\right]\), C₁ ↔ C₂
(iii) \(\left[\begin{array}{ccc}
3 & 1 & -1 \\
1 & 3 & 1 \\
-1 & 1 & 3
\end{array}\right]\) 3R₂ and C₂ → C₂ – 4C₁
Solution:

Question 2.
Transform \(\left[\begin{array}{ccc}
1 & -1 & 2 \\
2 & 1 & 3 \\
3 & 2 & 4
\end{array}\right]\) into an upper triangularmatrix by suitable row transformations.
Solution:

Question 3.
Find the cofactor matrix of the following matrices:
(i) \(\left[\begin{array}{cc}
1 & 2 \\
5 & -8
\end{array}\right]\)
(ii) \(\left[\begin{array}{ccc}
5 & 8 & 7 \\
-1 & -2 & 1 \\
-2 & 1 & 1
\end{array}\right]\)
Solution:

Question 4.
Find the adjoint of the following matrices:
(i) \(\left[\begin{array}{cc}
2 & -3 \\
3 & 5
\end{array}\right]\)
(ii) \(\left[\begin{array}{ccc}
1 & -1 & 2 \\
-2 & 3 & 5 \\
-2 & 0 & -1
\end{array}\right]\)
Solution:

Question 5.
Find the inverses of the following matrices by the adjoint mathod:
(i) \(\left[\begin{array}{rr}
3 & -1 \\
2 & -1
\end{array}\right]\)
(ii) \(\left[\begin{array}{cc}
2 & -2 \\
4 & 5
\end{array}\right]\)
(iii) \(\left[\begin{array}{lll}
1 & 2 & 3 \\
0 & 2 & 4 \\
0 & 0 & 5
\end{array}\right]\)
Solution:

Question 6.
Find the inverses of the following matrices by the transformation method:
(i) \(\left[\begin{array}{cc}
1 & 2 \\
2 & -1
\end{array}\right]\)
(ii) \(\left[\begin{array}{ccc}
2 & 0 & -1 \\
5 & 1 & 0 \\
0 & 1 & 3
\end{array}\right]\)
Solution:

Question 7.
Find the inverse of A = \(\left[\begin{array}{lll}
1 & 0 & 1 \\
0 & 2 & 3 \\
1 & 2 & 1
\end{array}\right]\) by elementary column transformations.
Solution:

Question 8.
Find the inverse of \(\left[\begin{array}{lll}
1 & 2 & 3 \\
1 & 1 & 5 \\
2 & 4 & 7
\end{array}\right]\) by the elementary row transformations.
Solution:

Question 9.
If A = \(\left[\begin{array}{lll}
1 & 0 & 1 \\
0 & 2 & 3 \\
1 & 2 & 1
\end{array}\right]\) and B = \(\left[\begin{array}{lll}
1 & 2 & 3 \\
1 & 1 & 5 \\
2 & 4 & 7
\end{array}\right]\), then find matrix X such that XA = B.
Solution:

Question 10.
Find matrix X, if AX = B, where A = \(\left[\begin{array}{ccc}
1 & 2 & 3 \\
-1 & 1 & 2 \\
1 & 2 & 4
\end{array}\right]\) and B = \(\left[\begin{array}{l}
1 \\
2 \\
3
\end{array}\right]\)
Solution:

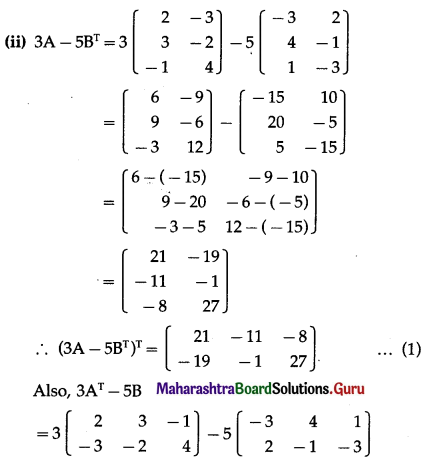
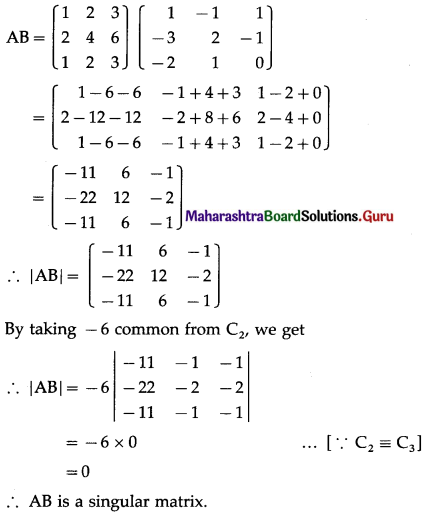
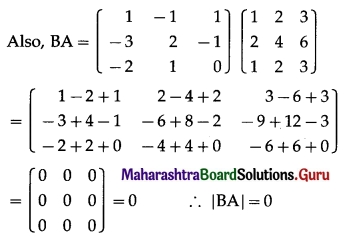
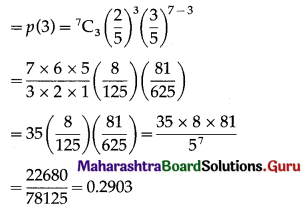
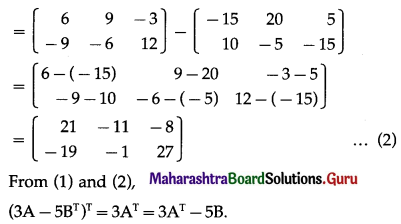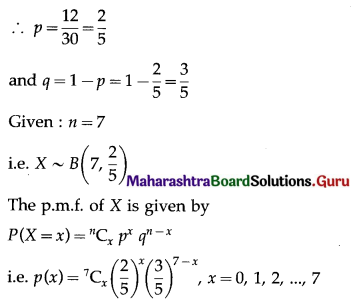Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 1 Mathematical Logic Miscellaneous Exercise 1 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 1 Mathematical Logic Miscellaneous Exercise 1

(I) Choose the correct alternative:

Question 1.
Which of the following is not a statement?
(a) Smoking is injurious to health
(b) 2 + 2 = 4
(c) 2 is only even prime number
(d) Come here
Answer:
(d) Come here

Question 2.
Which of the following is an open statement?
(a) x is a natural number
(b) Give me a glass of water
(c) Wish you best of luck
(d) Good morning to all
Answer:
(a) x is a natural number

Question 3.
Let p ∧ (q ∨ r) = (p ∧ q) ∨ (p ∧ r). Then this law is known as
(a) Commutative law
(b) Associative law.
(c) De Morgan’s law
(d) Distributive law
Answer:
(d) Distributive law

Question 4.
The false statement in the following is:
(a) p ∧ (~p) is a contradiction
(b) (p → q) ↔ (~q → ~p) is a contradiction
(c) ~(~p) ↔ p is a tautology
(d) p ∨ (~p) ↔ p is a tautology.
Answer:
(b) (p → q) ↔ (~q → ~p) is a contradiction

Question 5.
Consider the following three statements
p : 2 is an even number.
q : 2 is a prime number.
r : Sum of two prime numbers is always even.
Then, the symbolic statement (p ∧ q) → ~r means:
(a) 2 is an even and prime number and the sum of two prime numbers is always even.
(b) 2 is an even and prime number and the sum of two prime numbers is not always even.
(c) If 2 is an even and prime number, then the sum of two prime numbers is not always even.
(d) If 2 is an even and prime number, then the sum of two prime numbers is also even.
Answer:
(c) If 2 is an even and prime number, then the sum of two prime numbers is not always even.

Question 6.
If p : He is intelligent.
q : He is strong.
Then, symbolic form of statement: ‘It is wrong that, he is intelligent or strong’ is
(a) ~p ∨ ~p
(b) ~(p ∧ q)
(c) ~(p ∨ q)
(d) p ∨ ~q
Answer:
(c) ~(p ∨ q)

Question 7.
The negation of the proposition ‘If 2 is prime, then 3 is odd’, is
(a) If 2 is not prime, then 3 is not odd
(b) 2 is prime and 3 is not odd
(c) 2 is not prime and 3 is odd
(d) If 2 is not prime, then 3 is odd
Answer:
(b) 2 is prime and 3 is not odd

Question 8.
The statement (~p ∧ q) ∨ ~q is
(a) p ∨ q
(b) p ∧ q
(c) ~(p ∨ q)
(d) ~(p ∧ q)
Answer:
(d) ~(p ∧ q)
Hint:
(~p ∧ q) ∨ ~q = (~p ∨ ~q) ∧ (q ∨ ~q)
= (~p ∨ ~q) ∧ t
= ~p ∨ ~q
= ~(p ∧ q)

Question 9.
Which of the following is always true?
(a) ~(p → q) ≡ ~q → ~p
(b) ~(p ∨ q) ≡ ~p ∨ ~q
(c) ~(p → q) ≡ p ∧ ~q
(d) ~(p ∧ q) ≡ ~p ∧ ~q
Answer:
(c) ~(p → q) ≡ p ∧ ~q

Question 10.
~(p ∨ q) ∨ (~p ∧ q) is logically equivalent to
(a) ~p
(b) p
(c) q
(d) ~q
Answer:
(a) ~p
Hint:
~(p ∨ q) ∨ (~p ∧ q) ≡ (~p ∧ ~q) ∨ (~p ∧ q)
≡ ~p ∧ (~q ∨ q)
≡ ~p ∧ t
≡ ~p

Question 11.
If p and q are two statements, then (p → q) ↔ (~q → ~p) is
(a) contradiction
(b) tautology
(c) neither (a) nor (b)
(d) none of these
Answer:
(b) tautology

Question 12.
If p is the sentence ‘This statement is false’, then
(a) truth value of p is T
(b) truth value of p is F
(c) p is both true and false
(d) p is neither true nor false
Answer:
(d) p is neither true nor false

Question 13.
Conditional p → q is equivalent to
(a) p → ~q
(b) ~p ∨ q
(c) ~p → ~q
(d) p ∨ ~q
Answer:
(b) ~p ∨ q

Question 14.
Negation of the statement ‘This is false or That is true’ is
(a) That is true or This is false
(b) That is true and This is false
(c) This is true and That is false
(d) That is false and That is true
Answer:
(c) This is true and That is false

Question 15.
If p is any statement, then (p ∨ ~p) is a
(a) contingency
(b) contradiction
(c) tautology
(d) none of them
Answer:
(c) tautology

(II) Fill in the blanks:

Question 1.
The statement q → p is called as the ___________ of the statement p → q.
Answer:
Converse

Question 2.
Conjunction of two statements p and q is symbolically written as
Answer:
p ∧ q

Question 3.
If p ∨ q is true, then truth value of ~p ∨ ~q is ___________
Answer:
False

Question 4.
Negation of ‘some men are animal’ is ___________
Answer:
All men are not animal.
OR
No men are animals.

Question 5.
Truth value of if x = 2, then x² = -4 is ___________
Answer:
False

Question 6.
Inverse of statement pattern p → q is given by ___________
Answer:
~p → ~q

Question 7.
p ↔ q is false when p and q have ___________ truth values.
Answer:
Different

Question 8.
Let p : The problem is easy. r : It is not challenging. Then verbal form of ~p → r is ___________
Answer:
If the problem is not easy, then it is not challenging.

Question 9.
Truth value of 2 + 3 = 5 if and only if -3 > -9 is ___________
Answer:
T [Hint: T ↔ T = T]

(III) State whether each of the following is True or False:

Question 1.
Truth value of 2 + 3 < 6 is F.
Answer:
False

Question 2.
There are 24 months in a year is a statement.
Answer:
True

Question 3.
p ∧ q has truth value F if both p and q have truth value F.
Answer:
False

Question 4.
The negation of 10 + 20 = 30 is, it is false that 10 + 20 ≠ 30.
Answer:
False

Question 5.
Dual of (p ∧ ~q) ∨ t is (p ∨ ~q) ∨ c.
Answer:
False

Question 6.
Dual of ‘John and Ayub went to the forest’ is ‘John or Ayub went to the forest.’
Answer:
True

Question 7.
‘His birthday is on 29th February’ is not a statement.
Answer:
True

Question 8.
x² = 25 is true statement.
Answer:
False

Question 9.
The truth value of ‘√5 is not an irrational number’ is T.
Answer:
False

Question 10.
p ∧ t = p.
Answer:
True

(IV) Solve the following:

Question 1.
State which of the following sentences are statements in logic:
(i) Ice cream Sundaes are my favourite.
Solution:
It is a statement.

(ii) x + 3 = 8, x is variable.
Solution:
It is a statement.

(iii) Read a lot to improve your writing skill.
Solution:
It is an imperative sentence, hence it is not a statement.

(iv) z is a positive number.
Solution:
It is an open sentence, hence it is not a statement.

(v) (a + b)² = a² + 2ab + b² for all a, b ∈ R.
Solution:
It is a statement.

(vi) (2 + 1)² = 9.
Solution:
It is a statement.

(vii) Why are you sad?
Solution:
It is an interrogative sentence, hence it is not a statement.

(viii) How beautiful the flower is!
Solution:
It is an exclamatory sentence, hence it is not a statement.

(ix) The square of any odd number is even.
Solution:
It is a statement.

(x) All integers are natural numbers.
Solution:
It is a statement.

(xi) If x is a real number, then x² ≥ 0.
Solution:
It is a statement.

(xii) Do not come inside the room.
Solution:
It is an imperative sentence, hence it is not a statement.

(xiii) What a horrible sight it was!
Solution:
It is an exclamatory sentence, hence it is not a statement.

Question 2.
Which of the following sentences are statements? In case of a statement, write down the truth value:
(i) What is a happy ending?
Solution:
It is an interrogative sentence, hence it is not a statement.

(ii) The square of every real number is positive.
Solution:
It is a statement that is false, hence its truth value is F.

(iii) Every parallelogram is a rhombus.
Solution:
It is a statement that is true, hence its truth value is T.

(iv) a² – b² = (a + b)(a – b) for all a, b ∈ R.
Solution:
It is a mathematical identity that is true, hence its truth value is T.

(v) Please carry out my instruction.
Solution:
It is an imperative sentence, hence it is not a statement.

(vi) The Himalayas is the highest mountain range.
Solution:
It is a statement that is true, hence its truth value is T.

(vii) (x – 2)(x – 3) = x² – 5x + 6 for all x ∈ R.
Solution:
It is a mathematical identity that is true, hence its truth value is T.

(viii) What are the causes of rural unemployment?
Solution:
It is an interrogative sentence, hence it is not a statement.

(ix) 0! = 1.
Solution:
It is a statement that is true, hence its truth value is T.

(x) The quadratic equation ax² + bx + c = 0 (a ≠ 0) always has two real roots.
Solution:
It is a statement that is false, hence its truth value is F.

Question 3.
Assuming the first statement as p and second as q, write the following statements in symbolic form:
(i) The Sun has set and Moon has risen.
Solution:
Let p : The Sun has set.
q : Moon has risen.
Then the symbolic form of the given statement is p ∧ q.

(ii) Mona likes Mathematics and Physics.
Solution:
Let p : Mona likes Mathematics.
q : Mona likes Physics.
Then the symbolic form of the given statement is p ∧ q.

(iii) 3 is a prime number if 3 is a perfect square number.
Solution:
Let p : 3 be a prime number.
q : 3 is a perfect square number.
Then the symbolic form of the given statement is p ↔ q.

(iv) Kavita is brilliant and brave.
Solution:
Let p : Kavita is brilliant.
q : Kavita is brave.
Then the symbolic form of the given statement is p ∧ q.

(v) If Kiran drives a car, then Sameer will walk.
Solution:
Let p : Kiran drives a car.
q : Sameet will walk.
Then the symbolic form of the given statement is p → q.

(vi) The necessary condition for the existence of a tangent to the curve of the function is continuity.
Solution:
The given statement can be written as:
‘If the function is continuous, then the tangent to the curve exists.’
Let p : The function is continuous.
q : Tangent to the curve exists.
Then the symbolic form of the given statement is p → q.

(vii) To be brave is necessary and sufficient condition to climb Mount Everest.
Solution:
Let p : To be brave.
q : Climb Mount Everest.
Then the symbolic form of the given statement is p ↔ q.

(viii) x³ + y³ = (x + y)³, iff xy = 0.
Solution:
Let p : x³ + y³ = (x + y)³.
q : xy = 0.
Then the symbolic form of the given statement is p ↔ q.

(ix) The drug is effective though it has side effects.
Solution:
Let p : The drug is effective.
q : It has side effects.
Then the symbolic form of the given statement is p ∧ q.

(x) If a real number is not rational, then it must be irrational.
Solution:
Let p : A real number is not rational.
q : It must be irrational.
Then the symbolic form of the given statement is p → q.

(xi) It is not true that Ram is tall and handsome.
Solution:
Let p : Ram is tall.
q : Ram is handsome.
Then the symbolic form of the given statement is ~(p ∧ q).

(xii) Even though it is not cloudy, it is still raining.
Solution:
The given statement is equivalent to:
It is not cloudy and it is still raining,
Let p : It is not cloudy.
q : It is still raining.
Then the symbolic form of the given statement is p ∧ q.

(xiii) It is not true that intelligent persons are neither polite nor helpful.
Solution:
Let p : Intelligent persons are neither polite nor helpful.
Then the symbolic form of the given statement is ~p.

(xiv) If the question paper is not easy, then we shall not pass.
Solution:
Let p : The question paper is not easy.
q : We shall not pass.
Then the symbolic form of the given statement is p → q.

Question 4.
If p : Proof is lengthy.
q : It is interesting.
Express the following statements in symbolic form:
(i) Proof is lengthy and it is not interesting.
(ii) If the proof is lengthy, then it is interesting.
(iii) It is not true that the proof is lengthy but it is interesting.
(iv) It is interesting iff the proof is lengthy.
Solution:
The symbolic form of the given statements are:
(i) p ∧ ~q
(ii) p → q
(iii) ~(p ∧ q)
(iv) q ↔ p

Question 5.
Let p : Sachin win the match.
q : Sachin is a member of the Rajya Sabha.
r : Sachin is happy.
Write the verbal statement for each of the following:
(i) (p ∧ q) ∨ r
Solution:
Sachin wins the match and he is a member of the Rajya Sabha or Sachin is happy.

(ii) p → r
Solution:
If Sachin wins the match, then he is happy.

(iii) ~p ∨ q
Solution:
Sachin does not win the match or he is a member of the Rajya Sabha.

(iv) p → (q ∨ r)
Solution:
If Sachin wins the match, then he is a member of the Rajya Sabha or he is happy.

(v) p → q
Solution:
If Sachin wins the match, then he is a member of the Rajya Sabha.

(vi) (p ∧ q) ∧ ~r
Solution:
Sachin wins the match and he is a member of the Rajya Sabha but he is not happy.

(vii) ~(p ∨ q) ∧ r
Solution:
It is false that Sachin wins the match or he is a member of the Rajya Sabha but he is happy.

Question 6.
Determine the truth values of the following statements:
(i) 4 + 5 = 7 or 9 – 2 = 5.
Solution:
Let p : 4 + 5 = 7.
q : 9 – 2 = 5.
Then the symbolic form of the given statement is p ∨ q.
The truth values of both p and q are F.
∴ the truth value of p ∨ q is F. …….[F ∨ F ≡ F]

(ii) If 9 > 1, then x² – 2x + 1 = 0 for x = 1.
Solution:
Let p : 9 > 1.
q : x² – 2x + 1 = 0 for x = 1.
Then the symbolic form of the given statement is p → q.
The truth values of both p and q are T.
∴ the truth value of p → q is T. …..[T → T ≡ T]

(iii) x + y = 0 is the equation of a straight line if and only if y² = 4x is the equation of the parabola.
Solution:
Let p : x + y = 0 is the equation of a straight line.
q : y² = 4x is the equation of the parabola.
Then the symbolic form of the given statement is p ↔ q.
The truth values of both p and q are T.
∴ the truth value of p ↔ q is T. …..[T ↔ T ≡ T]

(iv) It is not true that 2 + 3 = 6 or 12 + 3 = 5.
Solution:
Let p : 2 + 3 = 6.
q : 12 + 3 = 5.
Then the symbolic form of the given statement is ~(p ∨ q).
The truth values of both p and q are F.
∴ the truth value of ~(p ∨ q) is T. …..[~(F ∨ F) ≡ ~F ≡ T]

Question 7.
Assuming the following statements
p : Stock prices are high.
q : Stocks are rising.
to be true, find the truth values of the following:

(i) Stock prices are not high or stocks are rising.
Solution:
p and q are true, i.e. T.
∴ ~p and ~q are false, i.e. F.
The given statement in symbolic form is ~p ∨ q.
Since, ~T ∨ T ≡ F ∨ T ≡ T, the given statement is true.
Hence, its truth value is ‘T’.

(ii) Stock prices are high and stocks are rising if and only if stock prices are high.
Solution:
The given statement in symbolic form is (p ∧ q) ↔ p.
Since (T ∧ T) ↔ T ≡ T ↔ T ≡ T, the given statement is true.
Hence, its truth value is ‘T’.

(iii) If stock prices are high, then stocks are not rising.
Solution:
The given statement in symbolic form is p → ~q.
Since, T → ~T ≡ T → F ≡ F, the given statement is false.
Hence, its truth value is ‘F’.

(iv) It is false that stocks are rising and stock prices are high.
Solution:
The given statement in symbolic form is ~(q ∧ p).
Since, ~(T ∧ T) ≡ ~T ≡ F, the given statement is false.
Hence, its truth value is ‘F’.

(v) Stock prices are high or stocks are not rising iff stocks are rising.
Solution:
The given statement in symbolic form is (p ∨ ~q) ↔ q.
Since (T ∨ ~T) ↔ T ≡ (T ∨ F) ↔ T
≡ T ↔ T
≡ T, the given statement is true.
Hence, its truth value is ‘T’.

Question 8.
Rewrite the following statements without using conditional:
[Hint: P → q ≡ ~p ∨ q]
(i) If price increases, then demand falls.
(ii) If demand falls, then the price does not increase.
Solution:
Since, p → q ≡ ~p ∨ q, the given statements can be written as:
(i) Price does not increase or demand falls.
(ii) Demand does not fall or price does not increase.

Question 9.
If p, q, r are statements with truth values T, T, F respectively, determine the truth values of the following:
(i) (p ∧ q) → ~p
Solution:
Truth values of p, q, r are T, T, F respectively.
(p ∧ q) → ~p ≡ (T ∧ T) → ~T
≡ T → F
≡ F
Hence, the truth value of the given statement is false, i.e. F.

(ii) p ↔ (q → ~p)
Solution:
p ↔ (q → ~p) ≡ T ↔ (T → ~T)
≡ T ↔ (T → F)
≡ T ↔ F
≡ F
Hence, the truth value of the given statement is false, i.e. F.

(iii) (p ∧ ~q) ∨ (~p ∧ q)
Solution:
(p ∧ ~q) ∨ (~p ∧ q) ≡ (T ∧ ~T) ∨ (~T ∧ T)
≡ (T ∧ F) ∨ (F ∧ T)
≡ F ∨ F
≡ F
Hence, the truth value of the given statement is false, i.e. F.

(iv) ~(p ∧ q) → ~(q ∧ p)
Solution:
~(p ∧ q) → ~(q ∧ p) ≡ ~(T ∧ T) → ~(T ∧ T)
≡ ~T → ~T
≡ F → F
≡ T
Hence, the truth value of the given statement is true, i.e. T.

(v) ~[(p → q) ↔ (p ∧ ~q)]
Solution:
~[(p → q) ↔ (p ∧ ~q)]
≡ ~[(T → T) ↔ (T ∧ ~T)]
≡ ~[T ↔ (T ∧ F)]
≡ ~[T ↔ F]
≡ ~F
≡ T.
Hence, the truth value of the given statement is true, i.e. T.

Question 10.
Write the negations of the following:
(i) If ΔABC is not equilateral, then it is not equiangular.
Solution:
Let p : ΔABC is not equilateral.
q : It is not equiangular.
Then the symbolic form of the given statement is p → q.
Since, ~(p → q) ≡ p ∧ ~q, the negation of the given statement is:
‘ΔABC is not equilateral and it is equiangular.’

(ii) Ramesh is intelligent and he is hard working.
Solution:
Let p : Ramesh is intelligent.
q : He is hard working.
Then the symbolic form of the given statement is p ∧ q.
Since, ~(p ∧ q) ≡ ~p ∨ ~q, the negation of the given statement is:
‘Ramesh is not intelligent or he is not hard-working.’

(iii) A angle is a right angle if and only if it is of measure 90°.
Solution:
Let p : An angle is a right angle.
q : It is of measure 90°.
Then the symbolic form of the given statement is p ↔ q.
Since, ~(p ↔ q) ≡ (p ∧ ~q) ∨ (q ∧ ~p), the negation of the given statement is:
‘An angle is a right angle and it is not of measure 90° or an angle is of measure 90° and it is not a right angle.’

(iv) Kanchanjunga is in India and Everest is in Nepal.
Solution:
Let p : Kanchenjunga is in India.
q : Everest is in Nepal.
Then the symbolic form of the given statement is p ∧ q.
Since, ~(p ∧ q) ≡ ~p ∨ ~q, the negation of the given statement is:
‘Kanchenjunga is not in India or Everest is not in Nepal.’

(v) If x ∈ A ∩ B, then x ∈ A and x ∈ B.
Solution:
Let p : x ∈ A ∩ B, q : x ∈ A, r : x ∈ B.
Then the symbolic form of the given statement is P → (q ∧ r)
Since, ~(p → q) ≡ p ∧ ~q and ~(p ∧ q)= ~p ∨ ~q,
the negation of the given statement is:
‘x ∈ A ∩ B and x ∉ A or x ∉ B.

Question 11.
Construct the truth table for each of the following statement patterns:
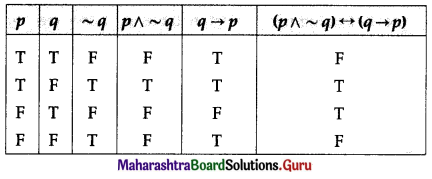
(i) (p ∧ ~q) ↔ (q → p)
Solution:
(p ∧ ~q) ↔ (q → p)

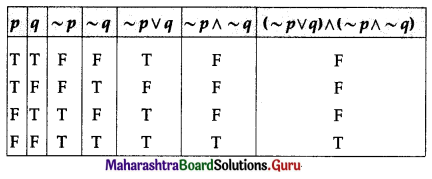
(ii) (~p ∨ q) ∧ (~p ∧ ~q)
Solution:
(~p ∨ q) ∧ (~p ∧ ~q)

(iii) (p ∧ r) → (p ∨ ~q)
Solution:
(p ∧ r) → (p ∨ ~q)

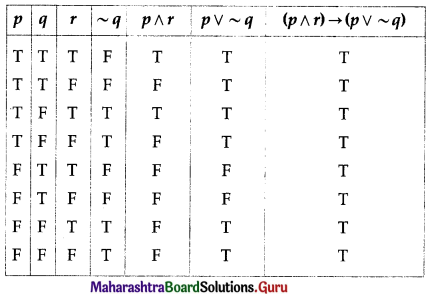
(iv) (p ∨ r) → ~(q ∧ r)
Solution:
(p ∨ r) → ~(q ∧ r)

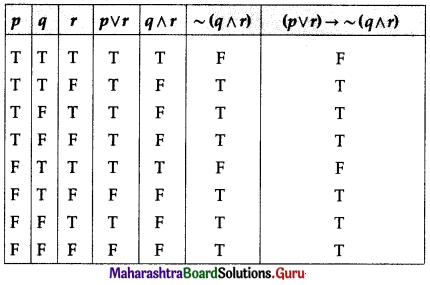
(v) (p ∨ ~q) → (r ∧ p)
Solution:
(p ∨ ~q) → (r ∧ p)

Question 12.
What is a tautology? What is a contradiction? Show that the negation of a tautology is a contradiction and the negation of a contradiction is a tautology.
Solution:
Tautology: A statement pattern that has all the entries in the last column of its truth table as T is called a tautology.
For example:

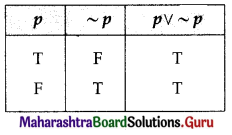
In the above truth table for the statement p ∨ ~p,
we observe that all the entries in the last column are T.
Hence, the statement p ∨ ~p is a tautology.

Contradiction: A statement pattern that has all the entries in the last column of its truth table as F is called a contradiction.
For example:

In the above truth table for the statement p ∧ ~p,
we observe that all the entries in the last column are F.
Hence, the statement p ∧ ~p is a contradiction.

To show that the negation of a tautology is a contradiction and vice versa:
A tautology is true on every row of its truth table.
Since, ~T = F and ~F = T, when we negate a tautology, the resulting statement is false on every row of its table.
i.e. the negation of tautology is a contradiction.
Similarly, the negation of a contradiction is a tautology.

Question 13.
Determine whether the following statement patterns is a tautology or a contradiction or a contingency:
(i) [(p ∧ q) ∨ (~p)] ∨ [p ∧ (~q)]
Solution:
[(p ∧ q) ∨ (~p)] ∨ [p ∧ (~q)]

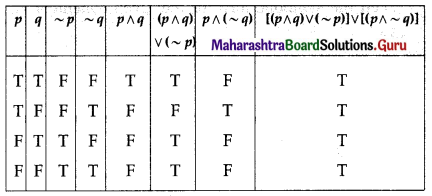
All the entries in the last column of the above truth table are T.
∴ [(p ∧ q) ∨ (~p)] ∨ [p ∧ (~q)] is a tautology.

(ii) [(~p ∧ q) ∧ (q ∧ r)] ∨ (~q)
Solution:
[(~p ∧ q) ∧ (q ∧ r)] ∨ (~q)

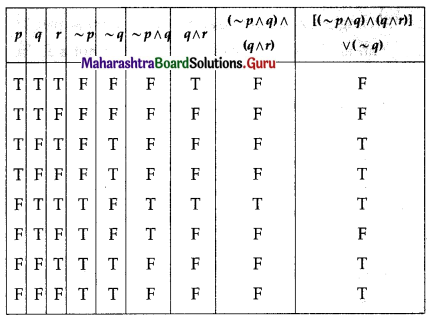
The entries in the last column of the above truth table are neither all T nor all F.
∴ [(~p ∧ q) ∧ (q ∧ r)] ∨ (~q) is a contingency.

(iii) [~(p ∨ q) → p] ↔ [(~p) ∧ (~q)]
Solution:
[~(p ∨ q) → p] ↔ [(~p) ∧ (~q)]

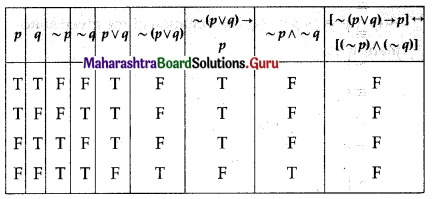
All the entries in the last column of the above truth table are F.
∴ [~(p ∨ q) → p] ↔ [(~p) ∧ (~q)] is a contradiction.

(iv) [~(p ∧ q) → p] ↔ [(~p) ∧ (~q)]
Solution:
[~(p ∧ q) → p] ↔ [(~p) ∧ (~q)]

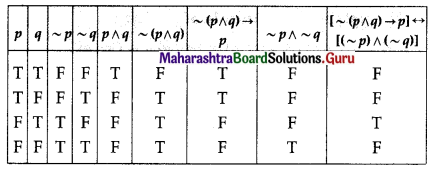
The entries in the last column of the above truth table are neither all T nor all F.
∴ [~(p ∧ q) → p] ↔ [(~p) ∧ (~q)] is a contingency.

(v) [p → (~q ∨ r)] ↔ ~[p → (q → r)]
Solution:
[p → (~q ∨ r)] ↔ ~[p → (q → r)]

All the entries in the last column of the above truth table are F.
∴ [p → (~q ∨ r)] ↔ ~[p → (q → r)] is a contradiction.

Question 14.
Using the truth table, prove the following logical equivalences:
(i) p ∧ (q ∨ r) ≡ (p ∧ q) ∨ (p ∧ r)
Solution:
p ∧ (q ∨ r) ≡ (p ∧ q) ∨ (p ∧ r)

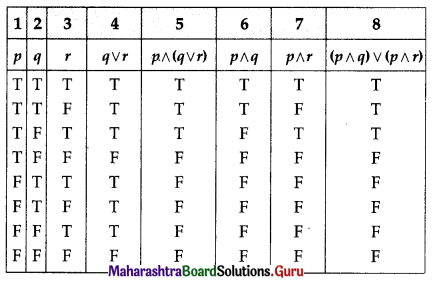
The entries in columns 5 and 8 are identical.
∴ p ∧ (q ∨ r) ≡ (p ∧ q) ∨ (p ∧ r)

(ii) [~(p ∨ q) ∨ (p ∨ q)] ∧ r ≡ r
Solution:
[~(p ∨ q) ∨ (p ∨ q)] ∧ r ≡ r

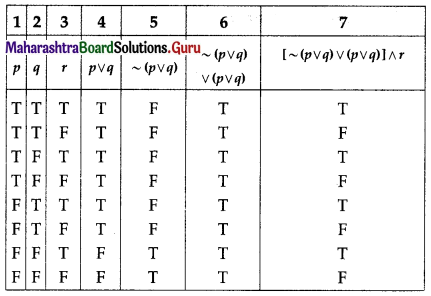
The entries in columns 3 and 7 are identical.
∴ [~(p ∨ q) ∨ (p ∨ q)] ∧ r ≡ r

(iii) p ∧ (~p ∨ q) ≡ p ∧ q
Solution:
p ∧ (~p ∨ q) ≡ p ∧ q

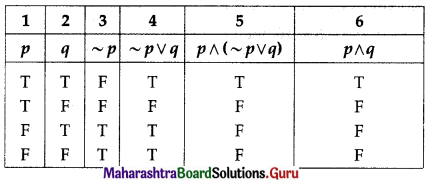
The entries in columns 5 and 6 are identical.
∴ p ∧ (~p ∨ q) ≡ p ∧ q

(iv) p ↔ q ≡ ~(p ∧ ~q) ∧ ~(q ∧ ~p)
Solution:
p ↔ q ≡ ~(p ∧ ~q) ∧ ~(q ∧ ~p)

The entries in columns 3 and 10 are identical.
∴ p ↔ q ≡ ~(p ∧ ~q) ∧ ~(q ∧ ~p)

(v) ~p ∧ q ≡ (p ∨ q) ∧ ~p
Solution:
~p ∧ q ≡ (p ∨ q) ∧ ~p

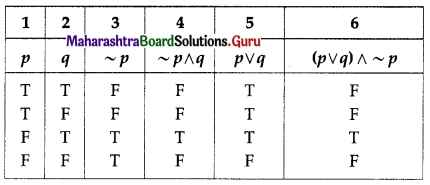
The entries in columns 4 and 6 are identical.
∴ ~p ∧ q ≡ (p ∨ q) ∧ ~p

Question 15.
Write the converse, inverse, contrapositive of the following statements:
(i) If 2 + 5 = 10, then 4 + 10 = 20.
Solution:
Let p : 2 + 5 = 10.
q : 4 + 10 = 20.
Then the symbolic form of the given statement is p → q.
Converse: q → p is the converse of p → q
i.e. If 4 + 10 = 20, then 2 + 5 = 10.
Inverse: ~p → ~q is the inverse of p → q
i.e. If 2 + 5 ≠ 10, then 4 + 10 ≠ 20.
Cotrapositive: ~q → ~p is the contrapositive of p → q,
i.e. If 4 +10 ≠ 20, then 2 + 5 ≠ 10.

(ii) If a man is a bachelor, then he is happy.
Solution:
Let p : A man is a bachelor.
q : He is happy.
Then the symbolic form of the given statement is p → q.
Converse: q → p is the converse of p → q
i.e. If a man is happy, then he is a bachelor.
Inverse: ~p → ~q is the inverse of p → q
i.e. If a man is not a bachelor, then he is not happy.
Contrapositive: ~q → ~p is the contrapositive of p → q
i.e., If a man is not happy, then he is not a bachelor.

(iii) If I do not work hard, then I do not prosper.
Solution:
Let p : I do not work hard.
q : I do not prosper.
Then the symbolic form of the given statement is p → q.
Converse: q → p is the converse of p → q
i.e. If I do not prosper, then I do not work hard.
Inverse: ~p → ~q is the inverse of p → q
i.e. If I work hard, then I prosper.
Contrapositive: ~q → ~p is the contrapositive of p → q
i.e. If I prosper, then I work hard.

Question 16.
State the dual of each of the following statements by applying the principle of duality:
(i) (p ∧ ~q) ∨ (~p ∧ q) ≡ (p ∨ q) ∧ ~(p ∧ q)
(ii) p ∨ (q ∨ r) ≡ ~[(p ∧ q) ∨ (r ∨ s)]
(iii) 2 is an even number or 9 is a perfect square.
Solution:
The duals are given by:
(i) (p ∨ ~q) ∧ (~p ∨ q) ≡ (p ∧ q) ∨ ~(p ∨ q)
(ii) p ∧ (q ∧ r) ≡ ~[(p ∨ q) ∧ (r ∧ s)]
(iii) 2 is an even number and 9 is a perfect square.

Question 17.
Rewrite the following statements without using the connective ‘If … then’:
(i) If a quadrilateral is a rhombus, then it is not a square.
(ii) If 10 – 3 = 7, then 10 × 3 ≠ 30.
(iii) If it rains, then the principal declares a holiday.
Solution:
Since, p → q ≡ ~p ∨ q the given statements can be written as:
(i) A quadrilateral is not a rhombus or it is not a square.
(ii) 10 – 3 ≠ 7 or 10 × 3 ≠ 30.
(iii) It does not rain or the principal declares a holiday.

Question 18.
Write the dual of each of the following:
(i) (~p ∧ q) ∨ (p ∧ ~q) ∨ (~p ∧ ~q)
(ii) (p ∧ q) ∧ r ≡ p ∧ (q ∧ r)
(iii) p ∨ (q ∧ r) ≡ (p ∨ q) ∧ (p ∨ r)
(iv) ~(p ∨ q) ≡ ~p ∧ ~q.
Solution:
The duals are given by:
(i) (~p ∨ q) ∧ (p ∨ ~q) ∧ (~p ∨ ~q)
(ii) (p ∨ q) ∨ r ≡ p ∨ (q ∨ r)
(iii) p ∧ (q ∨ r) ≡ (p ∧ q) ∨ (p ∧ r)
(iv) ~(p ∧ q) ≡ ~p ∧ ~q

Question 19.
Consider the following statements:
(i) If D is a dog, then D is very good.
(ii) If D is very good, then D is a dog.
(iii) If D is not very good, then D is not a dog.
(iv) If D is not a dog, then D is not very good.
Identify the pairs of statements having the same meaning. Justify.
Solution:
Let p : D is a dog. and q : D is very good.
Then the given statements in the symbolic form are:
(i) p → q
(ii) q → p
(iii) ~q → ~p
(iv) ~p → ~q

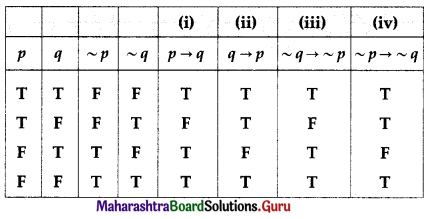
The entries in columns (i) and (iii) are identical. Hence, these statements are equivalent.
∴ the statements (i) and (iii) have the same meaning.
Similarly, the entries in columns (ii) and (iv) are identical. Hence, these statements are equivalent.
∴ the statements (ii) and (iv) have the same meaning.

Question 20.
Express the truth of each of the following statements by Venn diagrams:
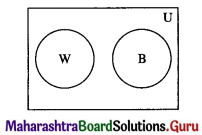
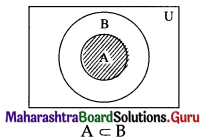
(i) All men are mortal.
Solution:
Let U : a set of all human being
A : set of all men
B : set of all mortals.
Then the Venn diagram represents the truth of the given statement is as below:

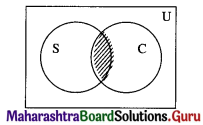
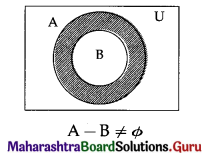
(ii) Some persons are not politicians.
Solution:
Let U : set of all human being
A : set of all persons
B : set of all politicians.
Then the Venn diagram represents the truth of the given statement is as follows:

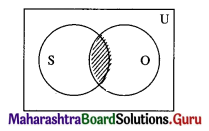
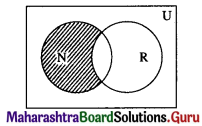
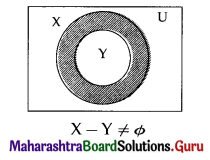
(iii) Some members of the present Indian cricket are not committed.
Solution:
Let U : set of all human being
X : set of all members of present Indian cricket
Y : set of all committed members of the present Indian cricket.
Then the Venn diagram represents the truth of the given statement is as below:

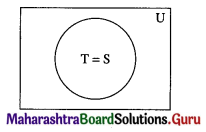
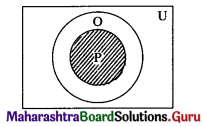
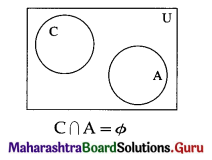
(iv) No child is an adult.
Solution:
Let U : set of all human beings
C : set of all children
A : set of all adults.
Then the Venn diagram represents the truth of the given statement is as below:

Question 21.
If A = {2, 3, 4, 5, 6, 7, 8}, determine the truth value of each of the following statements:
(i) ∃ x ∈ A, such that 3x + 2 > 9.
Solution:
Clearly x = 3, 4, 5, 6, 7, 8 ∈ A satisfy 3x + 2 > 9.
So, the given statement is true, hence its truth value is T.

(ii) ∀x ∈ A, x² < 18.
Solution:
x = 5, 6, 7, 8 ∈ A do not satisfy x² < 18.
So the given statement is false, hence its truth value is F.

(iii) ∃x ∈ A, such that x + 3 < 11.
Solution:
Clearly x = 2, 3, 4, 5, 6, 7 ∈ A which satisfy x + 3 < 11.
So, the given statement is True, hence its truth value is T.

(iv) ∀x ∈ A, x² + 2 ≥ 5.
Solution:
x² + 2 ≥ 5 for all x ∈ A.
So, the given statement is true, hence its truth value is T.

Question 22.
Write the negations of the following statements:
(i) 7 is a prime number and the Taj Mahal is in Agra.
Solution:
Let p : 7 be a prime number.
q : Taj Mahal is in Agra.
Then the symbolic form of the given statement is p ∧ q.
Since, (p ∧ q) ≡ ~p ∨ ~q,
the negation of the given statement is:
‘7 is not a prime number or Taj Mahal is not in Agra.’

(ii) 10 > 5 and 3 < 8.
Solution:
Let p : 10 > 5.
q : 3 < 8.
Then the symbolic form of the given statement is P ∧ q.
Since, ~(p ∧ q) = ~p ∨ ~q, the negation of the given statement is:
’10 ≤ 5 or 3 ≥ 8′
OR
’10 ≯ 5 or 3 ≮ 8′

(iii) I will have tea or coffee.
Solution:
The negation of the given statement is:
‘I will not have tea and coffee.’

(iv) ∀n ∈ N, n + 3 > 9.
Solution:
The negation of the given statement is:
‘∃n ∈ N, such that n + 3 ≯ 9.’
OR
‘∃n ∈ N, such that n + 3 ≤ 9.’

(v) ∃x ∈ A, such that x + 5 < 11.
Solution:
The negation of the given statement is:
‘∀x ∈ A, x + 5 ≮ 1.’
OR
‘∀x ∈ A, x + 5 ≥ 11.’

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 3 Differentiation Ex 3.1 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 3 Differentiation Ex 3.1

1. Find \(\frac{d y}{d x}\) if,

Question 1.
y = \(\sqrt{x+\frac{1}{x}}\)
Solution:

Question 2.
y = \(\sqrt[3]{a^{2}+x^{2}}\)
Solution:

Question 3.
y = (5x³ – 4x² – 8x)⁹
Solution:

2. Find \(\frac{d y}{d x}\) if:

Question 1.
y = log(log x)
Solution:
Given y = log(log x)
Let u = log x
Then y = log u

Question 2.
y = log(10x⁴ + 5x³ – 3x² + 2)
Solution:
Given y = log(10x⁴ + 5x³ – 3x² + 2)
Let u = 10x⁴ + 5x³ – 3x² + 2
Then y = log u

Question 3.
y = log(ax² + bx + c)
Solution:
Given y = log(ax² + bx + c)
Let u = ax² + bx + c
Then y = log u

3. Find \(\frac{d y}{d x}\) if:

Question 1.
y = \(e^{5 x^{2}-2 x+4}\)
Solution:

Question 2.
y = \(a^{(1+\log x)}\)
Solution:

Question 3.
y = \(5^{(x+\log x)}\)
Solution:

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 1 Mathematical Logic Ex 1.10 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 1 Mathematical Logic Ex 1.10

Question 1.
Express the truth of each of the following statements by Venn diagrams:
(i) Some hardworking students are obedient.
Solution:
Let U : set of all students
S : set of all hardworking students
O : set of all obedient students.
Then the Venn diagram represents the truth of the given statement is as below:

S ∩ O ≠ φ

(ii) No circles are polygons.
Solution:
Let U : set of closed geometrical figures in the plane
P : set of all polygons
C : set of all circles.
Then the Venn diagram represents the truth of the given statement is as follows:

P ∩ C = φ

(iii) All teachers are scholars and scholars are teachers.
Solution:
Let U : set of all human beings
T : set of all teachers
S : set of all scholars.
Then the Venn diagram represents the truth of the given statement is as below:

(iv) If a quadrilateral is a rhombus, then it is a parallelogram.
Solution:
Let U : set of all quadrilaterals
R : set of all rhombus
P : set of all parallelograms.
Then the Venn diagram represents the truth of the given statement is as below:

R ⊂ P

Question 2.
Draw the Venn diagrams for the truth of the following statements:
(i) Some share brokers are chartered accountants.
Solution:
Let U : set of all human beings
S : set of all share brokers
C : set of all chartered accountants.
Then the Venn diagram represents the truth of the given statement is as below:

S ∩ C ≠ φ

(ii) No wicket-keeper is a bowler in a cricket team.
Solution:
Let U : set of all human beings
W : set of all wicket keepers
B : set of all bowlers.
Then the Venn diagram represents the truth of the given statement is as follows:

W ∩ B = φ

Question 3.
Represent the following statements by Venn diagrams:
(i) Some non-resident Indians are not rich.
Solution:
Let U : set of all human beings
N : set of all non-resident Indians
R : set of all rich people.
Then the Venn diagram represents the truth of the given statement is as below:

N – R ≠ φ

(ii) No circle is a rectangle.
Solution:
Let U : set of all geometrical figures
C : set of all circles
R : set of all rectangles
Then the Venn diagram represents the truth of the given statement is as below:

C ∩ R = φ

(iii) If n is a prime number and n ≠ 2, then it is odd.
Solution:
Let U : set of all real numbers
P : set of all prime numbers n, where n ≠ 2
O : set of all odd numbers.
Then the Venn diagram represents the truth of the given statement is as below:

P ⊂ O

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 2 Matrices Ex 2.4 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 2 Matrices Ex 2.4

Question 1.
Find A^T, if
(i) A = \(\left[\begin{array}{cc}
1 & 3 \\
-4 & 5
\end{array}\right]\)
(ii) A = \(\left[\begin{array}{ccc}
2 & -6 & 1 \\
-4 & 0 & 5
\end{array}\right]\)
Solution:

Question 2.
If A = [a_ij]_3×3 where a_ij = 2(i – j). Find A and A^T. State whether A and A^T both are symmetric or skew-symmetric matrices.
Solution:


Hence, A and A^T are both skew-symmetric matrices.

Question 3.
If A = \(\left[\begin{array}{cc}
5 & -3 \\
4 & -3 \\
-2 & 1
\end{array}\right]\), prove that (A^T)^T = A.
Solution:

Question 4.
If A = \(\left[\begin{array}{ccc}
1 & 2 & -5 \\
2 & -3 & 4 \\
-5 & 4 & 9
\end{array}\right]\), prove that A^T = A.
Solution:

Question 5.
If A = \(\left[\begin{array}{cc}
2 & -3 \\
5 & -4 \\
-6 & 1
\end{array}\right]\), B = \(\left[\begin{array}{cc}
2 & 1 \\
4 & -1 \\
-3 & 3
\end{array}\right]\), C = \(\left[\begin{array}{cc}
1 & 2 \\
-1 & 4 \\
-2 & 3
\end{array}\right]\), then show that
(i) (A + B)^T = A^T + B^T
(ii) (A – C)^T = A^T – C^T
Solution:

Question 6.
If A = \(\left[\begin{array}{cc}
5 & 4 \\
-2 & 3
\end{array}\right]\) and B = \(\left[\begin{array}{cc}
-1 & 3 \\
4 & -1
\end{array}\right]\), then find C^T, such that 3A – 2B + C = I, where I is the unit matrix of order 2.
Solution:

Question 7.
If A = \(\left[\begin{array}{ccc}
7 & 3 & 0 \\
0 & 4 & -2
\end{array}\right]\), B = \(\left[\begin{array}{ccc}
0 & -2 & 3 \\
2 & 1 & -4
\end{array}\right]\), then find
(i) A^T + 4B^T
(ii) 5A^T – 5B^T
Solution:

Question 8.
If A = \(\left[\begin{array}{lll}
1 & 0 & 1 \\
3 & 1 & 2
\end{array}\right]\), B = \(\left[\begin{array}{lll}
2 & 1 & -4 \\
3 & 5 & -2
\end{array}\right]\) and C = \(\left[\begin{array}{ccc}
0 & 2 & 3 \\
-1 & -1 & 0
\end{array}\right]\), verify that (A + 2B + 3C)^T = A^T + 2B^T + 3C^T
Solution:

Question 9.
If A = \(\left[\begin{array}{ccc}
-1 & 2 & 1 \\
-3 & 2 & -3
\end{array}\right]\) and B = \(\left[\begin{array}{cc}
2 & 1 \\
-3 & 2 \\
-1 & 3
\end{array}\right]\), prove that (A + B^T)^T = A^T + B.
Solution:

From (1) and (2),
(A + B^T)^T = A^T + B.

Question 10.
Prove that A + A^T is symmetric and A – A^T is a skew-symmetric matrix, where
(i) A = \(\left[\begin{array}{ccc}
1 & 2 & 4 \\
3 & 2 & 1 \\
-2 & -3 & 2
\end{array}\right]\)
(ii) A = \(\left[\begin{array}{ccc}
5 & 2 & -4 \\
3 & -7 & 2 \\
4 & -5 & -3
\end{array}\right]\)
Solution:

Question 11.
Express each of the following matrix as the sum of a symmetric and a skew-symmetric matrix:
(i) \(\left[\begin{array}{ll}
4 & -2 \\
3 & -5
\end{array}\right]\)
(ii) \(\left[\begin{array}{ccc}
3 & 3 & -1 \\
-2 & -2 & 1 \\
-4 & -5 & 2
\end{array}\right]\)
Solution:

Question 12.
If A = \(\left[\begin{array}{cc}
2 & -1 \\
3 & -2 \\
4 & 1
\end{array}\right]\) and B = \(\left[\begin{array}{ccc}
0 & 3 & -4 \\
2 & -1 & 1
\end{array}\right]\), verify that
(i) (AB)^T = B^TA^T
(ii) (BA)^T = A^TB^T
Solution:

Balbharti 12th Maharashtra State Board Maths Solutions Book Pdf Chapter 8 Binomial Distribution Miscellaneous Exercise 8 Questions and Answers.

Maharashtra State Board 12th Maths Solutions Chapter 8 Binomial Distribution Miscellaneous Exercise 8

(I) Choose the correct option from the given alternatives:

Question 1.
The mean and the variance of a binomial distribution are 4 and 2 respectively. Then the probability of 2 successes is
(a) √50
(b) 5
(c) 25
(d) 10
Answer:
(b) 5

Question 2.
The mean and the variance of a binomial distribution are 4 and 2 respectively. Then the probablity of 2 successes is
(a) \(\frac{128}{256}\)
(b) \(\frac{219}{256}\)
(c) \(\frac{37}{256}\)
(d) \(\frac{28}{256}\)
Answer:
(d) \(\frac{28}{256}\)

Question 3.
For a binomial distribution, n = 5. If P(X = 4) = P(X = 3) then p = ___________
(a) \(\frac{1}{3}\)
(b) \(\frac{3}{4}\)
(c) 1
(d) \(\frac{2}{3}\)
Answer:
(d) \(\frac{2}{3}\)

Question 4.
In a binomial distribution, n = 4. If 2 P(X = 3) = 3 P(X = 2) then p = ___________
(a) \(\frac{4}{13}\)
(b) \(\frac{5}{13}\)
(c) \(\frac{9}{13}\)
(d) \(\frac{6}{13}\)
Answer:
(c) \(\frac{9}{13}\)

Question 5.
If X ~ B (4, p) and P (X = 0) = \(\frac{16}{81}\), then P (X = 4) = ___________
(a) \(\frac{1}{16}\)
(b) \(\frac{1}{81}\)
(c) \(\frac{1}{27}\)
(d) \(\frac{1}{8}\)
Answer:
(b) \(\frac{1}{81}\)

Question 6.
The probability of a shooter hitting a target is \(\frac{3}{4}\). How many minimum numbers of times must he fie so that the probability of hitting the target at least once is more than 0·99?
(a) 2
(b) 3
(c) 4
(d) 5
Answer:
(c) 4

Question 7.
If the mean and variance of a binomial distribution are 18 and 12 respectively, then n = ___________
(a) 36
(b) 54
(c) 18
(d) 27
Answer:
(b) 54

(II) Solve the following:

Question 1.
Let X ~ B(10, 0.2). Find
(i) P(X = 1)
(ii) P(X ≥ 1)
(iii) P(X ≤ 8).
Solution:
X ~ B(10, 0.2)
∴ n = 10, p = 0.2
∴ q = 1 – p = 1 – 0.2 = 0.8
The p,m.f. of X is given by

Question 2.
Let X ~ B(n, p).
(i) If n = 10, E(X) = 5, find p and Var(X).
(ii) If E(X) = 5 and Var(X) = 2.5, find n and p.
Solution:
X ~ B(n, p)
(i) Given: n = 10 and E(X) = 5
But E(X) = np
∴ np = 5.
∴ 10p = 5
∴ p = \(\frac{1}{2}\)
∴ q = 1 – p = 1 – \(\frac{1}{2}\) = \(\frac{1}{2}\)
Var(X) = npq = 10(\(\frac{1}{2}\))(\(\frac{1}{2}\)) = 2.5.
Hence, p = \(\frac{1}{2}\) and Var(X) = 2.5

(ii) Given: E(X) = 5 and Var(X) = 2.5
∴ np = 5 and npq = 2.5
∴ \(\frac{n p q}{n p}=\frac{2.5}{5}\)
∴ q = 0.5 = \(\frac{5}{10}=\frac{1}{2}\)
∴ p = 1 – q = 1 – \(\frac{1}{2}\) = \(\frac{1}{2}\)
Substituting p = \(\frac{1}{2}\) in np = 5, we get
n(\(\frac{1}{2}\)) = 5
∴ n = 10
Hence, n = 10 and p = \(\frac{1}{2}\)

Question 3.
If a fair coin is tossed 10 times and the probability that it shows heads (i) 5 times (ii) in the first four tosses and tail in the last six tosses.
Solution:
Let X = number of heads.
p = probability that coin tossed shows a head
∴ p = \(\frac{1}{2}\)
q = 1 – p = 1 – \(\frac{1}{2}\) = \(\frac{1}{2}\)
Given: n = 10
∴ X ~ B(10, \(\frac{1}{2}\))
The p.m.f. of X is given by
P(X = x) = \({ }^{n} C_{x} P^{x} q^{n-x}\)

(i) P(coin shows heads 5 times) = P[X = 5]

Hence, the probability that can shows heads exactly 5 times = \(\frac{63}{256}\)

(ii) P(getting heads in first four tosses and tails in last six tosses) = P(X = 4)

Hence, the probability that getting heads in first four tosses and tails in last six tosses = \(\frac{105}{512}\).

Question 4.
The probability that a bomb will hit a target is 0.8. Find the probability that out of 10 bombs dropped, exactly 2 will miss the target.
Solution:
Let X = the number of bombs hitting the target.
p = probability that bomb will hit the target
∴ p = 0.8 = \(\frac{8}{10}=\frac{4}{5}\)
∴ q = 1 – p = 1 – \(\frac{4}{5}\) = \(\frac{1}{5}\)
Given: n = 10
∴ X ~ B(10, \(\frac{4}{5}\))
The p.m.f. of X is given as:
P[X = x] = \({ }^{n} \mathrm{C}_{x} p^{x} q^{n-x}\)
i.e.p(x) = \({ }^{10} \mathrm{C}_{x}\left(\frac{4}{5}\right)^{x}\left(\frac{1}{5}\right)^{10-x}\)
P(exactly 2 bombs will miss the target) = P(exactly 8 bombs will hit the target)
= P[X = 8]
= p(8)

Hence, the probability that exactly 2 bombs will miss the target = 45\(\left(\frac{2^{16}}{5^{10}}\right)\)

Question 5.
The probability that a mountain bike travelling along a certain track will have a tire burst is 0.05. Find the probability that among 17 riders:
(i) exactly one has a burst tyre
(ii) at most three have a burst tyre
(iii) two or more have burst tyres.
Solution:
Let X = number of burst tyres.
p = probability that a mountain bike travelling along a certain track will have a tyre burst.
∴ p = 0.05
∴ q = 1 – p = 1 – 0.05 = 0.95
Given: n = 17
∴ X ~ B(17, 0.05)
The p.m.f. of X is given by
P(X = x) = \({ }^{n} \mathrm{C}_{x} P^{x} q^{n-x}\)
i.e.(x) = \({ }^{17} \mathrm{C}_{x}(0.05)^{x}(0.95)^{17-x}\), x = 0, 1, 2, ……, 17
(i) P(exactly one has a burst tyre)
P(X = 1) = p(1) = \({ }^{17} \mathrm{C}_{1}\) (0.05)¹ (0.95)^17-1
= 17(0.05) (0.95)¹⁶
= 0.85(0.95)¹⁶
Hence, the probability that riders has exactly one burst tyre = (0.85)(0.95)¹⁶

(ii) P(at most three have a burst tyre) = P(X ≤ 3)
= P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3)
= p(0) + p(1) + p(2) + p(3)


Hence, the probability that at most three riders have burst tyre = (2.0325)(0.95)¹⁴.

(iii) P(two or more have tyre burst) = P(X ≥ 2)
= 1 – P(X < 2)
= 1 – [P(X = 0) + P(X = 1)]
= 1 – [p(0) + p(1)]
= 1 – [\({ }^{17} \mathrm{C}_{0}\) (0.05)⁰ (0.95)¹⁷ + \({ }^{17} \mathrm{C}_{1}\) (0.05)(0.95)¹⁶]
= 1 – [1(1)(0.95)¹⁷ + 17(0.05)(0.95)¹⁶]
= 1 – (0.95)¹⁶[0.95 + 0.85]
= 1 – (1.80)(0.95)¹⁶
= 1 – (1.8)(0.95)¹⁶
Hence, the probability that two or more riders have tyre burst = 1 – (1.8)(0.95)¹⁶.

Question 6.
The probability that a lamp in a classroom will be burnt out is 0.3. Six such lamps are fitted in the classroom. If it is known that the classroom is unusable if the number of lamps burning in it is less than four, find the probability that the classroom cannot be used on a random occasion.
Solution:
Let X = number of lamps burnt out in the classroom.
p = probability of a lamp in a classroom will be burnt
∴ p = 0.3 = \(\frac{3}{10}\)
∴ q = 1 – p = 1 – \(\frac{3}{10}\) = \(\frac{7}{10}\)
Given: n = 6
∴ X ~ B(6, \(\frac{3}{10}\))
The p.m.f. of X is given as:
P[X = x] = \({ }^{n} \mathrm{C}_{x} p^{x} q^{n-x}\)
i.e., p(x) = \({ }^{6} \mathrm{C}_{x}\left(\frac{3}{10}\right)^{x}\left(\frac{7}{10}\right)^{6-x}\)
Since the classroom is unusable if the number of lamps burning in it is less than four, therefore
P(classroom cannot be used) = P[X < 4]
= P[X = 0] + P[X = 1] + P[X = 2] + P[X = 3]
= p(0) + p(1) + p(2) + p(3)

Hence, the probability that the classroom cannot be used on a random occasion is 0.92953.

Question 7.
A lot of 100 items contain 10 defective items. Five items are selected at random from the lot and sent to the retail store. What is the probability that the store will receive at most one defective item?
Solution:
Let X = number of defective items.
p = probability that item is defective
∴ p = \(\frac{10}{100}=\frac{1}{10}\)
∴ q = 1 – p = 1 – \(\frac{1}{10}\) = \(\frac{9}{10}\)
Given: n = 5
∴ X ~ B(5, \(\frac{1}{10}\))
The p.m.f. of X is given as:
P[X = x] = \({ }^{n} \mathrm{C}_{x} p^{x} q^{n-x}\)
i.e., p(x) = \({ }^{5} C_{x}\left(\frac{1}{10}\right)^{x}\left(\frac{9}{10}\right)^{5-x}\)
P (store will receive at most one defective item) = P[X ≤ 1]
=P[X = 0] + P[X = 1]
= p(0) + p(1)

Hence, the probability that the store will receive at most one defective item is (1.4)(0.9)⁴.

Question 8.
A large chain retailer purchases a certain kind of electronic device from a manufacturer. The manufacturer indicates that the defective rate of the device is 3%. The inspector of the retailer picks 20 items from a shipment. What is the probability that the store will receive at most one defective item?
Solution:
Let X = number of defective electronic devices.
p = probability that device is defective
∴ p = 3% = \(\frac{3}{100}\)
∴ q = 1 – p = 1 – \(\frac{3}{100}\) = \(\frac{97}{100}\)
Given: n = 20
∴ X ~ B(20, \(\frac{3}{100}\))
The p.m.f. of X is given as:

Hence, the probability that the store will receive at most one defective item = (1.57)(0.97)¹⁹.

Question 9.
The probability that a certain kind of component will survive a check test is 0.6. Find the probability that exactly 2 of the next 4 tested components tested survive.
Solution:
Let X = number of tested components survive.
p = probability that the component survives the check test

Hence, the probability that exactly 2 of the 4 tested components survive is 0.3456.

Question 10.
An examination consists of 10 multiple choice questions, in each of which a candidate has to deduce which one of five suggested answers is correct. A completely unprepared student guesses each answer completely randomly. What is the probability that this student gets 8 or more questions correct? Draw the appropriate moral.
Solution:
Let X = number of correct answers.
p = probability that student gets correct answer
∴ p = \(\frac{1}{5}\)
∴ q = 1 – p = 1 – \(\frac{1}{5}\) = \(\frac{4}{5}\)
Given: n = 10 (number of total questions)
∴ X ~ B(10, \(\frac{1}{5}\))
The p.m.f. of X is given by

Hence, the probability that student gets 8 or more questions correct = \(\frac{30.44}{5^{8}}\)

Question 11.
The probability that a machine will produce all bolts in a production run within specification is 0.998. A sample of 8 machines is taken at random. Calculate the probability that (i) all 8 machines (ii) 7 or 8 machines (iii) at most 6 machines will produce all bolts within specification.
Solution:
Let X = number of machines which produce the bolts within specification.
p = probability that a machine produce bolts within specification
p = 0.998 and q = 1 – p = 1 – 0.998 = 0.002
Given: n = 8
∴ X ~ B(8, 0.998)
The p.m.f. of X is given by
P(X = x) = \({ }^{n} \mathrm{C}_{x} p^{x} q^{n-x}\)
i.e. p(x) = \({ }^{8} \mathrm{C}_{x}(0.998)^{x}(0.002)^{8-x}\), x = 0, 1, 2, …, 8
(i) P(all 8 machines will produce all bolts within specification) = P[X = 8]
= p(8)
= \({ }^{8} \mathrm{C}_{8}\) (0.998)⁸ (0.002)^8-8
= 1(0.998)⁸ . (1)
= (0.998)⁸
Hence, the probability that all 8 machines produce all bolts with specification = (0.998)⁸.

(ii) P(7 or 8 machines will produce all bolts within i specification) = P (X = 7) + P (X = 8)

Hence, the probability that 7 or 8 machines produce all bolts within specification = (1.014)(0.998)⁷.

(iii) P(at most 6 machines will produce all bolts with specification) = P[X ≤ 6]
= 1 – P[x > 6]
= 1 – [P(X = 7) + P(X = 8)]
= 1 – [P(7) + P(8)]
= 1 – (1.014)(0.998)⁷
Hence, the probability that at most 6 machines will produce all bolts with specification = 1 – (1.014)(0.998)⁷.

Question 12.
The probability that a machine develops a fault within the first 3 years of use is 0.003. If 40 machines are selected at random, calculate the probability that 38 or more will develop any faults within the first 3 years of use.
Solution:
Let X = the number of machines who develop a fault.
p = probability that a machine develops a fait within the first 3 years of use
∴ p = 0.003 and q = 1 – p = 1 – 0.003 = 0.997
Given: n = 40
∴ X ~ B(40, 0.003)
The p.m.f. of X is given by

Hence, the probability that 38 or more machines will develop the fault within 3 years of use = (775.44)(0.003)³⁸.

Question 13.
A computer installation has 10 terminals. Independently, the probability that anyone terminal will require attention during a week is 0.1. Find the probabilities that (i) 0 (ii) 1 (iii) 2 (iv) 3 or more, terminals will require attention during the next week.
Solution:
Let X = number of terminals which required attention during a week.
p = probability that any terminal will require attention during a week
∴ p = 0.1 and q = 1 – p = 1 – 0.1 = 0.9
Given: n = 10
∴ X ~ B(10, 0.1)
The p.m.f. of X is given by
P(X = x) = \({ }^{n} \mathrm{C}_{x} p^{x} q^{n-x}\)
i.e. p(x) = \({ }^{10} C_{x}(0.1)^{x}(0.9)^{10-x}\), x = 0, 1, 2, …, 10
(i) P(no terminal will require attention) = P(X = 0)

Hence, the probability that no terminal requires attention = (0.9)¹⁰

(ii) P(1 terminal will require attention)

Hence, the probability that 1 terminal requires attention = (0.9)⁹.

(iii) P(2 terminals will require attention)

Hence, the probability that 2 terminals require attention = (0.45)(0.9)⁸.

(iv) P(3 or more terminals will require attention)

Hence, the probability that 3 or more terminals require attention = 1 – (2.16) × (0.9)⁸.

Question 14.
In a large school, 80% of the pupil like Mathematics. A visitor to the school asks each of 4 pupils, chosen at random, whether they like Mathematics.
(i) Calculate the probabilities of obtaining an answer yes from 0, 1, 2, 3, 4 of the pupils.
(ii) Find the probability that the visitor obtains answer yes from at least 2 pupils:
(a) when the number of pupils questioned remains at 4.
(b) when the number of pupils questioned is increased to 8.
Solution:
Let X = number of pupils like Mathematics.
p = probability that pupils like Mathematics

(i) The probabilities of obtaining an answer yes from 0, 1, 2, 3, 4 of pupils are P(X = 0), P(X = 1), P(X = 2), P(X = 3) and P(X = 4) respectively

(ii) (a) P(visitor obtains the answer yes from at least 2 pupils when the number of pupils questioned remains at 4) = P(X ≥ 2)
= P(X = 2) + P(X = 3) + P(X = 4)

(b) P(the visitor obtains the answer yes from at least 2 pupils when number of pupils questioned is increased to 8)

Question 15.
It is observed that it rains 12 days out of 30 days. Find the probability that
(i) it rains exactly 3 days of the week.
(ii) it will rain at least 2 days of a given week.
Solution:
Let X = the number of days it rains in a week.
p = probability that it rains

(i) P(it rains exactly 3 days of week) = P(X = 3)

Hence, the probability that it rains exactly 3 days of week = 0.2903.

(ii) P(it will rain at least 2 days of the given week)

Hence, the probability that it rains at least 2 days of a given week = 0.8414

Question 16.
If the probability of success in a single trial is 0.01. How many trials are required in order to have a probability greater than 0.5 of getting at least one success?
Solution:
Let X = number of successes.
p = probability of success in a single trial
∴ p = 0.01
and q = 1 – p = 1 – 0.01 = 0.99
∴ X ~ B(n, 0.01)
The p.m.f. of X is given by
P(X = x) = \({ }^{n} \mathrm{C}_{x} p^{x} q^{n-x}\)

Hence, the number of trials required in order to have a probability greater than 0.5 of getting at least one success is \(\frac{\log 0.5}{\log 0.99}\) or 68.

Question 17.
In binomial distribution with five Bernoulli’s trials, the probability of one and two success are 0.4096 and 0.2048 respectively. Find the probability of success.
Solution:
Given: X ~ B(n = 5, p)
The probability of X success is


Hence, the probability of success is \(\frac{1}{5}\).