Prasanna

Balbharti Maharashtra State Board Organisation of Commerce and Management 12th Textbook Solutions Chapter 3 Entrepreneurship Development Textbook Exercise Questions and Answers.

Maharashtra State Board 12th Organisation of Commerce and Management Solutions Chapter 3 Entrepreneurship Development

1. (A) Select the correct options and rewrite the sentences

Question 1.
The word ‘entrepreneur’ is derived from the ……………… word ‘entreprende’.
(a) Japanese
(b) English
(c) French
Answer:
(c) French

Question 2.
‘Entreprende’ means to ………………..
(a) Undertake
(b) Enterprise
(c) Businessman
Answer:
(a) Undertake

Question 3.
Start Up India is an initiative of the ………………
(a) RBI
(b) Government of India
(c) World Bank
Answer:
(b) Government of India

Question 4.
………………. is the idea of bringing urban residents to rural areas, by empowering to local communities, both socially and economically.
(a) Agro tourism
(b) Medical tourism
(c) Entertainment
Answer:
(a) Agro tourism

1. (B) Match the pairs

Answer:

1. (C) Give one word/phrase/term which can substitute each one of the following

Question 1.
An undertaking or adventure involving uncertainty and risk and requiring innovation.
Answer:
Enterprise

Question 2.
A function of creating something new for an economic activity.
Answer:
Innovation

Question 3.
A person who is an innovator who introduces new combinations of means of production.
Answer:
Entrepreneur

Question 4.
The process of enhancing entrepreneurial skills and knowledge through structured training and institution building programmes.
Answer:
Entrepreneur development

Question 5.
The process that motivates a person into action and induces him to continue the course of action for the achievement of goals.
Answer:
Motivation

Question 6.
An employee who has an authority and support of the organisation to implement his creative ideas.
Answer:
Intrapreneur

1. (D) State true or false

Question 1.
An entrepreneur should not be ready to work hard.
Answer:
False

Question 2.
Agritourism can support agricultural economy.
Answer:
True

Question 3.
Successful businessman takes calculated risk.
Answer:
True

Question 4.
Entrepreneurship is a full time job which requires dedication and hard work.
Answer:
True

Question 5.
Startup India is an initiative of the Government of France.
Answer:
False.

1. (E) Find the odd one

Question 1.
Communicator, Innovator, Self-starter, Inactive.
Answer:
Inactive

Question 2.
Trekking, Wildlife study, Horseback riding, Indoor games.
Answer:
Indoor games

Question 3.
Innovation, Lack of communication, Development of market, Determining the objectives.
Answer:
Lack of communication.

1. (F) Complete the sentences

Question 1.
An entrepreneur is a person who starts a …………………
Answer:
Business / Enterprise

Question 2.
‘Start-up’ India initiative was launched in ……………….
Answer:
2016.

1. (G) Select the correct option

(Innovation, Niche tourism, Agro tourism, Entrepreneurship)

Answer:

1. (H) Answer in one sentence

Question 1.
What is Agro tourism?
Answer:
Agro tourism is the idea to bring urban residents to rural areas for leisure travel and spending.

Question 2.
Who is ‘Entrepreneur’?
Answer:
Entrepreneur is a person who organises and operates a business or businesses, taking on greater than normal financial risks in order to do so.

Question 3.
Who described ‘Entrepreneurship’ as the founding of a private enterprise?
Answer:
John Sturt Mill, a famous economist, described ‘Entrepreneurship’ as founding of a private enterprise in 1948.

1. (I) Correct the underlined word and rewrite the sentences

Question 1.
The word ‘Entrepreneur’ comes from the German verb entreprendre, it means ‘to undertake’.
Answer:
French

Question 2.
Entrepreneurs try to make home a better place where the needs of consumers can be satisfied.
Answer:
market

Question 3.
The loan taken under stand-up India scheme is repayable in ten years.
Answer:
seven.

2. Explain the following term/concept

Question 1.
Entrepreneur.
Answer:
An entrepreneur is a person who starts a business and is willing to risk loss in order to make money. The entrepreneurs are passionate to invent, innovate, lead or pioneers with a disruptive product or technology. Entrepreneurs try to make market a better place where the needs of consumers can be satisfied. They have the courage to offer and share an idea or a product or a service with the world. A small businessman, founder of multi-billion company, freelancing carpenter are examples of entrepreneurs.

Question 2.
Agro tourism.
Answer:
Agro tourism is the idea of bringing urban residents to rural areas for leisure travel and spending. Agro tourism is a commercial enterprise at a working farm, ranch or agricultural plant conducted for the enjoyment of visitors that generates supplement income for the owner. Agrotourism activities include picking fruits, tending bees, milking cows and other educational pursuits. In short, Agro tourism provides the tourists a chance to reconnect with the land and provides a ‘hand on experience’ with local foods. Agro tourism can support agricultural economy when local producers can no longer complete economically.

Question 3.
Start-ups.
Answer:
A start-up is defined as an entity having its headquarter in India, which was opened less than 10 years ago and has an annual turnover of less than Rs 100 crores. It is an initiative of the Government of India, launched in 2016. It aims at building an ecosystem which will nurture start¬ups in the country. So that, sustainable economic growth and large scale employment opportunities can be generated. One of the objectives of the Indian Government is to make India a nation of job creator instead of job seekers.

Question 4.
Stand-ups.
Answer:
Stand-up India scheme is for financing SC/ST and/or women entrepreneurs. The objective of the stand-up India scheme is to facilitate bank loans between Rs 10 lakh and Rs 1 crore to at least one SC or ST borrower and at least one woman borrower per branch for setting up a greenfield enterprise. This enterprise maybe in manufacturing, services or the trading sector. In case of non-individual enterprise at least 51% of the share-holding and controlling stake should be held by either an SC/ST or woman, above 18 years of age.

Question 5.
Intrapreneur.
Answer:
Intrapreneurship is the entrepreneurship within an existing organisation. An intrapreneur is an employee who has the authority and support of his company/employer to implement his own innovative and creative ideas. His idea or products may or may not earn immediate revenue for the company. But the employee keeps receiving his salary. The company provides the infrastructure. Many large organisations have dedicated Research and Development Departments where employees are encouraged to use their creative abilities. These ideas or innovations may earn handsome profit to an organisation. So Intrapreneur is the entrepreneurship within an organisation.

3. Study the following case /situation and express your opinion

Mr. Soham is a young MBA degree holder, Mr. Navin is B.Com graduate. Mr. Soham is willing to start dairy farm at his village, Mr. Navin is willing to work as cashier in Private Company.

(i) Find out dream of Soham and Navin.
(ii) State anyone feature of Entrepreneur.
(iii) To become successful entrepreneur, which qualities Mr. Soham should have?
Answer:
(i) Dream of Soham is to become ; entrepreneur and dream of Navin is to take up job in a private company and get a fixed income as salary.
(ii) Entrepreneur is a person who is willing to take risk in order to earn money and start a business.
(iii) To become a successful entrepreneur Mr. Soham should have qualities like innovator, creator, reactive and risk bearer.

4. Answer in brief

Question 1.
Define Entrepreneur. Explain functions of entrepreneur.
Answer:
[A] Definition : According to Webster dictionary, “An entrepreneur is a person who starts a business and is willing to risk loss in order to make money.” The entrepreneurs are passionate to innovate, lend, invent or pioneer with a disruptive product or technology. A small businessman, a plumber or a founder of huge company are entrepreneurs.

[B] Functions : The functions of an entrepreneur are:
(1) Innovation : Usually, an entrepreneur has an innovative mind. He introduces new combination of means of production. He introduces something new or something different that would give his business a competitive advantage. Innovation sometimes involves problem solving and entrepreneur gets pleasure by using his talents to solve those problems.

(2) Determination of objectives : An entrepreneur is required to decide the aims and objectives of the business enterprise he intends to establish. He has to change those aims and ; objectives as per changing conditions or accept those which are beneficial to the enterprise as per the market situation.

(3) Development of market: An entrepreneur has to find new, different and innovative ways to market his products and services. As the markets are developing constantly, he has to conduct surveys, research to understand customer’s demand.

(4) New technology : Entrepreneur has to install new, advanced and efficient technology, new machinery, new and scientific methods of production to save overall cost and to improve the methods of production.

(5) Good relations : Prosperity, growth and development of enterprise mostly depend on the cordial and efficient relations of the superiors, subordinates and all employees. In this respect, co-ordination among the employees plays a significant role to make business enterprise successful.

(6) Organising funds : Finance is required to meet working capital and fixed capital needs of business. The entrepreneur has to raise adequate financial resources to keep enterprise in living condition. For this purpose, he has to keep good relation with the existing as well as potential investors.

(7) Taking decisions : Timely, correct and wise decisions are most important to run a successful business. An entrepreneur has to evaluate pros and cons of every business decision.

Question 2.
Define entrepreneur. Explain the qualities of successful entrepreneur.
Answer:
[A] Definition : According to Webster dictionary, “An entrepreneur is a person who starts a business and is willing to risk loss in order to make money.” The entrepreneurs are passionate to innovate, lend, invent or pioneer with a disruptive product or technology. A small businessman, a plumber or a founder of huge company are entrepreneurs.

[B] The qualities of a successful entrepreneur:
(1) Discipline : An entrepreneur has comprehensive strategies and tactics to accomplish the organisational goal. Successful entrepreneur is disciplined enough to take steps every day towards the attainment of his objectives. They eliminate any hindrance or distractions.

(2) Confidence : An entrepreneur is confident with the knowledge that he will make his businesses succeed. He shows the confidence in everything he does.

(3) Open-minded : An entrepreneur has the ability to look at everything around him and realises that every event and situation is a business opportunity. New ideas are constantly being generated about potential new business.

(4) Self starter : An entrepreneur is proactive, not waiting for someone to give him permission. Everything which needs to be done, he should start in himself. So, he sets parameters for the project.

(5) Competitive : An entrepreneur knows that he can do a job better than others. He needs to be competitive to win every game of the business.

(6) Creativity : An entrepreneur often comes up with solutions which are the synthesis of other item. He makes connections between two unrelated events or situations.

(7) Determination : An entrepreneur is determined to make all of their endeavours succeed, so will try again until it does. He sees opportunity for success in defeat.

(8) Strong communication skills : The entrepreneur has strong communication skill to sell the product and to motivate employees. He has to highlight benefits of situation and coach others to be successful.

(9) Strong work ethics : An entrepreneur mind is constantly on his work place to ensure that an outcome meets his expectations.

(10) Passion : Passion is the most important that of a successful entrepreneur. He genuinely loves his work because there is a joy that his business gives which goes beyond the money. He should always research and read to make his business grow and be better.

5. Justify the following statements

Question 1.
Entrepreneurship is the best source for self-employment.
Answer:
(1) Innovation : Entrepreneur need to be innovative. The essence of entrepreneurship is innovation. Innovation may take place in the following forms viz., the introduction of a new product in the market, the installation of new production technology, entry of specific product, the discovery of a new source of raw material, etc. In view of changing taste, preferences, etc., of the consumers, from time to time, entrepreneur undertakes research and development to manufacture products to satisfy the consumers’ needs.

(2) Economic activity: In order to satisfy human wants and as well as in exchange earn a better livelihood, an entrepreneur manufactures new products or modify the existing products as per the needs, preferences and demands of the consumers. For this purpose, he undertakes a systematic plan activity by using his skills, knowledge and experience. For this reason, entrepreneurship is considered as an economic activity.

(3) Creative activity : Innovation is a process of creating something new and creativity is most important for innovation. Therefore, innovation should be strongly supported by creativity, Innovation and creativity are supplement to each other. Introducing creativity in the production process is a challenging task before the entrepreneur. Hence, creativity is an essential element of entrepreneurship.

(4) Risk-bearing : An entrepreneur has to undertake many risks including fall in prices, changes in fashions, earthquake, etc. All these risks cannot be insured with insurance companies. A risk which cannot be insured against and measured is called uncertainty. Entrepreneur buys factors of production at certain prices to combine their contributions into the products and then sells those products at uncertain prices in future. Thus, entrepreneur is a risk-bearing agent of production.

Question 2.
Successful businessman takes calculated risk.
Answer:
[A] Definition : According to Webster dictionary, “An entrepreneur is a person who starts a business and is willing to risk loss in order to make money.” The entrepreneurs are passionate to innovate, lend, invent or pioneer with a disruptive product or technology. A small businessman, a plumber or a founder of huge company are entrepreneurs.

[B] Functions : The functions of an entrepreneur are:
(1) Innovation : Usually, an entrepreneur has an innovative mind. He introduces new combination of means of production. He introduces something new or something different that would give his business a competitive advantage. Innovation sometimes involves problem solving and entrepreneur gets pleasure by using his talents to solve those problems.

(2) Determination of objectives : An entrepreneur is required to decide the aims and objectives of the business enterprise he intends to establish. He has to change those aims and ; objectives as per changing conditions or accept those which are beneficial to the enterprise as per the market situation.

(3) Development of market: An entrepreneur has to find new, different and innovative ways to market his products and services. As the markets are developing constantly, he has to conduct surveys, research to understand customer’s demand.

(4) New technology : Entrepreneur has to install new, advanced and efficient technology, new machinery, new and scientific methods of production to save overall cost and to improve the methods of production.

(5) Good relations : Prosperity, growth and development of enterprise mostly depend on the cordial and efficient relations of the superiors, subordinates and all employees. In this respect, co-ordination among the employees plays a significant role to make business enterprise successful.

(6) Organising funds : Finance is required to meet working capital and fixed capital needs of business. The entrepreneur has to raise adequate financial resources to keep enterprise in living condition. For this purpose, he has to keep good relation with the existing as well as potential investors.

(7) Taking decisions : Timely, correct and wise decisions are most important to run a successful business. An entrepreneur has to evaluate pros and cons of every business decision.

Question 3.
Entrepreneur must be a good communicator.
Answer:
The following are the characteristics of entrepreneurship development:
(1) Innovation : Entrepreneur need to be innovative. The essence of entrepreneurship is innovation. Innovation may take place in the following forms viz., the introduction of a new product in the market, the installation of new production technology, entry of specific product, the discovery of a new source of raw material, etc. In view of changing taste, preferences, etc., of the consumers, from time to time, entrepreneur undertakes research and development to manufacture products to satisfy the consumers’ needs.

(2) Economic activity: In order to satisfy human wants and as well as in exchange earn a better livelihood, an entrepreneur manufactures new products or modify the existing products as per the needs, preferences and demands of the consumers. For this purpose, he undertakes a systematic plan activity by using his skills, knowledge and experience. For this reason, entrepreneurship is considered as an economic activity.

(3) Organisation building : Entrepreneurship is an activity of organising various factors of production and various resources such as financial, physical and human resources. By considering place utility, time utility, form utility, etc., entrepreneur has to assemble different factors j of production and resources under one roof for producing new products.

(4) Creative activity : Innovation is a process of creating something new and creativity is most important for innovation. Therefore, innovation should be strongly supported by creativity, Innovation and creativity are supplement to each other. Introducing creativity in the production process is a challenging task before the entrepreneur. Hence, creativity is an essential element of entrepreneurship.

(5) Managerial skill and leadership : The entrepreneur who has strong passion of doing or creating something new rather than just to earn profit will become a successful entrepreneur. Managerial skills and leadership are the most important features of successful entrepreneur. Other skills are not considered so important. Entrepreneur must be a good leader and manager of the groups working under him.

(6) Skilful management : The efficient and skilful management of the organisation is an important quality of entrepreneurship. With the help of professional management and skilled managers, entrepreneurship becomes easy and successful activity. The success of any entrepreneurship depends on its skilful management.

(7) Risk-bearing : An entrepreneur has to undertake many risks including fall in prices, changes in fashions, earthquake, etc. All these risks cannot be insured with insurance companies. A risk which cannot be insured against and measured is called uncertainty. Entrepreneur buys factors of production at certain prices to combine their contributions into the products and then sells those products at uncertain prices in future. Thus, entrepreneur is a risk-bearing agent of production.

(8) Gap filling function : Gap filling is considered as the most important feature of entrepreneur. It is the job of entrepreneur to find the gap and fill it or make up the deficiencies which always exist in the knowledge about the production function. Entrepreneur must have all the solutions of the problems.

Question 4.
An entrepreneur must be an innovator.
Answer:
Innovation is a dynamic change brought by entrepreneur by bringing new combinations of factors of production. Innovation by entrepreneur is must for development of an organisation. Entrepreneur can be an innovator in many ways. They are:
(1) Introduction of a new product: Entrepreneur through his dynamic skill and intelligence create new products by fulfilling innovation to commercialisation by embedding it in an environment where it did not exist previously.

(2) Introduction of a new method of production : By introducing new and latest technology an entrepreneur brings new life and energy in methods of production. Introduction of new technology, new machinery, scientific methods of production will save money and time of the organisation.

(3) Opening of a new market : An innovative idea with new products. It opens a new market which are not existing previously.

(4) Carrying out new forms of organisation for industry : An innovative entrepreneur is the one who discovers new methods and new materials. He utilises invention and discoveries in order to make new combinations. Thus, entrepreneur must be an innovator.

Question 5.
With creativity, farmers can expand their Agro tourism Business.
Answer:
With creativity, farmers can expand their Agro tourism business through recreation, fun, entertaining activities. The valuable activities which farmer can do creatively are:
(1) Outdoor recreation : Farmer can add value and can expand their agro tourism business by outdoor recreation like trekking, fishing, hunting, wild life study, horse back riding, etc. All such activities are the point of attraction for a tourist and this can be enjoyed with family and friends too.

(2) Educational experiences : Farmers can also be more creative in farming tours, rice plantation, cooking classes on chulha. All such activities help customer to get hands on experience which they enjoy with adding educational values.

(3) Entertainment : Entertainment through harvest festivals like Hurda Party’ in Maharashtra, local dances, folk songs are recreation also main attraction for a customer for agro tourism. Entertaining activities such as contest, adult and children classes, games, etc. can be arranged. This innovative touch helps farmer to expand his business.

(4) Hospitality services : Hospitality services like farm stays, guided forms makes customer more happy. Farmer can add value to guest experience by offering them refreshment, fresh fruits, juice, fresh food, etc.

Happy customer will definitely returns and also spread good word of mouth to their family and friends. Thus, with creativity, farmers can expand their agro tourism business.

6. Attempt the following

Question 1.
Explain the characteristics Entrepreneurship Development.
Answer:
The following are the characteristics of entrepreneurship development:
(1) Innovation : Entrepreneur need to be innovative. The essence of entrepreneurship is innovation. Innovation may take place in the following forms viz., the introduction of a new product in the market, the installation of new production technology, entry of specific product, the discovery of a new source of raw material, etc. In view of changing taste, preferences, etc., of the consumers, from time to time, entrepreneur undertakes research and development to manufacture products to satisfy the consumers’ needs.

(2) Economic activity: In order to satisfy human wants and as well as in exchange earn a better livelihood, an entrepreneur manufactures new products or modify the existing products as per the needs, preferences and demands of the consumers. For this purpose, he undertakes a systematic plan activity by using his skills, knowledge and experience. For this reason, entrepreneurship is considered as an economic activity.

(3) Organisation building : Entrepreneurship is an activity of organising various factors of production and various resources such as financial, physical and human resources. By considering place utility, time utility, form utility,etc., entrepreneur has to assemble different factors j of production and resources under one roof for producing new products.

(4) Creative activity : Innovation is a process of creating something new and creativity is most important for innovation. Therefore, innovation should be strongly supported by creativity, Innovation and creativity are supplement to each other. Introducing creativity in the production process is a challenging task before the entrepreneur. Hence, creativity is an essential element of entrepreneurship.

(5) Managerial skill and leadership : The entrepreneur who has strong passion of doing or creating something new rather than just to earn profit will become a successful entrepreneur. Managerial skills and leadership are the most important features of successful entrepreneur. Other skills are not considered so important. Entrepreneur must be a good leader and manager of the groups working under him.

(6) Skilful management : The efficient and skilful management of the organisation is an important quality of entrepreneurship. With the help of professional management and skilled managers, entrepreneurship becomes easy and successful activity. The success of any entrepreneurship depends on its skilful management.

(7) Risk-bearing : An entrepreneur has to undertake many risks including fall in prices, changes in fashions, earthquake, etc. All these risks cannot be insured with insurance companies. A risk which cannot be insured against and measured is called uncertainty. Entrepreneur buys factors of production at certain prices to combine their contributions into the products and then sells those products at uncertain prices in future. Thus, entrepreneur is a risk-bearing agent of production.

(8) Gap filling function : Gap filling is considered as the most important feature of entrepreneur. It is the job of entrepreneur to find the gap and fill it or make up the deficiencies which always exist in the knowledge about the production function. Entrepreneur must have all the solutions of the problems.

Question 2.
What is Entrepreneurship Development Programmes (EDP)?
Answer:
An entrepreneurship development
programme has been defined as “a programme designed to help a person in strengthening his entrepreneurial motive and in acquiring skills and capabilities necessary for playing his entrepreneurial role efficiently”.

EDP was first introduced in Gujarat in 1970 and was sponsored by the Gujarat Industrial Investment Corporation. EDP is basically a device through which people with entrepreneurial talents are identified, motivated to take up new industrial venture and guided in all aspects of starting a venture or an enterprise.

The following are the main objectives of EDP:
(1) Paster entreprenurial growth : The main objective is to increase the rate of all round entrepreneurial growth through training and educating them to develop the capability, talent and skills of existing entrepreneur.

(2) Optimum use of available resources : Another important objective is to use available resources to optimum level which result into minimisation of wastages and reduction in the overall cost of production. It also saves the invaluable resources for the future generation.

(3) Development of backward regions and improve economic status of socially disadvantage group : Its main objective is to establish different types of industries and business enterprise in the backward regions of the country. This leads to more employment opportunities and more income and savings of the people in backward group. By providing employment and other benefits to socially disadvantaged groups, EDP helps to improve their economic status.

(4) Generation of Employment opportunities : One of the important objectives of EDP is to generate employment opportunities for jobless people in the country by developing industries and business for them.

(5) Widening base for small and medium industries : The EDP helps to create, develop and widen the base for small and medium industries by strengthening them and create more and more entrepreneurs in the country. It helps in making country a job creator and not job seeker.

7. Answer the following

Question 1.
Define entrepreneur. Explain characteristics of entrepreneur.
Answer:
[A] Definition : According to Webster dictionary, “An entrepreneur is a person who starts a business and is willing to risk loss in order to make money.” The entrepreneurs are passionate to innovate, lend, invent or pioneer with a disruptive product or technology. A small businessman, a plumber or a founder of huge company are entrepreneurs.

[B] Characteristics : The characteristics of entrepreneur are as follows:
(1) Intellectual capabilities : An entrepreneur is a creative thinker. He always thinks more creatively and better than others. He always give innovative ideas which is the sign of his intellectual capabilities. He has ability to analyse any situation and take proper decision.

(2) Future vision : The entrepreneurs have the ability of foreseeing the future market conditions. He can take appropriate decision by considering recent market situations and changes in market conditions. He must have knowledge of external business environment. This enables them to take timely actions.

(3) Hard work : An entrepreneur is ready to work hard. Hard work is necessary in any type of venture or business activity to make it more successful. He is required to work more tediously, sincerely and seriously for long terms.

(4) Technical knowledge : The entrepreneur should have advance technical knowledge about the products and service, plans of production, etc. Entrepreneur should also update his technical knowledge from time to time to understand latest changes take place in technology.

(5) Communication skills : An entrepreneur needs to communicate effectively with different people like customers, suppliers, creditors, employees, etc. from time to time. He should have good communication skill and command over language he speaks, to be able to express his ideas and strategies effectively. Good communication skills mean proper understanding between sender and the receiver of the message.

(6) Highly optimistic : He should have positive thinking and positive approach in all the activities he undertakes. He is always hopeful and confident about the market situations even in failure or difficult times. It helps him to take the business out of difficulties and make it successful.

(7) Risk-bearing capacity : This is one of the main characteristics of an entrepreneur. He should be calculative in taking risk. He should be prepared to face challenges and look for opportunities in every adverse situation of business.

(8) Self confidence : He should be self confident to achieve his organisational goals. He I should always keep himself confident and motivated to face various obstacles and come out victorious every time in every challenge he faces.

Question 2.
Define entrepreneur. Explain its functions.
Answer:
[A] Definition : According to Webster dictionary, “An entrepreneur is a person who starts a business and is willing to risk loss in order to make money.” The entrepreneurs are passionate to innovate, lend, invent or pioneer with a disruptive product or technology. A small businessman, a plumber or a founder of huge company are entrepreneurs.

[B] Functions : The functions of an entrepreneur are:
(1) Innovation : Usually, an entrepreneur has an innovative mind. He introduces new combination of means of production. He introduces something new or something different that would give his business a competitive advantage. Innovation sometimes involves problem solving and entrepreneur gets pleasure by using his talents to solve those problems.

(2) Determination of objectives : An entrepreneur is required to decide the aims and objectives of the business enterprise he intends to establish. He has to change those aims and ; objectives as per changing conditions or accept those which are beneficial to the enterprise as per the market situation.

(3) Development of market: An entrepreneur has to find new, different and innovative ways to market his products and services. As the markets are developing constantly, he has to conduct surveys, research to understand customer’s demand.

(4) New technology : Entrepreneur has to install new, advanced and efficient technology, new machinery, new and scientific methods of production to save overall cost and to improve the methods of production.

(5) Good relations : Prosperity, growth and development of enterprise mostly depend on the cordial and efficient relations of the superiors, subordinates and all employees. In this respect, co-ordination among the employees plays a significant role to make business enterprise successful.

(6) Organising funds : Finance is required to meet working capital and fixed capital needs of business. The entrepreneur has to raise adequate financial resources to keep enterprise in living condition. For this purpose, he has to keep good relation with the existing as well as potential investors.

(7) Taking decisions : Timely, correct and wise decisions are most important to run a successful business. An entrepreneur has to evaluate pros and cons of every business decision.

Balbharti Maharashtra State Board 12th Physics Textbook Solutions Chapter 7 Wave Optics Textbook Exercise Questions and Answers.

Maharashtra State Board 12th Physics Solutions Chapter 7 Wave Optics

1. Choose the correct option.

i) Which of the following phenomenon proves that light is a transverse wave?
(A) reflection
(B) interference
(C) diffraction
(D) polarization
Answer:
(D) polarization

ii) Which property of light does not change when it travels from one medium to another?
(A) velocity
(B) wavelength
(C) amplitude
(D) frequency
Answer:
(D) frequency

iii) When unpolarized light is passed through a polarizer, its intensity
(A) increases
(B) decreases
(C) remains unchanged
(D) depends on the orientation of the polarizer
Answer:
(B) decreases

iv) In Young’s double slit experiment, the two coherent sources have different intensities. If the ratio of maximum intensity to the minimum intensity in the interference pattern produced is 25:1. What was the ratio of intensities of the two sources?
(A) 5:1
(B) 25:1
(C) 3:2
(D) 9:4
Answer:
(D) 9:4

v) In Young’s double slit experiment, a thin uniform sheet of glass is kept in front of the two slits, parallel to the screen having the slits. The resulting interference pattern will satisfy
(A) The interference pattern will remain unchanged
(B) The fringe width will decrease
(C) The fringe width will increase
(D) The fringes will shift.
Answer:
(A) The interference pattern will remain unchanged

2. Answer in brief.

i) What are primary and secondary sources of light?
Answer:
(1) Primary sources of light: The sources that emit light on their own are called primary sources. This emission of light may be due to
(a) the high temperature of the source, e.g., the Sun, the stars, objects heated to high temperature, a flame, etc.
(b) the effect of current being passed through the source, e.g., tubelight, TV, etc.
(c) chemical or nuclear reactions taking place in the source, e.g., firecrackers, nuclear energy generators, etc.

(2) Secondary sources of light: Some sources are not self luminous, i.e., they do not emit light on their own, but reflect or scatter the light incident on them. Such sources of light are called secondary sources, e.g. the moon, the planets, objects such as humans, animals, plants, etc. These objects are visible due to reflected light.
Many of the sources that we see around are secondary sources and most of them are extended sources.

ii) What is a wavefront? How is it related to rays of light? What is the shape of the wavefront at a point far away from the source of light?
Answer:
Wavefront or wave surface : The locus of all points where waves starting simultaneously from a source reach at the same instant of time and hence the particles at the points oscillate with the same phase, is called a wavefront or wave surface.

Consider a point source of light O in a homogeneous isotropic medium in which the speed of light is v. The source emits light in all directions. In time t, the disturbance (light energy) from the source, covers a distance vt in all directions, i.e., it reaches out to all points which are at a distance vt from the point source. The locus of these points which are in the same phase is the surface of a sphere with the centre O and radius vt. It is a spherical wavefront.

In a given medium, a set of straight lines can be drawn which are perpendicular to the wavefront. According to Huygens, these straight lines are the rays of light. Thus, rays are always normal to the wavefront. In the case of a spherical wavefront, the rays are radial.

If a wavefront has travelled a large distance away from the source, a small portion of this wavefront appears to be plane. This part is a plane wavefront.

iii) Why are multiple colours observed over a thin film of oil floating on water? Explain with the help of a diagram.
Answer:
Several phenomena that we come across in our day to day life are caused by interference and diffraction of light. These are the vigorous colours of soap bubbles as well as those seen in a thin oil film on the surface of water, the bright colours of butterflies and peacocks etc. Most of these colours are not due to pigments which absorb specific colours but are due to interference of light waves that are reflected by different layers.

Interference due to a thin film:

The brilliant colours of soap bubbles and thin films on the surface of water are due to the interference of light waves reflected from the upper and lower surfaces of the film. The two rays have a path difference which depends on the point on the film that is being viewed. This is shown in above figure.

The incident wave gets partially reflected from upper surface as shown by ray AE. The rest of the light gets refracted and travels along AB. At B it again gets partially reflected and travels along BC. At C it refracts into air and travels along CF. The parallel rays AE and CF have a phase difference due to their different path lengths in different media. As can be seen from the figure, the phase difference depends on the angle of incidence θ₁, i.e., the angle of incidence at the top surface which is the angle of viewing, and also on the wavelength of the light as the refractive index of the material of the thin film depends on it. The two waves propagating along AE and CF interfere producing maxima and minima for different colours at different angles of viewing. One sees different colours when the film is viewed at different angles.

As the reflection is from the denser boundary, there is an additional phase difference of π radians (or an
additional path difference λ). This should be taken into account for mathematical analysis.

iv) In Young’s double slit experiment what will we observe on the screen when white light is incident on the slits but one slit is covered with a red filter and the other with a violet filter? Give reasons for your answer.
Answer:
In Young’s double-slit experiment, when white light is incident on the slits and one of the slit is covered with a red filter, the light passing through this slit will emerge as the light having red colour. The other slit which is covered with a violet filter, will give light having violet colour as emergent light. The interference fringes will involve mixing of red and violet colours. At some points, fringes will be red if constructive interference occurs for red colour and destructive interference occurs for violet colour. At some points, fringes will be violet if constructive interference occurs for violet colour and destructive interference occurs for red colour. The central fringe will be bright with mixing of red and violet colours.

v) Explain what is optical path length. How is it different from actual path length?
Answer:
Consider, a light wave of angular frequency ω and wave vector k travelling through vacuum along the x-direction. The phase of this wave is (kx-ωt). The speed of light in vacuum is c and that in medium is v.
k = \(\frac{2 \pi}{\lambda}\) = \(\frac{2 \pi v}{v \lambda}\) = \(\frac{\omega}{v}\) as ω = 2πv and v = vλ, where v is the frequency of light.

If the wave travels a distance ∆x, its phase changes by ∆φ = k∆x = ω∆x/v.
Similarly, if the wave is travelling in vacuum, k = ω/c and ∆φ = ω∆x/c
Now, consider a wave travelling a distance ∆x in the medium, the phase difference generated is,
∆φ’ = k’∆x = ωn∆x/c = ω∆x’/c … (1)
where ∆x’ = n∆x … (2)
The distance n∆x is called the optical path length of the light in the medium; it is the distance the light would have travelled in the same time t in vacuum (with the speed c).

The optical path length in a medium is the corresonding path in vacuum that the light travels in the same time as it takes in the given medium.

Thus, a distance d travelled in a medium of refractive index n introduces a path difference = nd – d = d(n – 1) over a ray travelling equal distance through vacuum.

Question 3.
Derive the laws of reflection of light using Huygens’ principle.
Answer:
Consider a plane wavefront AB of monochromatic light propagating in the direction A’A incident obliquely at an angle i on a plane refracting surface MN. This plane refracting surface MN separates two uniform and optically transparent mediums.
Let v₁ and v₂ be the speeds of light in medium 1 (say, a rarer medium) and medium 2 (a denser medium) respectively.

When the wavefront reaches MN at point A at t = 0, A becomes a secondary source and emits secondary waves in the second medium, while ray B’B reaches the surface MN at C at time t = T. Thus, BC = v₁T. During the time T, the secondary wavelet originating at A covers a distance AE in the denser medium with radius v₂T.

As all the points on CE are in the same phase of wave motion, CE represents the refracted wavefront in the denser medium. CE is the tangent to the secondary wavelet starting from A. It is also a common tangent to all the secondary wavelets emitted by points between A and C. PP’ is the normal to the boundary at A.
∠A’AP = ∠BAC = the angle of incidence (i) and ∠P’AE = ∠ACE = the angle of refraction (r).
From ∆ABC and ∆AEC,

By definition, the refractive index of medium 2 with respect to medium 1,

Here, n₁ and n₂ are the absolute refractive indices of medium 1 and medium 2 respectively. Eq. (1) is Snell’s law of refraction. Also, it can be seen from the figure, that the incident ray and the refracted ray lie on the opposite sides of the normal and all three of them lie in the same plane.

Thus, the laws of refraction of light can be deduced by Huygens’ construction of a plane wavefront.
If v₁ > v₂, i.e. n₁ < n₂, then r < i (bending of the refracted ray towards the normal).
[Notes :
(1) Quite often, the terms vacuum and free space are used in the same sense. Absolute vacuum or a perfect vacuum-a region of space devoid of material, particles – does not exist. The term vacuum is also used to mean a region of space occupied by a gas at very low pressure. Free space means a region of space devoid of matter and fields. Its refractive index is 1 (by definition). Its temperature is 0 K. ε₀ and μ₀ are defined for free space. The refractive index of a medium with respect to air is very close to the absolute refractive index of the medium as the speed of light in air is very close to that in free space.
(2) There is no lateral inversion in refraction.
(3) There is no bending of light when the angle of incidence is zero (normal incidence), r = 0 for i = 0.]

Question 4.
Derive the laws of refraction of light using Huygens’ principle.
Answer:
Consider a plane wavefront AB of monochromatic light propagating in the direction A’A incident obliquely at an angle i on a plane refracting surface MN. This plane refracting surface MN separates two uniform and optically transparent mediums.
Let v₁ and v₂ be the speeds of light in medium 1 (say, a rarer medium) and medium 2 (a denser
medium) respectively.

When the wavefront reaches MN at point A at t = 0, A becomes a secondary source and emits secondary waves in the second medium, while ray B’B reaches the surface MN at C at time t = T. Thus, BC = v₁T. During the time T, the secondary wavelet originating at A covers a distance AE in the denser medium with radius v₂T.

As all the points on CE are in the same phase of wave motion, CE represents the refracted wavefront in the denser medium. CE is the tangent to the secondary wavelet starting from A. It is also a common tangent to all the secondary wavelets emitted by points between A and C. PP’ is the normal to the boundary at A.
∠A’AP = ∠BAC = the angle of incidence (i) and ∠P’AE = ∠ACE = the angle of refraction (r).
From ∆ABC and ∆AEC,

By definition, the refractive index of medium 2 with respect to medium 1,

Here, n₁ and n₂ are the absolute refractive indices of medium 1 and medium 2 respectively. Eq. (1) is Snell’s law of refraction. Also, it can be seen from the figure, that the incident ray and the refracted ray lie on the opposite sides of the normal and all three of them lie in the same plane.

Thus, the laws of refraction of light can be deduced by Huygens’ construction of a plane wavefront.
If v₁ > v₂, i.e. n₁ < n₂, then r < i (bending of the refracted ray towards the normal).
[Notes :

1. Quite often, the terms vacuum and free space are used in the same sense. Absolute vacuum or a perfect vacuum-a region of space devoid of material, particles – does not exist. The term vacuum is also used to mean a region of space occupied by a gas at very low pressure. Free space means a region of space devoid of matter and fields. Its refractive index is 1 (by definition). Its temperature is 0 K. ε₀ and μ₀ are defined for free space. The refractive index of a medium with respect to air is very close to the absolute refractive index of the medium as the speed of light in air is very close to that in free space.
2. There is no lateral inversion in refraction.
3. There is no bending of light when the angle of incidence is zero (normal incidence), r = 0 for i = 0.]

Question 5.
Explain what is meant by polarization and derive Malus’ law.
Answer:
According to the electromagnetic theory of light, a light wave consists of electric and magnetic fields vibrating at right angles to each other and to the direction of propagation of the wave. If the vibrations of \(\vec{E}\) in a light wave are in all directions perpendicular to the direction of propagation of light, the wave is said to be unpolarized.

If the vibrations of the electric field \(\vec{E}\) in a light wave are confined to a single plane containing the direction of propagation of the wave so that its electric field is restricted along one particular direction at right angles to the direction of propagation of the wave, the wave is said to be plane-polarized or linearly polarized.

This phenomenon of restricting the vibrations of light, i.e., of the electric field vector in a particular direction, which is perpendicular to the direction of the propagation of the wave is called polarization of light.

Consider an unpolarized light wave travelling along the x-direction. Let c, v and λ be the speed, frequency and wavelength, respectively, of the wave. The magnitude of its electric field (\(\vec{E}\)) is,
E = E₀ sin (kx – ωt), where E₀ = E_max = amplitude of the wave, ω = 2πv = angular frequency of the wave and k = \(\frac{2 \pi}{\lambda}\) = magnitude of the wave vector or propagation vector.

The intensity of the wave is proportional to \(\left|E_{0}\right|^{2}\). The direction of the electric field can be anywhere in the y-z plane. This wave is passed through two identical polarizers as shown in below figure.

When a wave with its electric field inclined at an angle φ to the axis of the first polarizer is passed through the polarizer, the component E₀ cos φ will pass through it. The other component E₀ sin φ which is perpendicular to it will be blocked.

Now, after passing through this polarizer, the intensity of this wave will be proportional to the square of its amplitude, i.e., proportional to |E₀ cos φ|².

The intensity of the plane-polarized wave emerging from the first polarizer can be obtained by averaging | E₀ cos φ|² over all values of φ between 0 and 180°. The intensity of the wave will be
proportional to \(\frac{1}{2}\)|E₀|² as the average value of cos² φ over this range is \(\frac{1}{2}\). Thus the intensity of an unpolarized wave reduces by half after passing through a polarizer.

When the plane-polarized wave emerges from the first polarizer, let us assume that its electric field (\(\overrightarrow{E_{1}}\)) is along the y-direction. Thus, this electric field is,
\(\overrightarrow{E_{1}}\) = \(\hat{\mathrm{j}}\) E₁₀ sin (kx – ωt) …. (1)
where, E₁₀ is the amplitude of this polarized wave. The intensity of the polarized wave,
I₁ ∝ |E₁₀|² …(2)

Now this wave passes through the second polarizer whose polarization axis (transmission axis) makes an angle θ with the y-direction. This allows only the component E₁₀ cos θ to pass through it. Thus, the amplitude of the wave which passes through the second polarizer is E₂₀ = E₁₀ cos θ and its intensity,
I₂ ∝ | E₂₀|²
∴ I₂ ∝ | E₁₀|² cos²θ
∴ I₂ = I₁ cos₂θ … (3)

Thus, when plane-polarized light of intensity I₁ is incident on the second identical polarizer, the intensity of light transmitted by the second polarizer varies as cos²θ, i.e., I₂ = I₁ cos²θ, where θ is the angle between the transmission axes of the two polarizers. This is known as Malus’ law

[Note : Etienne Louis Malus (1775-1812), French military engineer and physicist, discovered in 1809 that light can be polarized by reflection. He was the first to use the word polarization, but his arguments were based on Newton’s corpuscular theory.]

Question 6.
What is Brewster’s law? Derive the formula for Brewster angle.
Answer:
Brewster’s law : The tangent of the polarizing angle is equal to the refractive index of the reflecting medium with respect to the surrounding (₁n₂). If θ_B is the polarizing angle,
tan θ_B = ₁n₂ = \(\frac{n_{2}}{n_{1}}\)
Here n₁ is the absolute refractive index of the surrounding and n₂ is that of the reflecting medium.
The angle θ_B is called the Brewster angle.

Consider a ray of unpolarized monochromatic light incident at an angle θ_B on a boundary between two transparent media as shown in below figure. Medium 1 is a rarer medium with refractive index n₁ and medium 2 is a denser medium with refractive index n₂. Part of incident light gets refracted and the rest

gets reflected. The degree of polarization of the reflected ray varies with the angle of incidence.
The electric field of the incident wave is in the plane perpendicular to the direction of propagation of incident light. This electric field can be resolved into a component parallel to the plane of the paper, shown by double arrows, and a component perpendicular to the plane of the paper shown by dots, both having equal magnitude. Generally, the reflected and refracted rays are partially polarized, i.e., the two components do not have equal magnitude.

In 1812, Sir David Brewster discovered that for a particular angle of incidence. θ_B, the reflected wave is completely plane-polarized with its electric field perpendicular to the plane of the paper while the refracted wave is partially polarized. This particular angle of incidence (θ_B) is called the Brewster angle.

For this angle of incidence, the refracted and reflected rays are perpendicular to each other.
For angle of refraction θ_r,
θ_B + θ_r = 90° …… (1)
From Snell’s law of refraction,
∴ n₁ sin θ_B = n₂ sin θ_r … (2)
From Eqs. (1) and (2), we have,
n₁ sin θ_B = n₂ sin (90° – θ_B) = n₂ cos θ_B

This is called Brewster’s law.

Question 7.
Describe Young’s double slit interference experiment and derive conditions for occurrence of dark and bright fringes on the screen. Define fringe width and derive a formula for it.
Answer:
Description of Young’s double-slit interference experiment:

1. A plane wavefront is obtained by placing a linear source S of monochromatic light at the focus of a convex lens. It is then made to pass through an opaque screen AB having two narrow and similar slits S₁ and S₂. S₁ and S₂ are equidistant from S so that the wavefronts starting simultaneously from S and reaching S₁ and S₂ at the same time are in phase. A screen PQ is placed at some distance from screen AB as shown in below figure
   
2. S₁ and S₂ act as secondary sources. The crests/-troughs of the secondary wavelets superpose and interfere constructively along straight lines joining the black dots shown in above figure. The point where these lines meet the screen have high intensity and are bright.
3. Similarly, there are points shown with red dots where the crest of one wave coincides with the trough of the other. The corresponding points on the screen are dark due to destructive interference.
4. These dark and bright regions are called fringes or bands and the whole pattern is called interference pattern.

Conditions for occurence of dark and bright fringes on the screen :

Consider Young’s double-slit experimental set up. Two narrow coherent light sources are obtained by wavefront splitting as monochromatic light of wavelength λ emerges out of two narrow and closely spaced, parallel slits S₁ and S₂ of equal widths. The separation S₁S₂ = d is very small. The interference pattern is observed on a screen placed parallel to the plane of and at considerable distance D (D » d) from the slits. OO’ is the perpendicular bisector of segment S₁S₂.

Consider, a point P on the screen at a distance y from O’ (y « D). The two light waves from S₁ and S₂ reach P along paths S₁P and S₂P, respectively. If the path difference (∆l) between S₁P and S₂P is an integral multiple of λ, the two waves arriving there will interfere constructively producing a bright fringe at P. On the contrary, if the path difference between S₁P and S₂P is half integral multiple of λ, there will be destructive interference and a dark fringe will be produced at P.
From above figure,
(S₂P)² = (S₂S₂)² + (PS₂‘)²
= (S₂S₂‘)² + (PO’ + O’S₂‘)²

Expression for the fringe width (or band width) : The distance between consecutive bright (or dark) fringes is called the fringe width (or band width) W. Point P will be bright (maximum intensity), if the path difference, ∆l = y_n\(\frac{d}{D}\) = nλ where n = 0,1, 2, 3…, Point P will be dark (minimum intensity equal to zero), if y_m\(\frac{d}{D}\) = (2m – 1)\(\frac{\lambda}{2}\), where, m = 1, 2, 3…,
Thus, for bright fringes (or bands),

These conditions show that the bright and dark fringes (or bands) occur alternately and are equally – spaced. For Point O’, the path difference (S₂O’ – S₁O’) = 0. Hence, point O’ will be bright. It corresponds to the centre of the central bright fringe (or band). On both sides of O’, the interference pattern consists of alternate dark and bright fringes (or band) parallel to the slit.

Let y_n and y_{n + 1}, be the distances of the nth and (n + 1)^th bright fringes from the central bright fringe.

Alternately, let y_m and y_{m + 1} be the distances of the m th and (m + 1)^th dark fringes respectively from the central bright fringe.

Eqs. (7) and (11) show that the fringe width is the same for bright and dark fringes.

[Note : In the first approximation, the path difference is d sin θ.]

Question 8.
What are the conditions for obtaining good interference pattern? Give reasons.
Answer:
The conditions necessary for obtaining well defined and steady interference pattern :

1. The two sources of light should be coherent:
   The two sources must maintain their phase relation during the time required for observation. If the phases and phase difference vary with time, the positions of maxima and minima will also change with time and consequently the interference pattern will change randomly and rapidly, and steady interference pattern would not be observed. For coherence, the two secondary sources must be derived from a single original source.
2. The light should be monochromatic :
   Otherwise, interference will result in complex coloured bands (fringes) because the separation of successive bright bands (fringes) is different for different colours. It also may produce overlapping bands.
3. The two light sources should be of equal brightness, i.e., the waves must have the same amplitude.
   The interfering light waves should have the same amplitude. Then, the points where the waves meet in opposite phase will be completely dark (zero intensity). This will increase the contrast of the interference pattern and make it more distinct.
4. The two light sources should be narrow :
   If the source apertures are wide in comparison with the light wavelength, each source will be equivalent to multiple narrow sources and the superimposed pattern will consist of bright and less bright fringes. That is, the interference pattern will not be well defined.
5. The interfering light waves should be in the same state of polarization :
   Otherwise, the points where the waves meet in opposite phase will not be completely dark and the interference pattern will not be distinct.
6. The two light sources should be closely spaced and the distance between the screen and the sources should be large : Both these conditions are desirable for appreciable fringe separation. The separation of successive bright or dark fringes is inversely proportional to the closeness of the slits and directly proportional to the screen distance.

Question 9.
What is meant by coherent sources? What are the two methods for obtaining coherent sources in the laboratory?
Answer:
Coherent sources : Two sources of light are said to be coherent if the phase difference between the emitted waves remains constant.

It is not possible to observe interference pattern with light from any two different sources. This is because, no observable interference phenomenon occurs by superposing light from two different sources. This happens due to the fact that different sources emit waves of different frequencies. Even if the two sources emit light of the same frequency, the phase difference between the wave trains from them fluctuates randomly and rapidly, i.e., they are not coherent.
Consequently, the interference pattern will change randomly and rapidly, and steady interference pattern would not be observed.

In the laboratory, coherent sources can be obtained by using
(1) Lloyd’s mirror and
(2) Fresnel’s biprism.

(1) Lloyd’s mirror : A plane polished mirror is kept at some distance from the source of monochromatic light and light is made incident on the mirror at a grazing angle.

Some light falls directly on the screen as shown by the black lines in above figure, while some light falls on the screen after reflection from the mirror as shown by red lines. The reflected light appears to come from a virtual source and thus two sources can be obtained. These two sources are coherent as they are derived from a single source. Superposition of the waves coming from these coherent sources, under appropriate conditions, gives rise to interference pattern consisting of alternate bright and dark bands on the screen as shown in the figure.

(2) Fresnel’s biprism : It is a single prism having an obtuse angle of about 178° and the other two angles of about 1° each. The biprism can be considered as made of two thin prisms of very small refracting angle of about 1°. The source, in the form of an illuminated narrow slit, is aligned parallel to the refracting edge of the biprism. Monochromatic light from the source is made to pass through that narrow slit and fall on the biprism.

Two virtual images S₁ and S₂ are formed by the two halves of the biprism. These are coherent sources which are obtained from a single secondary source S. The two waves coming from S₁ and S₂ interfere under appropriate conditions and form interference fringes, like those obtained in Young’s double-slit experiment, as shown in the figure in the shaded region. The formula for y is the same as in Young’s experiment.

Question 10.
What is diffraction of light? How does it differ from interference? What are Fraunhoffer and Fresnel diffractions?
Answer:
1. Phenomenon of diffraction of light: When light passes by the edge of an obstacle or through a small opening or a narrow slit and falls on a screen, the principle of rectilinear propagation of light from geometrical optics predicts a sharp shadow. However, it is found that some of the light deviates from its rectilinear path and penetrates into the region of the geometrical shadow. This is a general characteristic of wave phenomena, which occurs whenever a portion of the wavefront is obstructed in some way. This bending of light waves at an edge into the region of geometrical shadow is called diffraction of light.

2. Differences between interference and diffraction :

1. The term interference is used to characterise the superposition of a few coherent waves (say, two). But when the superposition at a point involves a large number of waves coming from different parts of the same wavefront, the effect is referred to as diffraction.
2. Double-slit interference fringes are all of equal width. In single-slit diffraction pattern, only the non-central maxima are of equal width which is half of that of the central maximum.
3. In double-slit interference, the bright and dark fringes are equally spaced. In diffraction, only the non-central maxima lie approximately halfway between the minima.
4. In double-slit interference, bright fringes are of equal intensity. In diffraction, successive non-central maxima decrease rapidly in intensity.

[Note : Interference and diffraction both have their origin in the principle of superposition of waves. There is no physical difference between them. It is just a question of usage. When there are only a few sources, say two, the phenomenon is usually called interference. But, if there is a large number of sources the word diffraction is used.]

3. Diffraction can be classified into two types depending on the distances involved in the experimental setup :

(A) Fraunhofer diffraction : In this class of diffraction, both the source and the screen are at infinite distances from the aperture. This is achieved by placing the source at the focus of a convex lens and the screen at the focal plane of another convex lens.

(B) Fresnel diffraction : In this class of diffraction, either the source of light or the screen or both are at finite distances from the diffracting aperture. The incident wavefront is either cylindrical or spherical depending on the source. A lens is not needed to observe the diffraction pattern on the screen.

Question 11.
Derive the conditions for bright and dark fringes produced due to diffraction by a single slit.
Answer:
When a parallel beam of monochromatic light of wavelength λ illuminates a single slit of finite width a, we observe on a screen some distance from the slit, a broad pattern of alternate dark and bright fringes. The pattern consists of a central bright fringe, with successive dark and bright fringes of diminishing intensity on both sides. This is called ‘ the diffraction pattern of a single slit.

Consider a single slit illuminated with a parallel beam of monochromatic light perpendicular to the plane of the slit. The diffraction pattern is obtained on a screen at a distance D (» a) from the slit and at the focal plane of the convex lens, Fig. 7.33.

We can imagine the single slit as being made up of a large number of Huygens’ sources evenly distributed over the width of the slit. Then the maxima and minima of the pattern arise from the interference of the various Huygens’ wavelets.

Now, imagine the single slit as made up of two adjacent slits, each of width a/2. Since, the incident plane wavefronts are parallel to the plane of the slit, all the Huygens sources at the slit will be in phase. They will therefore also in phase at the point P₀ on the screen, where P₀ is equidistant from all the Huygens sources. At P₀, then, we get the central maximum.

For the first minimum of intensity on the screen, the path difference between the waves from the Huygens sources A and O (or O and B) is λ/2, which is the condition for destructive interference. Suppose, the nodal line OP for the first minimum subtends an angle θ at the slit; θ is very small. With P as the centre and PA as radius, strike an arc intersecting PB at C. Since, D » a, the arc AC can be considered a straight line at right angles to PB. Then, ∆ABC is a right-angled triangle similar to ∆OP₀P.
This means that, ∠BAC = θ
∴ BC = a sinθ
∴ Difference in path length,
BC = PB – PA = (PB – PO) + (PO – PA)

(∵ θ is very small and in radian)
The other nodal lines of intensity minima can be understood in a similar way. In general, then, for the with minimum (m = ±1, ±2, ±3, …).
θ_m = \(\frac{m \lambda}{a}\) (mth minimum) … (2)
as θ_m is very small and in radian.
Between the successive minima, the intensity rises to secondary maxima when the path difference is an odd-integral multiple of \(\frac{\lambda}{2}\) :
a sin θ_m = (2m + 1)\(\frac{\lambda}{2}\) = (m + \(\frac{1}{2}\))λ
i.e., at angles given by,
θ_m \(\simeq\) sin θ_m = (m + \(\frac{1}{2}\))\(\frac{\lambda}{a}\)
(with secondary maximum) … (3)

Question 12.
Describe what is Rayleigh’s criterion for resolution. Explain it for a telescope and a microscope.
Answer:
Rayleigh’s criterion for minimum resolution : Two overlapping diffraction patterns due to two point sources are acceptably or just resolved if the centre of the central peak of one diffraction pattern is as far as the first minimum of the other pattern.

The ‘sharpness’ of the central maximum of a diffraction pattern is measured by the angular separation between the centre of the peak and the first minimum. It gives the limit of resolution.

Two overlapping diffraction patterns due to two point sources are not resolved if the angular separation between the central peaks is less than the limit of resolution. They are said to be just separate, or resolved, if the angular separation between the central peaks is equal to the limit of resolution. They are said to be well resolved if the angular separation between the central peaks is more than the limit of resolution.

Resolving power of an optical instrument:
The primary aim of using an optical instrument is to see fine details, whether observing a star system through a telescope or a living cell through a microscope. After passing through an optical system, light from two adjacent parts of the object should produce sharp, distinct (separate) images of those parts. The objective lens or mirror of a telescope or microscope acts like a circular aperture. The diffraction pattern of a circular aperture consists of a central bright spot (called the Airy disc and corresponds to the central maximum) and concentric dark and bright rings.

Light from two close objects or parts of an object after passing through the aperture of an optical system produces overlapping diffraction patterns that tend to obscure the image. If these diffraction patterns are so broad that their central maxima overlap substantially, it is difficult to decide if the intensity distribution is produced by two separate objects or by one.

The resolving power of an optical instrument, e.g., a telescope or microscope, is a measure of its ability to produce detectably separate images of objects that are close together.

Definition : The smallest linear or angular separation between two point objects which appear just resolved when viewed through an optical instrument is called the limit of resolution of the instrument and its reciprocal is called the resolving power of the instrument.

Rayleigh’s criterion for minimum resolution : Two overlapping diffraction patterns due to two point sources are acceptably or just resolved if the centre of the central peak of one diffraction pattern is as far as the first minimum of the other pattern.

The ‘sharpness’ of the central maximum of a diffraction pattern is measured by the angular separation between the centre of the peak and the first minimum. It gives the limit of resolution.

Two overlapping diffraction patterns due to two point sources are not resolved if the angular separation between the central peaks is less than the limit of resolution. They are said to be just separate, or resolved, if the angular separation between the central peaks is equal to the limit of resolution. They are said to be well resolved if the angular separation between the central peaks is more than the limit of resolution.

The resolving power of a telescope is defined as the reciprocal of the angular limit of resolution between two closely-spaced distant objects so that they are just resolved when seen through the telescope.

Consider two stars seen through a telescope. The diameter (D) of the objective lens or mirror corresponds to the diffracting aperture. For a distant point source, the first diffraction minimum is at an angle θ away from the centre such that

Dsinθ = 1.22 λ
where λ is the wavelength of light. The angle θ is usually so small that we can substitute sin θ \(\approx\) θ (θ in radian). Thus, the Airy disc for each star will be spread out over an angular half-width θ = 1.22 λ/D about its geometrical image point. The radius of the Airy disc at the focal plane of the objective lens is r = fθ = 1.22fλ/D, where / is the focal length of the objective.

When observing two closely-spaced stars, the Rayleigh criterion for just resolving the images as that of two point sources (instead of one) is met when the centre of one Airy disc falls on the first minimum of the other pattern. Thus, the angular limit (or angular separation) of resolution is
θ = \(\frac{1.22 \lambda}{D}\) …. (1)
and the linear separation between the images at the focal plane of the objective lens is
y = fθ …(2)
∴ Resolving power of a telescope,
R = \(\frac{1}{\theta}\) = \(\frac{D}{1.22 \lambda}\) … (3)
It depends

1. directly on the diameter of the objective lens or mirror,
2. inversely on the wavelength of the radiation.

Question 13.
Whitelight consists of wavelengths from 400 nm to 700 nm. What will be the wavelength range seen when white light is passed through glass of refractive index 1.55? [Ans: 258.1 – 451.6 nm]
Answer:
Let λ₁ and λ₂ be the wavelengths of light in water for 400 nm and 700 nm (wavelengths in vacuum) respectively. Let λ_a be the wavelength of light in vacuum.

The wavelength range seen when white light is passed through the glass would be 258.06 nm to 451.61 nm.

Question 14.
The optical path of a ray of light of a given wavelength travelling a distance of 3 cm in flint glass having refractive index 1.6 is same as that on travelling a distance x cm through a medium having refractive index 1.25. Determine the value of x.
[Ans: 3.84 cm]
Answer:
Let d_fg and d_m be the distances by the ray of light in the flint glass and the medium respectively. Also, let n_fg and n_m be the refractive indices of the flint glass and the medium respectively.
Data : d_fg = 3 cm, n_fg = 1.6, nm = 1.25,
Optical path = n_m × d_m = n_fg × d_fg

Thus, x cm = 3.84 cm
∴ x = 3.84
This is the value of x.

Question 15.
A double-slit arrangement produces interference fringes for sodium light (λ = 589 nm) that are 0.20° apart. What
is the angular fringe separation if the entire arrangement is immersed in water (n = 1.33)? [Ans: 0.15°]
Answer:
Data : θ₁ = 0.20°, n_w = 1.33
In the first approximation,
D sin θ₁ = y₁ and D sin θ₂ = y₂

∴ θ₂ = sin^-10.026
= 9′ = 0.15°
This is the required angular fringe separation.
OR
In the first approximation,

Question 16.
In a double-slit arrangement the slits are separated by a distance equal to 100 times the wavelength of the light passing through the slits.
(a) What is the angular separation in radians between the central maximum and an adjacent maximum?
(b) What is the distance between these maxima on a screen 50.0 cm from the slits?
[Ans: 0.01 rad, 0.5 cm]
Answer:
Data : d = 100λ, D = 50.0 cm
(a) The condition for maximum intensity in Young’s experiment is, d sin θ = nλ, n = 0, 1, 2 …,
The angle between the central maximum and its adjacent maximum can be determined by setting n equal to 1,
∴ d sin θ = λ

(b) The distance between these maxima on the screen is D sin θ = D\(\left(\frac{\lambda}{d}\right)\)
= (50.0 cm)\(\left(\frac{\lambda}{100 \lambda}\right)\)
= 0.50 cm

Question 17.
Unpolarized light with intensity I₀ is incident on two polaroids. The axis of the first polaroid makes an angle of 50° with the vertical, and the axis of the second polaroid is horizontal. What is the intensity of the light after it has passed through the second polaroid? [Ans: I₀/2 × (cos 40°)²]
Answer:
According to Malus’ law, when the unpolarized light with intensity I₀ is incident on the first polarizer, the polarizer polarizes this incident light. The intensity of light becomes I₁ = I₀/2.
Now, I₂ = I₁ cos²θ
∴ I₂ = \(\left(\frac{I_{0}}{2}\right)\) cos²θ
Also, the angle θ between the axes of the two polarizers is θ₂ – θ₁.

The intensity of light after it has passed through the second polaroid = \(\left(\frac{\boldsymbol{I}_{0}}{\mathbf{2}}\right)\)cos²40° = \(\frac{I_{0}}{2}\)(0.7660)²
= 0.2934 I₀

Question 18.
In a biprism experiment, the fringes are observed in the focal plane of the eyepiece at a distance of 1.2 m from the slits. The distance between the central bright band and the 20^th bright band is 0.4 cm. When a convex lens is placed between the biprism and the eyepiece, 90 cm from the eyepiece, the distance between the two virtual magnified images is found to be 0.9 cm. Determine the wavelength of light used. [Ans: 5000 Å]
Answer:
Data : D = 1.2 m
The distance between the central bright band and the 20^th bright band is 0.4 cm.
∴ y₂₀ = 0.4 cm = 0.4 × 10^-2 m
W = \(\frac{y_{20}}{20}\) = \(\frac{0.4}{20}\) × 10^-2 m = 2 × 10^-4 m,
d₁ = 0.9 cm = 0.9 × 10^-2m, v₁ = 90 cm = 0.9 m
∴ u₁ = D – v₁ = 1.2m – 0.9m = 0.3 m

Question 19.
In Fraunhoffer diffraction by a narrow slit, a screen is placed at a distance of 2 m from the lens to obtain the diffraction pattern. If the slit width is 0.2 mm and the first minimum is 5 mm on either side of the central maximum, find the wavelength of light. [Ans: 5000 Å]
Answer:
Data : D = 2 m, y_1d = 5 mm = 5 × 10^-3 m, a = 0.2 mm = 0.2 × 10^-3 m = 2 × 10^-4 m

This is the wavelength of light.

Question 20.
The intensity of the light coming from one of the slits in Young’s experiment is twice the intensity of the light coming from the other slit. What will be the approximate ratio of the intensities of the bright and dark fringes in the resulting interference pattern? [Ans: 34]
Answer:
Data : I₁ : I₂ = 2 : 1
If E₁₀ and E₂₀ are the amplitudes of the interfering waves, the ratio of the maximum intensity to the minimum intensity in the fringe system is


The ratio of the intensities of the bright and dark fringes in the resulting interference pattern is 34 : 1.

Question 21.
A parallel beam of green light of wavelength 550 nm passes through a slit of width 0.4 mm. The intensity pattern of the transmitted light is seen on a screen which is 40 cm away. What is the distance between the two first order minima? [Ans: 1.1 mm]
Answer:
Data : λ = 550 nm = 546 × 10^-9 m, a = 0.4 mm = 4 × 10^-4 m, D = 40 cm = 40 × 10^-2 m
y_md = m\(\frac{\lambda D}{a}\)
∴ y_1d = 1\(\frac{\lambda D}{a}\) and
2y_1d = \(\frac{2 \lambda D}{a}\)
= \(\frac{2 \times 550 \times 10^{-9} \times 40 \times 10^{-2}}{4 \times 10^{-4}}\)
= 2 × 550 × 10^-6 = 1092 × 10^-6
= 1.100 × 10^-3m = 1.100 mm
This is the distance between the two first order minima.

Question 22.
What must be the ratio of the slit width to the wavelength for a single slit to have the first diffraction minimum at 45.0°? [Ans: 1.274]
Answer:
Data : θ = 45°, m = 1
a sin θ = mλ for (m = 1, 2, 3… minima)
Here, m = 1 (First minimum)
∴ a sin 45° = (1) λ
∴ \(\frac{a}{\lambda}\) = \(\frac{1}{\sin 45^{\circ}}\) = 1.414
This is the required ratio.

Question 23.
Monochromatic electromagnetic radiation from a distant source passes through a slit. The diffraction pattern is observed on a screen 2.50 m from the slit. If the width of the central maximum is 6.00 mm, what is the slit width if the wavelength is
(a) 500 nm (visible light);
(b) 50 µm (infrared radiation);
(c) 0.500 nm (X-rays)?
[Ans: 0.4167 mm, 41.67 mm, 4.167 × 10^-4 mm]
Answer:
Data:2W = 6mm ∴ W= 3 mm = 3 × 10^-3 m, y = 2.5 m,
(a) λ₁ = 500 nm = 5 × 10^-7 m
(b) λ₂ = 50 μm = 5 × 10^-5 m
(c) λ₃ = 0.500 nm = 5 × 10^-10 m
Let a be the slit width.

Question 24.
A star is emitting light at the wavelength of 5000 Å. Determine the limit of resolution of a telescope having an objective of diameter of 200 inch. [Ans: 1.2 × 10^-7 rad]
Answer:
Data : λ = 5000 Å = 5 × 10^-7 m,
D = 200 × 2.54 cm = 5.08 m
θ = \(\frac{1.22 \lambda}{D}\)
= \(\frac{1.22 \times 5 \times 10^{-7}}{5.08}\)
= 1.2 × 10^-7 rad
This is the required quantity.

Question 25.
The distance between two consecutive bright fringes in a biprism experiment using light of wavelength 6000 Å is 0.32 mm by how much will the distance change if light of wavelength 4800 Å is used? [Ans: 0.064 mm]
Answer:
Data : λ₁ = 6000 Å = 6 × 10^-7m, λ₂ = 4800 Å = 4.8 × 10^-7m, W₁ = 0.32 mm = 3.2 × 10^-4m
Distance between consecutive bright fringes,

∴ ∆W = W₁ – W₂
= 3.2 × 10^-4m – 2.56 × 10^-4m
= 0.64 × 10^-4m
= 6.4 × 10^-5
= 0.064 mm
This is the required change in distance.

12th Physics Digest Chapter 7 Wave Optics Intext Questions and Answers

Use your brain power (Textbook Page No. 167)

What will you observe if

Question 1.
you look at a source of unpolarized light through a polarizer ?
Answer:
When a source of unpolarized light is viewed through a polarizer, the intensity of the light transmitted by the polarizer is reduced and hence the source appears less bright.

Question 2.
you look at the source through two polarizers and rotate one of them around the path of light for one full rotation?
Answer:
When a source of unpolarized light is viewed through two polarizers and the second polarizer is rotated gradually, the intensity of the light transmitted by the second polarizer goes on decreasing. When the axes of polarization of the two polarizers are at 90° to each other, light almost disappears depending on the quality of the polarizers. (Ideally the intensity of the transmitted light should be zero.) The light reappears, i.e., its intensity increases, when the second polarizer is rotated further, and the intensity of the light becomes maximum when the axes of polarization are parallel again.

Question 3.
instead of rotating only one of the polaroids, you rotate both polaroids simultaneously in the same direction?
Answer:
If both the polaroids are rotated simultaneously in the same direction with the same angular velocity, then there would be no change in the intensity of the transmitted light observed.

Can you tell? (Textbook Page No. 168)

Question 1.
If you look at the sky in a particular direction through a polaroid and rotate the polaroid around that direction what will you see ?
Answer:
As the scattered light is polarized, the sky appears bright and dim alternately.

Question 2.
Why does the sky appear to be blue while the clouds appear white ?
Answer:
The blue colour of the sky is because of the scattering of light by air molecules and dust particles in the atmosphere. As the wavelength of blue light is less than that of red light, the blue light is preferentially scattered than the light corresponding to other colours in the visible region. Clouds are seen due to scattering of light from lower parts of the atmosphere. The clouds contain the dust particles and water droplets which are sometimes large enough to scatter light of all the wavelengths such that the combined effect makes the clouds appear white.

Remember this (Textbook Page No. 171)

Question 1.
For the interference pattern to be clearly visible on the screen, the distance (D) between the slits and the screen should be much larger than the distance (d) between the two slits (S₁ and S₂), i.e., D » d.
Answer:
The condition for constructive interference at P is,
∆l = y_n\(\frac{d}{D}\) = nλ …. (1)
y_n being the position (y-coordinate) of nth bright fringe (n = 0, ±1, ±2, …).
∴ y_n = nλ\(\frac{D}{d}\) ….. (2)
Similarly, the position of mth (m = +1, ±2,…) dark fringe (destructive interference) is given by,
∆l = y_m\(\frac{d}{D}\) = (2m – 1)λ giving
y_m = (2m – 1)λ\(\frac{D}{d}\) …(3)
The distance between any two consecutive bright or dark fringes, i.e., the fringe width
= W = ∆y = y_{n + 1} – y_n = λ\(\frac{D}{d}\) …(4)
Conditions given by Eqs. (1) to (4) and hence the location of the fringes are derived assuming that the two sources S₁ and S₂ are in phase. If there is a non-zero phase difference between them it should be added appropriately. This will shift the entire fringe pattern but will not change the fringe width.

Do you know (Textbook Page No. 172 & 173)

Several phenomena that we come across in our day to day life are caused by interference and diffraction of light. These are the vigorous colours of soap bubbles as well as those seen in a thin oil film on the surface of water, the bright colours of butterflies and peacocks etc. Most of these colours are not due to pigments which absorb specific colours but are due to interference of light waves that are reflected by different layers.

Interference due to a thin film :
The brilliant colours of soap bubbles and thin films on the surface of water are due to the interference of light waves reflected from the upper and lower surfaces of the film. The two rays have a path difference which depends on the point on the film that is being viewed. This is shown in above figure.

The incident wave gets partially reflected from upper surface as shown by ray AE. The rest of the light gets refracted and travels along AB. At B it again gets partially reflected and travels along BC. At C it refracts into air and travels along CF. The parallel rays AE and CF have a phase difference due to their different path lengths in different media. As can be seen from the figure, the phase difference depends on the angle of incidence θ₁ i.e., the angle of incidence at the top surface which is the angle of viewing, and also on the wavelength of the light as the refractive index of the material of the thin film depends on it. The two waves propagating along AE and CF interfere producing maxima and minima for different colours at different angles of viewing. One sees different colours when the film is viewed at different angles.

As the reflection is from the denser boundary, there is an additional phase difference of π radians (or an additional path difference λ). This should be taken into account for mathematical analysis.

Balbharti Maharashtra State Board 12th Physics Textbook Solutions Chapter 8 Electrostatics Textbook Exercise Questions and Answers.

Maharashtra State Board 12th Physics Solutions Chapter 8 Electrostatics

1. Choose the correct option

i) A parallel plate capacitor is charged and then isolated. The effect of increasing the plate separation on charge, potential, capacitance respectively are
(A) Constant, decreases, decreases
(B) Increases, decreases, decreases
(C) Constant, decreases, increases
(D) Constant, increases, decreases
Answer:
(A) Constant, decreases, decreases

ii) A slab of material of dielectric constant k has the same area A as the plates of a parallel plate capacitor and has thickness (3/4d), where d is the separation of the plates. The change in capacitance when the slab is inserted between the plates is

Answer:
(D) C = \(\frac{\varepsilon_{0} A}{d}\left(\frac{4 k}{k+3}\right)\)

iii) Energy stored in a capacitor and dissipated during charging a capacitor bear a ratio.
(A) 1 : 1
(B) 1 : 2
(C) 2 : 1
(D) 1 : 3
Answer:
(A) 1 : 1

iv) Charge + q and -q are placed at points A and B respectively which are distance 2L apart. C is the mid point of A and B. The work done in moving a charge +Q along the semicircle CRD as shown in the figure below is

(C) \(\frac{q Q}{6 \pi \varepsilon_{0} L}\)
(D) \(\frac{-q Q}{6 \pi \varepsilon_{0} L}\)
Answer:
(A) \(-\frac{Q q_{1}}{6 \pi \varepsilon_{0} L}\)

v) A parallel plate capacitor has circular plates of radius 8 cm and plate separation 1mm. What will be the charge on the plates if a potential difference of 100 V is applied?
(A) 1.78 × 10^-8 C
(B) 1.78 × 10^-5 C
(C) 4.3 × 10⁴ C
(D) 2 × 10^-9 C
Answer:
(A) 1.78 × 10^-8 C

2. Answer in brief.

i) A charge q is moved from a point A above a dipole of dipole moment p to a point B below the dipole in equitorial plane without acceleration. Find the work done in this process.

Answer:
The equatorial plane of an electric dipole is an equipotential with V = 0. Therefore, the no work is done in moving a charge between two points in the equatorial plane of a dipole.

ii) If the difference between the radii of the two spheres of a spherical capacitor is increased, state whether the capacitance will increase or decrease.
Answer:
The capacitance of a spherical capacitor is C = 4πε₀\(\left(\frac{a b}{b-a}\right)\) where a and b are the radii of the concentric inner and outer conducting shells. Hence, the capacitance decreases if the difference b – a is increased.

iii) A metal plate is introduced between the plates of a charged parallel plate capacitor. What is its effect on the capacitance of the capacitor?
Answer:
Suppose the parallel-plate capacitor has capacitance C₀, plates of area A and separation d. Assume the metal sheet introduced has the same area A.

Case (1) : Finite thickness t. Free electrons in the sheet will migrate towards the positive plate of the capacitor. Then, the metal sheet is attracted towards whichever capacitor plate is closest and gets stuck to it, so that its potential is the same as that of that plate. The gap between the capacitor plates is reduced to d – t, so that the capacitance increases.

Case (2) : Negligible thickness. The thin metal sheet divides the gap into two of thicknesses d₁ and d₁ of capacitances C₁ = ε₀A/d₁ and C₂ = ε₀A/d₂ in series.
Their effective capacitance is
C = \(\frac{C_{1} C_{2}}{C_{1}+C_{2}}=\frac{\varepsilon_{0} A}{d_{1}+d_{2}}=\frac{\varepsilon_{0} A}{d}\) = C₀
i.e., the capacitance remains unchanged.

iv) The safest way to protect yourself from lightening is to be inside a car. Justify.
Answer:
There is danger of lightning strikes during a thunderstorm. Because trees are taller than people and therefore closer to the clouds above, they are more likely to get hit by lightnings. Similarly, a person standing in open ground is the tallest object and more likely to get hit by a lightning. But car with a metal body is an almost ideal Faraday cage. When a car is struck by lightning, the charge flows on the outside surface of the car to the ground but the electric field inside remains zero. This leaves the passengers inside unharmed.

v) A spherical shell of radius b with charge Q is expanded to a radius a. Find the work done by the electrical forces in the process.
Answer:
Consider a spherical conducting shell of radius r placed in a medium of permittivity ε. The mechanical force per unit area on the charged conductor is
f = \(\frac{F}{d S}=\frac{\sigma^{2}}{2 \varepsilon}\)
where a is the surface charge density on the conductor. Given the charge on the spherical shell is Q, (σ = Q/πr². The force acts outward, normal to the surface.

Suppose the force displaces a charged area element adS through a small distance dx, then the work done by the force is
dW = Fdx = (\(\frac{\sigma^{2}}{2 \varepsilon}\) dS) dx
During the displacement, the area element sweeps out a volume dV = dS ∙ dx.

Therefore, the work done by the force in expanding the shell from radius r = b to r = a is

This gives the required expression for the work done.

Question 3.
A dipole with its charges, -q and + q located at the points (0, -b, 0) and (0 +b, 0) is present in a uniform electric field E whose equipotential surfaces are planes parallel to the YZ planes.
(a) What is the direction of the electric field E?
(b) How much torque would the dipole experience in this field?
Answer:
(a) Given, the equipotentials of the external uniform electric field are planes parallel to the yz plane, the electric field \(\vec{E}=\pm E \hat{\mathrm{i}}\) that is, \(\overrightarrow{E}\) is parallel to the x-axis.

(b) From above figure, the dipole moment, \(\vec{p}=q(2 b) \hat{\mathrm{j}}\)
The torque on this dipole,

So that the magnitude of the torque is τ = 2qbE.
If \(\vec{E}\) is in the direction of the + x-axis, the torque \(\vec{\tau}\) is in the direction of – z-axis, while if \(\vec{E}\) is in the direction of the -x-axis, the torque \(\vec{\tau}\) is in the direction of + z-axis.

Question 4.
Three charges – q, + Q and – q are placed at equal distance on straight line. If the potential energy of the system of the three charges is zero, then what is the ratio of Q : q?
Answer:

In the above figure, the line joining the charges is shown as the x-axis with the origin at the + Q charge. Let q₁ = +Q, and q₂ = q₃ = – q. Let the two – q charges be at (- a, 0) and (a, 0), since the charges are given to be equidistant.
∴ r₂₁ = r₃₁ = a and r₃₂ = 2a
The total potential energy of the system of three charges is

This gives the required ratio.

Question 5.
A capacitor has some dielectric between its plates and the capacitor is connected to a DC source. The battery is now disconnected and then the dielectric is removed. State whether the capacitance, the energy stored in it, the electric field, charge stored and voltage will increase, decrease or remain constant.
Answer:
Assume a parallel-plate capacitor, of plate area A and plate separation d is filled with a dielectric of relative permittivity (dielectric constant) k. Its capacitance is C = \(\frac{k \varepsilon_{0} A}{d}\) …………. (1)
If it is charged to a voltage (potential) V, the charge on its plates is Q = CV.

Since the battery is disconnected after it is charged, the charge Q on its plates, and consequently the product CV, remain unchanged.

On removing the dielectric completely, its capacitance becomes from Eq. (1),
C’ = \(\frac{\varepsilon_{0} A}{d}=\frac{1}{k} C\) ……………. (2)
that is, its capacitance decreases by the factor k. Since C’V’ = CV, its new voltage is
V’ = \(\frac{C}{C^{\prime}}\) V = kV …………… (3)

so that its voltage increases by the factor k. The stored potential energy, U = \(\frac{1}{2}\) QV, so that Q remaining constant, U increases by the factor k. The electric field, E = V/ d, so that E also increases by a factor k.

Question 6.
Find the ratio of the potential differences that must be applied across the parallel and series combination of two capacitors C₁ and C₂ with their capacitances in the ratio 1 : 2, so that the energy stored in these two cases becomes the same.
Answer:

This gives the required ratio.

Question 7.
Two charges of magnitudes -4Q and +2Q are located at points (2a, 0) and (5a, 0) respectively. What is the electric flux due to these charges through a sphere of radius 4a with its centre at the origin?
Answer:
The sphere of radius 4a encloses only the negative charge Q₁ = -4Q. The positive charge Q₂ = +2Q being located at a distance of 5a from the origin is outside the sphere. Only a part of the electric flux lines originating at Q₂ enters the sphere and exits entirely at other points. Hence, the electric flux through the sphere is only due to Q₁.
Therefore, the net electric flux through the sphere = \(\frac{Q_{1}}{\varepsilon_{0}}=\frac{-4 Q}{\varepsilon_{0}}\) . The minus sign shows that the flux is directed into the sphere, but not radially since the sphere is not centred on Q₁.

Question 8.
A 6 µF capacitor is charged by a 300 V supply. It is then disconnected from the supply and is connected to another uncharged 3µF capacitor. How much electrostatic energy of the first capacitor is lost in the form of heat and electromagnetic radiation ?
Answer:
Data: C = 6 µF = 6 × 10^-6 F = C₁, V = 300 V
C₂ = 3 µF
The electrostatic energy in the capacitor
= \(\frac{1}{2}\)Cv² = \(\frac{1}{2}\)(6 × 10^-6)(300)²
= 3 × 10^-6 × 9 × 10⁴ = 0.27J
The charge on this capacitor,
Q = CV = (6 × 10^-6)(300) = 1.8 mC
When two capacitors of capacitances C₁ and C₂ are connected in parallel, the equivalent capacitance C
= C₁ + C₂ = 6 + 3 = 9 µF
= 9 × 10^-6F
By conservation of charge, Q = 1.8 C.
∴ The energy of the system = \(\frac{Q^{2}}{2 C}\)
= \(\frac{\left(1.8 \times 10^{-3}\right)^{2}}{2\left(9 \times 10^{-6}\right)}=\frac{18 \times 10^{-8}}{10^{-6}}\) = 0.18 J
The energy lost = 0.27 – 0.18 = 0.09 J

Question 9.
One hundred twenty five small liquid drops, each carrying a charge of 0.5 µC and each of diameter 0.1 m form a bigger drop. Calculate the potential at the surface of the bigger drop.
Answer:
Data : n = 125, q = 0.5 × 10^-6 C, d = 0.1 m
The radius of each small drop, r = d/2 = 0.05 m
The volume of the larger drop being equal to the volume of the n smaller drops, the radius of the larger drop is
R = \(\sqrt[3]{n} r\) = \(\sqrt[3]{125}\) (0.05) = 5 × 0.05 = 0.25 m
The charge on the larger drop,
Q = nq = 125 × (0.5 × 10^-6) C
∴ The electric potential of the surface of the larger drop,
V = \(\frac{1}{4 \pi \varepsilon_{0}} \frac{Q}{R}\) = (9 × 10⁹) × \(\frac{125 \times\left(0.5 \times 10^{-6}\right)}{0.25}\)
= 9 × 125 × 2 × 10³ = 2.25 × 10⁶ V

Question 10.
The dipole moment of a water molecule is 6.3 × 10^-30 Cm. A sample of water contains 1021 molecules, whose dipole moments are all oriented in an electric field of strength 2.5 × 10⁵ N /C. Calculate the work to be done to rotate the dipoles from their initial orientation θ₁ = 0 to one in which all the dipoles are perpendicular to the field, θ₂ = 90°.
Answer:
Data: p = 6.3 × 10^-30 C∙m, N = 10²¹ molecules,
E = 2.5 × 10⁵ N/C, θ₀ = θ₁ = 0°, θ = θ₂ = 90°
W = pE(cos θ₀ – cos θ)
The total work required to orient N dipoles is
W = NpE(cos θ₁ – cos θ₂)
=(10²¹)(6.3 × 10^-30)(2.5 × 10⁵)
= 15.75 × 10^-4 J = 1.575 mJ

Question 11.
A charge 6 µC is placed at the origin and another charge -5 µC is placed on the y axis at a position A (0, 6.0) m.

(a) Calculate the total electric potential at the point P whose coordinates are (8.0, 0) m
(b) Calculate the work done to bring a proton from infinity to the point P. What is the significance of the sign of the work done ?
Answer:
Data : q₁ = 6 × 10^-6 C, q₂ = -5 × 10^-6 C,
A ≡ (0, 6.0 m), P ≡ (8.0 m, 0), r₁ = OP = 8 m, q = e = 1.6 × 10^-19C, 1/4πε₀ = 9 × 10⁹ N∙m²/C²
r₂ = AP = \(\sqrt{(8-0)^{2}+(0-6)^{2}}\) = \(\sqrt{64+36}\) = 10 m

(a) The net electric potential at P due to the system of two charges is

(b) The electric potential V at the point P is the negative of the work done per unit charge, by the electric field of the system of the charges q₁ and q₂, in bringing a test charge from infinity to that point.
V = \(-\frac{W}{q_{0}}\)
∴ W = -qV= -(1.6 × 10^-19)(2.25 × 10³)
= -3.6 × 10^-16 J= -2.25 keV
That is, in bringing the positively charged proton from a point of lower potential to a point of higher potential, the work done by the electric field on it is negative, which means that an external agent must bring the proton against the electric field of the system of the two source charges.

[Note : The potential V at a point is the work done per unit charge (W_ext) by an external agent in bringing a test charge from infinity to that point. In the above case, the work done by an external agent will be positive. The question does not specify this.]

Question 12.
In a parallel plate capacitor with air between the plates, each plate has an area of 6 × 10^-3 m² and the separation between the plates is 2 mm.
i) Calculate the capacitance of the capacitor.
ii) If this capacitor is connected to 100 V supply, what would be the charge on each plate?
iii) How would charge on the plates be affected if a 2 mm thick mica sheet of k = 6 is inserted between the plates while the voltage supply remains connected ?
Answer:
Data: k = 1(air), A = 6 × 10^-3 m², d = 2
mm = 2 × 10^-3 m,V = 100V, t = 2 mm = d, k₁ = 6,
ε₀ = 8.85 × 10^-12 F/m
(i) The capacitance of the air capacitor, C₀ = \(\frac{\varepsilon_{0} A}{d}\)
= \(\frac{\left(8.85 \times 10^{-12}\right)\left(6 \times 10^{-3}\right)}{2 \times 10^{-3}}\)
= 26.55 × 10^-12 F = 26.55 pF

(ii) Q₀ = C₀V = (26.55 × 10^-12)(100)
= 26.55 × 10^-10 C = 2.655 nC

(iii) The dielectric of relative permittivity k₁ completely fills the space between the plates (∵t = d), so that the new capacitance is C = k₁C₀.
With the supply still connected, V remains the same.
∴ Q = CV = kC₀V = kQ₀ =6(2.655 nF) = 15.93 nC
Therefore, the charge on the plates increases.
[Note: Ck₁C₀ = 6(26.55 pF)= 159.3 pF.]

Question 13.
Find the equivalent capacitance between P and Q. Given, area of each plate = A and separation between plates = d.

Answer:
(i) The capacitor in figure is a series combination of three capacitors of plate separations d/3 and plate areas A, with C₁ filled with air (k₁ = 1), C₂ filled with dielectric of k₂ = 3 and C₃ filled with dielectric of k₃ = 6

(ii) In figure, a series combination of two capacitors C₂(k₂ = 3) and C₃(k₃ = 6), of plate areas A/2 and plate separations d/2, is in parallel with a capacitor C₁ (k₁ = 4) of plate area A/2 and plate separation d.

12th Physics Digest Chapter 8 Electrostatics Intext Questions and Answers

Can you recall (Textbook Page No. 188)

Question 1.
What is gravitational Potential ?
Answer:
We measure the gravitational potential energy U of a body (1) by assigning U = 0 for a reference configuration (such as the body at a reference level) (2) then equating U to the work W done by an external force to move the body up or down from that level to a point. We then define gravitational potential of the point as gravitational potential energy per unit mass of the body.

We follow the same procedure with the electric force, which is also a conservative force with the only difference that while the gravitational force is always attractive, electric force can be attractive (for unlike charges) or repulsive (for like charges).

Remember this (Textbook Page No. 191)

Question 1.
Due to a single charge at a distance r, Force (F ) α 1/r², Electric field (E ) α 1/r² but Potential (V) α 1/ r.
Answer:
At a point a distance r from an isolated point charge, the force F on a point charge and the electric field E both vary as 1/r², while the potential energy U of a point charge and the electric potential V at the point both vary as 1/r.

Use your brain power (Textbook Page No.194)

Question 1.
Is electrostatic potential necessarily zero at a point where electric field strength is zero? Justify.
Answer:
Electric potential is a scalar quantity while electric field intensity is a vector quantity. When we add potentials at a point due two or more point charges, the operation is simple scalar addition along with the sign of V, determined by the sign of the q that produces V. At a point, the net field is the vector sum of the fields due to the individual charges. Midway between the two charges of an electric dipole, the potentials due to the two charges are equal in magnitude but opposite in sign, and thus add up to zero. But the electric fields due to the charges are equal in magnitude and direction-towards the negative charge-so that the net field there is not zero. But midway between two like charges of equal magnitudes, the potentials are equal in magnitude and have the same sign, so that the net potential is nonzero. However, the fields due to the two equal like charges are equal in magnitude but opposite in direction, and thus vectorially add up to zero.

Do you know (Textbook Page No. 203)

Question 1.
If we apply a large enough electric field, we can ionize the atoms and create a condition for electric charge to flow like a conductor. The fields required for the breakdown of dielectric is called dielectric strength.
Answer:
In a sufficiently strong electric field, the molecules of a dielectric material become ionized, allowing flow of charge. The insulating properties of the dielectric breaks down, permanently or temporarily, and the phenomenon is called dielectric breakdown. During dielectric breakdown, electrical discharge through the dielectric follows random-path patterns like tree branches, called a Lichtenberg figure. Dielectric strength is the voltage that an insulating material can withstand before breakdown occurs. It usually depends on the thickness of the material. It is expressed in kV/mm. For example, the dielectric strengths of air, polystyrene and mica in kV/mm are 3, 20 and 118. Higher dielectric strength corresponds to better insulation properties.

Remember this (Textbook Page No. 205)

Question 1.
Series combination is used when a high voltage is to be divided on several capacitors. Capacitor with minimum capacitance has the maximum potential difference between the plates.
Answer:
Series combination of capacitors

1. Equivalent capacitance is less than the smallest capacitance in series. For several capacitors of given capacitances, the equivalent capacitance of their series combination is minimum.
2. All capacitors in the combination have the same charge but their potential differences are in the inverse ratio of their capacitances.
3. Series combination of capacitors is sometimes used when a high voltage, which exceeds the breakdown voltage of a single capacitor, is to be divided on more than one capacitors. Capacitive voltage dividers are only useful in AC circuits, since capacitors do not pass DC signals.

Parallel combination of capacitors

1. For several capacitors of given capacitances, the equivalent capacitance of their parallel combination is maximum.
2. The same voltage is applied to all capacitors in the combination, but the charge stored in the combination is distributed in proportion to their capacitances. The maximum rated voltage of a parallel combination is only as high as the lowest voltage rating of all the capacitors used. That is, if several capacitors rated at 500 V are connected in parallel to a capacitor rated at 100 V, the maximum voltage rating of the capacitor bank is only 100 V.
3. Parallel combination of capacitors is used when a large capacitance is required, i.e., a large charge is to be stored, at a small potential difference.

Remember this (Textbook Page No. 207)

Question 1.
(1) If there are n parallel plates then there will be (n-1) capacitors, hence
C = (n – 1) \(\frac{A \varepsilon_{0}}{d}\)
(2) For a spherical capacitor, consisting of two concentric spherical conducting shells with inner and outer radii as a and b respectively, the capacitance C is given by
C = 4πε₀\(\left(\frac{a b}{b-a}\right)\)
(3) For a cylindrical capacitor, consisting of two coaxial cylindrical shells with radii of the inner and outer cylinders as a and b, and length l, the capacitance C is given by
C = \(\frac{2 \pi \varepsilon_{0} \ell}{\log _{e} \frac{b}{a}}\)
Answer:
1. Stacking together n identical conducting plates equally spaced and with alternate plates connected to two points P and Q, forms a parallel combination of n -1 identical capacitors between P and Q. Then, the capacitance between the points is (n – 1) times the capacitance between any two adjacent plates.

2. A cylindrical capacitor consists of a solid cylindrical conductor of radius a is surrounded by coaxial cylindrical shell of inner radius b. The length of both cylinders is L, such that L is much larger than b – a, the separation of the cylinders, so that edge effects can be ignored. The capacitance of the capacitor is C = \(\frac{2 \pi \varepsilon_{0} L}{\log _{e}(b / a)}\).

The capacitance depends only on the geometrical factors, L, a and b, as for a parallel-plate capacitor.

3. A spherical capacitor which consists of two concentric spherical shells of radii a and b. The capacitance of the capacitor is C = 4πε₀\(\left(\frac{a b}{b-a}\right)\)

Again, the capacitance depends only on the geometrical factors, a and b.

Balbharti Maharashtra State Board 12th Physics Textbook Solutions Chapter 9 Current Electricity Textbook Exercise Questions and Answers.

Maharashtra State Board 12th Physics Solutions Chapter 9 Current Electricity

1. Choose the correct option.

i) Kirchhoff’s first law, i.e., ΣI = 0 at a junction, deals with the conservation of
(A) charge
(B) energy
(C) momentum
(D) mass
Answer:
(A) charge

ii) When the balance point is obtained in the potentiometer, a current is drawn from
(A) both the cells and auxiliary battery
(B) cell only
(C) auxiliary battery only
(D) neither cell nor auxiliary battery
Answer:
(D) neither cell nor auxiliary battery

iii) In the following circuit diagram, an infinite series of resistances is shown. Equivalent resistance between points A and B is

(A) infinite
(B) zero
(C) 2 Ω
(D) 1.5 Ω
Answer:
(C) 2 Ω

iv) Four resistances 10 Ω, 10 Ω, 10 Ω and 15 Ω form a Wheatstone’s network. What shunt is required across 15 Ω resistor to balance the bridge
(A) 10 Ω
(B) 15 Ω
(C) 20 Ω
(D) 30 Ω
Answer:
(D) 30 Ω

v) A circular loop has a resistance of 40 Ω. Two points P and Q of the loop, which are one quarter of the circumference apart are connected to a 24 V battery, having an internal resistance of 0.5 Ω. What is the current flowing through the battery.
(A) 0.5 A
(B) 1A
(C) 2A
(D) 3A
Answer:
(D) 3A

vi) To find the resistance of a gold bangle, two diametrically opposite points of the bangle are connected to the two terminals of the left gap of a metre bridge. A resistance of 4 Ω is introduced in the right gap. What is the resistance of the bangle if the null point is at 20 cm from the left end?
(A) 2 Ω
(B) 4 Ω
(C) 8 Ω
(D) 16 Ω
Answer:
(A) 2 Ω

2. Answer in brief.

i) Define or describe a Potentiometer.
Answer:
The potentiometer is a device used for accurate measurement of potential difference as well as the emf of a cell. It does not draw any current from the circuit at the null point. Thus, it acts as an ideal voltmeter and it can be used to determine the internal resistance of a cell. It consist of a long uniform wire AB of length L, stretched on a wooden board. A cell of stable emf (E), with a plug key K in series, is connected across AB as shown in the following figure.

ii) Define Potential Gradient.
Answer:
Potential gradient is defined as the potential difference (the fall of potential from the high potential end) per unit length of the wire.

iii) Why should not the jockey be slided along the potentiometer wire?
Answer:
Sliding the jockey on the potentiometer wire decreases the cross sectional area of the wire and thereby affects the fall of potential along the wire. This affects the potentiometer readings. Flence, the jockey should not be slided along the potentiometer wire.

iv) Are Kirchhoff’s laws applicable for both AC and DC currents?
Answer:
Kirchhoff’s laws are applicable to both AC and DC ’ circuits (networks). For AC circuits with different loads, (e.g. a combination of a resistor and a capacitor, the instantaneous values for current and voltage are considered for addition.

[Note : Gustav Robert Kirchhoff (1824-87), German physicist, devised laws of electrical network and, with Robert Wilhelm Bunsen, (1811-99), German chemist, did pioneering work in chemical spectroscopy. He also con-tributed to radiation.]

v) In a Wheatstone’s meter-bridge experiment, the null point is obtained in middle one third portion of wire. Why is it recommended?
Answer:

1. The value of unknown resistance X, may not be accurate due to non-uniformity of the bridge wire and development of contact resistance at the ends of the wire.
2. To minimise these errors, the value of R is so adjusted that the null point is obtained in the middle one-third of the wire (between 34 cm and 66 cm) so that the percentage errors in the measurement of I_X and I_R are minimum and nearly the same.

vi) State any two sources of errors in meterbridge experiment. Explain how they can be minimized.
Answer:
The chief sources of error in the metre bridge experiment are as follows :

1. The bridge wire may not be uniform in cross section. Then the wire will not have a uniform resistance per unit length and hence its resistance will not be proportional to its length.
2. End resistances at the two ends of the wire may be introduced due to
   1. the resistance of the metal strips
   2. the contact resistance of the bridge wire with the metal strips
   3. unmeasured lengths of the wire at the ends because the contact points of the wire with the metal strips do not coincide with the two ends of the metre scale attached.

Such errors are almost unavoidable but can be minimized considerably as follows :

1. Readings must be taken by adjusting the standard known resistance such that the null point is obtained close to the centre of the wire. When several readings are to be taken, the null points should lie in the middle one-third of the wire.
2. The measurements must be repeated with the standard resistance (resistance box) and the unknown resistance interchanged in the gaps of the bridge, obtaining the averages of the two results.

vii) What is potential gradient? How is it measured? Explain.
Answer:
Consider a potentiometer consisting of a long uniform wire AB of length L and resistance R, stretched on a wooden board and connected in series with a cell of stable emf E and internal resistance r and a plug key K as shown in below figure.

Let I be the current flowing through the wire when the circuit is closed.
Current through AB, I = \(\frac{E}{R+r}\)
Potential difference across AB. V_AB = IR
∴ V_AB = \(\frac{E R}{(R+r)}\)
The potential difference (the fall of potential from the high potential end) per unit length of the wire,
\(\frac{V_{\mathrm{AB}}}{L}=\frac{E R}{(R+r) L}\)
As long as E and r remain constant, \(\frac{V_{\mathrm{AB}}}{L}\) will remain constant, \(\frac{V_{\mathrm{AB}}}{L}\) is known as potential gradient along

AB and is denoted by K. Thus the potential gradient is calculated by measuring the potential difference between ends of the potentiometer wire and dividing it by the length of the wire.
Let P be any point on the wire between A and B and AP = l = length of the wire between A and P.
Then V_AP = Kl
∴ V_AP ∝ l as K is constant in a particular case. Thus, the potential difference across any length of the potentiometer wire is directly proportional to that length. This is the principle of the potentiometer.

viii) On what factors does the potential gradient of the wire depend?
Answer:
The potential gradient depends upon the potential difference between the ends of the wire and the length of the wire.

ix) Why is potentiometer preferred over a voltmeter for measuring emf?
Answer:
A voltmeter should ideally have an infinite resistance so that it does not draw any current from the circuit. However a voltmeter cannot be designed to have infinite resistance. A potentiometer does not draw any current from the circuit at the null point. Therefore, it gives more accurate measurement. Thus, it acts as an ideal voltmeter.

x) State the uses of a potentiometer.
Answer:
The applications (uses) of the potentiometer :

1. Voltage divider :
   The potentiometer can be used as a voltage divider to change the output voltage of a voltage supply.
2. Audio control:
   Sliding potentiometers are commonly used in modern low-power audio systems as audio control devices. Both sliding (faders) and rotary potentiometers (knobs) are regularly used for frequency attenuation, loudness control and for controlling different characteristics of audio signals.
3. Potentiometer as a sensor:
   If the slider of the potentiometer is connected to the moving part of a machine, it can work as a motion sensor. A small displacement of the moving part causes a change in potential which is further amplified using an amplifier circuit. The potential difference is calibrated in terms of displacement of the moving part.
4. To measure the emf (for this, the emf of the standard cell and potential gradient must be known).
5. To compare the emf’s of two cells.
6. To determine the internal resistance of a cell.

xi) What are the disadvantages of a potentiometer?
Answer:
Disadvantages of a potentiometer over a voltmeter :

1. The use of a potentiometer is an indirect measurement method while a voltmeter is a direct reading instrument.
2. A potentiometer is unwieldy while a voltmeter is portable.
3. Unlike a voltmeter, the use of a potentiometer in measuring an unknown emf requires a standard . source of emf and calibration.

xii) Distinguish between a potentiometer and a voltmeter.
Answer:

xiii) What will be the effect on the position of zero deflection if only the current flowing through the potentiometer wire is
(i) increased (ii) decreased.
Answer:
(1) On increasing the current through the potentiometer wire, the potential gradient along the wire will increase. Hence, the position of zero deflection will occur at a shorter length.

(2) On decreasing the current through the potentiometer wire, the potential gradient along the wire will decrease. Hence, the position of zero deflection will occur at a longer length.
[Note : In the usual notation,
E₁ = (\(\frac{I R}{L}\)) l₁ = constant
Hence, (i) E, decreases when I is increased (ii) l₁ increases when I is decreased.]

Question 3.
Obtain the balancing condition in case of a Wheatstone’s network.
Answer:
Wheatstone’s network or bridge is a circuit for indirect measurement of resistance by null com-parison method by comparing it with a standard known resistance. It consists of four resistors with resistances P, Q, R and S arranged in the form of a quadrilateral ABCD. A cell (E) with a plug key (K) in series is connected across one diagonal AC and a galvanometer (G) across the other diagonal BD as shown in the following figure.

With the key K dosed, currents pass through the resistors and the galvanometer. One or more of the resistances is adjusted until no deflection in the galvanometer can be detected. The bridge is then said to be balanced.

Let I be the current drawn from the cell. At junction A, it divides into a current I₁ through P and a current I₂ through S.
I = I₁ + I₂ (by Kirchhoff’s first law).
At junction B, current I_g flows through the galvanometer and current I₁ – I_g flows through Q. At junction D, I₂ and I_g combine. Hence, current I₂ + I_g flows through R from D to C. At junction C, I₁ – I_g and I₂ + I_g combine. Hence, current I₁ + I₂(= I) leaves junction C.

Applying Kirchhoff’s voltage law to loop ABDA in a clockwise sense, we get,
– I₁P – I_gG + I₂S = 0 …………… (1)
where G is the resistance of the galvanometer.
Applying Kirchhoff’s voltage law to loop BCDB in a clockwise sense, we get,
– (I₁ – I_g)Q + (I₂ + I_g)R + I_gG = 0 ………….. (2)
When I_g = 0, the bridge (network) is said to be balanced. In that case, from Eqs. (1) and (2), we get,
I₁P = I₂S …………… (3)
and I₁Q = I₂R ………….. (4)
From Eqs. (3) and (4), we get,
\(\frac{P}{Q}=\frac{S}{R}\)
This is the condition of balance.
Alternative Method: When no current flows through the galvanometer, points B and D must be at the same potential.
∴ V_B = V_D
∴ V_A – V_B = V_A – V_D …………. (1)
and V_B – V_C = V_D – V_C ………… (2)
Now, V_A – V_B = I₁P and V_A – V_D = I₂S ………….. (3)
Also, V_B – V_C = I₁Q and V_D – V_C = I₂R …………. (4)
Substituting Eqs. (3) and (4) in Eqs. (1) and (2), we get,
I₁P = I₂S . ………… (5)
and I₁Q = I₂R …(6)
Dividing Eq. (5) by Eq. (6), we get,
\(\frac{P}{Q}=\frac{S}{R}\)
This is the condition of balance.

[ Note : In the determination of an unknown resistance using Wheatstone’s network, the unknown resistance is connected in one arm of the network (say, AB), and a standard known variable resistance is connected in an adjacent arm. Then, the other two arms are called the ratio arms. Also, because the positions of the cell and galvanometer can be interchanged, without a change in the condition of balance, the branches AC and BD in figure are called the conjugate arms. ]

Question 4.
Explain with neat circuit diagram, how you will determine the unknown resistance by using a meter-bridge.
Answer:
A metre bridge consists of a rectangular wooden board with two L-shaped thick metallic strips fixed along its three edges. A single thick metallic strip separates two L-shaped strips. A wire of length one metre and uniform cross section is stretched on a metre scale fixed on the wooden board. The ends of the wire are fixed to the L-shaped metallic strips.

An unknown resistance X is connected in the left gap and a resistance box R is connected in the right gap as shown in above figure. One end of a centre-zero galvanometer (G) is connected to terminal C and the other end is connected to a pencil jockey (J). A cell (E) of emf E, plug key (K) and rheostat (Rh) are connected in series between points A and B.

Working : Keeping a suitable resistance (R) in the resistance box, key K is closed to pass a current through the circuit. The jockey is tapped along the wire to locate the equipotential point D when the galvanometer shows zero deflection. The bridge is then balanced and point D is called the null point and the method is called as null deflection method. The distances l_X and l_R of the null point from the two ends of the wire are measured.

As R, l_X and l_R are known, the unknown resistance X can be calculated.

Question 5.
Describe Kelvin’s method to determine the resistance of a galvanometer by using a meter bridge.
Answer:
Kelvin’s method :
Circuit: The metre bridge circuit for Kelvin’s method of determination of the resistance of a galvanometer is shown in below figure. The galvanometer whose resistance G is to be determined, is connected in one gap of the metre bridge. A resistance box providing a variable known resistance R is connected in the other gap. The junction B of the galvanometer and the resistance box is con-nected directly to a pencil jockey. A cell of emf E, a key (K) and a rheostat (Rh) are connected across AC.

Working : Keeping a suitable resistance R in the resistance box and maximum resistance in the rheostat, key K is closed to pass the current. The rheostat resistance is slowly reduced such that the galvanometer shows about 2 / 3rd of the full-scale deflection.

On tapping the jockey at end-points A and C, the galvanometer deflection should change to opposite sides of the initial deflection. Only then will there be a point D on the wire which is equipotential with point B. The jockey is tapped along the wire to locate the equipotential point D when the galvanometer shows no change in deflection. Point D is now called the balance point and Kelvin’s method is thus an equal deflection method. At this balanced condition,
\(\frac{G}{R}=\frac{\text { resistance of the wire of length } l_{G}}{\text { resistance of the wire of length } l_{R}}\)
where I_G = the length of the wire opposite to the galvanometer, I_R = the length of the wire opposite to the resistance box.
If λ = the resistance per unit length of the wire,
\(\frac{G}{R}=\frac{\lambda l_{G}}{\lambda l_{R}}=\frac{l_{G}}{l_{R}}\)
∴ G = R\(\frac{l_{G}}{l_{R}}\)
The quantities on the right hand side are known, so that G can be calculated.
[Note : The method was devised by William Thom-son (Lord Kelvin, 1824-1907), British physicist.]

Question 6.
Describe how a potentiometer is used to compare the emfs of two cells by connecting the cells individually.
Answer:
A battery of stable emf E is used to set up a potential gradient V/L along a potentiometer wire, where V = potential difference across the length L of the wire. The positive terminals of the cells, whose emf’s (E₁ and E₂) are to be compared, are connected to the high potential terminal A. The negative terminals of the cells are connected to a galvanometer G through a two-way key. The other terminal of the galvanometer is connected to a pencil jockey. The emf E should be greater than both the emf’s E₁ and E₂.

Connecting point P to C, the cell with emf E1 is brought into the circuit. The jockey is tapped along the wire to locate the null point D at a distance l₁ from A. Then,
E₁ = Z₁(V/L)
Now, without changing the potential gradient (i.e., without changing the rheostat setting) point Q (instead of P) is connected to C, bringing the cell with emf E₂ into the circuit. Let its null point D’ be at a distance l₂ from A, so that
E₂ = l₂(V/L)
∴ \(\frac{E_{1}}{E_{2}}=\frac{l_{1}}{l_{2}}\)
Hence, by measuring the corresponding null lengths l₁ and l₂, E₁/E₂ can be calculated. The experiment is repeated for different potential gradients using the rheostat.
[Note : This method is used when the two emf’s have comparable magnitudes. Then, the errors of measurement of their balancing lengths will also be of comparable magnitudes.]

Question 7.
Describe how a potentiometer is used to compare the emfs of two cells by combination method.
Answer:
A battery of stable emf E is used to set up a potential gradient V/L, along the potentiometer wire, where V = potential difference across length L of the wire. The positive terminal of the cell 1 is connected to the higher potential terminal A of the potentiometer; the negative terminal is connected to the galvanometer G through the reversing key. The other terminal of the galvanometer is connected to a pencil jockey. The cell 2 is connected across the remaining two opposite terminals of the reversing key. The other terminal of the galvanometer is connected to a pencil jockey. The emf E₁ should be greater than the emf E₂; this can be adjusted by trial and error.

Two plugs are inserted in the reversing key in positions 1 – 1. Here, the two cells assist each other so that the net emf is E₁ + E₂. The jockey is tapped along the wire to locate the null point D. If the null point is a distance l₁ from A,
E₁ + E₂ = l₁ (V/L)

For the same potential gradient (without changing the rheostat setting), the plugs are now inserted into position 2 – 2. (instead of 1 – 1). The emf E₂ then opposes E₁ and the net emf is E₁ – E₂. The new null point D’ is, say, a distance l₂ from A and
E₁ – E₂ = l₂ (V/L)
∴ \(\frac{E_{1}+E_{2}}{E_{1}-E_{2}}=\frac{l_{1}}{l_{2}}\) ∴ \(\frac{E_{1}}{E_{2}}=\frac{l_{1}-l_{2}}{l_{1}-l_{2}}\)
Here, the emf E should be greater than E₁ + E₂. The experiment is repeated for different potential gradients using the rheostat.
[Note : This method is used when E₁ » E₂, so that E₁ + E₂ and E₁ – E₂ have comparable magnitudes. Then, the errors of measurement of l₁ and l₂ will also be of comparable magnitudes.]

Question 8.
Describe with the help of a neat circuit diagram how you will determine the internal resistance of a cell by using a potentiometer. Derive the necessary formula.
Answer:
Principle : A cell of emf £ and internal resistance r, which is connected to an external resistance R, has its terminal potential difference V less than its emf E. If I is the corresponding current,
\(\frac{E}{V}=\frac{I(R+r)}{I R}=\frac{R+r}{R}\) = 1 + \(\frac{r}{R}\) (when R → ∞, V → E)
∴ r = \(\frac{E-V}{V}\) R
Working : A battery of stable emf E’ is used to set up a potential gradient V/L, along the potentiometer wire, where V = potential difference across the length L of the wire. The negative terminal is connected through a centre-zero galvanometer G to a pencil jockey. A resistance box R with a plug key K in series is connected across the cell.

Firstly, key K is kept open; then, effectively, R = ∞. The jockey is tapped on the potentiometer wire to locate the null point D. Let the null length
AD = l₁, so that
E = (V_AB/L)l₁

With the same potential gradient, and a small resistance R in the resistance box, key K is closed. The new null length AD’ = l2 for the terminal potential difference V is found :

R, l₁, and l₂ being known, r can be calculated. The experiment is repeated with different potential gradients using the rheostat or with different values of R.

Question 9.
On what factors does the internal resistance of a cell depend?
Answer:
The internal resistance of a cell depends on :

1. Nature of the electrolyte :
   The greater the conductivity of the electrolyte, the lower is the internal resistance of the cell.
2. Separation between the electrodes :
   The larger the seperation between the electrodes of the cell, the higher is the internal resistance of the cell. This is because the ions have to cover a greater distance before reaching an electrode.
3. Nature of the electrodes.
4. The internal resistance is inversly proportional to the common area of the electrodes dipping in the electrolyte.

Question 10.
A battery of emf 4 volt and internal resistance 1 Ω is connected in parallel with
another battery of emf 1 V and internal resistance 1 Ω (with their like poles connected together). The combination is used to send current through an external resistance of 2 Ω. Calculate the current through the external resistance.
Answer:
Let I₁ and I₂ be the currents through the two branches as shown in below figure. The current through the 2 Q resistance will be (I₁ + I₂) [Kirchhoff’s current law].
Applying Kirchhoff’s voltage law to loop ABCDEFA, we get,
-2(I₁ + I₂) – 1(I₁) + 4 = 0
∴ 3I₁ + 2I₁ = 4 …………… (1)

Applying Kirchhoff’s voltage law to loop BCDEB, we get,
-2(I₁ + I₂) – 1(I₂) + 1 = 0
2I₁ + 3I₂ = 1 ………… (2)
Multiplying Eq. (1) by 2 and Eq. (2) by 3, we get,
6I₁ + 4I₂ = 8 ………….(3)
and 6I₁ + 9I₂ = 3 ……………. (4)
Subtracting Eq. (4) from Eq. (3), we get,
– 5I₂ =5
∴ I₂ = -1A
The minus sign shows that the direction of current I2 is opposite to that assumed. Substituting this value of 12 in Eq. (1), we get,
3I₁ + 2(-1) = 4
∴3I₁ = 4 + 2 = 6
∴ I₁ = 2A
Current through the 2 0 resistance = I₁ + I₂ = 2 – 1
= 1 A. It is in the direction as shown in the figure.
[Note : We may as well consider loop ABEFA and write the corresponding equation. But it does not provide any additional information.]

Question 11.
Two cells of emf 1.5 Volt and 2 Volt having respective internal resistances of 1 Ω and 2 Ω are connected in parallel so as to send current in same direction through an external resistance of 5 Ω. Find the current through the external resistance.
Answer:
Let I₁ and I₂ be the currents through the two branches as shown in below figure. The current through the 5 Q resistor will be I₁ + I₂ [Kirchhoff’s current law].

Applying Kirchhoff’s voltage law to loop w ABCDEFA, we get,
– 5(I₁ + I₂) – I₁ + 1.5 = 0
∴ 6I₁ + 5I₂ = 1.5 ……………. (1)
Applying Kirchhoff’s voltage law to loop BCDEB, we get,
– 5(I₁ + I₂) – 2I₂ + 2 = 0
∴ 5I₁ + 7I₂ = 2 ……………(2)
Multiplying Eq. (1) by 5 and Eq. (2) by 6, we get,
30I₁ + 25I₂ = 7.5 …………… (3)
and 30I₁ + 42I₂ = 12 …………. (4)
Subtracting Eq. (3) from Eq. (4), we get,
17I₂ = 4.5
∴ I₂ = \(\frac{4.5}{17}\) A
Substituting this value of I2 in Eq. (1), we get,
6I₁ + 5(\(\frac{4.5}{17}\)) = 1.5
∴ 6I₁ + \(\frac{22.5}{17}\) = 1.5
∴ 6I₁ = 1.5 – \(\frac{22.5}{17}\) = \(\frac{28.5-22.5}{17}\)
= \(\frac{3}{17}\)
∴ I₁ = \(\frac{3}{17 \times 6}=\frac{0.5}{17}\) A
Current through the 5 Q resistance (external resistance)
= I₁ + I₂ = \(\frac{0.5}{17}+\frac{4.5}{17}=\frac{5}{17}\) A

Question 12.
A voltmeter has a resistance 30 Ω. What will be its reading, when it is connected across a cell of emf 2 V having internal resistance 10 Ω?
Answer:
Data: E = 2V, r = 10 Ω, R = 30 Ω
The voltmeter reading, V = IR
= (\(\frac{E}{R+r}\)) R
= (\(\frac{2}{30+10}\)) 30
= (\(\frac{2}{40}\)) 30
= 1.5 V

Question 13.
A set of three coils having resistances 10 Ω, 12 Ω and 15 Ω are connected in parallel. This combination is connected in series with series combination of three coils of the same resistances. Calculate the total resistance and current through the circuit, if a battery of emf 4.1 Volt is used for drawing current.
Answer:
Below figure shows the electrical network. For resistances 10 Ω, 12 Ω and 15 Ω connected in parallel the equivalent resistance (R_p) is given by,

For resistance R_p, 10 Ω, 12 Ω and 15 Ω connected in series, the equivalent resistance,
R_s = 4 + 10 + 12 + 15 = 41 Ω
Thus, the total resistance = R_s = 41 Ω
Now, V = IR_s
∴ 4.1 = 1 × 41
∴ I = 0.1A
The total resistance and current through the circuit are 41 Ω and 0.1 A respectively.

Question 14.
A potentiometer wire has a length of 1.5 m and resistance of 10 Ω. It is connected in series with the cell of emf 4 Volt and internal resistance 5 Ω. Calculate the potential drop per centimeter of the wire.
Answer:
Data : L = 1.5 m, R = 10 Ω, E = 4 V, r = 5 Ω

The potential drop per centimeter of the wire is 0.0178 \(\frac{\mathrm{V}}{\mathrm{cm}}\)

Question 15.
When two cells of emfs. E₁ and E₂ are connected in series so as to assist each other, their balancing length on a potentiometer is found to be 2.7 m. When the cells are connected in series so as to oppose each other, the balancing length is found to be 0.3 m. Compare the emfs of the two cells.
Answer:
Data : l₁ = 2.7m (cells assisting),
l₂ = 0.3 m (cells opposing)
E₁ + E₂ = Kl₁ and E₁ – E₂ = Kl₂
∴\(\frac{E_{1}+E_{2}}{E_{1}-E_{2}}=\frac{K l_{1}}{K l_{2}}\)
∴ \(\frac{E_{1}}{E_{2}}=\frac{l_{1}+l_{2}}{l_{1}-l_{2}}=\frac{2.7+0.3}{2.7-0.3}=\frac{3}{2.4}=\frac{30}{24}\) = 1.25
The ratio of the emf’s of the two cells is 1.25.

Question 16.
The emf of a cell is balanced by a length of 120 cm of potentiometer wire. When the cell is shunted by a resistance of 10 Ω, the balancing length is reduced by 20 cm. Find the internal resistance of the cell.
Answer:
Data :R = 10 Ω, l₁ = 120 cm, l₂ = 120 – 20 = 100 cm
r = R(\(\frac{l_{1}-l_{2}}{l_{2}}\))
= 10 (\(\frac{120-100}{100}\))
= 2 Ω
The internal resistance of the cell is 2 Ω.

Question 17.
A potential drop per unit length along a wire is 5 × 10^-3 V/m. If the emf of a cell balances against length 216 cm of this potentiometer wire, find the emf of the cell.
Answer:
Data: K = 5 × 10^-3 \(\frac{\mathrm{V}}{\mathrm{m}}\), L = 216 cm = 216 × 10^-2 m
E = KL
∴ E = 5 × 10^-3 × 216 × 10^-2
= 1080 × 10^-5
= 0.01080V
The emf of the cell is 0.01080 volt.
(Note: For K = 0.5 V/m, we get, E = 1.08V (reasonable value)]

Question 18.
The resistance of a potentiometer wire is 8 Ω and its length is 8 m. A resistance box and a 2 V battery are connected in series with it. What should be the resistance in the box, if it is desired to have a potential drop of 1 µV/mm?
Answer:
Data: R = 8 Ω, L = 8 m, E = 2 V, K = 1 µV/mm
= 1 × \(\frac{10^{-6} \mathrm{~V}}{10^{-3} \mathrm{~m}}\) = 10^-3 \(\frac{\mathrm{V}}{\mathrm{m}}\)
K = \(\frac{V}{L}=\frac{E R}{\left(R+R_{\mathrm{B}}\right) L^{\prime}}\) where R_B is the resistance in the box.
∴ 10^-3 = \(\frac{2 \times 8}{\left(8+R_{B}\right) 8}\)
∴ 8 + R_B = \(\frac{2}{10^{-3}}\)
= 2 × 10³
∴ R_B = 2000 – 8
= 1992 Ω

Question 19.
Find the equivalent resistance between the terminals F and B in the network shown in the figure below given that the resistance of each resistor is 10 ohm.

Answer:
Applying Kirchhoff’s voltage law to loop FGHF, we get,
– 10I₁ – 10(I₁ – I₂) + 10(I – I₁) + 10(I – I₁) = 0
∴ – 10I₁ – 10I₁ + 10I₂ + 10I – 10I₁ + 10I – 10I₁ = 0
∴ 201 – 40I₁ + 10I₂ = 0
∴ 2I – 4I₁ + I₂ = 0 …………….. (1)
Applying Kirchhoff’s voltage law to loop GABHG, we get,
– 10I₂ – 10I₂ + 10(I – I₂) + 10(I₁ – I₂) = 0
∴ – 20I₂ + 10I – 10I₂ + 10I₁ – 10I₂ = 0
∴ 10I + 10I₁ – 40I₂ = 0 .
∴ I + I₁ – 4I₂ = 0 ……………… (2)
Applying Kirchhoff’s voltage law to loop EFHBCDE, we get,
– 10(I – I₁) – 10(I – I₁) – 10(I – I₂) + E = 0
∴ -10I + 10I₁ – 10I + 10I₁ – 10I + 10I₂ + E = 0
∴E = 30I – 20I₁ – 10I₂ ………….. (3)
From Eq. (1), we get, I₂ = 4I₁ – 2I …………. (4)
From Eqs. (2) and (4), we get,
I + I₁ – 4(4I₁ – 2I) = 0
∴ I + I₁ – 16I₁ + 8I = 0 .
∴ 9I = 15I₁ ∴ I₁ = \(\frac{9}{15}\)I = \(\frac{3}{5}\)I …………. (5)
From Eqs. (4) and (5), we get,
I₂ = 4(\(\frac{3}{5}\)I) – 2I = \(\frac{12}{5}\)I – 2I
= \(\frac{12 I-10 I}{5}\) = \(\frac{2}{5}\) I
From Eqs. (3), (5) and (6), we get
E = 30I – 20(\(\frac{3}{5}\) I) – 10(\(\frac{2}{5}\) I)
= 30I – 12I – 4I = 30I – 16I
∴ E = 14I
If R is the equivalent resistance between E and C,
E = RI
∴ R = 14 Ω

Question 20.
A voltmeter has a resistance of 100 Ω. What will be its reading when it is connected across a cell of emf 2 V and internal resistance 20 Ω?
Answer:
Data: E = 2V, r = 20 Ω, R = 100 Ω
The voltmeter reading, V = IR
V = (\(\frac{2}{100+20}\))100 = \(\frac{200}{120}=\frac{10}{6}\) = 1.667 V.

12th Physics Digest Chapter 9 Current Electricity Intext Questions and Answers

Observe and Discuss (Textbook Page No. 220)

Question 1.
Post Office Box
A post office box (PO Box) is a practical form of Wheatstone bridge as shown in the figure.

It consists of three arms P, Q and R. The resistances in these three arms are adjustable. The two ratio arms P and Q contain resistances of 10 ohm, 100 ohm and 1000 ohm each. The third arm R contains resistances from 1 ohm to 5000 ohm. The unknown resistance X (usually, in the form of a wire) forms the fourth arm of the Wheatstone’s bridge. There are two tap keys K₁ and K₂ .
Answer:
The resistances in the arms P and Q are fixed to a desired ratio. The resistance in the arm R is adjusted so that the galvanometer shows no deflection. Now the bridge is balanced. The unknown resistance X = RQ/P, where P and Q are the fixed resistances in the ratio arms and R is the adjustable known resistance.

If L is the length of the wire used to prepare the resistor with resistance X and r is its radius, then the specific resistance (resistivity) of the material of the wire is given by
ρ = \(\frac{X \pi r^{2}}{L}\)

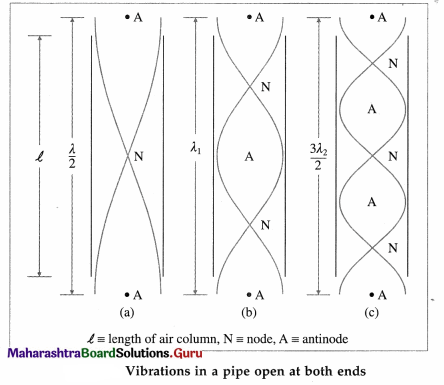
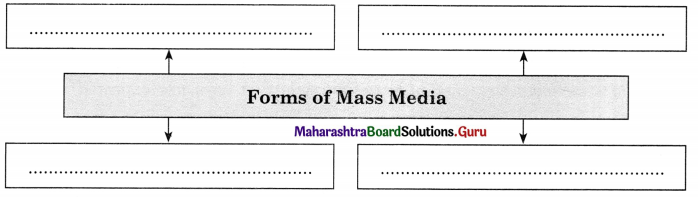
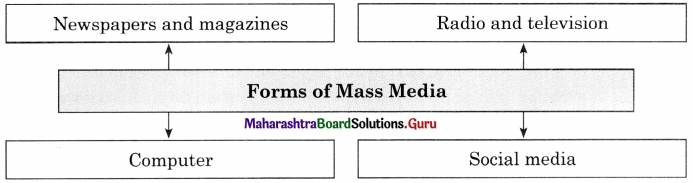
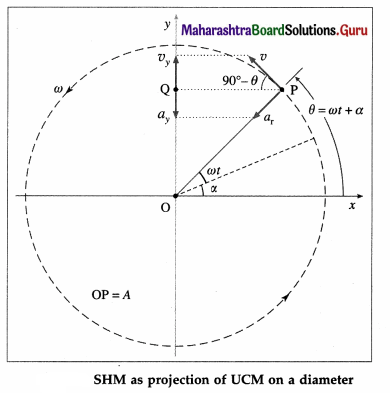
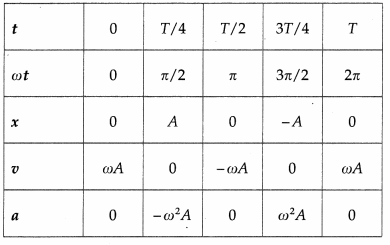
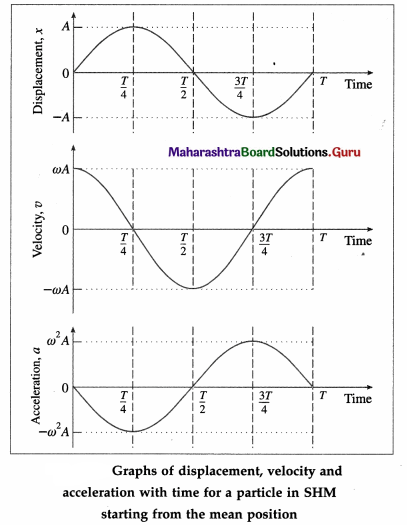
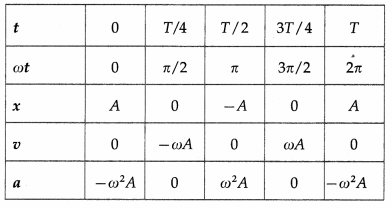
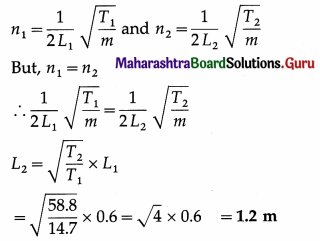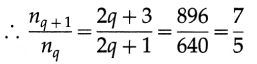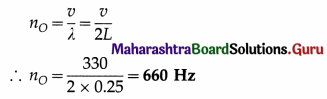Balbharti Maharashtra State Board Organisation of Commerce and Management 12th Textbook Solutions Chapter 2 Functions of Management Textbook Exercise Questions and Answers.

Maharashtra State Board 12th Organisation of Commerce and Management Solutions Chapter 2 Functions of Management

1. (A) Select the correct options and rewrite the sentences

Question 1.
The functions of management start with ……………… function.
(a) organising
(b) planning
(c) co-ordinating
Answer:
planning

Question 2.
The functions of management end with ………………
(a) directing
(b) staffing
(c) controlling
Answer:
controlling

Question 3.
……………. sets out standards for controlling.
(a) Staffing
(b) Planning
(c) Co-ordinating
Answer:
Planning

Question 4.
Organizational function is important for execution of the plans which have been prepared by ……………. management.
(a) top level
(b) middle level
(c) lower level
Answer:
top level

Question 5.
……………… is the function which supports to activate the plans with the help of employees.
(a) Staffing
(b) Directing
(c) Co-ordinating
Answer:
Directing

Question 6.
………………. is the function of execution according to the plan and the organisational structure.
(a) Controlling
(b) Directing
(c) Staffing
Answer:
Directing

Question 7.
………………. arranges the work in such a way that minimum conflicts are raised.
(a) Co-ordinating
(b) Organizing
(c) Controlling
Answer:
Co-ordination.

1. (B) Match the pairs

Question 1.

Answer:

1. (C) Give one word/phrase/term for the following statements

Question 1.
The right person at the job with right pay.
Answer:
Staffing

Question 2.
A person who shows the correct path as well as guides employees in solving the problems.
Answer:
Director

Question 3.
First function of management.
Answer:
Planning

Question 4.
Last function of management.
Answer:
Controlling

Question 5.
It is an intellectual process of logical thinking and rational decision-making.
Answer:
Planning

Question 6.
The term that is used to denote the structure.
Answer:
Organisation

Question 7.
It is the process of attracting, recruiting, selecting, placing, appraising and remunerating the people.
Answer:
Staffing

Question 8.
The process that leads the employees towards the accomplishment of organisational goals.
Answer:
Directing

Question 9.
It increases the team spirit of work place.
Answer:
Co-ordinating

Question 10.
It is the process of comparing the actual performance with the predetermined standard performance.
Answer:
Controlling.

1. (D) State whether the following statements are True or False

Question 1.
Every function of management is not based on planning.
Answer:
False

Question 2.
Specialization in activities leads to increase in organisational efficiency.
Answer:
True

Question 3.
Qualified, efficient and skilled work force is always an asset of the organization.
Answer:
True

Question 4.
Cooperation is not necessary for smooth flow of organisational activities.
Answer:
False

Question 5.
Co-ordination motivates the employees to take initiative while completing their assigned task.
Answer:
True

Question 6.
Standards are not set for every performance in controlling function.
Answer:
False

1. (E) Find the odd one

Question 1.
Planning, Organizing, Staffing, Writing.
Answer:
Writing

Question 2.
Selecting, Training, Co-ordinating, Placing
Answer:
Co-ordinating.

1. (F) Complete the sentences

Question 1.
The tasks of getting the things done by others is known as ……………..
Answer:
Management

Question 2.
The functions of manager start with …………………
Answer:
Planning

Question 3.
The …………….. function of management initiates action
Answer:
Directing

Question 4.
Recruitments are done under ……………….. function.
Answer:
Staffing

Question 5.
………………. is the fundamental function of management.
Answer:
Planning

Question 6.
………………. integrates departmental activities for achieving common goal of the organisation.
Answer:
Co-ordinating

Question 7.
……………… is the last function of management.
Answer:
Controlling

1. (G) Select the correct option from the bracket

Question 1.
Planning is a detailed programme of (present/ future/past) course of action.
Answer:
future

Question 2.
Directing is a responsibility of (manager/ workers/people) at all levels.
Answer:
manager

Question 3.
Qualified, efficient and skilled workforce is always an (liabilities/assets/expenses) of the organization.
Answer:
assets.

1. (H) Answer in one sentence

Question 1.
What is management?
Answer:
The tasks of getting the things done by others to achieve organisational goal is called management.

Question 2.
What is planning?
Answer:
Planning means deciding in advance what to do when to do, how to do, where to do it and who is to do it.

Question 3.
What is staffing?
Answer:
The process of attracting, recruiting, selecting, placing, appraising, remunerating, developing and retaining the best workforce is called staffing.

Question 4.
What is directing?
Answer:
Directing is the process of instructing, guiding, communicating, inspiring, motivating and supervising the employees to achieve the pre-determined goals of the organisation.

Question 5.
What is controlling ?
Answer:
Controlling is a function of comparing the actual performance with the predetermined standard performance to measure deviation if any, identifying causes of deviation and suggest corrective measures.

1. (I) Correct the underlined word and rewrite the following sentences

Question 1.
Factors of business environment are always fixed.
Answer:
Factors of business environment are always changing.

Question 2.
Staffing is concerned with machines.
Answer:
Staffing is concerned with humans.

Question 3.
Directing is a function of comparing the actual performance with the pre-determined performance.
Answer:
Controlling is a function of comparing the actual performance with the pre-determined performance.

Question 4.
Co-ordination helps to maximise the wastage of resources and controls the cost of work.
Answer:
Co-ordination helps to minimise the wastage of resources and controls the cost of work.

Question 5.
Controlling measures are rigid to some extent.
Answer:
Controlling measures are flexible to some extent.

1. (J) Arrange in proper order

Question 1.
Controlling, Organizing, Planning.
Answer:
Planning, Organising, Controlling.

Question 2.
Directing, Co-ordinating, Staffing.
Answer:
Staffing, Directing, Co-ordinating.

2. Explain the following terms/concepts

Question 1.
Management
Answer:
The task of getting the work done by others to achieve organisational goal is called management. According to L. A. Allen, ‘Management is what manager does. Management is a set of principles which relate to the various functions such as planning, organising, staffing, directing, co-ordinating, controlling, etc. which are helpful in achieving organisational goals.

Question 2.
Planning
Answer:
Planning is the basic function of management. Planning is an intellectual process of logical thinking and rational decision-making. It includes deciding the things to be done in advance. In short, planning is a detailed programme of future course of action. Proper planning and its implementation is key to achieve the objectives of an organisation.

Question 3.
Organising
Answer:
Organising is the process of identifying, bringing the required resources together such as men, money, material, machines and method and arranging them in proper manner to achieve the goals of an organisation. It is prepared by the top level management. Organising function decides the ways and means to achieve what has been planned. Organising is more important in executing the plan.

Question 4.
Staffing
Answer:
Staffing is the function of execution according to plan and organisational structure. It is the process of attracting, recruiting, training, developing, appraising, remunerating, developing and retaining the best workforce. Right person at right job with right pay is the basic principle of staffing. This function is concerned with managing humans and not material.

Question 5.
Directing
Answer:
Directing is the process of instructing, guiding, communicating, inspiring, motivating and supervising the employees to achieve pre-determined goals of an organisation. Director shows correct path as well as guides the employees in solving the problems wherever necessary. Directing is the soul of management function.

3. Study the following case/situation and express your opinion

Question 1.
Mr. Ram, an emerging entrepreneur has designed a structure of his business organization by taking into consideration the required resources such as land, money, machinery, workforce, etc. for his new business. He appointed Mr. Shyam as a manager. Mr. Ram has assigned the responsibilities such as recruitment, selection, training and development and to determine the remuneration of the employees to Mr. Shyam. Mr, Ram. has also appointed Mr. Shubham to supervise the work done by the employees according to the standards given to the employees, Mr. Shubham has also to suggest the remedies to the employees wherever necessary. On this context, find out the management functions performed by
(i) Mr. Ram
(ii) Mr. Shyam
(iii) Mr. Shubham
Answer:
(i) Mr. Ram performs the function of planning and organising. He is an emerging entrepreneur and plans the business structure and organises different resources.

(ii) Mr Shyam is performing the function of staffing as his main duty is to recruit, select, train and develop the employees and to decide their remuneration accordingly.

(iii) Mr. Subham is performing the function of controlling. He compares actual performances of employees with standard performance given. He discovers causes of deviations and suggests remedies to overcome deviations.

Question 2.
In XYZ Company, Mr. Lele gives instructions to the employees working under him, provide guidance and motivates them for their best performance. On the other hand, Mr. Sawed takes effort to harmonize the work done by the employees of different departments while achieving organisational goal. Mr. Desai is looking after the arrangement of required resources the business organization.
Mention the name of employee engaged in following functions :
(i) Organisation
(ii) Direction
(iii) Coordination
Answer:
(i) Mr Desai is engaged in the organising function as he is looking after arrangement of required resources for the business organisation.
(ii) Mr. Lele is engaged in the function of directing as he gives instructions to the employees working under him, provides guidance and motivates them for their best performance.
(iii) Mr. Sayyed is engaged in the function of co-ordination as he takes effort to harmonize the work done by the employees of different departments.

4. Distinguish between

Question 1.
Planning and Organising
Answer:

Question 2.
Organising and Staffing
Answer:

Question 3.
Staffing and Directing
Answer:

Question 4.
Directing and Controlling

Question 5.
Co-ordination and Controlling
Answer:

Question 6.
Planning and Controlling
Answer:

Question 7.
Organising and Directing

Question 8.
Organising and Co-ordinating
Answer:

5. Answer in brief

Question 1.
Explain any five points of importance of planning.
Answer:
Importance : The importance of planning is explained as follows:
(1) Helps to set clear objectives : Planning is the process of setting objectives, targets and formulating plans to achieve these objectives. With the help of proper planning, management can analyse the present condition of the organisation and can identify the ways of attaining the desired position in future.

(2) Provides path of action : Planning ensures that the goals or objectives are clearly set. It acts as a guide and provides direction for doing the right things at the right time and in a right way. It helps the employees to understand the organisational goals and what they must do to achieve the same.

(3) Planning improves performance : It helps manager to improve future performances of employees by setting clear objectives and selecting a right course of action. It leads to efficiency in working of the employees. Due to proper planning the employees can work according to guidelines which helps them to improve performance. This results into higher profitability of the organisation.

(4) Minimizes the risk : Planning is the process of looking into the future and anticipating the future changes. By deciding in advance the task to be performed, planning helps to deal with future changes and unforeseen events. Planning helps in anticipation of risk and decide preventive measures accordingly. Though changes or risks cannot be eliminated but proper planning minimizes them.

(5) Planning leads to optimum utilization of resources: Plans are made on the basis of availability of resources with proper allocation for various activities. Proper allocation of resources brings higher efficiency and desired results with minimum wastages.

Question 2.
Explain any five points of importance of organizing.
Answer:
Importance : The importance of organising is explained as follows:
(1) Facilitates administration as well as operation : Organising is the process of identifying, grouping and assigning the activities of administration and proceeding according to its operational activity. Due to proper grouping of the task and employees, there is a reduction in duplication of work results in effective delegation.

(2) Brings specialisation : Organising starts from dividing the total work into smaller units and assigning them to different individuals according to their qualification, capabilities and experience. It leads to increase in overall productivity.

(3) Defines job properly : In the organising function the employees are assigned different jobs according to their qualification, skill and experience and the managers clearly define the details of each job. It clearly spells out what exactly has to be done in every job by each employee.

(4) Clarifies authority and responsibility : The organising function clearly defines authority, power, position of every manager and responsibility, accountability of every employee. This enables proper execution of work and at the same time eliminates confusion, duplication, misunderstanding. It also helps to bring efficiency in working of managers.

(5) Establishes Co-ordination : Organising function helps in establishing co-ordination among different activities of different department. Organising defines clear cut relationship among various positions and ensures mutual co-operation amongst them. Organising helps in co-ordination between different levels of managers of different departments for smooth functioning of an organisation.

Question 3.
Describe any five points of importance of staffing.
Answer:
Importance : The importance of staffing is given as follows:
(1) Effective management function : Staffing is considered an effective managerial function as it deals with human resource. Employees appointed in the organisation through staffing function perform various activities in different areas of the organisation such as production, marketing, finance, etc.

(2) Effective utilization of Human Resources : A well organised staffing department discovers the talented, skilful, experienced and qualified staff. Proper care is taken at every stage of recruitment, selection, placement, etc. It ensures smooth functioning of all the managerial areas of the organisation.

(3) Builds relationship : A sound staffing policy creates a team spirit among the employees. Due to team spirit, a sense of belongingness among the employees is developed. This in turn leads to better communication and co-ordination of managerial efforts in an organisation. A smooth human relation is the key to better flow of co-ordination in an organisation.

(4) Helps Human Resource Development: Skilled and experienced employee is an asset of a business organisation. Staffing function of management is mainly concerned with human factor of production. Efforts are made to utilise the human resources more efficiently.

(5) Helps in effective use of technology and other resources : Staffing function trains employees to use latest technology, capital, material, and method of work more effectively. This brings competitive strength to the organisation. It also helps in improving standard of work and productively in terms of quality and quantity.

(6) Improve efficiency : Regular training and development programmes provide to employees to improve their performance levels. Through proper selection the organisation gets talented and quality employees.

Question 4.
Explain any five points of importance of directing.
Answer:
Importance : The importance of Directing is as follows:
(1) Initiates action : Direction initiates action. It activates employees to put in their best efforts, to achieve the goals. Without effective direction, other managerial functions like planning, organising, staffing, co-ordinating and controlling become ineffective. Managers have to stimulate action by issuing instructions like what to do, how to do, etc. to the subordinates and by supervising them from time to time.

(2) Integrates efforts: At every level of the management there are subordinates under managers. The work assigned to these subordinates is interrelated. The directing function integrates the activities of the subordinates by guidance, supervision and counselling. It results in achievement of organisational goals.

(3) Means of motivation: Objectives of an organisation can be achieved only if the people working in it are properly motivated through monetary and non-monetary incentives. It boosts the morale of employees, contribute their maximum efforts and motivates them to give their best.

(4) Provides stability: Effective direction through supervision, motivation, leadership and communication provides stability and maintains balance in the organisation. This in turn results in the growth of the enterprise at faster rate. For long term survival of the organisation, stability in the organisation are necessary.

(5) Coping up with the changes : Effective direction facilitates changes in the organisation. It enables the enterprise to adopt advance technology, new methods of production, modern techniques of management, etc. It is a direction function which helps the superiors to motivate the subordinates to adapt to the new changes, new challenges, etc. Adapting to the environmental changes is necessary for the growth of the organisation.

Question 5.
Describe any five points of importance of ! coordinating.
Answer:
Importance : The importance of Co-ordinating is explained as follows:
(1) Encourages Team Spirit : Co-ordination is concerned with integrated group efforts. Team work under the direction of the manager encourages the subordinates to work sincerely and give better performance to achieve organisational goals. Co-ordination helps to reduce the conflicts between the employees and departments regarding policies, roles, etc. and increase their team spirit.

(2) Gives Proper Direction : Group or combined efforts of all the employees in an organisation helps to co-ordinate with each other and achieve the desired goals. Thus, combined efforts of all the employees always help an organisation to remove its limitations and achieve organisational objectives. The interdependence of departments gives proper direction to the employees.

(3) Facilitates motivation : In the process of co-ordination the superiors motivate their subordinates by providing them with monetary and other incentives. An effective co-ordination increases efficiency and results in growth and prosperity of the organisation which encourages job security, high income, promotion and incentives.

(4) Optimum utilisation of resources : Proper and effective co-ordination helps to bring together all the resources of the organisation. This in turn helps to make the optimum possible use of available resources to achieve organisational goals. Co-ordination also helps to avoid wastage of resources and control the cost.

(5) Achieve organisational objectives : Proper coordination helps to reduce wastages, delays in completion of targets, departmental disputes, etc. of the organisation to a great extent. This ensures smooth working of the organisation in the process of achievement of objectives.

6. Justify the following statements

Question 1.
Planning is the first function of management.
Answer:
(1) Planning is the basic function of management. Every function of management is based on planning. Planning is an intellectual process of logical thinking and rational decision i making.

(2) Designing i.e. doing a proper planning and implementing it accordingly is the key of achieving the objectives of organisation.

(3) Planning means deciding in advance what to do, when to do, how to do, where to do and who is to do it. Thus, it is a detailed programme of future courses of action.

(4) Planning involves setting objectives, identifying alternative courses of action and selecting the best plan. It focuses on organisation’s objective and develop various course of action to achieve those goals.

Question 2.
Controlling is the last function of management.
Answer:
(1) It is important for am organisation to keep a check on whether things are moving as per plan or not. So controlling function comes as the last but indispensable function of management. The effectiveness of planning can be determined with the function of controlling.

(2) Controlling function helps in comparing the actual performance with the pre-determined standard and performance. It is the process of bringing about conformity of performance with planned action.

(3) Controlling function helps in measuring deviation, if any, identifies the course of deviation and suggests corrective measures. The process of controlling helps in formulation of future plans also.

(4) Controlling helps in checking and measuring performance at all the levels of management, as it compares and finds deviation, analyses the causes of deviation and suggests corrective measures. All planning may fail in the absence of proper controlling measures.

Question 3.
Organizing facilitates administration as well as operation of the organization.
Answer:
(1) Organising function is also called as ‘doing function’ i.e. putting the plan into action. Administration and operation both are doing function as organising is the process of putting together various resources and activities of the organisation into a system.

(2) Organising involves identifying the activities and grouping of relative activities of administration and operational department.

(3) Organising function defines, departmentalizes and assigns activities so that they can be most effectively executed for the smooth flow of administration.

(4) Due to proper grouping of the tasks and the employees, there is increase in production and reduction in wastage. The duplication of work can be avoided and effective delegation becomes possible.

Question 4.
Right person at right job with right pay is the basic principle of staffing.
Answer:
(1) The main function of staffing is to select the right person for the right job with right pay. Selecting the right person for the right job brings efficiency and specialisation in the organisation.

(2) It also bring job satisfaction as adequate remuneration increases morale of the employees. Training and development programmes and job security are the factors which are important in providing job satisfaction.

(3) Proper selection of qualified, efficient and skilful work force is always an asset of the organisation. Proper selection of employees contributes in the higher efficiency and leads to long term positive effects in the organisation.

(4) With proper selection process, right persons for right jobs are placed and regularly appraised on merit basis. The criteria of appraised are duly communicated which brings peace and harmony in the organisation.

Question 5.
Co-ordination between different functions and all levels of management is the essence of organisational success,
Answer:
(1) Co-ordination is an integration of different activities which is essential for their smooth flow. It establishes harmony among all the activities of an organisation in achieving desired goals. Co-ordination will not exist unless efforts are taken at all levels of management.

(2) Co-ordination is the synchronization of the efforts of a group so as to provide unity of action for organisational goals. It is a hidden force which binds all other functions at all levels of management.

(3) In an organisation, a number of persons are working together to achieve a common goal. Their work is closely linked with each other. Co-ordination function brings all the group efforts together and harmonise them carefully.

(4) Co-ordination is orderly arrangement of group efforts to provide unity of action to achieve common goals. Co-operation, team work and higher efficiency level lead to attainment of goals and thus, it is the essence of organisational success.

7. Attempt the following

Question 1.
Explain the importance of planning.
Answer:
Importance : The importance of planning is explained as follows:
(1) Helps to set clear objectives : Planning is the process of setting objectives, targets and formulating plans to achieve these objectives. With the help of proper planning, management can analyse the present condition of the organisation and can identify the ways of attaining the desired position in future.

(2) Provides path of action : Planning ensures that the goals or objectives are clearly set. It acts as a guide and provides direction for doing the right things at the right time and in a right way. It helps the employees to understand the organisational goals and what they must do to achieve the same.

(3) Planning improves performance : It helps manager to improve future performances of employees by setting clear objectives and selecting a right course of action. It leads to efficiency in working of the employees. Due to proper planning the employees can work according to guidelines which helps them to improve performance. This results into higher profitability of the organisation.

(4) Minimizes the risk : Planning is the process of looking into the future and anticipating the future changes. By deciding in advance the task to be performed, planning helps to deal with future changes and unforeseen events. Planning helps in anticipation of risk and decide preventive measures accordingly. Though changes or risks cannot be eliminated but proper planning minimizes them.

(5) Planning leads to optimum utilization of resources: Plans are made on the basis of availability of resources with proper allocation for various activities. Proper allocation of resources brings higher efficiency and desired results with minimum wastages.

Question 2.
Describe the importance of organizing.
Answer:
Importance : The importance of organising is explained as follows:
(1) Facilitates administration as well as operation : Organising is the process of identifying, grouping and assigning the activities of administration and proceeding according to its operational activity. Due to proper grouping of the task and employees, there is a reduction in duplication of work results in effective delegation.

(2) Brings specialisation : Organising starts from dividing the total work into smaller units and assigning them to different individuals according to their qualification, capabilities and experience. It leads to increase in overall productivity.

(3) Defines job properly : In the organising function the employees are assigned different jobs according to their qualification, skill and experience and the managers clearly define the details of each job. It clearly spells out what exactly has to be done in every job by each employee.

(4) Clarifies authority and responsibility : The organising function clearly defines authority, power, position of every manager and responsibility, accountability of every employee. This enables proper execution of work and at the same time eliminates confusion, duplication, misunderstanding. It also helps to bring efficiency in working of managers.

(5) Establishes Co-ordination : Organising function helps in establishing co-ordination among different activities of different department. Organising defines clear cut relationship among various positions and ensures mutual co-operation amongst them. Organising helps in co-ordination between different levels of managers of different departments for smooth functioning of an organisation.

Question 3.
Explain the importance of staffing.
Answer:
Importance : The importance of staffing is given as follows:
(1) Effective management function : Staffing is considered an effective managerial function as it deals with human resource. Employees appointed in the organisation through staffing function perform various activities in different areas of the organisation such as production, marketing, finance, etc.

(2) Effective utilization of Human Resources : A well organised staffing department discovers the talented, skilful, experienced and qualified staff. Proper care is taken at every stage of recruitment, selection, placement, etc. It ensures smooth functioning of all the managerial areas of the organisation.

(3) Builds relationship : A sound staffing policy creates a team spirit among the employees. Due to team spirit, a sense of belongingness among the employees is developed. This in turn leads to better communication and co-ordination of managerial efforts in an organisation. A smooth human relation is the key to better flow of co-ordination in an organisation.

(4) Helps Human Resource Development: Skilled and experienced employee is an asset of a business organisation. Staffing function of management is mainly concerned with human factor of production. Efforts are made to utilise the human resources more efficiently.

(5) Helps in effective use of technology and other resources : Staffing function trains employees to use latest technology, capital, material, and method of work more effectively. This brings competitive strength to the organisation. It also helps in improving standard of work and productively in terms of quality and quantity.

(6) Improve efficiency : Regular training and development programmes provide to employees to improve their performance levels. Through proper selection the organisation gets talented and quality employees.

Question 4.
Explain the importance of directing.
Answer:
Importance : The importance of Directing is as follows:
(1) Initiates action : Direction initiates action. It activates employees to put in their best efforts, to achieve the goals. Without effective direction, other managerial functions like planning, organising, staffing, co-ordinating and controlling become ineffective. Managers have to stimulate action by issuing instructions like what to do, how to do, etc. to the subordinates and by supervising them from time to time.

(2) Integrates efforts: At every level of the management there are subordinates under managers. The work assigned to these subordinates is interrelated. The directing function integrates the activities of the subordinates by guidance, supervision and counselling. It results in achievement of organisational goals.

(3) Means of motivation: Objectives of an organisation can be achieved only if the people working in it are properly motivated through monetary and non-monetary incentives. It boosts the morale of employees, contribute their maximum efforts and motivates them to give their best.

(4) Provides stability: Effective direction through supervision, motivation, leadership and communication provides stability and maintains balance in the organisation. This in turn results in the growth of the enterprise at faster rate. For long term survival of the organisation, stability in the organisation are necessary.

(5) Coping up with the changes : Effective direction facilitates changes in the organisation. It enables the enterprise to adopt advance technology, new methods of production, modern techniques of management, etc. It is a direction function which helps the superiors to motivate the subordinates to adapt to the new changes, new challenges, etc. Adapting to the environmental changes is necessary for the growth of the organisation.

Question 5.
Describe the importance of coordinating.
Answer:
Importance : The importance of Co-ordinating is explained as follows:
(1) Encourages Team Spirit : Co-ordination is concerned with integrated group efforts. Team work under the direction of the manager encourages the subordinates to work sincerely and give better performance to achieve organisational goals. Co-ordination helps to reduce the conflicts between the employees and departments regarding policies, roles, etc. and increase their team spirit.

(2) Gives Proper Direction : Group or combined efforts of all the employees in an organisation helps to co-ordinate with each other and achieve the desired goals. Thus, combined efforts of all the employees always help an organisation to remove its limitations and achieve organisational objectives. The interdependence of departments gives proper direction to the employees.

(3) Facilitates motivation : In the process of co-ordination the superiors motivate their subordinates by providing them with monetary and other incentives. An effective co-ordination increases efficiency and results in growth and prosperity of the organisation which encourages job security, high income, promotion and incentives.

(4) Optimum utilisation of resources : Proper and effective co-ordination helps to bring together all the resources of the organisation. This in turn helps to make the optimum possible use of available resources to achieve organisational goals. Co-ordination also helps to avoid wastage of resources and control the cost.

(5) Achieve organisational objectives : Proper coordination helps to reduce wastages, delays in completion of targets, departmental disputes, etc. of the organisation to a great extent. This ensures smooth working of the organisation in the process of achievement of objectives.

Question 6.
Explain the importance of controlling.
Answer:
Importance : The importance of controlling function is explained as follows:
(1) Fulfilling goals of organisation : Controlling helps to fulfil and achieve organisational goals. The controlling function ensures that the activities take place according to the plans and if there is any deviation, timely action is taken. When all the activities are conducted successfully, according to plan the organisational goals can be achieved as desired.

(2) Making efficient utilisation of resources : By using various control techniques, managers can keep a close watch over the utilisation of human, physical and financial resources. They can prevent the misuse or wastage of resources and ensure proper utilisation of the same.

(3) Accuracy of standards : Proper and efficient control system help the management to check the standards set are accurate or not. This system also keeps check on the changes taking place in the organisation from time to time. Controlling functions are flexible to some extent. This in turn facilitates the organisation to review the standards by considering such changes.

(4) Motivates Employees : A good control system gives information in advance about the standard performance and discovers efficient and inefficient employees. Efficient employees may be given Financial rewards or incentives to motivate them further. The manager may recommend motivational measures in case it finds that deviations are due to insufficient motivation.

(5) Ensures order and discipline : An efficient and good control system ensures order and discipline in the organisation. It prevents and reduces unnecessary behaviour on the part of employees. Under this system, regular checking is done by the managers or departmental heads and preventive measures are taken against deviation or indiscipline.

8. Answer the following

Question 1.
Define the term Planning and explain the importance of planning.
Answer:
[A] Meaning : Planning is the fundamental and basic function of management. It is a process of setting goals and choosing the means to achieve these goals. Planning means deciding the future course of action which determines what is to be done, how to do it, when to do it, who is to do it and how results are to be evaluated. It is a detailed programme in which all activities to be performed in future are mentioned keeping in mind the objectives. Thus, it is an intellectual process of logical thinking and rational decision-making.

[B] Importance : The importance of planning is explained as follows:
(1) Helps to set clear objectives : Planning is the process of setting objectives, targets and formulating plans to achieve these objectives. With the help of proper planning, management can analyse the present condition of the organisation and can identify the ways of attaining the desired position in future.

(2) Provides path of action : Planning ensures that the goals or objectives are clearly set. It acts as a guide and provides direction for doing the right things at the right time and in a right way. It helps the employees to understand the organisational goals and what they must do to achieve the same.

(3) Planning improves performance : It helps manager to improve future performances of employees by setting clear objectives and selecting a right course of action. It leads to efficiency in working of the employees. Due to proper planning the employees can work according to guidelines which helps them to improve performance. This results into higher profitability of the organisation.

(4) Minimizes the risk : Planning is the process of looking into the future and anticipating the future changes. By deciding in advance the task to be performed, planning helps to deal with future changes and unforeseen events. Planning helps in anticipation of risk and decide preventive measures accordingly. Though changes or risks cannot be eliminated but proper planning minimizes them.

(5) Planning leads to optimum utilization of resources: Plans are made on the basis of availability of resources with proper allocation for various activities. Proper allocation of resources brings higher efficiency and desired results with minimum wastages.

(6) Helps in decision-making : Planning helps the management to achieve to take a rational decision and to select best alternatives by considering all positive and negative outcomes of all the alternatives the decisions are taken after selecting the best suitable alternatives a predefined goals.

(7) Useful is setting the standards for controlling: Planning sets the standards of performance to be achieved and which can be measured with the actual performance for find out about any deviation. Such deviation can be taken care by controlling steps. Thus, planning provides basis for maintaining discipline in an organisation.

(8) Facilitates co-ordination of all activities : Proper planning reduces the overlapping among all activities of business which are closely linked with each other. Planning interrelates such activities of all department work as per overall plan and thus management co-ordination is achieved.

(9) Facilitates other functions : Planning is the primary function of all the functions of management. Every organisational function is set to achieve the organisational goals at the planning stage. Other management functions such as organising, staffing, etc. cannot be undertaken till the plan is ready.

(10) Promotes innovative ideas : Planning is the basic function. The process of decision-making involves promotion of innovative ideas after critical
thinking. It is the most challenging activity for the management as it guides all future activities and actions of an organisation. In the end, these innovative plans result in attainment of the organisation goals.

Question 2.
What is Organising? Explain the importance of organising.
Answer:
[A] Meaning: Organising is the process of putting together various activities, resource and people into a system so that people work together for achieving planned objectives. Organising means arranging everything in an orderly manner. It means making arrangements like money, machinery, materials, man-power and other physical resources to achieve the predefined goals. The synchronization and combination of workforce, physical, financial and information resources in the process of organising.

[B] Importance : The importance of organising is explained as follows:
(1) Facilitates administration as well as operation : Organising is the process of identifying, grouping and assigning the activities of administration and proceeding according to its operational activity. Due to proper grouping of the task and employees, there is a reduction in duplication of work results in effective delegation.

(2) Brings specialisation : Organising starts from dividing the total work into smaller units and assigning them to different individuals according to their qualification, capabilities and experience. It leads to increase in overall productivity.

(3) Defines job properly : In the organising function the employees are assigned different jobs according to their qualification, skill and experience and the managers clearly define the details of each job. It clearly spells out what exactly has to be done in every job by each employee.

(4) Clarifies authority and responsibility : The organising function clearly defines authority, power, position of every manager and responsibility, accountability of every employee. This enables proper execution of work and at the same time eliminates confusion, duplication, misunderstanding. It also helps to bring efficiency in working of managers.

(5) Establishes Co-ordination : Organising function helps in establishing co-ordination among different activities of different department. Organising defines clear cut relationship among various positions and ensures mutual co-operation amongst them. Organising helps in co-ordination between different levels of managers of different departments for smooth functioning of an organisation.

(6) Helps for effective administration : A sound organising structure facilitates in defining the right job to the right individual. Similarly, the functions, duties and role of each and every employee are well defined in the organising function. This facilitates effective administration and ultimately leads to efficient administration.

(7) Helpful for growth and diversification : Smooth and efficient functioning, clearly defined authority and responsibilities and smooth co-ordination leads to the growth of the organisation. Use of appropriate techniques of control brings efficiency and reduces wastages which ultimately leads to higher profitability of the organisation. All this is possible when the structure of the organisation is well defined.

(8) Creates sense of security: Organising function defines and clarifies the jobs, functions and roles, powers and authority assigned to every manager and employee. Clarity in job profile eliminates confusion and gives responsibility. It helps a lot in getting mental satisfaction and develops sense of security.

(9) Scope for innovation : The manager can use his talent, knowledge and experience to take decisions on various matters and problems. For instance, decision to adopt new technique of production in the organisation. Thus, his talent flourishes by adopting new changes in the methods of work.

(10) Optimum utilisation of resources : Organising function lays down the best possible uses of resources for a specific job. Thus, it is possible to use the available resources to their optimum level and thereby avoid wastage as well as their excessive use.

Question 3.
What do you mean by Staffing? Describe the importance of staffing.
Answer:
[A] Meaning : Right person at right job with right pay is the basic principle of staffing, Staffing is the process involved in attracting, identifying, assessing, recruiting, placing, evaluating and directing employees. It is recruitment, selection, development, training and compensation of employees. It is very challenging for the organisation to focus on best utilisation of workforce by using their talents and skills, retaining them and arranging training and t development programme.

[B] Importance : The importance of staffing is given as follows:
(1) Effective management function : Staffing is considered an effective managerial function as it deals with human resource. Employees appointed in the organisation through staffing function perform various activities in different areas of the organisation such as production, marketing, finance, etc.

(2) Effective utilization of Human Resources : A well organised staffing department discovers the talented, skilful, experienced and qualified staff. Proper care is taken at every stage of recruitment, selection, placement, etc. It ensures smooth functioning of all the managerial areas of the organisation.

(3) Builds relationship : A sound staffing policy creates a team spirit among the employees. Due to team spirit, a sense of belongingness among the employees is developed. This in turn leads to better communication and co-ordination of managerial efforts in an organisation. A smooth human relation is the key to better flow of co-ordination in an organisation.

(4) Helps Human Resource Development: Skilled and experienced employee is an asset of a business organisation. Staffing function of management is mainly concerned with human factor of production. Efforts are made to utilise the human resources more efficiently.

(5) Helps in effective use of technology and other resources : Staffing function trains employees to use latest technology, capital, material, and method of work more effectively. This brings competitive strength to the organisation. It also helps in improving standard of work and productively in terms of quality and quantity.

(6) Improve efficiency : Regular training and development programmes provide to employees to improve their performance levels. Through proper selection the organisation gets talented and quality employees.

(7) Long term effect : Sub-functions of staffing, namely, proper selection, training, development, motivation, etc. help to achieve long-term benefits such as increase in productivity and efficiency, loyalty of customers and employees, etc.

(8) Essential contribution : The selection of employees should be based on the ability of the prospective candidates to meet the future challenges. Selection is based on the ability of the prospective employee so that organisation can meet the future challenges wisely. Therefore, in staff selection, the selectors should take into account the contribution of the employees in their future roles.

(9) Provides job satisfaction : A good staffing policy creates job satisfaction in the minds of the employees. For instance, proper placement of the individuals according to their knowledge, experience and aptitude, timely promotions, training etc. give job satisfaction. Fair remuneration and job security are the factors which are important in providing job satisfaction.

(10) Maintains harmony: Proper staffing policy helps to develop good labour relations. The performances of employees are regularly appraised and promotions are made on merits. Due to this, employees develop positive attitude towards the management which, in turn, helps to bring about peace and harmony in the organisation.

Question 4.
Give the meaning of Directing and explain the importance of directing.
Answer:
[A] Meaning : Directing is the process of instructing, guiding, inspiring, motivating and supervising the employees to achieve pre-determined organisational goals. It is a continuous function started at top level and flows through the lower level of an organisation. It is continued through out the tenure of an organisation. A few philosophers called it as ‘Life spark of an Enterprise. Director shows the correct path as well as guides the employees in solving the problems.

[B] Importance : The importance of Directing is as follows:
(1) Initiates action : Direction initiates action. It activates employees to put in their best efforts, to achieve the goals. Without effective direction, other managerial functions like planning, organising, staffing, co-ordinating and controlling become ineffective. Managers have to stimulate action by issuing instructions like what to do, how to do, etc. to the subordinates and by supervising them from time to time.

(2) Integrates efforts: At every level of the management there are subordinates under managers. The work assigned to these subordinates is interrelated. The directing function integrates the activities of the subordinates by guidance, supervision and counselling. It results in achievement of organisational goals.

(3) Means of motivation: Objectives of an organisation can be achieved only if the people working in it are properly motivated through monetary and non-monetary incentives. It boosts the morale of employees, contribute their maximum efforts and motivates them to give their best.

(4) Provides stability: Effective direction through supervision, motivation, leadership and communication provides stability and maintains balance in the organisation. This in turn results in the growth of the enterprise at faster rate. For long term survival of the organisation, stability in the organisation are necessary.

(5) Coping up with the changes : Effective direction facilitates changes in the organisation. It enables the enterprise to adopt advance technology, new methods of production, modern techniques of management, etc. It is a direction function which helps the superiors to motivate the subordinates to adapt to the new changes, new challenges, etc. Adapting to the environmental changes is necessary for the growth of the organisation.

(6) Efficient utilisation of resources : Constant instructions can be given to the subordinates to make the maximum use of the available resources and to make every possible effort to minimize the wastages of resources. Thus, effective direction helps in optimum use of available resources such as men, materials, money and methods which helps to reduce cost and increase profit.

(7) Creates team spirit : The supervisors through proper direction can guide, lead and motivate their subordinates to co-ordinate the activities. Thus, team spirit is created which helps the employees to perform their activities more efficiently and on time. This results in faster achievement of organisational goals.

(9) Increases efficiency level : Effective direction and guidance results in better performance of the employees. It also enables the managers and other superiors to guide the subordinates as the leader while performing their jobs.

(8) Exploring capabilities of individuals: Every employee in the organisation has certain capabilities and potential. Through proper direction, motivation and encouragement manager can utilise them to their optimum level to achieve organisational goals and increases efficiency of organisation.

(10) Co-operation : Co-operation between different departments from top level to the bottom level and among the people within the department is must. Co-operation is essential for the success and achievement of organisational goals and for smooth flow of all organisational activities.

Question 5.
What is Coordinating? Describe the importance of coordinating.
Answer:
[A] Meaning : Co-ordination as a function of management refers to the task of developing harmony between various sections of departments and between various departments of the organisation. Thus, co-ordination is a hidden force which binds all other functions of the management integrating the group activities to accomplish the organisational goal efficiency. As the work of each one is linked in an organisation is necessary in co-ordination.

[B] Importance : The importance of Co-ordinating is explained as follows:
(1) Encourages Team Spirit : Co-ordination is concerned with integrated group efforts. Team work under the direction of the manager encourages the subordinates to work sincerely and give better performance to achieve organisational goals. Co-ordination helps to reduce the conflicts between the employees and departments regarding policies, roles, etc. and increase their team spirit.

(2) Gives Proper Direction : Group or combined efforts of all the employees in an organisation helps to co-ordinate with each other and achieve the desired goals. Thus, combined efforts of all the employees always help an organisation to remove its limitations and achieve organisational objectives. The interdependence of departments gives proper direction to the employees.

(3) Facilitates motivation : In the process of co-ordination the superiors motivate their subordinates by providing them with monetary and other incentives. An effective co-ordination increases efficiency and results in growth and prosperity of the organisation which encourages job security, high income, promotion and incentives.

(4) Optimum utilisation of resources : Proper and effective co-ordination helps to bring together all the resources of the organisation. This in turn helps to make the optimum possible use of available resources to achieve organisational goals. Co-ordination also helps to avoid wastage of resources and control the cost.

(5) Achieve organisational objectives : Proper coordination helps to reduce wastages, delays in completion of targets, departmental disputes, etc. of the organisation to a great extent. This ensures smooth working of the organisation in the process of achievement of objectives.

(6) Improve relations : Co-ordination brings develops good relations among the employees working at different levels of management. For instance, marketing department depends upon production department, production department : depends upon purchase department and so on. Proper co-ordination always helps employees to improve and build strong relations among the employees working in different departments.

(7) Leads to Higher Efficiency : Co-ordination facilitates the optimum use of physical and human resources. This leads to higher returns at lesser cost, thereby higher efficiency. Co-ordination ultimately leads to the optimum use of the resources, higher efficiency reduction in cost and reduction in wastages.

(8) Improves goodwill : Higher sales and higher profitability can be achieved due to synchronized efforts. It earns a name and goodwill in the corporate world. This leads to better value of shares in the stock exchange (market).

(9) Unity of direction : Co-ordinating function helps to bring together activities of different departments to achieve common goals and objectives of the organisation. Therefore, co¬ordination is needed to give proper direction to all the departments of the organisation.

(10) Specialisation : In every business organisation all departments are headed by qualified and specialised professionals in their respective field. The specialised knowledge of these departmental heads helps in various managerial decisions. Proper and efficient co-ordination among these professionals helps to achieve organisational goals (targets) as planned by the top management.

Question 6.
Define the term Controlling and explain the importance of controlling.
Answer:
[A] Meaning : Controlling is a function of comparing the actual performance with the predetermined standard performance. It measures deviation, if any, identifies the causes and suggest the corrective measures. It is performed by all levels of management. Controlling is an indispensable function at all levels of management.

[B] Importance : The importance of controlling function is explained as follows:
(1) Fulfilling goals of organisation : Controlling helps to fulfil and achieve organisational goals. The controlling function ensures that the activities take place according to the plans and if there is any deviation, timely action is taken. When all the activities are conducted successfully, according to plan the organisational goals can be achieved as desired.

(2) Making efficient utilisation of resources : By using various control techniques, managers can keep a close watch over the utilisation of human, physical and financial resources. They can prevent the misuse or wastage of resources and ensure proper utilisation of the same.

(3) Accuracy of standards : Proper and efficient control system help the management to check the standards set are accurate or not. This system also keeps check on the changes taking place in the organisation from time to time. Controlling functions are flexible to some extent. This in turn facilitates the organisation to review the standards by considering such changes.

(4) Motivates Employees : A good control system gives information in advance about the standard performance and discovers efficient and inefficient employees. Efficient employees may be given Financial rewards or incentives to motivate them further. The manager may recommend motivational measures in case it finds that deviations are due to insufficient motivation.

(5) Ensures order and discipline : An efficient and good control system ensures order and discipline in the organisation. It prevents and reduces unnecessary behaviour on the part of employees. Under this system, regular checking is done by the managers or departmental heads and preventive measures are taken against deviation or indiscipline.

(6) Facilitates co-ordination : Every manager or superior co-ordinates the activities of subordinates towards the process of controlling. Controlling reveals the weak points where co¬ordination falls short, so that the management can take timely action.

(7) Psychological pressure : Efficient control system puts psychological pressure on the employees to perform better. Their performance is measured and compared with standards set from time to time. All the employees know that their performance will be evaluated and hence they put on their best to perform well.

(8) Ensures Organisational Efficiency and Effectiveness : Efficient and proper control system ensures organisational efficiency and ; effectiveness. The factors of controlling such as motivation for better performance, achievement of co-ordination in the performance and managers’ responsibility ensure that the organisation works i more efficiently.

(9) Build good Corporate image : An efficient controlling system helps to improve overall efficiency and quality of work. As a result organisation achieves its goals according set standards. This in turn helps to build a good corporate image and develops reputation of the business.

(10) Acts as a Guide : Controlling function provides set of standard performance. All levels of managers and employees work according to it. They follow these standards to achieve desired results. The steps taken for controlling an activity guide the management while planning any future activity.

Balbharti Maharashtra State Board 12th Physics Textbook Solutions Chapter 10 Magnetic Fields due to Electric Current Textbook Exercise Questions and Answers.

Maharashtra State Board 12th Physics Solutions Chapter 10 Magnetic Fields due to Electric Current

1. Choose the correct option.

i) A conductor has 3 segments; two straight and of length L each and a semicircular with radius R. It carries a current I. What is the magnetic field B at point P?

Answer:
(C) \(\frac{\mu_{0}}{4} \frac{I}{R}\)

ii) Figure a, b show two Amperian loops associated with the conductors carrying current I in the sense shown. The \(\oint \vec{B} \cdot d \vec{l}\) in the cases a and b will be, respectively,

Answer:
(A) -μ₀ I, 0

iii) A proton enters a perpendicular uniform magnetic field B at origin along the positive x axis with a velocity v as shown in the figure. Then it will follow the following path. [The magnetic field is directed into the paper].

(A) It will continue to move along positive x axis.
(B) It will move along a curved path, bending towards positive x axis.
(C) It will move along a curved path, bending towards negative y axis.
(D) It will move along a sinusoidal path along the positive x axis.
Answer:
(C) It will move along a curved path, bending towards negative y axis.

(iv) A conducting thick copper rod of length 1 m carries a current of 15 A and is located on the Earth’s equator. There the magnetic flux lines of the Earth’s magnetic field are horizontal, with the field of 1.3 × 10^-4 T, south to north. The magnitude and direction of the force on the rod, when it is oriented so that current flows from west to east, are
(A) 14 × 10^-4 N, downward.
(B) 20 × 10^-4 N, downward.
(C) 14 × 10^-4 N, upward.
(D) 20 × 10^-4 N, upward.
Answer:
(D) 20 × 10^-4 N, upward.

v) A charged particle is in motion having initial velocity \(\overrightarrow{\mathrm{V}}\) when it enter into a region of uniform magnetic field perpendicular to \(\overrightarrow{\mathrm{V}}\) . Because of the magnetic force the kinetic energy of the particle will
(A) remain uncharged.
(B) get reduced.
(C) increase.
(D) be reduced to zero.
Answer:
(A) remain uncharged.

Question 2.
A piece of straight wire has mass 20 g and length 1m. It is to be levitated using a current of 1 A flowing through it and a perpendicular magnetic field B in a horizontal direction. What must be the magnetic of B?

Answer:
Data: m = 20 g = 2 × 10^-2 kg, l = 1 m, I = 1 A,
g = 9.8 m/s²
To balance the wire, the upward magnetic force must be equal in magnitude to the downward force due to gravity.
∴ F_m = IlB = mg
Therefore, the magnitude of the magnetic field,
B = \(\frac{m g}{I l}=\frac{\left(2 \times 10^{-2}\right)(9.8)}{(1)(1)}\) = 0.196 T

Question 3.
Calculate the value of magnetic field at a distance of 2 cm from a very long straight wire carrying a current of 5 A (Given: µ₀ = 4π × 10^-7 Wb/Am).
Answer:
Data : I = 5A, a = 0.02 m, \(\frac{\mu_{0}}{4 \pi}\) = 10^-7 T∙m/A
The magnetic induction,
B = \(\frac{\mu_{0} I}{2 \pi a}=\frac{\mu_{0}}{4 \pi} \frac{2 I}{a}\) = 10^-7 × \(\frac{2(5)}{2 \times 10^{-2}}\) = 5 × 10^-5 T

Question 4.
An electron is moving with a speed of 3.2 × 10⁶ m/s in a magnetic field of 6.00 × 10^-4 T perpendicular to its path. What will be the radius of the path? What will be frequency and the kinetic energy in keV ? [Given: mass of electron = 9.1 × 10^-31 kg, charge e = 1.6 × 10^-19 C, 1 eV = 1.6 × 10^-19 J]
Answer:

Question 5.
An alpha particle (the nucleus of helium atom) (with charge +2e) is accelerated and moves in a vacuum tube with kinetic energy = 10.00 MeV.On applying a transverse a uniform magnetic field of 1.851 T, it follows a circular trajectory of radius 24.60 cm. Obtain the mass of the alpha particle. [charge of electron = 1.62 × 10^-19 C]
Answer:
Data: 1 eV = 1.6 × 10^-19 J,
E = 10MeV = 10⁷ × 1.6 × 10^-19 J = 1.6 × 10^-12 J
B = 1.88 T, r = 0.242 m, e = 1.6 × 10^-19 C
Charge of an -partic1e,
q = 2e = 2(1.6 × 10^-19)=3.2 × 10^-19 C

This gives the mass of the α-particle.
[Note : The value of r has been adjusted to match with the answer. The CODATA (Committee on Data for Science and Technology) accepted value of m_x is approximately 6.6446 × 10^-27 kg.]

Question 6.
Two wires shown in the figure are connected in a series circuit and the same amount of current of 10 A passes through both, but in apposite directions. Separation between the two wires is 8 mm. The length AB is S = 22 cm. Obtain the direction and magnitude of the magnetic field due to current in wire 2 on the section AB of wire 1. Also obtain the magnitude and direction of the force on wire 1. [µ₀ = 4π × 10^-7 T.m/A]

Answer:
Data: I₁ = I₂ = 10 A, s = 8 mm = 8 × 10^-3 m, l = 0.22 m
By right hand grip rule, the direction of the magnetic field \(\overrightarrow{B_{2}}\) due to the current in wire 2 at AB is into the page and its magnitude is
B₂ = \(\frac{\mu_{0}}{4 \pi} \frac{2 I}{s}=10^{-7} \times \frac{2(10)}{8 \times 10^{-3}}=\frac{1}{4} \times \mathbf{1 0}^{-3}\) T
The current in segment AB is upwards. Then, by Fleming’s left hand rule, the force on it due to \(\overrightarrow{B_{2}}\) is to the left of the diagram, i.e., away from wire 1, or repulsive. The magnitude of the force is
F_{on 1 by 2} = I₁lB₂ = (10)(0.22) × \(\frac{1}{4}\) × 10^-3
= 5.5 × 10^-4 N

Question 7.
A very long straight wire carries a current 5.2 A. What is the magnitude of the magnetic field at a distance 3.1 cm from the wire? [ µ₀ = 4π × 10^-7 T∙m/A]
Answer:
Data : I = 5.2 A, a = 0.031 m, \(\frac{\mu_{0}}{4 \pi}\) = 10^-7 T∙m/A
The magnetic induction,
B = \(\frac{\mu_{0} I}{2 \pi a}=\frac{\mu_{0}}{4 \pi} \frac{2 I}{a}\) = 10^-7 × \(\frac{2(5.2)}{3.1 \times 10^{-2}}\)
= 3.35 × 10^-5 T

Question 8.
Current of equal magnitude flows through two long parallel wires having separation of 1.35 cm. If the force per unit length on each of the wires in 4.76 × 10^-2 N, what must be I ?
Answer:

Question 9.
Magnetic field at a distance 2.4 cm from a long straight wire is 16 µT. What must be current through the wire?
Answer:
Data: a = 2.4 × 10^-2 m, B = 1.6 × 10^-5 T,
\(\frac{\mu_{0}}{4 \pi}\) = 10^-7 T∙m/A
B = \(\frac{\mu_{0} I}{2 \pi a}=\frac{\mu_{0}}{4 \pi} \frac{2 I}{a}\)
The current through the wire,
I = \(\frac{1}{\mu_{0} / 4 \pi} \frac{a B}{2}=\frac{1}{10^{-7}} \frac{\left(2.4 \times 10^{-2}\right)\left(1.6 \times 10^{-5}\right)}{2}\) = 1.92 A

Question 10.
The magnetic field at the centre of a circular current carrying loop of radius 12.3 cm is 6.4 × 10^-6T. What will be the magnetic moment of the loop?
Answer:
Data: R = 12.3cm = 12.3 × 10^-2 m,
B = 6.4 × 10^-6 T, µ₀ = 4π × 10^-7 T∙m/A

= 5.955 × 10^-2 J/T (or A∙m²)

Question 11.
A circular loop of radius 9.7 cm carries a current 2.3 A. Obtain the magnitude of the magnetic field (a) at the centre of the loop and (b) at a distance of 9.7 cm from the centre of the loop but on the axis.
Answer:
Data: R = z = 9.7 cm = 9.7 × 10^-2 m, I = 2.3A, N = 1
(a) At the centre of the coil :
The magnitude of the magnetic induction,
B = \(\frac{\mu_{0} N I}{2 R}\)

Question 12.
A circular coil of wire is made up of 100 turns, each of radius 8.0 cm. If a current of 0.40 A passes through it, what be the magnetic field at the centre of the coil?
Answer:
Data: N = 100,R = 8 × 10^-2 m, I = 0.4A,
µ₀ = 4π × ^-7 T∙m/A
B = \(\frac{\mu_{0} N I}{2 R}\)
= \(\frac{\left(4 \pi \times 10^{-7}\right)(100)(0.4)}{2\left(8 \times 10^{-2}\right)}\) = 3.142 × 10^-4 T

Question 13.
For proton acceleration, a cyclotron is used in which a magnetic field of 1.4 Wb/m² is applied. Find the time period for reversing the electric field between the two Ds.
Answer:
Data: B = 1.4 Wb/m², m = 1.67 × 10^-27 kg,
q = 1.6 × 10^-19 C
T = \(\frac{2 \pi m}{q B}\)
t = \(\frac{T}{2}=\frac{\pi m}{q B}=\frac{(3.142)\left(1.67 \times 10^{-27}\right)}{\left(1.6 \times 10^{-19}\right)(1.4)}\)
= 2.342 × 10^-8 s
This is the required time interval.

Question 14.
A moving coil galvanometer has been fitted with a rectangular coil having 50 turns and dimensions 5 cm × 3 cm. The radial magnetic field in which the coil is suspended is of 0.05 Wb/m². The torsional constant of the spring is 1.5 × 10^-9 Nm/ degree. Obtain the current required to be passed through the galvanometer so as to produce a deflection of 30°.
Answer:
Data : N = 50, C = 1.5 × 10^-9 Nm/degree,
A = lb = 5 cm × 3 cm = 15 cm² = 15 × 10^-4 m²,
B = 0.05Wb/m², θ = 30°
NIAB = Cθ
∴ The current through the coil, I = \(\frac{C \theta}{N A B}\)
= \(\frac{1.5 \times 10^{-9} \times 30}{50 \times 15 \times 10^{-4} \times 0.05}=\frac{3 \times 10^{-5}}{5 \times 0 \cdot 5}\)
= 1.2 × 10^-5 A

Question 15.
A solenoid of length π m and 5 cm in diameter has winding of 1000 turns and carries a current of 5 A. Calculate the magnetic field at its centre along the axis.
Answer:
Data: L = 3.142 m, N = 1000, I = 5A,
μ₀ = 4π × 10^-7 T∙m/A
The magnetic induction,
B = μ₀ nI = μ₀(\(\frac{N}{L}\))I
= (4π × 10^-7)(\(\frac{1000}{3.142}\))(5) = \(\frac{20 \times 3.142 \times 10^{-4}}{3.142}\)
= 2 × 10^-3 T

Question 16.
A toroid of narrow radius of 10 cm has 1000 turns of wire. For a magnetic field of 5 × 10^-2 T along its axis, how much current is required to be passed through the wire?
Answer:
Data : CentraI radius, r = 10 cm = 0.1 m, N = 1000,
B = 5 × 10^-2 T, = 4π × 10^-7 T∙m/A
The magnetic induction,
B = \(\frac{\mu_{0} N I}{2 \pi r}=\frac{\mu_{0}}{4 \pi} \frac{2 N I}{r}\)
2irr 4ir r
∴ 5 × 10^-2 = 10^-7 × \(\frac{2(1000) I}{0.1}\)
∴ I = \(\frac{50}{2}\) = 25A
This is the required current.

Question 17.
In a cyclotron protons are to be accelerated. Radius of its D is 60 cm. and its oscillator frequency is 10 MHz. What will be the kinetic energy of the proton thus accelerated?
(Proton mass = 1.67 × 10^-27 kg, e = 1.60 × 10^-19 C, 1eV = 1.6 × 10^-19 J)
Answer:
Data : R = 0.6 m, f = 10⁷ Hz, m_p = 1.67 × 10^-27 kg,
e = 1.6 × 10^-19C, 1 eV = 1.6 × 10^-19 J

Question 18.
A wire loop of the form shown in the figure carries a current I. Obtain the magnitude and direction of the magnetic field at P. Given : \(\frac{\mu_{0} I}{4 \pi R} \sqrt{2}\)

Answer:
The wire loop is in the form of a circular arc AB of radius R and a straight conductor BCA. The arc AB subtends an angle of Φ = 270° = \(\frac{3 \pi}{2}\) rad at the centre of the loop P. Since PA = PB = R and C is the midpoint of AB, AB = \(\sqrt{2} R\) and AC = CB = \(\frac{\sqrt{2} R}{2}\) = \(\frac{R}{\sqrt{2}}\). Therefore, a = PC = \(\frac{R}{\sqrt{2}}\).

The magnetic inductions at P due to the arc AB and the straight conductor BCA are respectively,
B₁ = \(\frac{\mu_{0}}{4 \pi} \frac{I \phi}{R}\) and B₂ = \(\frac{\mu_{0}}{4 \pi} \frac{2 I}{a}\)
Therefore, the net magnetic induction at P is

This is the required expression.

Question 19.
Two long parallel wires going into the plane of the paper are separated by a distance R, and carry a current I each in the same direction. Show that the magnitude of the magnetic field at a point P equidistant from the wires and subtending angle θ from the plane containing the wires, is B = \(\frac{\mu_{0}}{\pi} \frac{I}{R}\) sin 2θ What is the direction of the magnetic field?
Answer:

In above figure, \(\vec{B}_{1}\) and \(\vec{B}_{2}\) are the magnetic fields in the plane of the page due to the currents in wires 1 and 2, respectively. Their directions are given by the right hand grip rule: \(\vec{B}_{1}\) is perpendicular to AP and makes an angle Φ with the horizontal. \(\vec{B}_{2}\) is perpendicular to BP and also makes an angle Φ with the horizontal.
AP = BP = a = \(\frac{R / 2}{\cos \theta}\)
and B₁ = B₂ = \(\frac{\mu_{0}}{4 \pi} \frac{2 I}{a}=\frac{\mu_{0}}{4 \pi} \frac{2 I(2 \cos \theta)}{R}\)
= \(\frac{\mu_{0}}{\pi} \frac{I}{R}\) cos θ
Since the vertical components cancel out, the magnitude of the net magnetic induction at P is
B_net = 2B₁ cos Φ = 2B₁ cos(90° – θ) = 2B₁ sinθ
= 2(\(\frac{\mu_{0}}{\pi} \frac{I}{R}\) cos θ) sin θ = \(\frac{\mu_{0}}{\pi} \frac{I}{R}\) sin 2θ
as required. is in the plane parallel to that of the wires and to the right as shown in the figure.

Question 20.
Figure shows a section of a very long cylindrical wire of diameter a, carrying a current I. The current density which is in the direction of the central axis of the wire varies linearly with radial distance r from the axis according to the relation J = J_or/a. Obtain the magnetic field B inside the wire at a distance r from its centre.
[Answer: B J r

Answer:
Consider an annular differential element of radius r and width dr. The current through the area dA of this element is
dI = JdA = (J_o \(\frac{r}{a}\))2πrdr = \(\frac{2 \pi J_{0} r^{2} d r}{a}\) …………….. (1)

To apply the Ampere’s circuital law to the circular path of integration, we note that the wire has perfect cylindrical symmetry with all the charges moving parallel to the wire. So, the magnetic field must be tangent to circles that are concentric with the wire. The enclosed current is the current within radius r. Thus,

Question 21.
In the above problem, what will be the magnetic field B inside the wire at a distance r from its axis, if the current density J is uniform across the cross section of the wire?
Answer:
Figure shows the cross section of a long straight wire of radius a that carries a current I out of the page. Because the current is uniformly distributed over the cross section of the wire, the magnet ic field \(\vec{B}\) due to the current must be cylindrically symmetrical. Thus, along the Amperian loop of radius r(r < a), symmetry suggests that \(\vec{B}\) is tangent to the loop, as shown in the figure.
\(\oint \vec{B} \cdot \overrightarrow{d l}=B \oint d l\) = B(2πr) ……….. (1)
Because the current is uniformly distributed, the current I_encl enclosed by the loop is proportional to the area encircled by the loop; that is,
I_encl = Jπr²
By right-hand rule, the sign of ‘d is positive. Then, by Ampere’s law,
B (2πr) = µ₀ I_encl = µ₀ Jπr² ……………….. (2)
∴ B = \(\frac{\mu_{0} J}{2} r\) ……………… (3)
OR
I_encl = I\(\frac{\pi r^{2}}{\pi a^{2}}\)
By right-hand rule, the sign of I\frac{\pi r^{2}}{\pi a^{2}} is positive. Then, by Ampere’s law,
\(\oint B d l\) = µ₀ I_encl
∴ B(2πr) = µ₀I \(\frac{r^{2}}{a^{2}}\) …………. (4)
∴ B = (\(\frac{\mu_{0} I}{2 \pi a^{2}}\) )r ………… (5)

[Note: Thus, inside the wire, the magnitude B of the magnetic field is proportional to distance r from the centre. At a distance r outside a straight wire, B = (\(\frac{\mu_{0} I}{2 \pi r}\) i.e., B ∝ \(\frac{1}{r}\).]

Theory Exercise

Question 1.
Distinguish between the forces experienced by a moving charge in a uniform electric field and in a uniform magnetic field.
Answer:
A charge q moving with a velocity \(\vec{v}\) through a magnetic field of induction \(\vec{B}\) experiences a magnetic force perpendicular both to \(\vec{B}\) and \(\vec{v}\) . Experimental observations show that the magnitude of the force is proportional to the magnitude of \(\vec{B}\), the speed of the particle, the charge q and the sine of the angle θ between \(\vec{v}\) and \(\vec{B}\). That is, the magnetic force, F_m = qv B sin θ
∴ \(\vec{F}_{\mathrm{m}}=q(\vec{v} \times \vec{B})\)
Therefore, at every instant \(\vec{F}_{\mathrm{m}}\) acts in a direction perpendicular to the plane of \(\vec{v}\) and \(\vec{B}\) .

If the moving charge is negative, the direction of the force \(\vec{F}_{\mathrm{m}}\) acting on it is opposite to that given by the right-handed screw rule for the cross-product \(\vec{v}\) × \(\vec{B}\).

If the charged particle moves through a region of space where both electric and magnetic fields are present, both fields exert forces on the particle.
The force due to the electric field \(\vec{E}\) is \(\vec{F}_{\mathrm{e}}=q \vec{E}\) .
The total force on a moving charge in electric and magnetic fields is called the Lorentz force :
\(\vec{F}=\vec{F}_{\mathrm{e}}+\vec{F}_{\mathrm{m}}=q(\vec{E}+\vec{v} \times \vec{B})\)
Special cases :
(i) \(\vec{v}\) is parallel or antiparallel to \(\vec{B}\): In this case, F_m = qvB sin 0° = 0. That is, the magnetic force on the charge is zero.
(ii) The charge is stationary {v = 0) : In this case, even if q ≠ 0 and B ≠ 0, F_m = q(0)B sin θ = 0. That is, the magnetic force on a stationary charge is zero.

Question 2.
Under what condition a charge undergoes uniform circular motion in a magnetic field? Describe, with a neat diagram, cyclotron as an application of this principle. Obtain an expression for the frequency of revolution in terms of the specific charge and magnetic field.
Answer:
Suppose a particle of mass m and charge q enters a region of uniform magnetic field of induction \(\vec{B}\). In Fig., \(\vec{B}\) points into the page. The magnetic force \(\vec{F}_{\mathrm{m}}\) on the particle is always perpendicular to the velocity of the particle, \(\vec{v}\) . Assuming the charged particle started moving in a plane perpendicular to \(\vec{B}\), its motion in the magnetic field is a uniform circular motion, with the magnetic force providing the centripetal acceleration.

If the charge moves in a circle of radius R,
F_m = |q|vB = \(\frac{m v^{2}}{R}\)
∴ mv = p = |q|BR …………. (1)
where p = mv is the linear momentum of the particle. Equation (1) is known as the cyclotron formula because it describes the motion of a particle in a cyclotron-the first of the modern particle accelerators.

Question 3.
What is special about a radial magnetic field ? Why is it useful in a moving coil galvanometer ?
Answer:

1. Advantage of radial magnetic field in a moving- coil galvanometer:
   • As the coil rotates, its plane is always parallel to the field. That way, the deflecting torque is always a ximum depending only on the current in the coil, but not on the position of the coil.
   • The restoring torque is proportional to the deflection so that a radial field makes the deflection proportional to the current. The instrument then has a linear scale, i.e., the divisions of the scale are evenly spaced. This makes it particularly straight forward to calibrate and to read.
2. Producing radial magnetic field :
   • The pole pieces of the permanent magnet are made cylindrically concave, concentric with the axis of the coil.
   • A soft iron cylinder is centred between the pole pieces so that it forms a narrow cylindrical gap in which the sides of the coil can move. Together, they produce a radial magnetic, field; that is, the magnetic lines of force in the gap are along radii to the central axis.

Question 4.
State Biot-Savert law. Apply it to
(i) infinitely long current carrying conductor and (ii) a point on the axis of a current carrying circular loop.
Answer:
Consider a very short segment of length dl of a wire carrying a current I. The product I \(\overrightarrow{d l}\) is called a current element; the direction of the vector \(\overrightarrow{d l}\) is along the wire in the direction of the current.

Biot-Savart law (Laplace law) : The magnitude of the incremental magnetic induction \(\overrightarrow{d B}\) produced by a current element I \(\overrightarrow{d l}\) at a distance r from it is directly proportional to the magnitude I \(\overrightarrow{d l}\) of the current element, the sine of the angle between the current element Idl and the unit vector r directed from the current element toward the point in question, and inversely proportional to the square of the distance of the point from the current element; the magnetic induction is directed perpendicular to both I \(\overrightarrow{d l}\) and \(\hat{r}\) as per the cross product rule.

where \(\hat{\mathrm{r}}=\frac{\vec{r}}{r}\) and the constant µ₀ is the permeability of free space. Equations (1) and (2) are called Biot-Savart law.

The incremental magnetic induction \(\overrightarrow{d B}\) is given by the right-handed screw rule of vector crossproduct \(I \overrightarrow{d l} \times \hat{\mathrm{r}}\). In Fig, the current element I\(\overrightarrow{d l}\) and \(\hat{\mathbf{r}}\) are in the plane of the page, so that \(\overrightarrow{d B}\) points out of the page at point P shown by ⊙; at the point Q, \(\overrightarrow{d B}\) points into the page shown by ⊗.

The magnetic induction \(\vec{B}\) at the point due to the entire wire is, by the principle of superposition, the vector sum of the contributions \(\overrightarrow{d B}\) from all the current elements making up the wire.
From Eq. (2),
\(\vec{B}=\int \overrightarrow{d B}=\frac{\mu_{0}}{4 \pi} \int \frac{I \overrightarrow{d l} \times \hat{\mathrm{r}}}{r^{2}}\)
[Notes : (1) The above law is based on experiments by Jean Baptiste Biot (1774-1862) and Felix Savart (1791-1841), French physicists. From their observations Laplace deduced the law mathematically. (2) The Biot- Savart law plays a similar role in magnetostatics as Coulomb’s law does in electrostatics.]

Question 5.
State Ampere’s law. Explain how is it useful in different situations.
Answer:
Ampere’s circuital law : In free space, the line integral of magnetic induction around a closed path in a magnetic field is equal to p0 times the net steady current enclosed by the path.
In mathematical form,
\(\oint \vec{B} \cdot \overrightarrow{d l}\) = μ₀ I …………… (1)
where \(\vec{B}\) is the magnetic induction at any point on the path in vacuum, \(\overrightarrow{d l}\) is the length element of the path, I is the net steady current enclosed and μ₀ is the permeability of free space.

Explanation : Figure shows two wires carrying currents I₁ and I₂ in vacuum. The magnetic induction \(\vec{B}\) at any point is the net effect of these currents.

To find the magnitude B of the magnetic induction :
We construct an imaginary closed curve around the conductors, called an Amperian loop, and imagine it divided into small elements of length \(\overrightarrow{d l}\). The direction of dl is the direction along which the loop is traced.

(ii) We assign signs to the currents using the right hand rule : If the fingers of the right hand are curled in the direction in which the loop is traced, then a current in the direction of the outstretched thumb is taken to be positive while a current in the opposite direction is taken to be negative.

For each length element of the Amperian loop, \(\vec{B} \cdot \overrightarrow{d l}\) gives the product of the length dl of the element and the component of \(\vec{B}\) parallel to \(\overrightarrow{d l}\) . If θ r is the angle between \(\overrightarrow{d l}\) and \(\vec{B}\) ,
\(\vec{B} \cdot \overrightarrow{d l}\) = (B cos θ) dl
Then, the line integral,
\(\oint \vec{B} \cdot \overrightarrow{d l}=\oint B \cos \theta d l\) …………. (2)
For the case shown in Fig., the net current I through the surface bounded by the loop is
I = I₂ – I₁
∴ \(\oint B \cos \theta d l\) = μ₀ I
= μ₀(I₂ – I₁) …………… (3)
Equation (3) can be solved only when B is uniform and hence can be taken out of the integral.

[Note : Ampere’s law in magnetostatics plays the part of Gauss’s law of electrostatics. In particular, for currents with appropriate symmetry, Ampere’s law in integral form offers an efficient way of calculating the magnetic field. Like Gauss’s law, Ampere’s law is always true (for steady currents), but it is useful only when the symmetry of the problem enables B to be taken out of the integral \(\oint \vec{B} \cdot \overrightarrow{d l}\). The current configurations that can be handled by Ampere’s law are infinite straight conductor, infinite plane, infinite solenoid and toroid.]

12th Physics Digest Chapter 10 Magnetic Fields due to Electric Current Intext Questions and Answers

Do you know (Textbook Page No. 230)

Question 1.
You must have noticed high tension power transmission lines, the power lines on the big tall steel towers. Strong magnetic fields are created by these lines. Care has to be taken to reduce the exposure levels to less than 0.5 milligauss (mG).
Answer:
With increasing population, many houses are constructed near high voltage overhead power transmission lines, if not right below them. Large transmission lines configurations with high voltage and current levels generate electric and magnetic fields and raises concerns about their effects on humans located at ground surfaces. With conductors typically 20 m above the ground, the electric field 2 m above the ground is about 0.2 kV / m to I kV / m. In comparison, that due to thunderstorms can reach 20 kV/m. For the same conductors, magnetic field 2 m above the ground is less than 6 μT. In comparison, that due to the Earth is about 40 μT.

Do you know (Textbook Page No. 232)

Question 1.
Magnetic Resonance Imaging (MRI) technique used for medical imaging requires a magnetic field with a strength of 1.5 T and even upto 7 T. Nuclear Magnetic Resonance experiments require a magnetic field upto 14 T. Such high magnetic fields can be produced using superconducting coil electromagnet. On the other hand, Earth’s magnetic field on the surface of the Earth is about 3.6 × 10^-5 T = 0.36 gauss.
Answer:
Magnetic Resonance Imaging (MRI) is a non-invasive imaging technology that produces three dimensional detailed anatomical images. Although MRI does not emit the ionizing radiation that is found in X-ray imaging, it does employ a strong magnetic field, e.g., medical MRIs usually have strengths between 1.5 T and 3 T.

The 21.1 T superconducting magnet at Maglab (Florida, US) is the world’s strongest MRI scanner used for Nuclear Magnetic Resonance (NMR) research. Since its inception in 2004, it has been continually conducting electric current of 284 A by itself. Because it is superconducting, the current runs through some 152 km of wire without resistance, so no outside energy source is needed. However, 2400 litres of liquid helium is cycled to keep the magnet at a superconducting temperature of 1.7 K. Even when not in use this magnet is kept cold; if it warms up to room temperature, it takes at least six weeks to cool it back down to operating temperature. The 45 T Hybrid Magnet of the Lab (which combines a superconducting magnet of 11.5 T with a resistive magnet of 33.5 T) is kept at 1.8 K using 2800 L of liquid helium and 15142 L of cold water.

Question 2.
Let us look at a charged particle which is moving in a circle with a constant speed. This is uniform circular motion that you have studied earlier. Thus, there must be a net force acting on the particle, directed towards the centre of the circle. As the speed is constant, the force also must be constant, always perpendicular to the velocity of the particle at any given instant of time. Such a force is provided by the uniform magnetic field \(\vec{B}\) perpendicular to the plane of the circle along which the charged particle moves.
Answer:
When a charged particle moves in uniform circular motion inside a uniform magnetic field \(\vec{B}\) in a plane perpendicular to \(\vec{B}\), the centripetal force is the magnetic force on the particle. As in any UCM, this magnetic force is constant in magnitude and perpendicular to the velocity of the particle.

Remember this (Textbook Page No. 233)

Question 1.
Field penetrating into the paper is represented as ⊗, while that coming out of the paper is shown by ⊙.
Answer:
In a two-dimensional diagram, a vector pointing perpendicularly into the plane of the diagram is shown by a cross ⊗ while that pointing out of the plane is shown by a dot ⊙ .

Do you know (Textbook Page No. 234)

Question 1.
Particle accelerators are important for a variety of research purposes. Large accelerators are used in particle research. There have been several accelerators in India since 1953. The Department of Atomic Energy (DAE), Govt. of India, had taken initiative in setting up accelerators for research. Apart from ion accelerators, the DAE has developed and commissioned a 2 GeV electron accelerator which is a radiation source for research in science. This accelerator, ‘Synchrotron’, is fully functional at Raja Ramanna Centre for Advanced Technology, Indore. An electron accelerator, Microtron with electron energy 8-10 MeV is functioning at Physics Department, Savitribai Phule Pune University, Pune.
Answer:
Particle accelerators are machines that accelerate charged subatomic particles to high energy for research and applications. They play a major role in the field of basic and applied sciences, in our understanding of nature and the universe. The size and cost of particle accelerators increase with the energy of the particles they produce. Medical Cyclotrons across the country are dedicated for medical isotope productions and for medical sciences. There are many existing and upcoming particle accelerators in India in different parts of country.

(https: / / www.researchgate.net/ publication/ 3209480 83_Existing and upcoming_particle_accelerators_in. India). For cutting-edge high energy particle physics, Indian particle physicists collaborate with those at Large Hadron Collider CERN, Geneva.

Can you recall (Textbook Page No. 238)

Question 1.
How does the coil in a motor rotate by a full rotation? In a motor, we require continuous rotation of the current carrying coil. As the plane of the coil tends to become parallel to the magnetic field \(\vec{B}\), the current in the coil is reversed externally. Referring to Fig. the segment ab occupies the position cd. At this position of rotation, the current is reversed. Instead of from b to a, it flows from a to b, force \(\vec{F}_{\mathrm{m}}\) continues to act in the same direction so that the torque continues to rotate the coil. The reversal of the current is achieved by using a commutator which connects the wires of the power supply to the coil via carbon brush contacts.
Answer:
Electric Motor
From Fig. we see that the torque on a current loop rotates the loop to smaller values of 9 until the torque becomes zero, when the plane of the loop is perpendicular to the magnetic field and θ = 0. If the current in the loop remains in the same direction when the loop turns past this position, the torque will reverse direction and turn the loop in the opposite direction, i.e., anticlockwise. To provide continuous rotation in the same sense, the current in the loop must periodically reverse direction, as shown in Fig.

In an electric motor, the current reversal is achieved externally by brushes and a split-ring commutator.

Use your brain power (Textbook Page No. 242)

Question 1.
Currents in two infinitely long, parallel wires exert forces on each other. Is this consistent with Newton’s third law?
Answer:
Yes, they are equal in magnitude and opposite in direction and act on the contrary parts : \(\vec{F}\)_{on 2 by 1} = \(\vec{F}\)_{on 1 by 2}. Thus, they form action-reaction pair.

Do you know (Textbook Page No. 244)

Question 1.
So far we have used the constant µ₀ everywhere. This means in each such case, we have carried out the evaluation in free space (vacuum). µ₀ is the permeability of free space.
Answer:
Permeability of free space or vacuum, µ₀ = 4π × 10^-7 H/m.
Earlier SI (2006) had fixed this value of µ₀ as exact but revised SI fixes the value of e, requiring µ₀ (and ε₀) to be determined experimentally.

Use your brain power (Textbook Page No. 244)

Question 1.
Using electrostatic analogue, obtain the magnetic field \(\vec{B}\)_equator at a distance d on the perpendicular bisector of a magnetic dipole of magnetic length 2l and moment \(\vec{M}\). For far field, verify that
\(\vec{B}_{\text {equator }}=\left(\frac{\mu_{0}}{4 \pi}\right) \frac{-\vec{M}}{\left(d^{2}+l^{2}\right)^{3 / 2}}\)
Answer:
The magnitude of the electric intensity at a point at a distance r from an electric charge q in vacuum is given by
E = \(\frac{1}{4 \pi \varepsilon_{0}} \frac{|q|}{r^{2}}\)
where ε₀ is the permittivity of free space. This intensity is directed away from the charge, if the charge is positive and towards the charge, if the charge is negative.

A magnetic pole is similar to an electric charge. The N-pole is similar to a positive charge and the S-pole is similar to a negative charge. Like an electric charge, a magnetic pole is assumed to produce a magnetic field in the surrounding region. The magnetic field at any point is denoted by a vector quantity called magnetic induction. Thus, by analogy, the magnitude of the magnetic induction at a point at a distance r from a magnetic pole of strength q_m is given by
B = \(\frac{\mu_{0}}{4 \pi} \frac{q_{\mathrm{m}}}{r^{2}}\)
This induction is directed away from the pole if it is an N-pole (strength + q_m) and towards the pole if it is an S-pole (strength -q_m).

Consider a point P on the equator of a magnetic dipole with pole strengths + q_m and – q_m and of magnetic length 21. Let P be at a distance d from the centre of the dipole,

The magnetic induction of a bar magnet at an equatorial point

The magnetic induction at P due to the N-pole is directed along NP (away from the N-pole) while that due to the S-pole is along PS (towards the S-pole), each having a magnitude
B_N = B_S = \(\left(\frac{\mu_{0}}{4 \pi}\right) \frac{q_{\mathrm{m}}}{\left(d^{2}+l^{2}\right)}\)
(∵ NP = SP = \(\sqrt{d^{2}+l^{2}}\))
The inductions due to the two poles are equal in magnitude so that the two, oppositely directed equatorial components, B_N sin θ and B_S sin θ, cancel each other.

Therefore, the resultant induction is in a direction’ parallel to the axis of the magnetic dipole and has direction opposite to that of the magnetic moment of the magnetic dipole. The component of the induction due to the two poles along the axis is
\(\left(\frac{\mu_{0}}{4 \pi}\right) \frac{q_{\mathrm{m}}}{\left(d^{2}+l^{2}\right)}\) cos θ
where θ is the angle shown in the diagram. From the diagram,

Thus, for a short dipole the induction varies in-versely as the cube of the distance from it.

Question 2.
What is the fundamental difference between an electric dipole and a magnetic dipole?
Answer:
If a magnet is carefully and repeatedly cut, it would expose two new faces with opposite poles such that each piece would still be a magnet. This suggests that magnetic fields are essentially dipolar in character. The most elementary magnetic structure always behaves as a pair of two magnetic poles of opposite types and of equal strengths. Hence, analogous to an electric dipole, we hypothesize that there are positive and negative magnetic charges (or north and south poles) of equal strengths a finite distance apart within a magnet. Also, they are assumed to act as the source of the magnetic field in exactly the same way that electric charges act as the source of electric field. The magnitude of each ‘magnetic charge’ is referred to as its ‘pole strength’ and is equal to q_m = \(\frac{M}{2 l}\), where \(\vec{M}\) is the magnetic dipole moment, pointing from the negative (or south, S) pole to the positive (or north, N) pole.

However, while two types of electric charges exist in nature and have separate existence, isolated magnetic charges, or magnetic monopoles, are not observed. A magnetic pole is not an experimental fact: there are no real poles. To put it in another way, there are no point sources for \(\vec{B}\), as there are for \(\vec{E}\); there exists no magnetic analog to electric charge. Every experimental effort to demonstrate the existence of magnetic charges has failed. Hence, magnetic poles are called fictitious.

The electric field diverges away from a (positive) charge; the magnetic field line curls around a current. Electric field lines originate on positive charges and terminate on negative ones; magnetic field lines do not begin or end anywhere, they typically form closed loops or extend out to infinity.

Do you know (Textbook Page No. 247)

Question 1.
What is an ideal solenoid?
Answer:
A solenoid is a long wire wound in the form of a helix. An ideal solenoid is tightly wound and infinitely long, i.e., its turns are closely spaced and the solenoid is very long compared to its crosssectional radius.

Each turn of a solenoid acts approximately as a circular loop. Suppose the solenoid carries a steady current I. The net magnetic field due to the current in the solenoid is the vector sum of the fields due to the current in all the turns. In the case of a tightly- wound solenoid of finite length, Fig. 10.39, the magnetic field lines are approximately parallel only near the centre of the solenoid, indicating a nearly uniform field there. However, close to the ends, the field lines diverge from one end and converge at the other end. This field distribution is similar to that of a bar magnet. Thus, one end of the solenoid behaves like the north pole of a magnet and the opposite end behaves like the south pole. The field outside is very weak near the midpoint.

For an ideal solenoid, the magnetic field inside is reasonably uniform over the cross section and parallel to the axis throughout the volume enclosed by the solenoid. The field outside is negligible in this case.

Use your brain power (Textbook Page No. 248)

Question 1.
Choosing different Amperean loops, show that out-side an ideal toroid B = 0.
Answer:
From below figure, the inner Amperean loop does not enclose any current while the outer Amperean loop encloses equal number of I_in and I_out. Hence, by Ampere’s law, B = 0 outside an ideal toroid.

Question 2.
What is an ideal toroid?
Answer:
A toroid is a toroidal solenoid. An ideal toroid consists of a long conducting wire wound tightly around a torus, a doughnut-shaped ring, made of a nonconducting material.

In an ideal toroid carrying a steady current, the magnetic field in the interior of the toroid is tangential to any circle concentric with the axis of the toroid and has the same value on this circle (the dashed line in figure). Also, the magnitude of the magnetic induction external to the toroid is negligible.

Balbharti Maharashtra State Board 12th Physics Textbook Solutions Chapter 6 Superposition of Waves Textbook Exercise Questions and Answers.

Maharashtra State Board 12th Physics Solutions Chapter 6 Superposition of Waves

1. Choose the correct option.

i) When an air column in a pipe closed at one end vibrates such that three nodes are formed in it, the frequency of its
vibrations is …….times the fundamental frequency.
(A) 2
(B) 3
(C) 4
(D) 5
Answer:
(D) 5

ii) If two open organ pipes of length 50 cm and 51 cm sounded together produce 7 beats per second, the speed of sound is.
(A) 307 m/s
(B) 327m/s
(C) 350m/s
(D) 357m/s
Answer:
(D) 357m/s

iii) The tension in a piano wire is increased by 25%. Its frequency becomes ….. times the original frequency.
(A) 0.8
(B) 1.12
(C) 1.25
(D) 1.56
Answer:
(B) 1.12

iv) Which of the following equations represents a wave travelling along the y-axis?
(A) x = A sin(ky – ωt)
(B) y = A sin(kx – ωt)
(C) y = A sin(ky) cos(ωt)
(D) y = A cos(ky)sin(ωt)
Answer:
(A) x = A sin(ky – ωt)

v) A standing wave is produced on a string fixed at one end with the other end free. The length of the string
(A) must be an odd integral multiple of λ/4.
(B) must be an odd integral multiple of λ/2.
(C) must be an odd integral multiple of λ.
(D) must be an even integral multiple ofλ.
Answer:
(A) must be an odd integral multiple of λ/4.

2. Answer in brief.

i) A wave is represented by an equation y = A sin (Bx + Ct). Given that the constants A, B and C are positive, can you tell in which direction the wave is moving?
Answer:
The wave is travelling along the negative x-direction.

ii) A string is fixed at the two ends and is vibrating in its fundamental mode. It is known that the two ends will be at rest. Apart from these, is there any position on the string which can be touched so as not to disturb the motion of the string? What will be the answer to this question if the string is vibrating in its first and second overtones?
Answer:
Nodes are the points where the vibrating string can be touched without disturbing its motion.
When the string vibrates in its fundamental mode, the string vibrates in one loop. There are no nodes formed between the fixed ends. Hence, there are no point on the string which can be touched without disturbing its motion.

When the string vibrates in its first overtone (second harmonic), there are two loops of the stationary wave on the string. Apart from the two nodes at the two ends, there is now a third node at its centre. Hence, the string can be touched at its centre without disturbing the stationary wave pattern.

When the string vibrates in its second overtone (third harmonic), there are three loops of the stationary wave on the string. So, apart from the two end nodes, there are two additional nodes in between, at distances one-third of the string length from each end. Thus, now the string can be touched at these two nodes.

iii) What are harmonics and overtones?
Answer:
A stationary wave is set up in a bounded medium in which the boundary could be a rigid support (i.e., a fixed end, as for instance a string stretched between two rigid supports) or a free end (as for instance an air column in a cylindrical tube with one or both ends open). The boundary conditions limit the possible stationary waves and only a discrete set of frequencies is allowed.

The lowest allowed frequency, n₁, is called the fundamental frequency of vibration. Integral multiples of the fundamental frequency are called the harmonics, the fundamental frequency being the fundamental or 2n₁, the third harmonic is 3n₁, and so on.

The higher allowed frequencies are called the overtones. Above the fundamental, the first allowed frequency is called the first overtone, the next higher frequency is the second overtone, and ‘so on. The relation between overtones and allowed harmonics depends on the system under consideration.

iv) For a stationary wave set up in a string having both ends fixed, what is the ratio of the fundamental frequency to the
second harmonic?
Answer:
The fundamental is the first harmonic. Therefore, the ratio of the fundamental frequency (n) to the second harmonic (n₁) is 1 : 2.

v) The amplitude of a wave is represented by y = 0.2 sin 4π[\(\frac{t}{0.08}\) – \(\frac{x}{0.8}\)] in SI units. Find
(a) wavelength,
(b) frequency and
(c) amplitude of the wave. [(a) 0.4 m (b) 25 Hz (c) 0.2 m]
Answer:

y = 0.2 sin 2π[\(\frac{t}{0.04}\) – \(\frac{x}{0.4}\)]
Let us compare above equation with the equation of a simple harmonic progressive wave:
y = A sin 2π[\(\frac{t}{T}\) – \(\frac{x}{\lambda}\)] = 0.2 sin 2π[\(\frac{t}{0.04}\) – \(\frac{x}{0.4}\)]
Comparing the quantities on both sides, we get,
A = 0.2 m, T = 0.04 s, λ = 0.4 m
∴ (a) Wavelength (λ) = 0.4 m
(b) Frequency (n) = \(\frac{1}{T}\) = \(\frac{1}{0.04}\) = 25 Hz
(c) Amplitude (A) = 0.2 m

Question 3.
State the characteristics of progressive waves.
Answer:
Characteristics of a progressive wave :

1. Energy is transmitted from particle to particle without the physical transfer of matter.
2. The particles of the medium vibrate periodically about their equilibrium positions.
3. In the absence of dissipative forces, every particle vibrates with the same amplitude and frequency, but differs in phase from its adjacent particles. Every particle lags behind in its state of motion compared to the one before it.
4. A wave motion is doubly periodic, i.e., it is periodic in time and periodic in space.
5. The velocity of propagation through a medium depends upon the properties of the medium.
6. Progressive waves are of two types : transverse and longitudinal. In a transverse mechanical wave, the individual particles of the medium vibrate perpendicular to the direction of propagation of the wave. The progressively changing phase of the successive particles results in the formation of alternate crests and troughs that are periodic in space and time. In an em wave, the electric and magnetic fields oscillate in mutually perpendicular directions, perpendicular to the direction of propagation.
   In a longitudinal mechanical wave, the individual particles of the medium vibrate along the line of propagation of the wave. The progressively changing phase of the successive particles results in the formation of typical alternate regions of compressions and rarefactions that are periodic in space and time. Periodic compressions and rarefactions result in periodic pressure and density variations in the medium. There are no longitudinal em wave.
7. A transverse wave can propagate only through solids, but not through liquids and gases while a longitudinal wave can propagate through any material medium.

Question 4.
State the characteristics of stationary waves.
Answer:
Characteristics of stationary waves :

1. Stationary waves are produced by the interference of two identical progressive waves travelling in opposite directions, under certain conditions.
2. The overall appearance of a standing wave is of alternate intensity maximum (displacement antinode) and minimum (displacement node).
3. The distance between adjacent nodes (or antinodes) is λ/2.
4. The distance between successive node and antinode is λ/4.
5. There is no progressive change of phase from particle to particle. All the particles in one loop, between two adjacent nodes, vibrate in the same phase, while the particles in adjacent loops are in opposite phase.
6. A stationary wave does not propagate in any direction and hence does not transport energy through the medium.
7. In a region where a stationary wave is formed, the particles of the medium (except at the nodes) perform SHM of the same period, but the amplitudes of the vibrations vary periodically in space from particle to particle.

[Note : Since the nodes are points where the particles are always at rest, energy cannot be transmitted across a node. The energy of the particles within a loop remains localized, but alternates twice between kinetic and potential energy during each complete vibration. When all the particles are in the mean position, the energy is entirely kinetic. When they are in their extreme positions, the energy is entirely potential.]

Question 5.
Derive an expression for equation of stationary wave on a stretched string.
Answer:
When two progressive waves having the same amplitude, wavelength and speed propagate in opposite directions through the same region of a medium, their superposition under certain conditions creates a stationary interference pattern called a stationary wave.

Consider two simple harmonic progressive waves, of the same amplitude A, wavelength A and frequency n = ω/2π, travelling on a string stretched along the x-axis in opposite directions. They may be represented by
y₁ = A sin (ωt – kx) (along the + x-axis) and … (1)
y₂ = A sin (ωt + kx) (along the – x-axis) …. (2)
where k = 2π/λ is the propagation constant.

By the superposition principle, the resultant displacement of the particle of the medium at the point at which the two waves arrive simultaneously is the algebraic sum
y = y₁ + y₂ = A [sin (ωt – kx) + sin (ωt + kx)]
Using the trigonometrical identity,
sin C + sin D = 2 sin \(\left(\frac{C+D}{2}\right)\) cos \(\left(\frac{C-D}{2}\right)\),
y = 2A sin ωt cos (- kx)
= 2A sin ωt cos kx [∵ cos(- kx) = cos(kx)]
= 2A cos kx sin ωt … (3)
∴ y = R sin ωt, … (4)
where R = 2A cos kx. … (5)
Equation (4) is the equation of a stationary wave.

Question 6.
Find the amplitude of the resultant wave produced due to interference of two waves given as y₁ = A₁ sin ωt y₂ = A₂ sin (ωt + φ)
Answer:
The amplitude of the resultant wave produced due to the interference of the two waves is
A = \(\sqrt{A_{1}^{2}+2 A_{1} A_{2} \cos \varphi+A_{2}^{2}}\).

Question 7.
State the laws of vibrating strings and explain how they can be verified using a sonometer.
Answer:
The fundamental is the first harmonic. Therefore, the ratio of the fundamental frequency (n) to the second harmonic (n₁) is 1 : 2.

Here, L = 3\(\frac{\lambda}{2}\)
∴ Wavelength, λ = \(\frac{2 L}{3}\) = \(\frac{2 \times 30}{3}\) = 20 cm.

Question 8.
Show that only odd harmonics are present in the vibrations of air column in a pipe closed at one end.
Answer:
Consider a narrow cylindrical pipe of length l closed at one end. When sound waves are sent down the air column in a cylindrical pipe closed at one end, they are reflected at the closed end with a phase reversal and at the open end without phase reversal. Interference between the incident and reflected waves under appropriate conditions sets up stationary waves in the air column.

The stationary waves in the air column in this case are subject to two boundary conditions that there must be a node at the closed end and an antinode at the open end.

Taking into account the end correction e at the open end, the resonating length of the air column is L = l + e.

Let v be the speed of sound in air. In the simplest mode of vibration, there is a node at the closed end and an antinode at the open end. The distance between a node and a consecutive anti-node is \(\frac{\lambda}{4}\), where λ is the wavelength of sound. The corresponding wavelength λ and frequency n are
λ = 4L and n = \(\frac{v}{\lambda}\) = \(\frac{v}{4 L}\) = \(\frac{v}{4(l+e)}\) …… (1)
This gives the fundamental frequency of vibration and the mode of vibration is called the fundamental mode or first harmonic.

In the next higher mode of vibration, the first overtone, two nodes and two antinodes are formed. The corresponding wavelength λ₁ and frequency n₁ are
λ₁ = \(\frac{4 L}{3}\) and n₁ = \(\frac{v}{\lambda_{1}}\) = \(\frac{3 v}{4 L}\) = \(\frac{3 v}{4(l+e)}\) = 3n … (2)
Therefore, the frequency in the first overtone is three times the fundamental frequency, i.e., the first overtone is the third harmonic.

In the second overtone, three nodes and three antinodes are formed. The corresponding wavelength λ₂ and frequency n₂ are

which is the fifth harmonic.
Therefore, in general, the frequency of the pth overtone (p = 1, 2, 3, ,..) is
n_p = (2p + 1)n … (4)
i.e., the pth overtone is the (2p + 1)th harmonic.

Equations (1), (2) and (3) show that allowed frequencies in an air column in a pipe closed at one end are n, 3n, 5n, …. That is, only odd harmonics are present as overtones.

Question 9.
Prove that all harmonics are present in the vibrations of the air column in a pipe open at both ends.
Answer:
Consider a cylindrical pipe of length l open at both the ends. When sound waves are sent down the air column in a cylindrical open pipe, they are reflected at the open ends without a change of phase. Interference between the incident and reflected waves under appropriate conditions sets up stationary waves in the air column.

The stationary waves in the air column in this case are subject to the two boundary conditions that there must be an antinode at each open end.

Taking into account the end correction e at each of the open ends, the resonating length of the air column is L = l + 2e.

Let v be the speed of sound in air. In the simplest mode of vibration, the fundamental mode or first harmonic, there is a node midway between the two antinodes at the open ends. The distance between two consecutive antinodes is λ/2, where λ is the wavelength of sound. The corresponding wavelength λ and the fundamental frequency n are
λ = 2L and n = \(\frac{v}{\lambda}\) = \(\frac{v}{2 L}\) = \(\frac{v}{2(l+2 e)}\) …. (1)

In the next higher mode, the first overtone, there are two nodes and three antinodes. The corresponding wavelength λ₁ and frequency n₁
λ₁ = L and n₁ = \(\frac{v}{\lambda_{1}}\) = \(\frac{v}{L}\) = \(\frac{v}{(l+2 e)}\) = 2n …. (2)
i.e., twice the fundamental. Therefore, the first overtone is the second harmonic.

In the second overtone, there are three nodes and four antinodes. The corresponding wavelength λ₂ and frequency n₂ are
λ₂ = \(\frac{2 L}{3}\) and n₂ = \(\frac{v}{\lambda_{2}}\) = \(\frac{3v}{2L}\) = \(\frac{3 v}{2(l+2 e)}\) = 3n …. (3)
or thrice the fundamental. Therefore, the second overtone is the third harmonic.

Therefore, in general, the frequency of the pth overtone (p = 1, 2, 3, …) is
n_p = (p + 1)n … (4)
i.e., the pth overtone is the (p + 1)th harmonic.
Equations (1), (2) and (3) show that allowed frequencies in an air column in a pipe open at both ends are n, 2n, 3n, …. That is, all the harmonics are present as overtones.

Question 10.
A wave of frequency 500 Hz is travelling with a speed of 350 m/s.
(a) What is the phase difference between two displacements at a certain point at times 1.0 ms apart?
(b) what will be the smallest distance between two points which are 45º out of phase at an instant of time?
[Ans : π, 8.75 cm ]
Answer:
Data : n = 500 Hz, v = 350 m/s
D = n × λ
∴ λ = \(\frac{350}{500}\) = 0.7 m
(a) in t = 1.0 ms = 0.001 s, the path difference is the distance covered v × t = 350 × 0.001 = 0.35 m
∴ Phase difference = \(\frac{2 \pi}{\lambda}\) × Path difference
= \(\frac{2 \pi}{0.7}\) × 0.35 = π rad

(b) Phase difference = 45° = \(\frac{\pi}{4}\) rad
∴ Path difference = \(\frac{\lambda}{2 \pi}\) × Phase difference
= \(\frac{0.7}{2 \pi}\) × \(\frac{\pi}{4}\) = 0.0875 m

Question 11.
A sound wave in a certain fluid medium is reflected at an obstacle to form a standing wave. The distance between two successive nodes is 3.75 cm. If the velocity of sound is 1500 m/s, find the frequency. [Ans : 20 kHz]
Answer:
Data : Distance between two successive nodes =
\(\frac{\lambda}{2}\) = 3.75 × 10^-2 m, v = 1500 m/s
∴ λ = 7.5 × 10^-2m
v = n × λ
∴ n = \(\frac{1500}{7.5 \times 10^{-2}}\) = 20 kHz

Question 12.
Two sources of sound are separated by a distance 4 m. They both emit sound with the same amplitude and frequency (330 Hz), but they are 180º out of phase. At what points between the two sources, will the sound intensity be maximum? (Take velocity of sound to be 330 m/s) [Ans: ± 0.25, ± 0.75, ± 1.25 and ± 1.75 m from the point at the center]
Answer:
∴ λ = \(\frac{v}{n}\) = \(\frac{330}{330}\) = 1 m
Directly at the cenre of two sources of sound, path difference is zero. But since the waves are 180° out of phase, two maxima on either sides should be at a distance of \(\frac{\lambda}{4}\) from the point at the centre. Other
maxima will be located each \(\frac{\lambda}{2}\) further along.
Thus, the sound intensity will be maximum at ± 0.25, ± 0.75, ± 1.25, ± 1.75 m from the point at the centre.

Question 13.
Two sound waves travel at a speed of 330 m/s. If their frequencies are also identical and are equal to 540 Hz, what will be the phase difference between the waves at points 3.5 m from one source and 3 m from the other if the sources are in phase? [Ans : 1.636 π]
Answer:
Data : v = 330 m/s, n₁ = n₂ = 540 Hz
v = n × λ
∴ λ = \(\frac{330}{540}\) = 0.61 m
Here, the path difference = 3.5 – 3 m = 0.5 m
Phase difference = \(\frac{2 \pi}{\lambda}\) × Path difference
= \(\frac{2 \pi}{0.61}\) × 0.5 = 1.64π rad

Question 14.
Two wires of the same material and same cross-section are stretched on a sonometer. One wire is loaded with 1.5 kg and another is loaded with 6 kg. The vibrating length of first wire is 60 cm and its fundamental frequency of vibration is the same as that of the second wire. Calculate vibrating length of the other wire. [Ans: 1.2 m]
Answer:
Data : m₁ = m₂ = m, L₁ = 60 cm = 0.6 m, T₁ = 1.5 kg = 14.7 N, T₂ = 6 kg = 58.8 N

The vibrating length of the second wire is 1.2 m.

Question 15.
A pipe closed at one end can produce overtones at frequencies 640 Hz, 896 Hz and 1152 Hz. Calculate the fundamental
frequency. [Ans: 128 Hz]
Answer:
The difference between the given frequencies of the overtones is 256 Hz. This implies that they are consecutive overtones. Let n_C be the fundamental frequency of the closed pipe and n_q, n_q-1, n_q-1 = the frequencies of the q^th, (q + 1)^th and (q + 2)^th consecutive overtones, where q is an integer.

Data : n_q = 640 Hz, n_q-1 = 896 Hz, n_q+2 = 1152 Hz
Since only odd harmonics are present as overtones, n_q = (2q +1) n_C
and n_q+1 = [2(q + 1) + 1] n_C = (2q + 3) n_C

∴ 14q + 7 = 10q + 15 ∴ 4q = 8 ∴ q = 2
Therefore, the three given frequencies correspond to the second, third and fourth overtones, i.e., the fifth, seventh and ninth harmonics, respectively.
∴ 5n_C = 640 ∴ b_C = 128Hz

Question 16.
A standing wave is produced in a tube open at both ends. The fundamental frequency is 300 Hz. What is the length
of tube in the fundamental mode? (speed of the sound = 340 m s^-1). [Ans: 0.5666 m]
Answer:
Data : For the tube open at both the ends, n = 300 Hz and v = 340 m / s Igonoring end correction, the fundamental frequency of the tube is
n = \(\frac{v}{2 L}\) ∴ L = \(\frac{v}{2 n}\) = \(\frac{340}{2 \times 300}\) = 0.566m
The length of the tube open at both the ends is 0.5667 m.

Question 17.
Find the fundamental, first overtone and second overtone frequencies of a pipe, open at both the ends, of length 25 cm if the speed of sound in air is 330 m/s. [Ans: 660 Hz, 1320 Hz, 1980 Hz]
Answer:
Data : Open pipe, ∠25 cm = 0.25 m, v = 330 m / s
The fundamental frequency of an open pipe ignoring end correction,

Since all harmonics are present as overtones, the first overtone is, n₁ = 2n₀ = 2 × 660 = 1320 Hz
The second overtone is n₂ = 3n = 3 × 660 = 1980 Hz

Question 18.
A pipe open at both the ends has a fundamental frequency of 600 Hz. The first overtone of a pipe closed at one end has the same frequency as the first overtone of the open pipe. How long are the two pipes? (Take velocity of sound to be 330 m/s) [Ans : 27.5 cm, 20.625 cm]
Answer:
Data : Open pipe, n_o = 600 Hz, n_{C, 1} = n_{o, 1} (first overtones)
For an open pipe, the fundamental frequency,
n_o = \(\frac{v}{2 L_{O}}\)
∴ The length of the open pipe is
L₀ = \(\frac{v}{2 n_{O}}\) = \(\frac{330}{2 \times 600}\) = 0.275 m
For the open pipe, the frequency of the first overtone is
2n₀ = 2 × 600 = 1200 Hz
For the pipe closed at one end, the frequency of the first overtone is \(\frac{3 v}{L_{O}}\).
By the data, \(\frac{3 v}{4 L}\) = 1200
∴ L_C = \(\frac{3 \times 330}{4 \times 1200}\) = 0.206 m
The length of the pipe open at both ends is 27.5 cm

Question 19.
A string 1m long is fixed at one end. Transverse vibrations of frequency 15 Hz are imposed at the free end. Due to this, a stationary wave with four complete loops, is produced on the string. Find the speed of the progressive wave which produces the stationary wave.[Hint: Remember that the free end is an antinode.] [Ans: 6.67 m s^-1]
Answer:
Data : L = 1 m, n = 15 Hz.
The string is fixed only at one end. Hence, an antinode will be formed at the free end. Thus, with four and half loops on the string, the length of the string is
L = \(\frac{\lambda}{4}\) + 4\(\left(\frac{\lambda}{2}\right)\) = \(\frac{9}{4}\)λ
∴ λ = \(\frac{4 L}{9}\) = \(\frac{4}{9}\) × 1 = \(\frac{4}{9}\) m
v = nλ
∴ Speed of the progressive wave
v = 15 × \(\frac{4}{9}\) = \(\frac{60}{9}\) =6.667m/s

Question 20.
A violin string vibrates with fundamental frequency of 440Hz. What are the frequencies of first and second overtones? [Ans: 880 Hz, 1320 Hz]
Answer:
Data: n =440Hz
The first overtone, n₁ = 2n =2 × 400 = 880 Hz
The second overtone, n₁ = 3n = 3 × 400 = 1320 Hz

Question 21.
A set of 8 tuning forks is arranged in a series of increasing order of frequencies. Each fork gives 4 beats per second with the next one and the frequency of last for k is twice that of the first. Calculate the frequencies of the first and the last for k. [Ans: 28 Hz, 56 Hz]
Answer:
Data : n₈ = 2n₁, beat frequency = 4 Hz
The set of tuning fork is arranged in the increasing order of their frequencies.
∴ n₂ = n₁ + 4
n₃ = n₂ + 4 = n₁ + 2 × 4
n₄ = n₃ + 4 = n₁ + 3 × 4
∴ n₈ = n₇ + 4 = n₁ + 7 × 4 = n₁ + 28
Since n₈ = 2n₁,
2n₁ = n₁ + 28
∴ The frequency of the first fork, n₁ = 28 Hz
∴ The frequency of the last fork,
n₈ = n₁ + 28 = 28 + 28 = 56 Hz

Question 22.
A sonometer wire is stretched by tension of 40 N. It vibrates in unison with a tuning fork of frequency 384 Hz. How
many numbers of beats get produced in two seconds if the tension in the wire is decreased by 1.24 N? [Ans: 12 beats]
Answer:
Data : T₁ =40N, n₁ = 384 Hz, T₂ = 40 – 1.24 = 38.76 N

∴ The number of beats produced in two seconds = 2 × 6 = 12

Question 23.
A sonometer wire of length 0.5 m is stretched by a weight of 5 kg. The fundamental frequency of vibration is 100 Hz. Calculate linear density of wire. [Ans: 4.9 × 10^-3 kg/m]
Answer:
Data : L = 0.5 m, T = 5 kg = 5 × 9.8 = 49 N, n = 100 Hz
n = \(\frac{1}{2 L} \sqrt{\frac{T}{m}}\)
∴ Linear density, m = \(\frac{T}{4 L^{2} n^{2}}\)
= \(\frac{49}{4(0.5)^{2}(100)^{2}}\)
= 4.9 × 10^-3 kg/m

Question 24.
The string of a guitar is 80 cm long and has a fundamental frequency of 112 Hz. If a guitarist wishes to produce a frequency
of 160 Hz, where should the person press the string? [Ans : 56 cm from one end]
Answer:
Data : L₁ = 80 cm n₁ = 112 Hz, n₂ = 160 Hz
According to the law of length, n₁L₁ = n₂L₂.
∴ The vibrating length to produce the fundamental frequency of 160 Hz,
L₂ = \(\frac{n_{1} L_{1}}{n_{2}}\) = \(\frac{112(80)}{160}\) = 56 cm

12th Physics Digest Chapter 6 Superposition of Waves Intext Questions and Answers

Can you tell? (Textbook Page No. 132)

Question 1.
What is the minimum distance between any two particles of a medium which always have the same speed when a sinusoidal wave travels through the medium ?
Answer:
When a sinusoidal wave travels through a medium the minimum distance between any two particles of the medium which always have the same speed is \(\frac{\lambda}{2}\).
Such particles are opposite in phase, i.e., their instantaneous velocities are opposite in direction.
[Note : The minimum distance between any two particles which have the same velocity is λ]

Do you know? (Textbook Page No. 140)

Question 1.
What happens if a simple pendulum is pulled aside and released ?
Answer:
If a simple pendulum is pulled aside and released, it oscillates freely about its equilibrium position at its natural frequency which is inversely proportional to the square root of its length and directly proportional to the square root of the acceleration of gravity at the place. These oscillations, called as free oscillations, are periodic and tautochronous if the displacement of its bob is small and the dissipative forces can be ignored.

Question 2.
What happens when a guitar string is plucked ?
Answer:
When a guitar string is plucked, two wave pulses of the same amplitude, frequency and phase move out from that point towards the fixed ends of the string where they get reflected. For certain ratios of wavelength to length of the string, these reflected pulses moving towards each other will meet in phase to form standing waves on the string. The vibrations of the string cause the air molecules to oscillate, forming sound waves that radiate away from the string. The frequency of the sound waves is equal to the frequency of the vibrating string. In general, the wavelengths of the sound waves and the waves on the string are different because their speeds in the two mediums are not the same.

Question 3.
Have you noticed vibrations in a drill machine or in a washing machine ? How do they differ from vibrations in the above two cases ?
Answer:
Vibrations in the body of a drill machine or that of a washing machine are forced vibrations induced by the vibrations of the motors of these machines. On the other hand, the oscillations of a simple pendulum or a guitar string are free oscillations, produced when they are disturbed from their equilibrium position and released.

Question 4.
A vibrating tuning fork of certain frequency is held in contact with a tabletop and its vibrations are noticed and then another vibrating tuning fork of different frequency is held on the tabletop. Are the vibrations produced in the tabletop the same for both the tuning forks ? Why ?
Answer:
No. Because the tuning forks have different frequencies, the forced vibrations in the tabletop differ both in frequency and amplitude. The tuning fork whose frequency is closer to a natural frequency of the tabletop induces forced vibrations of a larger amplitude.

Balbharti Maharashtra State Board 12th Physics Textbook Solutions Chapter 12 Electromagnetic Induction Textbook Exercise Questions and Answers.

Maharashtra State Board 12th Physics Solutions Chapter 12 Electromagnetic Induction

1. Choose the correct option.

i) A circular coil of 100 turns with a cross-sectional area (A) of 1 m² is kept with its plane perpendicular to the magnetic field (B) of 1 T. What is the magnetic flux linkage with the coil?
(A) 1 Wb
(B) 100 Wb
(C) 50 Wb
(D) 200 Wb
Answer:
(B) 100 Wb

ii) A conductor rod of length (l) is moving with velocity (v) in a direction normal to a uniform magnetic field (B). What will be the magnitude of induced emf produced between the ends of the moving conductor?
(A) BLv
(B) BLv²
(C) \(\frac{1}{2}\)Blv
(D) \(\frac{2 B l}{\mathrm{v}}\)
Answer:
(A) BLv

iii) Two inductor coils with inductance 10 mH and 20 mH are connected in series. What is the resultant inductance of the combination of the two coils?
(A) 20 mH
(B) 30 mH
(C) 10 mH
(D) \(\frac{20}{3}\) mH
Answer:
(A) 20 mH

iv) A current through a coil of self inductance 10 mH increases from 0 to 1 A in 0.1 s. What is the induced emf in the coil?
(A) 0.1 V
(B) 1 V
(C) 10 V
(D) 0.01 V
Answer:
(A) 0.1 V

v) What is the energy required to build up a current of 1 A in an inductor of 20 mH?
(A) 10 mJ
(B) 20 mJ
(C) 20 J
(D) 10 J
Answer:
(A) 10 mJ

2. Answer in brief.

i) What do you mean by electromagnetic induction? State Faraday’s law of induction.
Answer:
The phenomenon of production of emf in a conductor or circuit by a changing magnetic flux through the circuit is called electromagnetic induction.

Faraday’s laws of electromagnetic induction :
(1) First law ; Whenever there is a change in the magnetic flux associated with a circuit, an emf is induced in the circuit.
(2) Second law : The magnitude of the induced emf is directly proportional to the time rate of change of magnetic flux through the circuit.
[Note : The phenomenon was discovered in 1830 by Joseph Henry (1797-1878), US physicist, and independently in 1832 by Michael Faraday (1791 -1867), British chemchemist and physicist.]

ii) State and explain Lenz’s law in the light of principle of conservation of energy.
Answer:
Lenz’s law : The direction of the induced current is such as to oppose the change that produces it.

The change that induces a current may be (i) the motion of a conductor in a magnetic field or (ii) the change of the magnetic flux through a stationary circuit.

Explanation : Consider Faraday’s magnet-and- coil experiment. If the bar magnet is moved towards the coil with its N-pole facing the coil, as in Fig., the number of magnetic lines of induction (pointing to the left) through the coil increases. The induced current in the coil sets up a magnetic field of its own pointing to the right (as given by Amperes right-hand rule) to oppose the growing flux due to the magnet. Hence, to move the magnet towards the coil against this repulsive flux of the induced current, we must do work. The work done shows up as electric energy in the coil.

When the magnet is withdrawn, with its N-pole still facing the coil, the number of magnetic lines of induction (pointing left) through the coil decreases. The induced current reverses its direction to supplement the decreasing flux with its own, as shown in Fig.. Facing the coil along the magnet, the induced current is in the clockwise sense. The electric energy in the coil comes from the work done to withdraw the magnet, now against an attractive force. Thus, we see that Lenz’s law is a consequence of the law of conservation of energy.

[Note : The above law was discovered by Heinrich Friedrich Emil Lenz (1804-65), Russian physicist.]

iii) What are eddy currents? State applications of eddy currents.
Answer:
Whenever a conductor or a part of it is moved in a magnetic field “cutting” magnetic field lines, or placed in a changing magnetic field, the free electrons in the bulk of the metal start circulating in closed paths equivalent to current-carrying loops. These loop currents resemble eddies in a fluid stream and are hence called eddy or Foucault currents [after Jean Bernard Leon Foucault (1819-68), French physicist, who first detected them].

Applications :
(1) Dead-beat galvanometer : A pivoted moving-coil galvanometer used for measuring current has the coil wound on a light aluminium frame. The rotation of the metal frame in magnetic field produces eddy currents in the frame which opposes the rotation and the coil is brought to rest quickly. This makes the galvanometer dead-beat.

(2) Electric brakes : When a conducting plate is pushed into a magnetic field, or pulled out, very quickly, the interaction between the eddy currents in the moving conductor and the field retards the motion. This property of eddy currents is used as a method of braking in vehicles.

iv) If the copper disc of a pendulum swings between the poles of a magnet, the pendulum comes to rest very quickly. Explain the reason. What happens to the mechanical energy of the pendulum?
Answer:
As the copper disc enters and leaves the magnetic field, the changing magnetic flux through it induces eddy current in the disc. In both cases, Fleming’s right hand rule shows that opposing magnetic force damps the motion. After a few swings, the mechanical energy becomes zero and the motion comes to a stop.

Joule heating due to the eddy current warms up the disc. Thus, the mechanical energy of the pendulum is transformed into thermal energy.

v) Explain why the inductance of two coils connected in parallel is less than the inductance of either coil.
Answer:
Assuming that their mutual inductance can be ignored, the equivalent inductance of a parallel combination of two coils is given by
\(\frac{1}{L_{\text {parallel }}}=\frac{1}{L_{1}}+\frac{1}{L_{2}}\) or L_parallel = \(\frac{L_{1} L_{2}}{L_{1}+L_{2}}\)
Hence, the equivalent inductance is less than the inductance of either coil.

Question 3.
In a Faraday disc dynamo, a metal disc of radius R rotates with an angular velocity ω about an axis perpendicular to the plane of the disc and passing through its centre. The disc is placed in a magnetic field B acting perpendicular to the plane of the disc. Determine the induced emf between the rim and the axis of the disc.
Answer:
Suppose a thin conducting disc of radius R is rotated anticlockwise, about its axis, in a plane perpendicular to a uniform magnetic field of induction \(\vec{B}\) (see the figure in the above Note for reference). \(\vec{B}\) points downwards. Let the constant angular speed of the disc be ω.

Consider an infinitesimal element of radial thickness dr at a distance r from the rotation axis. In one rotation, the area traced by the element is dA = 2πrdr. Therefore, the time rate at which the element traces out the area is
\(\frac{d A}{d t}\) = frequency of rotation x dA = fdA
where f = \(\frac{\omega}{2 \pi}\) is the frequency of rotation.
.’. \(\frac{d A}{d t}=\frac{\omega}{2 \pi}\) (2 πr dr) = ωr dr
The total emf induced between the axle and the rim of the rotating disc is
\(|e|=\int B \frac{d A}{d t}=\int_{0}^{R} B \omega r d r=B \omega \int_{0}^{R} r d r=B \omega \frac{R^{2}}{2}\)
For anticlockwise rotation in \(\vec{B}\) pointing down, the axle is at a higher potential.

Question 4.
A horizontal wire 20 m long extending from east to west is falling with a velocity of 10 m/s normal to the Earth’s magnetic field of 0.5 × 10^-4 T. What is the value of induced emf in the wire?
Answer:
Data : l = 20 m, v = 10 m/s. B = 5 × 10^-5 T
The magnitude of the induced emf,
|e| = Blv = (5 × 10^-5)(20)(10) = 10^-2V = 10 mV

Question 5.
A metal disc is made to spin at 20 revolutions per second about an axis passing through its centre and normal to its plane. The disc has a radius of 30 cm and spins in a uniform magnetic field of 0.20 T, which is parallel to the axis of rotation. Calculate
(a) The area swept out per second by the radius of the disc,
(b) The flux cut per second by a radius of the disc,
(c) The induced emf in the disc.
Answer:
Data: R = 0.3m, f = 20 rps, B = 0.2T
(a) The area swept out per unit time by a given radius = (the frequency of rotations) × (the area swept out per rotation) = f(πr²)
= (20)(3.142 × 0.09) = 5.656 m²

(b) The time rate at which a given radius cuts magnetic flux
= \(\frac{d \Phi_{\mathrm{m}}}{d t}\) = B f(πr²)
= (0.2)(5.656) = 1.131 Wb/s

(c) The magnitude of the induced emf,
|e| = \(\frac{d \Phi_{\mathrm{m}}}{d t}\) = 1.131 V

Question 6.
A pair of adjacent coils has a mutual inductance of 1.5 H. If the current in one coil changes from 0 to 10 A in 0.2 s, what is the change of flux linkage with the other coil?
Answer:
Data: M = 1.5 H, I_1i = 0, I_1f = 10A, ∆f = 0.2s
The flux linked per unit turn with the second coil due to current I₁ in the first coil is
Φ₂₁ = MI₁
Therefore, the change in the flux due to change in I₁ is
∆₂₁ =M(∆I₁) = M(I_1f – I_1i) = 1.5 (10 – 0)
= 15 Wb
[Note: The rate of change of flux linkage is M(∆I₁/∆t) = 15/0.2 = 75 Wb/s] .

Question 7.
A long solenoid has 1500 turns/m. A coil C having cross sectional area 25 cm2 and 150 turns (N_c) is wound tightly around the centre of the solenoid. If a current of 3.0A flows through the solenoid, calculate :
(a) the magnetic flux density at the centre of the solenoid,
(b) the flux linkage in the coil C,
(c) the average emf induced in coil C if the direction of the current in the solenoid is reversed in a time of 0.5 s. (µ₀ = 4π × 10^-7 H/m)
Answer:
Data: n = 1.5 × 10³ m , A = 25 × 10^-4 m²,
N_c = 150, I = 3A, ∆t = 0.5s,
µ₀ = 4π × 10^-7 H/m
(a) Magnetic flux density inside the solenoid,
B = u₀ nI = (4π × 10^-7)(1500)(3)
= 5.656 × 10^-3 T = 5.656 mT

(b) Flux per unit turn through the coils of the solenoid, Φ_m = BA
Since the coil C is wound tightly over the solenoid, the flux linkage of C is
N_CΦ_m = N_CBA = (150)(5.656 × 10^-3)(25 × 10^-4)
= 2.121 × 10^-3 Wb = 2.121 mWb

(c) Initial flux through coil C,
Φ_i = N_CΦ_m = 2.121 × 10^-3 Wb
Reversing the current in the solenoid reverses the flux through coil C, the magnitude remaining the same. But since the flux enters through the other face of the coil, the final flux through C is Φ_f = -2.121 × 10^-3 Wb
Therefore, the average emf induced in coil C,
e = \(-\frac{\Phi_{\mathrm{f}}-\Phi_{1}}{\Delta t}=-\frac{(-2.121-2.121) \times 10^{-3}}{0.5}\)
= 2 × 4.242 × 10^-3 = 8.484 × 10^-3 V = 8.484 mV

Question 8.
A search coil having 2000 turns with area 1.5 cm² is placed in a magnetic field of 0.60T. The coil is moved rapidly out of the field in a time of 0.2 second. Calculate the induced emf across the search coil.
Answer:
Data: N = 2000, A_i = 1.5 × 10^-4 m², A_f = 0,
B = 0.6T, ∆t = 0.2s
Initial flux, NΦ_f = NBA_i = 2000(0.6)(1.5 × 10^-4)
= 0.18 Wb
Final flux, NΦ_f = 0, since the coil is withdrawn out of the field.
Induced emf,e = \(-N \frac{\Delta \Phi_{\mathrm{m}}}{\Delta t}=-N \frac{\Phi_{\mathrm{f}}-\Phi_{1}}{\Delta t}\)
∴ e = \(-\frac{0-0.18}{0.2}\) = 0.9V

Question 9.
An aircraft of wing span of 50 m flies horizontally in earth’s magnetic field of 6 × 10^-5 T at a speed of 400 m/s. Calculate the emf generated between the tips of the wings of the aircraft.
Answer:
Data : l = 50 m, B = 6 × 10^-5T, v = 400 m/s
The magnitude of the induced emf,
|e| = Blv = (6 × 10^-5)(400)(50) = 1.2V

Question 10.
A stiff semi-circular wire of radius R is rotated in a uniform magnetic field B about an axis passing through its ends. If the frequency of rotation of the wire is f, calculate the amplitude of the alternating emf induced in the wire.
Answer:
In one rotation, the wire traces out a circle of radius R, i.e., an area A = πR².
Therefore, the rate at which the wire traces out the area is
\(\frac{d A}{d t}\) = frequency or rotation × A = fA
If the angle between the uniform magnetic field \(\vec{B}\) and the rotation axis is θ, the magnitude of the induced emf is
|e|= B\(\frac{d A}{d t}\) cosθ = BfA cosθ = Bf(πR²)cosθ
so that the required amplitude is equal to Bf(πR²).

Question 11.
Calculate the value of induced emf between the ends of an axle of a railway carriage 1.75 m long traveling on level ground with a uniform velocity of 50 km per hour. The vertical component of Earth’s magnetic field (B_v) is given to be 5 × 10^-5T.
Answer:
Data: l = 1.75 m, v = 50 km/h = 50 × \(\frac{5}{18}\) m/s.
B_v = 5 × 10^-5 T
The area swept out by the wing per unit time = 1v.
∴ The magnetic flux cut by the wing per unit time
= \(\frac{d \Phi_{\mathrm{m}}}{d t}\) = B_v(lv)
=(5 × 10^-5)(1.75)(50 × \(\frac{5}{18}\))= 121.5 × 10^-5
= 1.215 mWb/s
Therefore, the magnitude of the induced emf,
|e| =1.215 mV
[Note: In the northern hemisphere, the vertical com ponent of the Earth’s magnetic induction is downwards. Using Fleming’s right hand rule, the port (left) wing-tip would be positive.]

Question 12.
The value of mutual inductance of two coils is 10 mH. If the current in one of the coil changes from 5A to 1A in 0.2 s, calculate the value of emf induced in the other coil.
Answer:
Data: M = 10 mH = 10^-2 H, I_1i = 5A, I_1f = 1 A,
∆t = 0.2s
The mutually induced emf in coil 2 due to the changing current in coil 1,
e₂₁ = \(-M \frac{\Delta I_{1}}{\Delta t}=-M \frac{I_{1 \mathrm{f}}-I_{1 \mathrm{i}}}{\Delta t}\)
= -(10^-2) \(\left(\frac{1-5}{0.2}\right)\) = 0.2 V

Question 13.
An emf of 96.0 mV is induced in the windings of a coil when the current in a nearby coil is increasing at the rate of 1.20 A/s. What is the mutual inductance (M) of the two coils?
Answer:
Data: |e₂| = 9.6 × 10^-2 V, dI₁/dt = 1.2 A/s
|e₂| = M\(\frac{d I_{1}}{d t}\)
Mutual inductance,
M = \(\frac{\left|e_{2}\right|}{d I_{1} / d t}=\frac{9.6 \times 10^{-2}}{1.2}\)
= 8 × 10^-2 H
= 80 mH

Question 14.
A long solenoid of length l, cross-sectional area A and having N₁ turns (primary coil) has a small coil of N₂ turns (secondary coil) wound about its centre. Determine the Mutual inductance (M) of the two coils.
Answer:
We assume the solenoid to be ideal and that all the flux from the solenoid passes through the outer coil C. For a steady current Is through the solenoid, the uniform magnetic field inside the solenoid is
B = µ₀nI_s ……………….. (1)
Then, the magnetic flux through each turn of the coil due to the current in the solenoid is
Φ_CS = BA = (µ₀nI_s)(πR²) ……………… (2)
Thus, their mutual inductance is
M = \(\frac{N \Phi_{\mathrm{CS}}}{I_{\mathrm{S}}}\) = µ₀πR²nN ……………. (3)
Equation (2) is true as long as the magnetic field of the solenoid is entirely contained within the cross section of the coil C. Hence, M does not depend on the shape, size, or possible lack of close packing of the coil.
replacing n with N₁/l and N with N₂. M = µ₀A = \(\frac{N_{1} N_{2}}{l}\)
[Note: The answer given in the textbook misses out the factor of 1.] .

Question 15.
The primary and secondary coil of a transformer each have an inductance of 200 × 10^-6H. The mutual inductance (M) between the windings is 4 × 10^-6H. What percentage of the flux from one coil reaches the other?
Answer:
Data: L_P = L_S = 2 × 10^-4 H, M = 4 × 10^-6 H
M = K\(\sqrt{L_{\mathrm{P}} L_{\mathrm{S}}}\)
The coupling coefficient is
K = \(\frac{M}{\sqrt{L_{\mathrm{P}} L_{\mathrm{S}}}}=\frac{4 \times 10^{-6}}{\sqrt{\left(2 \times 10^{-4}\right)^{2}}}=\frac{4 \times 10^{-6}}{2 \times 10^{-4}}\)
= 2 × 10^-2
Therefore, the percentage of flux of the primary reaching the secondary is
0.02 × 100% = 2%

Question 16.
A toroidal ring, having 100 turns per cm of a thin wire is wound on a nonmagnetic metal rod of length 1 m and diameter 1 cm. If the permeability of bar is equal to that of free space (µ₀), calculate the magnetic field inside the bar (B) when the current (i) circulating through the turns is 1 A. Also determine the self-inductance (L) of the coil.
Answer:
Data : l = 1 m, d = 1 cm, n = 100 cm^-1 = 10⁴ m^-1,
I = 100 A, µ₀ = 4π × 10^-7 H/m
The radius of cross section, r = \(\frac{d}{2}\) = 0.5 cm
= 5 × 10^-3 m
(a) Magnetic field inside the toroid,
B = µ₀nI = (4π × 10^-7)(10⁴)(100)
= 0.4 × 3.142 = 1.257 T

(b) Self inductance of the toroid,
L = µ₀2πRn²A = µ₀n²lA = µ₀n²l(πr²)
= (4π × 10^-7)(10⁴)²(1) [π(5 × 10^-3)²]
= π² × 10^-3 = 9.87 × 10^-3 H = 9.87 mH

Question 17.
A uniform magnetic field B(t), pointing upward fills a circular region of radius, s in horizontal plane. If B is changing with time, find the induced electric field.
[Hint : Part of Maxwell’s equation, applied to a time varying magnetic flux, leads us to the equation \(\oint \vec{E} \cdot \overline{\mathrm{d} l}=\frac{-d \phi_{m}}{d t}\), where \(\vec{E}\) is the electric field induced when the magnetic flux changes at the rate of \(\frac{d \phi_{m}}{d t}\)]
Answer:
The area of the region, A = πs², remains constant while B = B(f) is a function of time. Therefore, the induced emf,
e = \(-\frac{d \Phi_{\mathrm{m}}}{d t}=-\frac{d}{d t}(B A)=-A \frac{d B(t)}{d t}=-\pi s^{2} \frac{d B(t)}{d t}\)
[Note : Emf and electric field are different physical quantities, whose respective SI units are the volt and the volt per metre. The question has accordingly been corrected.]

12th Physics Digest Chapter 12 Electromagnetic induction Intext Questions and Answers

Do you know (Textbook Page No. 274)

Question 1.
If a wire without any current is kept in a magnetic field, then it experiences no force as shown in figure (a). But when the wire is carrying a current into the plane of the paper in the magnetic field, a force will be exerted on the wire towards the left as shown in the figure (b). The field will be strengthened on the right side of the wire where the lines of force are in the same direction as that of the magnetic field and weakened on the left side where the field lines are in opposite direction to that of the applied magnetic field. For a wire carrying a current out of the plane of the paper, the force will act to the right as shown in figure (c).

Answer:
Force on a current-carrying conductor in a magnetic field, \(\vec{F}=I \vec{L} \times \vec{B}\) (Refer unit 10.5). The field due to acurrent-carrying straight conductor is given by right- hand grip rule. As shown in the figure below, the combined field due to a permanent magnet and a current-carrying conductor force the conductor out of the field. The field is strengthened where the two fields are in the same direction and add constructively while the field is weakened where the two fields are opposite in direction.

Use your brain power (Textbook Page No. 282)

Question 1.
It can be shown that the mutual potential energy of two circuits is W = MI₁I₂. Therefore, the mutual inductance (M) may also be defined as the mutual potential energy (W) of two circuits corresponding to unit current flowing in each circuit.
M = \(\frac{W}{I_{1} I_{2}}\)
M = W[I₁ = I₂ = 1]
Answer:
Mutual inductance of two magnetically linked coils equals the potential energy for unit currents in the coils.
1 H = 1 T∙m²/A (= 1 V∙s/A = 1 Ω ∙ s = 1 J/A²)

Use your brain power (Textbook Page No. 284)

Question 1.
Prove that the inductance of parallel wires of length l in the same circuit is given by L = \(\left(\frac{\mu_{0} l}{\pi}\right)\) ln (d / a), where a is the radius of wire and d is separation between wire axes.
Answer:
If l is the current in each wire, from Ampe’re’s law the magnitude of the magnetic field outside each wire is
B = \(\frac{\mu_{0} I}{2 \pi r}\)
By right hand grip rule, the direction of the magnetic field due to both the wires are in the same direction at the point shown. Hence, by the symmetry of the setup, the total magnetic flux through an area dA = l dr shown is two times that due to one wire.

Do you know (Textbook Page No. 285)

Question 1.
The flux rule is the terminology that Feynman used to refer to the law relating magnetic flux to emf. (RP Feynman, Feynman lectures on Physics, Vol II)
Answer:
Modern applications of Faraday’s law of induction :

• Electric generators and motors
• Dynamos in vehicles
• Transformers
• Induction furnaces (industrial), induction cooking stoves (domestic)
• Radio communication
• Magnetic flow meters and energy meters
• Metal detectors at security checks .
• Magnetic hard disk and tape, storage and retrieval
• Graphics tablets
• ATM Credit/debit cards, ATM and point-of-sale (POS) machines
• Pacemakers

Faraday’s second law of electromagnetic induction is referred by some as the “flux rule”.

Balbharti Maharashtra State Board 12th Physics Textbook Solutions Chapter 11 Magnetic Materials Textbook Exercise Questions and Answers.

Maharashtra State Board 12th Physics Solutions Chapter 11 Magnetic Materials

1. Choose the correct option.

i) Intensity of magnetic field of the earth at the point inside a hollow iron box is.
(A) less than that outside
(B) more than that outside
(C) same as that outside
(D) zero
Answer:
(D) zero

ii) Soft iron is used to make the core of transformer because of its
(A) low coercivity and low retentivity
(B) low coercivity and high retentivity
(C) high coercivity and high retentivity
(D) high coercivity and low retentivity
Answer:
(A) low coercivity and low retentivity

iii) Which of the following statements is correct for diamagnetic materials?
(A) µ_r < 1
(B) χ is negative and low
(C) χ does not depend on temperature
(D) All of above
Answer:
(D) All of above

iv) A rectangular magnet suspended freely has a period of oscillation equal to T. Now it is broken into two equal halves ( each having half of the original length) and one piece is made to oscillate freely. Its period of oscillation is T′, the ratio of T′ / T is.
(A) \(\frac{1}2 \sqrt{2}\)
(B) \(\frac{1}{2}\)
(C) 2
(D) \(\frac{1}{4}\)
Answer:
(B) \(\frac{1}{2}\)

v) A magnetising field of 360 Am -1 produces a magnetic flux density (B ) = 0.6 T in a ferromagnetic material. What is its permeability in Tm A^-1 ?
(A) \(\frac{1}{300}\)
(B) 300
(C) \(\frac{1}{600}\)
(D) 600
Answer:
(C) \(\frac{1}{600}\)

2 Answer in brief.

i) Which property of soft iron makes it useful for preparing electromagnet?
Answer:
An electromagnet should become magnetic when a current is passed through its coil but should lose its magnetism once the current is switched off. Hence, the ferromagnetic core (usually iron-based) used for an electromagnet should have high permeability and low retentivity, i.e., it should be magnetically ‘soft’.

ii) What happens to a ferromagnetic material when its temperature increases above curie temperature?
Answer:
A ferromagnetic material is composed of small regions called domains. Within each domain, the atomic magnetic moments of nearest-neighbour atoms interact strongly through exchange interaction, a quantum mechanical phenomenon, and align themselves parallel to each other even in the absence of an external magnetic field. A domain is, therefore, spontaneously magnetized to saturation.

The material retains its domain structure only up to a certain temperature. On heating, the increased thermal agitation works against the spontaneous domain magnetization. Finally, at a certain critical temperature, called the Curie point or Curie temperature, thermal agitation overcomes the exchange forces and keeps the atomic magnetic moments randomly oriented. Thus, above the Curie point, the material becomes paramagnetic. The ferromagnetic to paramagnetic transition is an order to disorder transition. When cooled below the Curie point, the material becomes ferromagnetic again.

iii) What should be retentivity and coercivity of permanent magnet?
Answer:
A permanent magnet should have a large zero-field magnetization and should need a very large reverse field to demagnetize. In other words, it should have a very broad hysteresis loop with high retentivity and very high coercivity.

iv) Discuss the Curie law for paramagnetic material.
Answer:
Curie’s law : The magnetization of a paramagnetic material is directly proportional to the external magnetic field and inversely proportional to the absolute temperature of the material.

If a paramagnetic material at an absolute temperature T is placed in an external magnetic field of induction \(\), the magnitude of its magnetization
M_z ∝ \(\frac{B_{\text {ext }}}{T}\) ∴ M_z = C\(\frac{B_{\text {ext }}}{T}\)
where the proportionality constant C is called the Curie constant.
[Notes : (1) The above law, discovered experimentally in 1895 by Pierre Curie (1859-1906) French physcist, is true only for values of Bext/ T below about 0.5 tesla per kelvin.
(2) [C] = [M_z ∙ T] / [B_ext] = [L^-1I ∙ ] /[MT^-2I^-1]
= [M^-1L^-1T²I²],
where  denotes the dimension of temperature.]

v) Obtain and expression for orbital magnetic moment of an electron rotating about the nucleus in an atom.
Answer:
In the Bohr model of a hydrogen atom, the electron of charge – e performs a uniform circular motion around the positively charged nucleus. Let r, v and T be the orbital radius, speed and period of motion of the electron. Then,
T = \(\frac{2 \pi r}{v}\) …………….. (1)
Therefore, the orbital magnetic moment asso-ciated with this orbital current loop has a magnitude,
I = \(\frac{e}{T}=\frac{e v}{2 \pi r}\) …………… (2)
Therefore, the magnetic dipole moment associated with this electronic current loop has a magnitude
M₀ = current × area of the loop
= I(πr²) = \(\frac{e v}{2 \pi r}\) × πr² = \(\frac{1}{2}\) evr ……………… (3)
Multiplying and dividing the right hand side of the above expression by the electron mass m_e,
M₀ = \(\frac{e}{2 m_{\mathrm{e}}}\) (m_evr) = \(\frac{e}{2 m_{\mathrm{e}}}\) L₀ ……………. (4)
where L₀ = m_evr is the magnitude of the orbital angular momentum of the electron. \(\vec{M}_{0}\) is opposite to \(\vec{L}_{0}\).
∴ \(\vec{M}_{0}=-\frac{e}{2 m_{e}} \overrightarrow{L_{0}}\) ……………. (5)
which is the required expression.

According to Bohr’s second postulate of stationary orbits in his theory of hydrogen atom, the angular momentum of the electron in the nth stationary orbit is equal to n \(\frac{h}{2 \pi}\) , where h is the Planck constant and n is a positive integer. Thus, for an orbital electron,

L₀ = m_evr = \(\frac{nh}{2 \pi}\) …………… (6)
Substituting for L₀ in Eq. (4),
M₀ = \(\frac{e n h}{4 \pi m_{\mathrm{e}}}\)
For n = 1, M₀ = \(\frac{e n h}{4 \pi m_{\mathrm{e}}}\)
The quantity \(\frac{e n h}{4 \pi m_{\mathrm{e}}}\) is a fundamental constant called the Bohr magneton,
µ_B ∙ µ_B = 9.274 × 10^-24 J/T (or A∙m²) = 5.788 × 10^-5 eV/T.
[ Notes : (1) Magnetic dipole moment is conventionally denoted by µ. (2) The magnetic moment of an atom is expressed in terms of Bohr magneton (vµ_B). (3) According to quantum mechanics, an atomic electron also has an intrinsic spin angular momentum and an associated spin magnetic moment of magnitude µ₅. It is this spin magnetic moment that gives rise to magnetism in matter. (4) The total magnetic moment of the atom is the vector sum of its orbital magnetic moment and spin magnetic moment.]

vi) What does the hysteresis loop represents?
Answer:
A magnetic hysteresis loop is a closed curve obtained by plotting the magnetic flux density B of a ferromagnetic material against the corresponding magnetizing field H when the material is taken through a complete magnetizing cycle. The area enclosed by the loop represents the hysteresis loss per unit volume in taking the material through the magnetizing cycle.

vii) Explain one application of electromagnet.
Answer:
Applications of an electromagnet:

1. Electromagnets are used in electric bells, loud speakers and circuit breakers.
2. Large electromagnets are used in junkyard cranes and industrial cranes to lift iron scraps.
3. Superconducting electromagnets are used in MRI and NMR machines, as well as in particle accelerators of cyclotron family.
4. Electromagnets are used in data storage devices such as computer hard disks and magnetic tapes.

Question 3.
When a plate of magnetic material of size 10 cm × 0.5 cm × 0.2 cm (length , breadth and thickness respectively) is located in magnetising field of 0.5 × 10⁴ Am^-1 then a magnetic moment of 0.5 A∙m² is induced in it. Find out magnetic induction in plate.
Answer:
Data : l = 10 cm, b = 0.5 cm, h = 0.2 cm,
H = 0.5 × 10⁴ Am^-1, M = 5 A∙m²
The volume of the plate,
V = 10 × 0.5 × 0.2 = 1 cm² = 10^-6 m²
B = μ₀ (H + M_z) = μ₀ (H + \(\frac{M}{V}\))
The magnetic induction in the plate,
∴ B = 4π × 10^-7 (0.5 × 10⁴ + \(\frac{5}{10^{-6}}\))
= 6.290 T

Question 4.
A rod of magnetic material of cross section 0.25 cm² is located in 4000 Am^-1 magnetising field. Magnetic flux passing through the rod is 25 × 10^-6 Wb. Find out (a) relative permeability (b) magnetic susceptibility and (c) magnetisation of the rod.
Answer:
Data: A = 0.25 cm² = 25 × 10^-6 m²,
H = 4000 A∙m^-1, Φ = 25 × 10^-6 Wb
Magnetic induction is
B = \(\frac{\phi}{A}=\frac{25 \times 10^{-6}}{25 \times 10^{-6}}\) = 1 Wb/m²
(a) B = µ₀µ_rK
∴ The relative permeability of the material,
µ_r = \(\frac{B}{\mu_{0} H}=\frac{1}{4 \times 3.142 \times 10^{-7} \times 4000}\)
= \(\frac{10000}{50.272}\) = 198.91 = 199

(b) µ_r = 1 + χ_m
∴ The magnetic susceptibility of the material,
χ_m = µ_r – 1 = 199 – 1 = 198

(c) χ_m = \(\frac{M_{\mathrm{z}}}{H}\)
The magnetization of the rod,
M_z = χ_mH = 198 × 4000 = 7.92 × 10⁵ A/m

Question 5.
The work done for rotating a magnet with magnetic dipole momentm, through 90° from its magnetic meridian is n times the work done to rotate it through 60°. Find the value of n.
Answer:
Data : θ₀ = 0°, θ₁ = 90°, θ₂ = 60°, W₁ = nW₂
The work done by an external agent to rotate the magnet from θ₀ to θ is
W = MB (cos θ₀ – cos θ)
∴ W1 = MB(cos θ₀ – cosθ₁)
= MB (cos 0° – cos 90°)
= MB (1 – 0)
= MB

∴ W2 = MB (cos 0°- cos 60°)
= MB(1 – \(\frac{1}{2}\))
= 0.5MB
∴ W₁ = 2W₂ = MB
Given W₁ = nW₂. Therefore n = 2.

Question 6.
An electron in an atom is revolving round the nucleus in a circular orbit of radius 5.3 × 10^-11 m, with a speed of 2 × 10⁶ ms^-1 Find the resultant orbital magnetic moment and angular momentum of electron. (charge on electron e = 1.6 × 10^-19 C, mass of electron m_e = 9.1 × 10^-31 kg.)
Answer:
Data: r = 5.3 × 10^-11 m, v = 2 × 10⁶ m/s,
e = 1.6 × 10^-19 C, m_e = 9.1 × 10^-31 kg
The orbital magnetic moment of the electron is
M₀ = \(\frac{1}{2}\) evr
= \(\frac{1}{2}\) (1.6 × 10^-19) (2 × 10⁶) (5.3 × 10^-11)
= 8.48 × 10^-24 A∙m²
The angular momentum of the electron is
L₀ = m_evr
=(9.1 × 10^-31) (2 × 10⁶) (5.3 × 10^-11)
= 96.46 × 10^-36 = 9.646 × 10^-35 kg∙m²/s

Question 7.
A paramagnetic gas has 2.0 × 10²⁶ atoms/m with atomic magnetic dipole moment of 1.5 × 10^-23 A m² each. The gas is at 27° C. (a) Find the maximum magnetization intensity of this sample. (b) If the gas in this problem is kept in a uniform magnetic field of 3 T, is it possible to achieve saturation magnetization? Why? (k_B = 1.38 × 10^-23 JK^-1)[Answer: 3.0× 103 A m-1, No]
(Hint: Find the ratio of Thermal energy of atom of a gas ( 3/2 kBT) and maximum potential energy of the atom (mB) and draw your conclusion)
Answer:
Data: \(\frac{N}{V}\) = 2.0 × 10²⁶ atoms/m³,
μ = 1.5 × 10^-23 Am2, T = 27 + 273 = 300 K,
B = 3T, k^B = 1.38 × 10^-23 J/K, 1 eV = 1.6 × 10^-19 J
(a) The maximum magnetization of the material,
M_z = \(\frac{N}{V}\)μ =(2.0 × 10²⁶) (1.5 ×10^-23)
= 3 × 10³ A/m

(b) The maximum orientation energy per atom is
U_m = -μB cos 180° = μB
= (1.5 × 10^-23) (3) = \(\frac{4.5 \times 10^{-23}}{1.6 \times 10^{-19}}\)
= 2.8 × 10^-4 eV

The average thermal energy of each atom,
E = \(\frac{3}{2}\) k_BT
where k_B is the Botzmann constant.
∴ E = 1.5(1.38 × 10^-23)(300)
= 6.21 × 10^-21 J = \(\frac{6.21 \times 10^{-21}}{1.6 \times 10^{-19}}\)
= 3.9 × 10^-2 eV
Since the thermal energy of randomization is about two orders of magnitude greater than the magnetic potential energy of orientation, saturation magnetization will not be achieved at 300 K.

Question 8.
A magnetic needle placed in uniform magnetic field has magnetic moment of 2 × 10^-2 A m², and moment of inertia of 7.2 × 10^-7 kg m². It performs 10 complete oscillations in 6 s. What is the magnitude of the magnetic field ?
Answer:
Data: M = 2 × 10^-2 A∙m², I = 7.2 × 10^-7 kg∙m²,
T = \(\frac{6}{10}\) = 0.6 S
T = 2π\(\sqrt{\frac{I}{M B}}\)
The magnitude of the magnetic field is
B = \(\frac{4 \pi^{2} I}{M T^{2}}\)
= \(\frac{(4)(3.14)^{2}\left(7.2 \times 10^{-7}\right)}{\left(2 \times 10^{-2}\right)(0.6)^{2}}\)
= 3.943 × 10^-3 T = 3.943 mT

Question 9.
A short bar magnet is placed in an external magnetic field of 700 guass. When its axis makes an angle of 30° with the external magnetic field, it experiences a torque of 0.014 Nm. Find the magnetic moment of the magnet, and the work done in moving it from its most stable to most unstable position.
Answer:
Data : B = 700 gauss = 0.07 tesla, θ = 30°,
τ = 0.014 N∙m
τ = MB sin θ
The magnetic moment of the magnet is
M = \(\frac{\tau}{B \sin \theta}=\frac{(0.014)}{(0.07)\left(\sin 30^{\circ}\right)}\) = 0.4 A∙m²
The most stable state of the bar magnet is for θ = 0°.
It is in the most unstable state when θ = 180°. Thus, the work done in moving the bar magnet from 0° to 180° is
W = MB(cos θ₀ – cos θ)
= MB (cos 0° – cos 180°)
= MB [1 – (-1)]
= 2 MB = (2) (0.4) (0.07)
= 0.056 J
This the required work done.

Question 10.
A magnetic needle is suspended freely so that it can rotate freely in the magnetic meridian. In order to keep it in the horizontal position, a weight of 0.1 g is kept on one end of the needle. If the pole strength of this needle is 20 Am , find the value of the vertical component of the earth’s magnetic field. (g = 9.8 m s^-2)
Answer:
Data: M = 0.2g = 2 × 10^-4kg, q_m = 20 A∙m, g =9.8 m/s²

Without the added weight at one end, the needle will dip in the direction of the resultant magnetic field inclined with the horizontal. The torque due to the added weight about the vertical axis through the centre balances the torque of the couple due to the vertical component of the Earth’s magnetic field.
∴ (Mg)\(\left(\frac{L}{2}\right)\) = (q_m B_v) L
The vertical component of the Earth’s magnetic field,
B_v = \(\frac{M g}{2 q_{\mathrm{m}}}=\frac{\left(2 \times 10^{-4}\right)(9.8)}{2(20)}\) = 4.9 × 10^-5 T

Question 11.
The susceptibility of a paramagnetic material is χ at 27° C. At what temperature its susceptibility be \(\frac{\chi}{3}\) ?
Answer:
Data: χ_m1 = χ, T₁ = 27°C = 300 K, χ_m2 = \(\frac{\chi}{3}\)
By Curie’s law,
M_z = C\(\frac{B_{0}}{T}\)
Since M_z = χ_mH = B₀ = μ₀H

This gives the required temperature.

12th Physics Digest Chapter 11 Magnetic Materials Intext Questions and Answers

Activity (Textbook Page No. 251)

Question 1.
You have already studied in earlier classes that a short bar magnet suspended freely always aligns in North South direction. Now if you try to forcefully move and bring it in the direction along East West and leave it free, you will observe that the magnet starts turning about the axis of suspension. Do you know from where does the torque which is necessary for rotational motion come from? (as studied in rotational dynamics a torque is necessary for rotational motion).
Answer:
Suspend a short bar magnet such that it can rotate freely in a horizontal plane. Let it come to rest along the magnetic meridian. Rotate the magnet through some angle and release it. You will see that the magnet turns about the vertical axis in trying to return back to its equilibrium position along the magnetic meridian. Where does the torque for the rotational motion come from?

Take another bar magnet and bring it near the suspended magnet resting in the magnetic meridian. Observe the interaction between the like and unlike poles of the two magnets facing each other. Does the suspended magnet rotate continuously or rotate through certain angle and remain stable? Note down your observations and conclusions.

Do you know (Textbook Page No. 255)

Effective magneton numbers for iron group ions (No. of Bohr magnetons)

(Courtsey: Introduction to solid state physics by Charles Kittel, pg. 306 )
These magnetic moments are calculated from the experimental value of magnetic susceptibility. In several ions the magnetic moment is due to both orbital and spin angular momenta.
Answer:
In terms of Bohr magneton (µ_B), the effective magnetic moments of some iron group ions are as follows. In several cases, the magnetic moment is due to both orbital and spin angular momenta.

Remember this (Textbook Page No. 256)

Question 1.
Permeability and Permittivity:
Magnetic Permeability is a term analogous to permittivity in electrostatics. It basically tells us about the number of magnetic lines of force that are passing through a given substance when it is kept in an external magnetic field. The number is the indicator of the behaviour of the material in magnetic field. For superconductors χ = – 1. If you substitute in the Eq. (11.18), it is observed that permeability of material µ = 0. This means no magnetic lines will pass through the superconductor.

Magnetic Susceptibility (χ) is the indicator of measure of the response of a given material to the external applied magnetic field. In other words it indicates as to how much magnetization will be produced in a given substance when kept in an external magnetic field. Again it is analogous to electrical susceptibility. This means when the substance is kept in a magnetic field, the atomic dipole moments either align or oppose the external magnetic field. If the atomic dipole moments of the substance are opposing the field, χ is observed to be negative, and if the atomic dipole moments align themselves in the direction of field, χ is observed to be positive. The number of atomic dipole moments of getting aligned in the direction of the applied magnetic field is proportional to χ. It is large for soft iron (χ >1000).
Answer:
Magnetic permeability is analogous to electric permittivity, both indicating the extent to which a material permits a field to pass through or permeate into the material. For a superconductor, χ = -1 which makes µ = 0, so that a superconductor does not allow magnetic field lines to pass through it.

Magnetic susceptibility (χ). analogous to electrical susceptibility, is a measure of the response of a given material to an applied magnetic field. That is, it indicates the extent of the magnetization produced in the material when it is placed in an external magnetic field. χ is positive when the atomic dipole moments align themselves in the direction of the applied field; χ is negative when the atomic dipole moments align antiparallel to the field. χ is large for soft iron (χ > 1000).

Use your brain power (Textbook Page No. 259)

Question 1.
Classify the following atoms as diamagnetic or paramagnetic.
H, O, Zn, Fe, F, Ar, He
(Hint : Write down their electronic configurations)
Is it true that all substances with even number of electrons are diamagnetic?
Answer:

It can be seen that all substances with an even number of electrons are not necessarily diamagnetic.

Do you know (Textbook Page No. 260)

Question 1.
Exchange Interaction: This exchange interaction in stronger than usual dipole-dipole interaction by an order of magnitude. Due to this exchange interaction, all the atomic dipole moments in a domain get aligned with each other. Find out more about the origin of exchange interaction.
Answer:
Exchange Interaction :
Quantum mechanical exchange interaction be-tween two neighbouring spin magnetic moments in a ferromagnetic material arises as a consequence of the overlap between the magnetic orbitals of two adjacent atoms. The exchange interaction in particular for 3d metals is stronger than the dipole-dipole interaction by an order of magnitude. Due to this, all the atomic dipole moments in a domain get aligned with each other and each domain is spontaneously magnetized to saturation. (Quantum mechanics and exchange interaction are beyond the scope of the syllabus.)

Use your brain power (Textbook Page No. 262)

Question 1.
What does the area inside the curve B – H (hysteresis curve) indicate?
Answer:
A magnetic hysteresis loop is a closed curve obtained by plotting the magnetic flux density B of a ferromagnetic material against the corresponding magnetizing field H when the material is taken through a complete magnetizing cycle. The area enclosed by the loop represents the hysteresis loss per unit volume in taking the material through the magnetizing cycle.

Do you know (Textbook Page No. 262)

Question 1.
What is soft magnetic material?
Soft ferromagntic materials can be easily magnetized and demagnetized.

Hysteresis loop for hard and soft ferramagnetic materials.
Answer:
A soft magnetic material, usually iron-based, has high permeability, low retentivity and low coercivity. In other words, it does not have appreciable hysteresis, i.e., its hysteresis loop is very narrow. Such a material magnetizes and demagnetizes more easily, by small external fields.

Do you know (Textbook Page No. 263)

Question 1.
There are different types of shielding available like electrical and accoustic shielding apart from magnetic shielding discussed above. Electrical insulator functions as an electrical barrier or shield and comes in a wide array of materials. Normally the electrical wires used in our households are also shielded. In case of audio recording it is necessary to reduce other stray sound which may interfere with the sound to be recorded. So the recording studios are sound insulated using acoustic material.
Answer:
There are different types of shielding, such as electrical, electromagnetic. magnetic, RF (radio fre quency) and acoustic, to shield a given space or sensitive instrument from unwanted fields of each type.