Class 12

Balbharti 12th Maharashtra State Board Maths Solutions Book Pdf Chapter 3 Indefinite Integration Ex 3.1 Questions and Answers.

Maharashtra State Board 12th Maths Solutions Chapter 3 Indefinite Integration Ex 3.1

I. Integrate the following functions w.r.t. x:

(i) x³ + x² – x + 1
Solution:

(ii) \(x^{2}\left(1-\frac{2}{x}\right)^{2}\)
Solution:

(iii) \(3 \sec ^{2} x-\frac{4}{x}+\frac{1}{x \sqrt{x}}-7\)
Solution:

(iv) \(2 x^{3}-5 x+\frac{3}{x}+\frac{4}{x^{5}}\)
Solution:

(v) \(\frac{3 x^{3}-2 x+5}{x \sqrt{x}}\)
Solution:

II. Evaluate:

(i) ∫tan² x . dx
Solution:

(ii) \(\int \frac{\sin 2 x}{\cos x} \cdot d x\)
Solution:

(iii) \(\int \frac{\sin x}{\cos ^{2} x} \cdot d x\)
Solution:

(iv) \(\int \frac{\cos 2 x}{\sin ^{2} x} \cdot d x\)
Solution:

(v) \(\int \frac{\cos 2 x}{\sin ^{2} x \cdot \cos ^{2} x} \cdot d x\)
Solution:

= -cot x – tan x + c

(vi) \(\int \frac{\sin x}{1+\sin x} \cdot d x\)
Solution:

(vii) \(\int \frac{\tan x}{\sec x+\tan x} \cdot d x\)
Solution:

(viii) \(\int \sqrt{1+\sin 2 x} \cdot d x\)
Solution:

(ix) \(\int \sqrt{1-\cos 2 x} \cdot d x\)
Solution:

(x) ∫sin 4x cos 3x dx
Solution:

III. Evaluate:

(i) \(\int \frac{x}{x+2} \cdot d x\)
Solution:

(ii) \(\int \frac{4 x+3}{2 x+1} \cdot d x\)
Solution:

(iii) \(\int \frac{5 x+2}{3 x-4} \cdot d x\)
Solution:

(iv) \(\int \frac{x-2}{\sqrt{x+5}} \cdot d x\)
Solution:

(v) \(\int \frac{2 x-7}{\sqrt{4 x-1}} \cdot d x\)
Solution:

(vi) \(\int \frac{\sin 4 x}{\cos 2 x} \cdot d x\)
Solution:

(vii) \(\int \sqrt{1+\sin 5 x} \cdot d x\)
Solution:

(viii) ∫cos² x . dx
Solution:

(ix) \(\int \frac{2}{\sqrt{x}-\sqrt{x+3}} \cdot d x\)
Solution:

(x) \(\int \frac{3}{\sqrt{7 x-2}-\sqrt{7 x-5}} \cdot d x\)
Solution:

IV.

Question 1.
If f'(x) = x – \(\frac{3}{x^{3}}\), f(1) = \(\frac{11}{2}\), find f(x).
Solution:
By the definition of integral,

Balbharti 12th Maharashtra State Board Maths Solutions Book Pdf Chapter 2 Applications of Derivatives Miscellaneous Exercise 2 Questions and Answers.

Maharashtra State Board 12th Maths Solutions Chapter 2 Applications of Derivatives Miscellaneous Exercise 2

I. Choose the correct option from the given alternatives:

Question 1.
If the function f(x) = ax³ + bx² + 11x – 6 satisfies conditions of Rolle’s theorem in [1, 3] and f'(2 + \(\frac{1}{\sqrt{3}}\)) = 0, then values of a and b are respectively.
(a) 1, -6
(b) -2, 1
(c) -1, -6
(d) -1, 6
Answer:
(a) 1, -6

Hint: f(x) = ax³ + bx² + 11x – 6 satisfies the conditions of Rolle’s theorem in [1, 3]
∴ f(1) = f(3)
a(1)³ + b(1)² + 11(1) – 6 = a(3)³ + b(3)² + 11(3) – 6
a + b + 11 = 27a + 9b + 33
26a + 8b = -22
13a + 4b = -11
Only a = 1, b = -6 satisfy this equation.

Question 2.
If f(x) = \(\frac{x^{2}-1}{x^{2}+1}\), for every real x, then the minimum value of f is
(a) 1
(b) 0
(c) -1
(d) 2
Answer:
(c) -1

Question 3.
A ladder 5 m in length is resting against a vertical wall. The bottom of the ladder is pulled along the ground away from the wall at the rate of 1.5 m/sec. The length of the higher point of the ladder when the foot of the ladder is 4.0 m away from the wall decreases at the rate of
(a) 1
(b) 2
(c) 2.5
(d) 3
Answer:
(b) 2

Question 4.
Let f(x) and g(x) be differentiable for 0 < x < 1 such that f(0) = 0, g(0) = 0, f(1) = 6. Let there exist a real number c in (0, 1) such that f'(c) = 2g'(c), then the value of g(1) must be
(a) 1
(b) 3
(c) 2.5
(d) -1
Answer:
(b) 3

Hint: f(x) and g(x) both satisfies the conditions of LMVT in (0, 1).
∴ f'(c) = \(\frac{f(1)-f(0)}{1-0}=\frac{6-0}{1}=6\)
and g'(c) = \(\frac{g(1)-g(0)}{1-0}=\frac{g(1)-0}{1}\) = g(1)
But f'(c) = 2g'(c)
6 = 2g(1)
∴ g(1) = 3

Question 5.
Let f(x) = x³ – 6x² + 9x + 18, then f(x) is strictly decreasing in
(a) (-∞, 1)
(b) [3, ∞)
(c) (-∞, 1] ∪ [3, ∞)
(d) (1, 3)
Answer:
(d) (1, 3)

Question 6.
If x = -1 and x = 2 are the extreme points of y = α log x + βx² + x, then
(a) α = -6, β = \(\frac{1}{2}\)
(b) α = -6, β = \(\frac{-1}{2}\)
(c) α = 2, β = \(\frac{-1}{2}\)
(d) α = 2, β = \(\frac{1}{2}\)
Answer:
(c) α = 2, β = \(\frac{-1}{2}\)

Hint: y = α log x + βx² + x
∴ \(\frac{d y}{d x}=\frac{\alpha}{x}+\beta \times 2 x+1=\frac{\alpha}{x}+2 \beta x+1\)
f(x) has extreme values at x = -1 and x = 2
∴ f'(-1) = 0 and f(2) = 0
α + 2β = 1
and \(\frac{\alpha}{2}\) + 4β = -1
By solving these two equations, we get
α = 2, β = \(\frac{-1}{2}\)

Question 7.
The normal to the curve x² + 2xy – 3y² = 0 at (1, 1)
(a) meets the curve again in the second quadrant
(b) does not meet the curve again
(c) meets the curve again in the third quadrant
(d) meets the curve again in the fourth quadrant
Answer:
(d) meets the curve again in fourth quadrant

Hint: x² + 2xy – 3y² = 0

= slope of the tangent at (1, 1)
∴ equation of the tangent at (1, 1) is -1
∴ equation of the normal is
y – 1= -1 (x – 1) = -x + 1
∴ x + y = 2
∴ y = 2 – x
Substituting y = 2 – x in x² + 2xy – 3y² = 0, we get
x² + 2x(2 – x) – 3 (2 – x)² = 0
⇒ x² + 4x – 2x² – 3(4 – 4x + x²) = 0
⇒ x² – 4x + 3 = 0
⇒ (x – 1)(x – 3) = 0
⇒ x = 1, x = 3
When x = 1, y = 2 – 1 = 1
When x = 3, y = 2 – 3 = -1
∴ the normal at (1, 1) meets the curve at (3, -1) which is in the fourth quadrant.

Question 8.
The equation of the tangent to the curve y = 1 – \(e^{\frac{x}{2}}\) at the point of intersection with Y-axis is
(a) x + 2y = 0
(b) 2x + y = 0
(c) x – y = 2
(d) x + y = 2
Answer:
(a) x + 2y = 0
Hint: The point of intersection of the curve with the Y-axis is the origin (0, 0).

Question 9.
If the tangent at (1, 1) on y² = x(2 – x)² meets the curve again at P, then P is
(a) (4, 4)
(b) (-1, 2)
(c) (3, 6)
(d) \(\left(\frac{9}{4}, \frac{3}{8}\right)\)
Answer:
(d) \(\left(\frac{9}{4}, \frac{3}{8}\right)\)
Hint: y² = x(2 – x)²
= x(4 – 4x + x²)
= x³ – 4x² + 4x

= slope of the tangent at (1, 1)
∴ equation of the tangent at (1, 1) is
y – 1 = –\(\frac{1}{2}\) (x – 1)
∴ 2y – 2 = -x + 1
∴ x + 2y = 3
Only the coordinates \(\left(\frac{9}{4}, \frac{3}{8}\right)\) satisfy both the equations y² = x(2 – x)² and x + 2y = 3
∴ P is \(\left(\frac{9}{4}, \frac{3}{8}\right)\)

Question 10.
The approximate value of tan (44° 30′), given that 1° = 0.0175, is
(a) 0.8952
(b) 0.9528
(c) 0.9285
(d) 0.9825
Answer:
(d) 0.9825

II. Solve the following:

Question 1.
If the curves ax² + by² = 1 and a’x² + b’y² = 1, intersect orthogonally, then prove that \(\frac{1}{a}-\frac{1}{b}=\frac{1}{a^{\prime}}-\frac{1}{b^{\prime}}\)
Solution:
Let P(x₁, y₁) be the point of intersection of the curves.

Question 2.
Determine the area of the triangle formed by the tangent to the graph of the function y = 3 – x² drawn at the point (1, 2) and the coordinate axes.
Solution:
y = 3 – x²

= slope of the tangent at (1, 2)
∴ equation of the tangent at (1, 2) is
y – 2= -2(x – 1)
⇒ y – 2= -2x + 2
⇒ 2x + y = 4
Let this tangent cuts the coordinate axes at A(a, 0) and B(0, b).
∴ 2a + 0 = 4 and 2(0) + b = 4
∴ a = 2 and b = 4
∴ area of required triangle = \(\frac{1}{2}\) × l(OA) × l(OB)
= \(\frac{1}{2}\) ab
= \(\frac{1}{2}\) (2)(4)
= 4 sq units.

Question 3.
Find the equation of the tangent and normal drawn to the curve y⁴ – 4x⁴ – 6xy = 0 at the point M (1, 2).
Solution:
y⁴ – 4x⁴ – 6xy = 0
Differentiating both sides w.r.t. x, we get

= slope of the tangent at (1, 2)
∴ the equation of normal at M (1, 2) is
y – 2 = \(\frac{14}{13}\) (x – 1)
∴ 13y – 26 = 14x – 14
∴ 14x – 13y + 12 = 0
The slope of normal at (1, 2)
\(=\frac{-1}{\left(\frac{d y}{d x}\right)_{\mathrm{at}(1,2)}}=\frac{-1}{\left(\frac{14}{13}\right)}=-\frac{13}{14}\)
∴ the equation of normal at M (1, 2) is
y – 2 = \(\frac{-13}{14}\) (x – 1)
14y – 28 = -13x + 13
13x + 14y – 41 = 0
Hence, the equations of tangent and normal are 14x – 13y + 12 = 0 and 13x + 14y – 41 = 0 respectively.

Question 4.
A water tank in the form of an inverted cone is being emptied at the rate of 2 cubic feet per second. The height of the cone is 8 feet and the radius is 4 feet. Find the rate of change of the water level when the depth is 6 feet.
Solution:

Let r be the radius of the base, h be the height and V be the volume of the water level at any time t.
Since, the height of the cone is 8 feet and the radius is 4 feet,
\(\frac{r}{h}=\frac{4}{8}=\frac{1}{2}\)
r = \(\frac{h}{2}\) ……..(1)

Hence, the rate of change of water level is \(\left(\frac{2}{9 \pi}\right)\) ft/sec.

Question 5.
Find all points on the ellipse 9x² + 16y² = 400, at which the y-coordinate is decreasing and the x-coordinate is increasing at the same rate.
Solution:
Let P(x₁, y₁) be the point on the ellipse 9x² + 16y² = 400 whose y-coordinate decreasing x-coordinate is increasing at the same rate.

Question 6.
Verify Rolle’s theorem for the function f(x) = \(\frac{2}{e^{x}+e^{-x}}\) on [-1, 1].
Solution:
The functions e^x, e^-x, and 2 are continuous and differentiable in their respective domains.
∴ f(x) = \(\frac{2}{e^{x}+e^{-x}}\) is continuous on [-1, 1] and differentiable on (-1, 1), because e^x + e^-x ≠ 0 for all x ∈ [-1, 1].
Now, f(-1) = \(\frac{2}{e^{-1}+e}=\frac{2}{e+e^{-1}}\) and f(1) = \(\frac{2}{e+e^{-1}}\)
∴ f(-1) = f(1)
Thus, the function f satisfies all the conditions of the Rolle’s theorem.
∴ there exist c ∈ (-1, 1) such that f'(c) = 0
Now, f(x) = \(\frac{2}{e^{x}+e^{-x}}\)

Hence, Rolle’s theorem is verified.

Question 7.
The position of a particle is given by the function s(t) = 2t² + 3t – 4. Find the time t = c in the interval 0 ≤ f ≤ 4 when the instantaneous velocity of the particle is equal to its average velocity in this interval.
Solution:
s(t) = 2t² + 3t – 4
∴ s(0) = 2(0)² + 3(0) – 4 = -4
and s(4) = 2(4)² + 3(4) – 4 = 32 + 12 – 4 = 40
∴ average velocity = \(\frac{s(4)-s(0)}{4-0}\)
= \(\frac{40-(-4)}{4}\)
= 11
Also, instantaneous velocity = \(\frac{d s}{d t}\)
= \(\frac{d}{d t}\) (2t² + 3t – 4)
= 2 × 2t + 3 × 1 – 0
= 4t + 3
∴ instantaneous velocity at t = c is \(\left(\frac{d s}{d t}\right)_{t=c}\) = 4c + 3
When instantaneous velocity at t = c equal to its average velocity, we get
4c + 3 = 11
4c = 8
∴ c = 2 ∈ [0, 4]
Hence, t = c = 2.

Question 8.
Find the approximate value of the function f(x) = \(\sqrt{x^{2}+3 x}\) at x = 1.02.
Solution:
f(x) = \(\sqrt{x^{2}+3 x}\)

Question 9.
Find the approximate value of cos^-1(0.51), given π = 3.1416, \(\frac{2}{\sqrt{3}}\) = 1.1547.
Solution:
Let f(x) = cos^-1 x

The formula for approximation is f(a + h)= f(a) + h . f'(a)
∴ cos^-1 (0.51) = f(0.51)
= f(0.5 + 0.01)
= f(0.5) + (0.01) f'(0.5)
= \(\frac{\pi}{3}\) + 0.01 × (-1.1547)
= \(\frac{3.1416}{3}\) – 0.011547
= 1.0472 – 0.011547
= 1.035653
∴ cos^-1 (0.51) = 1.035653.

Question 10.
Find the intervals on which the function y = x^x, (x > 0) is increasing and decreasing.
Solution:
y = x^x
∴ log y = log x^x = x log x
Differentiating both sides w.r.t. x, we get

y is increasing if \(\frac{d y}{d x}\) ≥ 0
i.e. if x^x (1 + log x) ≥ 0
i.e. if 1 + log x ≥ 0 ……[∵ x > 0]
i.e. if log x ≥ -1
i.e. if log x ≥ -log e …….[∵ log e = 1]
i.e. if logx ≥ log \(\frac{1}{e}\)
i.e. if x ≥ \(\frac{1}{e}\)
∴ y is increasing in \(\left[\frac{1}{e^{\prime}}, \infty\right)\)
y is decreasing if \(\frac{d y}{d x}\) ≤ 0
i.e. if x^x (1 + log x) ≤ 0
i.e. if 1 + log x ≤ 0 ……[∵ x > 0]
i.e. if log x ≤ -1
i.e. if log x ≤ -log e
i.e. if log x ≤ log \(\frac{1}{e}\)
i.e. if x ≤ \(\frac{1}{e}\) where x > 0
∴ y is decreasing is \(\left(0, \frac{1}{e}\right]\)
Hence, the given function is increasing in \(\left[\frac{1}{e^{\prime}}, \infty\right)\) and decreasing in \(\left(0, \frac{1}{e}\right]\)

Question 11.
Find the intervals on which the function f(x) = \(\frac{x}{\log x}\) is increasing and decreasing.
Solution:
f(x) = \(\frac{x}{\log x}\)

f is increasing if f'(x) ≥ 0
i.e. if \(\frac{\log x-1}{(\log x)^{2}}\) ≥ 0
i.e. if log x – 1 ≥ 0 ……..[∵ (log x)² > 0]
i.e. if log x ≥ 1
i.e. if log x ≥ log e ………[∵ log e = 1]
i.e. if x ≥ e
∴ f is increasing on [e, ∞)
f is decreasing if f'(x) ≤ 0
i.e. if \(\frac{\log x-1}{(\log x)^{2}}\) ≤ 0
i.e. if log x – 1 ≤ 0 ……..[∵ (log x)² > 0]
i.e. if log x ≤ 1
i.e. if log x ≤ log e
i.e. if x ≤ e
Also, x > 0 and x ≠ 1 because f(x) = \(\frac{x}{\log x}\) is not defined at x = 1.
∴ f is decreasing in (0, e] – {1}
Hence, f is increasing in [e, ∞) and decreasing in (0, e] – {1}.

Question 12.
An open box with a square base is to be made out of the given quantity of sheet of area a². Show that the maximum volume of the box is \(\frac{a^{3}}{6 \sqrt{3}}\).
Solution:
Let x be the side of square base and h be the height of the box.
Then x² + 4xh = a²
∴ h = \(\frac{a^{2}-x^{2}}{4 x}\) …….(1)
Let V be the volume of the box.
Then V = x²h


Hence, the maximum volume of the box is \(\frac{a^{3}}{6 \sqrt{3}}\) cu units.

Question 13.
Show that of all rectangles inscribed in a given circle, the square has the maximum area.
Solution:

Let ABCD be a rectangle inscribed in a circle of radius r.
Let AB = x and BC = y.
Then x² + y² = 4r² …….(1)
Area of rectangle = xy
= \(x \sqrt{4 r^{2}-x^{2}}\) ……[By (1)]
Let f(x) = x²(4r² – x²)
= 4r²x² – x⁴
∴ f'(x) = \(\frac{d}{d x}\) (4r2x2 – x4)
= 4r² × 2x – 4x³
= 8r²x – 4x³
and f”(x) = \(\frac{d}{d x}\) (8r²x – 4x³)
= 8r² × 1 – 4 × 3x²
= 8r² – 12x²
For maximum area, f'(x) = 0
⇒ 8r²x – 4x³ = 0
⇒ 4x³ = 8r²x
⇒ x² = 2r² ……..[∵ x ≠ 0]
⇒ x = √2r …..[x > 0]
and f”(√2r) = 8r² – 12(√2r)² = -16r² < 0
∴ f(x) is maximum when x = √2r
If x = √2r, then from (1),
(√2r)² + y² = 4r²
⇒ y² = 4r² – 2r² = 2r²
⇒ y = √2r ……[∵ y > 0]
⇒ x = y
∴ rectangle is a square.
Hence, amongst all rectangles inscribed in a circle, the square has maximum area.

Question 14.
Show that a closed right circular cylinder of a given surface area has maximum volume if its height equals the diameter of its base.
Solution:
Let r be the radius of the base, h be the height and V be the volume of the closed right circular cylinder, whose surface area is a² sq units (which is given).
2πrh + 2πr² = a²
⇒ 2πr(h + r) = a²
⇒ h = \(\frac{a^{2}}{2 \pi r}\) – r ……(1)


Hence, the volume of the cylinder is maximum if its height is equal to the diameter of the base.

Question 15.
A window is in the form of a rectangle surmounted by a semicircle. If the perimeter is 30 m, find the dimensions so that the greatest possible amount of light may be admitted.
Solution:

Let x be the length, y be the breadth of the rectangle and r be the radius of the semicircle.
Then perimeter of the window = x + 2y + πr, where x = 2r
This is given to be 30 m
⇒ 2r + 2y + πr = 30
⇒ 2y = 30 – (π + 2)r
⇒ y = 15 – \(\frac{(\pi+2) r}{2}\) ……..(1)
The greatest possible amount of light may be admitted if the area of the window is maximized.
Let A be the area of the window.


Hence, the required dimensions of the window are as follows:
Length of rectangle = \(\left(\frac{60}{\pi+4}\right)\) metres
breadth of rectangle = \(\left(\frac{30}{\pi+4}\right)\) metres
radius of the semicircle = \(\left(\frac{30}{\pi+4}\right)\) metres

Question 16.
Show that the height of a right circular cylinder of greatest volume that can be inscribed in a right circular cone is one-third of that of the cone.
Solution:

Given the right circular cone of fixed height h and semi-vertical angle a.
Let R be the radius of the base and H be the height of the right circular cylinder that can be inscribed in the right circular cone.
In the figure, ∠GAO = α, OG = r, OA = h, OE = R, CE = H.
We have, \(\frac{r}{h}\) = tan α
∴ r = h tan α ……(1)
Since ∆AOG and ∆CEG are similar.



Hence, the height of the right circular cylinder is one-third of that of the cone.

Question 17.
A wire of length l is cut into two parts. One part is bent into a circle and the other into a square. Show that the sum of the areas of the circle and the square is the least if the radius of the circle is half of the side of the square.
Solution:
Let r be the radius of the circle and x be the length of the side of the square. Then
(circumference of the circle) + (perimeter of the square) = l
∴ 2πr + 4x = l
∴ r = \(\frac{l-4 x}{2 \pi}\)
A = (area of the circle) + (area of the square)
= πr² + x²

This shows that the sum of the areas of circle and square is least when the radius of the circle = (\(\frac{1}{2}\)) side of the square.

Question 18.
A rectangular sheet of paper of fixed perimeter with the sides having their lengths in the ratio 8 : 15 converted into an open rectangular box by folding after removing the squares of the equal area from all comers. If the total area of the removed squares is 100, the resulting box has maximum volume. Find the lengths of the rectangular sheet of paper.
Solution:

The sides of the rectangular sheet of paper are in the ratio 8 : 15.
Let the sides of the rectangular sheet of paper be 8k and 15k respectively.
Let x be the side of the square which is removed from the comers of the sheet of paper.
The total area of removed squares is 4x², which is given to be 100.
4x² = 100
⇒ x² = 25
⇒x = 5 ……[x > 0]
Now, the length, breadth, and height of the rectangular box are 15k – 2x, 8k – 2x, and x respectively.
Let V be the volume of the box.
Then V = (15k – 2x) (8k – 2x) . x
⇒ V = (120k² – 16kx – 30kx + 4x²) . x
⇒ V = 4x³ – 46kx² + 120k²x
\(\frac{d V}{d x}=\frac{d}{d x}\) (4x³ – 46kx² + 120k²x)
= 4 × 3x² – 46k × 2x + 120k² × 1
= 12x² – 92kx + 120k²
Since, volume is maximum when the square of side x = 5 is removed from the corners,
\(\left(\frac{d V}{d x}\right)_{\text {at } x=5}=0\)
⇒ 12(5)² – 92k(5) + 120k² = 0
⇒ 60 – 92k + 24k² = 0
⇒ 6k² – 23k + 15 = 0
⇒ 6k² – 18k – 5k + 15 = 0
⇒ 6k(k – 3) – 5 (k – 3) = 0
⇒ (k – 3)(6k – 5) = 0
⇒ k = 3 or k = \(\frac{5}{6}\)
If k = \(\frac{5}{6}\), then
8k – 2x = 8k – 10 < 0
∴ k ≠ \(\frac{5}{6}\)
∴ k = 3
∴ 8k = 8 × 3 = 24 and 15k = 15 × 3 = 45
Hence, the lengths of the rectangular sheet are 24 and 45.

Question 19.
Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius r is \(\frac{4 r}{3}\).
Solution:

Let x be the radius of the base and h be the height of the cone which is inscribed in a sphere of radius r.
In the figure, AD = h and CD = x = BD
Since, ΔABD and ΔBDE are similar,
\(\frac{\mathrm{AD}}{\mathrm{BD}}=\frac{\mathrm{BD}}{\mathrm{DE}}\)
BD² = AD . DE = AD (AE – AD)
x² = h(2r – h) …… (1)
Let V be the volume of the cone.


∴ V is maximum when h = \(\frac{4 r}{3}\)
Hence, the altitude (i.e. height) of the right circular cone of maximum volume = \(\frac{4 r}{3}\).

Question 20.
Show that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is \(\frac{2 R}{\sqrt{3}}\). Also, find the maximum Volume.
Solution:
Let R be the radius and h be the height of the cylinder which is inscribed in a sphere of radius r cm.
Then from the figure,
\(R^{2}+\left(\frac{h}{2}\right)^{2}=r^{2}\)
∴ R² = r² – \(\frac{h^{2}}{4}\) ………(1)
Let V be the volume of the cylinder.
Then V = πR²h



Hence, the volume of the largest cylinder inscribed in a sphere of radius ‘r’ cm = \(\frac{4 R^{3}}{3 \sqrt{3}}\) cu cm.

Question 21.
Find the maximum and minimum values of the function f(x) = cos²x + sin x.
Solution:
f(x) = cos²x + sin x
∴ f'(x) = \(\frac{d}{d x}\) (cos²x + sin x)
= 2 cos x . \(\frac{d}{d x}\) (cos x) + cos x
= 2 cos x(-sin x) + cos x
= -sin 2x + cos x
and f”(x) = \(\frac{d}{d x}\) (-sin 2x + cos x)
= -cos 2x . \(\frac{d}{d x}\) (2x) – sin x
= -cos 2x × 2 – sin x
= -2 cos 2x – sin x
For extreme values of f(x), f'(x) = 0
-sin 2x + cos x = 0
-2 sin x cos x + cos x = 0
cos x (-2 sin x + 1) = 0
cos x = 0 or -2 sin x + 1 = 0
cos x = cos \(\frac{\pi}{2}\) or sin x = \(\frac{1}{2}\) = sin \(\frac{\pi}{6}\)
∴ x = \(\frac{\pi}{2}\) or x = \(\frac{\pi}{6}\)

(i) f”(\(\frac{\pi}{2}\)) = -2 cos π – sin \(\frac{\pi}{2}\)
= -2(-1) – 1
= 1 > 0
∴ by the second derivative test, f is minimum at x = \(\frac{\pi}{2}\) and minimum value of f at x = \(\frac{\pi}{2}\)
= f(\(\frac{\pi}{2}\))
= \(\cos ^{2} \frac{\pi}{2}+\sin \frac{\pi}{2}\)
= 0 + 1
= 1

(ii) f”(\(\frac{\pi}{6}\)) = \(-2 \cos \frac{\pi}{3}-\sin \frac{\pi}{6}\)
= \(-2\left(\frac{1}{2}\right)-\frac{1}{2}\)
= \(-\frac{3}{2}\) < 0
∴ by the second derivative test, f is maximum at x = \(\frac{\pi}{6}\) and maximum value of f at x = \(\frac{\pi}{6}\)
= f(\(\frac{\pi}{6}\))
= \(\cos ^{2} \frac{\pi}{6}+\sin \frac{\pi}{6}\)
= \(\left(\frac{\sqrt{3}}{2}\right)^{2}+\frac{1}{2}\)
= \(\frac{5}{4}\)
Hence, the maximum and minimum values of the function f(x) are \(\frac{5}{4}\) and 1 respectively.

Balbharti 12th Maharashtra State Board Maths Solutions Book Pdf Chapter 1 Differentiation Miscellaneous Exercise 1 Questions and Answers.

Maharashtra State Board 12th Maths Solutions Chapter 1 Differentiation Miscellaneous Exercise 1

(I) Choose the correct option from the given alternatives:

Question 1.
Let f(1) = 3, f'(1) = \(-\frac{1}{3}\), g(1) = -4 and g'(1) = \(-\frac{8}{3}\). The derivative of \(\sqrt{[f(x)]^{2}+[g(x)]^{2}}\) w.r.t. x at x = 1 is
(a) \(-\frac{29}{15}\)
(b) \(\frac{7}{3}\)
(c) \(\frac{31}{15}\)
(d) \(\frac{29}{15}\)
Answer:
(d) \(\frac{29}{15}\)

Question 2.
If y = sec(tan^-1 x), then \(\frac{d y}{d x}\) at x = 1, is equal to
(a) \(\frac{1}{2}\)
(b) 1
(c) \(\frac{1}{\sqrt{2}}\)
(d) 2
Answer:
(c) \(\frac{1}{\sqrt{2}}\)

Question 3.
If f(x) = \(\sin ^{-1}\left(\frac{4^{x+\frac{1}{2}}}{1+2^{4 x}}\right)\), which of the following is not the derivative of f(x)?
(a) \(\frac{2 \cdot 4^{x} \log 4}{1+4^{2 x}}\)
(b) \(\frac{4^{x+1} \log 2}{1+4^{2 x}}\)
(c) \(\frac{4^{x+1} \log 4}{1+4^{4 x}}\)
(d) \(\frac{2^{2(x+1)} \log 2}{1+2^{4 x}}\)
Answer:
(c) \(\frac{4^{x+1} \log 4}{1+4^{4 x}}\)

Question 4.
If x^y = y^x, then\(\frac{d y}{d x}\) = _______
(a) \(\frac{x(x \log y-y)}{y(y \log x-x)}\)
(b) \(\frac{y(y \log x-x)}{x(x \log y-y)}\)
(c) \(\frac{y^{2}(1-\log x)}{x^{2}(1-\log y)}\)
(d) \(\frac{y(1-\log x)}{x(1-\log y)}\)
Answer:
(b) \(\frac{y(y \log x-x)}{x(x \log y-y)}\)

Question 5.
If y = sin (2 sin^-1 x), then \(\frac{d y}{d x}\) = _______
(a) \(\frac{2-4 x^{2}}{\sqrt{1-x^{2}}}\)
(b) \(\frac{2+4 x^{2}}{\sqrt{1-x^{2}}}\)
(c) \(\frac{4 x^{2}-1}{\sqrt{1-x^{2}}}\)
(d) \(\frac{1-2 x^{2}}{\sqrt{1-x^{2}}}\)
Answer:
(a) \(\frac{2-4 x^{2}}{\sqrt{1-x^{2}}}\)

Question 6.
If y = \(\tan ^{-1}\left(\frac{x}{1+\sqrt{1-x^{2}}}\right)+\sin \left[2 \tan ^{-1}\left(\sqrt{\frac{1-x}{1+x}}\right)\right]\), then \(\frac{d y}{d x}\) = _______
(a) \(\frac{x}{\sqrt{1-x^{2}}}\)
(b) \(\frac{1-2 x}{\sqrt{1-x^{2}}}\)
(c) \(\frac{1-2 x}{2 \sqrt{1-x^{2}}}\)
(d) \(\frac{1-2 x^{2}}{\sqrt{1-x^{2}}}\)
Answer:
(c) \(\frac{1-2 x}{2 \sqrt{1-x^{2}}}\)

Question 7.
If y is a function of x and log(x + y) = 2xy, then the value of y'(0) = _______
(a) 2
(b) 0
(c) -1
(d) 1
Answer:
(d) 1

Question 8.
If g is the inverse of function f and f'(x) = \(\frac{1}{1+x^{7}}\), then the value of g'(x) is equal to:
(a) 1 + x⁷
(b) \(\frac{1}{1+[g(x)]^{7}}\)
(c) 1 + [g(x)]⁷
(d) 7x⁶
Answer:
(c) 1 + [g(x)]⁷

Question 9.
If \(x \sqrt{y+1}+y \sqrt{x+1}=0\) and x ≠ y, then \(\frac{d y}{d x}\) = _______
(a) \(\frac{1}{(1+x)^{2}}\)
(b) \(-\frac{1}{(1+x)^{2}}\)
(c) (1 + x)²
(d) \(-\frac{x}{x+1}\)
Answer:
(b) \(-\frac{1}{(1+x)^{2}}\)

Question 10.
If y = \(\tan ^{-1}\left(\sqrt{\frac{a-x}{a+x}}\right)\), where -a < x < a, then \(\frac{d y}{d x}\) = _______
(a) \(\frac{x}{\sqrt{a^{2}-x^{2}}}\)
(b) \(\frac{a}{\sqrt{a^{2}-x^{2}}}\)
(c) \(-\frac{1}{2 \sqrt{a^{2}-x^{2}}}\)
(d) \(\frac{1}{2 \sqrt{a^{2}-x^{2}}}\)
Answer:
(c) \(-\frac{1}{2 \sqrt{a^{2}-x^{2}}}\)
[Hint: Put x = a cos θ]

Question 11.
If x = a (cos θ + θ sin θ), y = a (sin θ – θ cos θ), then \(\left[\frac{d^{2} y}{d x^{2}}\right]_{\theta=\frac{\pi}{4}}\) = _______
(a) \(\frac{8 \sqrt{2}}{a \pi}\)
(b) \(-\frac{8 \sqrt{2}}{a \pi}\)
(c) \(\frac{a \pi}{8 \sqrt{2}}\)
(d) \(\frac{4 \sqrt{2}}{a \pi}\)
Answer:
(a) \(\frac{8 \sqrt{2}}{a \pi}\)

Question 12.
If y = a cos (log x) and \(A \frac{d^{2} y}{d x^{2}}+B \frac{d y}{d x}+C=0\), then the values of A, B, C are _______
(a) x², -x, -y
(b) x², x, y
(c) x², x, -y
(d) x², -x, y
Answer:
(b) x², x, y

(II) Solve the following:

Question 1.


Let u(x) = f[g(x)], v(x) = g[f(x)] and w(x) = g[g(x)]. Find each derivative at x = 1, if it exists i.e. find u'(1), v'(1) and w'(1). if it doesn’t exist then explain why?
Solution:

Question 2.
The values of f(x), g(x), f'(x) and g'(x) are given in the following table:

Match the following:

Solution:

Question 3.
Suppose that the functions f and g and their derivatives with respect to x have the following values at x = 0 and x = 1.

(i) The derivative of f[g(x)] w.r.t. x at x = 0 is _______
(ii) The derivative of g[f(x)] w.r.t. x at x = 0 is _______
(iii) The value of \(\left[\frac{d}{d x}\left[x^{10}+f(x)\right]^{-2}\right]_{x=1}\) is _______
(iv) The derivative of f[(x+g(x))] w.r.t. x at x = 0 is _______
Solution:

Question 4.
Differentiate the following w.r.t. x:
(i) \(\sin \left[2 \tan ^{-1}\left(\sqrt{\frac{1-x}{1+x}}\right)\right]\)
Solution:
Let y = \(\sin \left[2 \tan ^{-1}\left(\sqrt{\frac{1-x}{1+x}}\right)\right]\)
Put x = cos θ, Then θ = cos^-1 x and

(ii) \(\sin ^{2}\left[\cot ^{-1}\left(\sqrt{\frac{1+x}{1-x}}\right)\right]\)
Solution:
Let y = \(\sin ^{2}\left[\cot ^{-1}\left(\sqrt{\frac{1+x}{1-x}}\right)\right]\)
Put x = cos θ. Then θ = cos^-1 x and

(iii) \(\tan ^{-1}\left[\frac{\sqrt{x}(3-x)}{1-3 x}\right]\)
Solution:

(iv) \(\cos ^{-1}\left(\frac{\sqrt{1+x}-\sqrt{1-x}}{2}\right)\)
Solution:
Let y = \(\cos ^{-1}\left(\frac{\sqrt{1+x}-\sqrt{1-x}}{2}\right)\)
Put x = cos θ. Then θ = cos^-1 x and

(v) \(\tan ^{-1}\left(\frac{x}{1+6 x^{2}}\right)+\cot ^{-1}\left(\frac{1-10 x^{2}}{7 x}\right)\)
Solution:

(vi) \(\tan ^{-1}\left[\sqrt{\frac{\sqrt{1+x^{2}+x}}{\sqrt{1+x^{2}}-x}}\right]\)
Solution:

Question 5.
(i) If \(\sqrt{y+x}+\sqrt{y-x}=c\), show that \(\frac{d y}{d x}=\frac{y}{x}-\sqrt{\frac{y^{2}}{x^{2}}-1}\)
Solution:
\(\sqrt{y+x}+\sqrt{y-x}=c\)
Differentiating both sides w.r.t. x, we get

(ii) If \(x \sqrt{1-y^{2}}+y \sqrt{1-x^{2}}=1\), then show that \(\frac{d y}{d x}=-\sqrt{\frac{1-y^{2}}{1-x^{2}}}\)
Solution:
\(x \sqrt{1-y^{2}}+y \sqrt{1-x^{2}}=1\)
\(y \sqrt{1-x^{2}}+x \sqrt{1-y^{2}}=1\)
Differentiating both sides w.r.t. x, we get

(iii) If x sin(a + y) + sin a cos(a + y) = 0, then show \(\frac{d y}{d x}=\frac{\sin ^{2}(a+y)}{\sin a}\)
Solution:
x sin(a + y) + sin a . cos (a + y) = 0 ….. (1)
Differentiating w.r.t. x, we get

(iv) If sin y = x sin(a + y), then show that \(\frac{d y}{d x}=\frac{\sin ^{2}(a+y)}{\sin a}\)
Solution:

(v) If x = \(e^{\frac{x}{y}}\), then show that \(\frac{d y}{d x}=\frac{x-y}{x \log x}\)
Solution:
x = \(e^{\frac{x}{y}}\)
\(\frac{x}{y}\) = log x …..(1)
y = \(\frac{x}{\log x}\)

(vi) If y = f(x) is a differentiable function of x, then show that \(\frac{d^{2} x}{d y^{2}}=-\left(\frac{d y}{d x}\right)^{-3} \cdot \frac{d^{2} y}{d x^{2}}\)
Solution:
If y = f(x) is a differentiable function of x such that inverse function x = f^-1(y) exists,

Question 6.
(i) Differentiate \(\tan ^{-1}\left(\frac{\sqrt{1+x^{2}}-1}{x}\right)\) w.r.t. \(\tan ^{-1}\left(\frac{2 x \sqrt{1-x^{2}}}{1-2 x^{2}}\right)\)
Solution:

(ii) Differentiate \(\log \left[\frac{\sqrt{1+x^{2}}+x}{\sqrt{1+x^{2}}-x}\right]\) w.r.t. cos(log x)
Solution:

(iii) Differentiate \(\tan ^{-1}\left(\frac{\sqrt{1+x^{2}}-1}{x}\right)\) w.r.t. \(\cos ^{-1}\left(\sqrt{\frac{1+\sqrt{1+x^{2}}}{2 \sqrt{1+x^{2}}}}\right)\)
Solution:

Question 7.
(i) If y² = a² cos²x + b² sin²x, show that \(y+\frac{d^{2} y}{d x^{2}}=\frac{a^{2} b^{2}}{y^{3}}\)
Solution:
y² = a² cos²x + b² sin²x …… (1)
Differentiating both sides w.r.t. x, we get

(ii) If log y = log(sin x) – x², show that \(\frac{d^{2} y}{d x^{2}}+4 x \frac{d y}{d x}+\left(4 x^{2}+3\right) y=0\)
Solution:

(iii) If x = a cos θ, y = b sin θ, show that \(a^{2}\left[y \frac{d^{2} y}{d x^{2}}+\left(\frac{d y}{d x}\right)^{2}\right]+b^{2}=0\)
Solution:
x = a cos θ, y = b sin θ
Differentiating x and y w.r.t. θ, we get

(iv) If y = A cos(log x) + B sin(log x), show that x²y₂ + xy₁ + y = o.
Solution:
y = A cos (log x) + B sin (log x) …… (1)
Differentiating both sides w.r.t. x, we get

(v) If y = A e^mx + B e^nx, show that y₂ – (m + n) y₁ + (mn) y = 0.
Solution:
y = A e^mx + B e^nx
Differentiating w.r.t. x, we get

Balbharti 12th Maharashtra State Board Maths Solutions Book Pdf Chapter 1 Differentiation Ex 1.1 Questions and Answers.

Maharashtra State Board 12th Maths Solutions Chapter 1 Differentiation Ex 1.1

Question 1.
Differentiate the following w.r.t. x :
(i) (x³ – 2x – 1)⁵
Solution:
Method 1:
Let y = (x³ – 2x – 1)⁵
Put u = x³ – 2x – 1. Then y = u⁵

Method 2:
Let y = (x³ – 2x – 1)⁵
Differentiating w.r.t. x, we get

(ii) \(\left(2 x^{\frac{3}{2}}-3 x^{\frac{4}{3}}-5\right)^{\frac{5}{2}}\)
Solution:
Let y = \(\left(2 x^{\frac{3}{2}}-3 x^{\frac{4}{3}}-5\right)^{\frac{5}{2}}\)
Differentiating w.r.t. x, we get

(iii) \(\sqrt{x^{2}+4 x-7}\)
Solution:

(iv) \(\sqrt{x^{2}+\sqrt{x^{2}+1}}\)
Solution:
Let y = \(\sqrt{x^{2}+\sqrt{x^{2}+1}}\)
Differentiating w.r.t. x, we get

(v) \(\frac{3}{5 \sqrt[3]{\left(2 x^{2}-7 x-5\right)^{5}}}\)
Solution:
Let y = \(\frac{3}{5 \sqrt[3]{\left(2 x^{2}-7 x-5\right)^{5}}}\)
Differentiating w.r.t. x, we get

(vi) \(\left(\sqrt{3 x-5}-\frac{1}{\sqrt{3 x-5}}\right)^{5}\)
Solution:
Let y = \(\left(\sqrt{3 x-5}-\frac{1}{\sqrt{3 x-5}}\right)^{5}\)
Differentiating w.r.t. x, we get

Question 2.
Diffrentiate the following w.r.t. x
(i) cos(x² + a²)
Solution:
Let y = cos(x² + a²)
Differentiating w.r.t. x, we get
\(\frac{d y}{d x}\) = \(\frac{d}{d x}\)[cos(x² + a²)]
= -sin(x² + a²)∙\(\frac{d}{d x}\)x² + a²)
= -sin(x² + a²)∙(2x + 0)
= -2xsin(x² + a²)

(ii) \(\sqrt{e^{(3 x+2)}+5}\)
Solution:
Let y = \(\sqrt{e^{(3 x+2)}+5}\)
Differentiating w.r.t. x, we get

(iii) log[tan(\(\frac{x}{2}\))]
Solution:
Let y = log[tan(\(\frac{x}{2}\))]
Differentiating w.r.t. x, we get

(iv) \(\sqrt{\tan \sqrt{x}}\)
Solution:
Let y = \(\sqrt{\tan \sqrt{x}}\)
Differentiating w.r.t. x, we get

(v) cot³[log (x³)]
Solution:
Let y = cot³[log (x³)]
Differentiating w.r.t. x, we get

(vi) 5^{sin3x+ 3}
Solution:
Let y = 5^{sin3x+ 3}
Differentiating w.r.t. x, we get

(vii) cosec (\(\sqrt{\cos X}\))
Solution:
Let y = cosec (\(\sqrt{\cos X}\))
Differentiating w.r.t. x, we get

(viii) log[cos (x³ – 5)]
Solution:
Let y = log[cos (x³ – 5)]
Differentiating w.r.t. x, we get

(ix) e^{3 sin2x – 2 cos2x}
Solution:
Let y = e^{3 sin2x – 2 cos2x}
Differentiating w.r.t. x, we get

(x) cos²[log (x²+ 7)]
Solution:
Let y = cos²[log (x²+ 7)]
Differentiating w.r.t. x, we get

(xi) tan[cos (sinx)]
Solution:
Let y = tan[cos (sinx)]
Differentiating w.r.t. x, we get

(xii) sec[tan (x⁴ + 4)]
Solution:
Let y = sec[tan (x⁴ + 4)]
Differentiating w.r.t. x, we get

= sec[tan(x⁴ + 4)]∙tan[tan(x⁴ + 4)]∙sec²(x⁴ + 4)(4x³ + 0)
= 4x³sec²(x⁴ + 4)∙sec[tan(x⁴ + 4)]∙tan[tan(x⁴ + 4)].

(xiii) e^{log[(logx)2 – logx2]}
Solution:
Let y = e^{log[(logx)2 – logx2]}
= (log x)² – log x² …[∵ e^{log x} = x]
Differentiating w.r.t. x, we get

(xiv) sin\(\sqrt{\sin \sqrt{x}}\)
Solution:
Let y = sin\(\sqrt{\sin \sqrt{x}}\)
Differentiating w.r.t. x, we get

(xv) log[sec(e^x2)]
Solution:
Let y = log[sec(e^x2)]
Differentiating w.r.t. x, we get

(xvi) log_e²(logx)
Solution:
Let y = log_e²(logx) = \(\frac{\log (\log x)}{\log e^{2}}\)

(xvii) [log{log(logx)}]²
Solution:
let y = [log{log(logx)}]²
Differentiating w.r.t. x, we get

(xviii) sin²x² – cos²x²
Solution:
Let y = sin²x² – cos²x²
Differentiating w.r.t. x, we get

= 2sinx²∙cosx² × 2x + 2sinx²∙cosx² × 2x
= 4x(2sinx²∙cosx²)
= 4xsin(2x²).

Question 3.
Diffrentiate the following w.r.t. x
(i) (x² + 4x + 1)³ + (x³ – 5x – 2)⁴
Solution:
Let y = (x² + 4x + 1)³ + (x³ – 5x – 2)⁴
Differentiating w.r.t. x, we get
\(\frac{d y}{d x}\) = \(\frac{d}{d x}\)[(x² + 4x + 1)³ + (x³ – 5x – 2)⁴]
= \(\frac{d}{d x}\) = (x² + 4x + 1)³ + \(\frac{d}{d x}\)(x³ – 5x – 2)⁴
= 3(x² + 4x + 1)²∙\(\frac{d}{d x}\)(x² + 4x + 1) + 4(x³ – 5x – 2)⁴∙\(\frac{d}{d x}\)(x³ – 5x – 2)
= 3(x² + 4x + 1)³∙(2x + 4 × 1 + 0) + 4(x³ – 5x – 2)³∙(3x² – 5 × 1 – 0)
= 6 (x + 2)(x² + 4x + 1)² + 4 (3x² – 5)(x³ – 5x – 2)³.
(ii) (1 + 4x)⁵(3 + x − x²)⁸
Solution:
Let y = (1 + 4x)⁵(3 + x − x²)⁸
Differentiating w.r.t. x, we get

= 8 (1 + 4x)⁵ (3 + x – x²)⁷∙(0 + 1 – 2x) + 5 (1 + 4x)⁴ (3 + x – x²)⁸∙(0 + 4 × 1)
= 8 (1 – 2x)(1 + 4x)⁵(3 + x – x²)⁷ + 20(1 + 4x)⁴(3 + x – x²)⁸.

(iii) \(\frac{x}{\sqrt{7-3 x}}\)
Solution:
Let y = \(\frac{x}{\sqrt{7-3 x}}\)
Differentiating w.r.t. x, we get

(iv) \(\frac{\left(x^{3}-5\right)^{5}}{\left(x^{3}+3\right)^{3}}\)
Solution:
Let y = \(\frac{\left(x^{3}-5\right)^{5}}{\left(x^{3}+3\right)^{3}}\)
Differentiating w.r.t. x, we get
\(\frac{d y}{d x}\) = \(\frac{d}{d x}\left[\frac{\left(x^{3}-5\right)^{5}}{\left(x^{3}+3\right)^{3}}\right]\)

(v) (1 + sin²x)²(1 + cos²x)³
Solution:
Let y = (1 + sin²x)²(1 + cos²x)³
Differentiating w.r.t. x, we get

= 3(1 + sin²x)² (1 + cos²x)²∙[2cosx(-sinx)] + 2 (1 + sin²x)(1 + cos²x)³∙[2sinx-cosx]
= 3 (1 + sin²x)² (1 + cos²x)² (-sin 2x) + 2(1 + sin²x)(1 + cos²x)³(sin 2x)
= sin2x (1 + sin²x) (1 + cos²x)² [-3(1 + sin²x) + 2(1 + cos²x)]
= sin2x (1 + sin²x)(1 + cos²x)²(-3 – 3sin²x + 2 + 2cos²x)
= sin2x (1 + sin²x)(1 + cos²x)² [-1 – 3 sin²x + 2 (1 – sin²x)]
= sin 2x(1 + sin²x)(1 + cos²x)² (-1 – 3 sin²x + 2 – 2 sin²x)
= sin2x (1 + sin²x)(1 + cos²x)²(1 – 5 sin²x).

(vi) \(\sqrt{\cos x}+\sqrt{\cos \sqrt{x}}\)
Solution:
Let y = \(\sqrt{\cos x}+\sqrt{\cos \sqrt{x}}\)
Differentiating w.r.t. x, we get
\(\frac{d y}{d x}\) = \(\frac{d}{d x}[\sqrt{\cos x}+\sqrt{\cos \sqrt{x}}]\)

(vii) log(sec 3x+ tan 3x)
Solution:
Let y = log(sec 3x+ tan 3x)
Differentiating w.r.t. x, we get

(viii) \(\frac{1+\sin x^{\circ}}{1-\sin x^{\circ}}\)
Solution:

(ix) cot\(\left(\frac{\log x}{2}\right)\) – log\(\left(\frac{\cot x}{2}\right)\)
Solution:
Let y = cot\(\left(\frac{\log x}{2}\right)\) – log\(\left(\frac{\cot x}{2}\right)\)
Differentiating w.r.t. x, we get

(x) \(\frac{e^{2 x}-e^{-2 x}}{e^{2 x}+e^{-2 x}}\)
Solution:

(xi) \(\frac{e^{\sqrt{x}}+1}{e^{\sqrt{x}}-1}\)
Solution:
let y = \(\frac{e^{\sqrt{x}}+1}{e^{\sqrt{x}}-1}\)
Differentiating w.r.t. x, we get

(xii) log[tan³x·sin⁴x·(x² + 7)⁷]
Solution:
Let y = log [tan³x·sin⁴x·(x² + 7)⁷]
= log tan³x + log sin⁴x + log (x² + 7)⁷
= 3 log tan x + 4 log sin x + 7 log (x² + 7)
Differentiating w.r.t. x, we get

= 6cosec2x + 4 cotx + \(\frac{14 x}{x^{2}+7}\)

(xiii) log\(\left(\sqrt{\frac{1-\cos 3 x}{1+\cos 3 x}}\right)\)
Solution:

(xiv) log\(\left(\sqrt{\left.\frac{1+\cos \left(\frac{5 x}{2}\right)}{1-\cos \left(\frac{5 x}{2}\right)}\right)}\right.\)
Solution:
Using log\(\left(\frac{a}{b}\right)\) = log a – log b
log a^b = b log a


\(-\frac{5}{2}\)cosec\(\left(\frac{5 x}{2}\right)\)

(xv) log\(\left(\sqrt{\frac{1-\sin x}{1+\sin x}}\right)\)
Solution:

(xvi) log\(\left[4^{2 x}\left(\frac{x^{2}+5}{\sqrt{2 x^{3}-4}}\right)^{\frac{3}{2}}\right]\)
Solution:

(xvii) log\(\left[\frac{e^{x^{2}}(5-4 x)^{\frac{3}{2}}}{\sqrt[3]{7-6 x}}\right]\)
Solution:

(xviii) log\(\left[\frac{a^{\cos x}}{\left(x^{2}-3\right)^{3} \log x}\right]\)
Solution:

(xix) y= (25)^log₅(secx) − (16)^log₄(tanx)
Solution:
y = (25)^log₅(secx) − (16)^log₄(tanx)
= 5^{2log₅(secx)} – 4^{2log₄(tanx)}
= 5^{log₅(sec5x)} – 4^{log₄(tan2x)}
= sec²x – tan²x … [∵ = x]
∴ y = 1
Differentiating w.r.t. x, we get
\(\frac{d y}{d x}\) = \(\frac{d}{d x}\)(1) = 0

(xx) \(\frac{\left(x^{2}+2\right)^{4}}{\sqrt{x^{2}+5}}\)
Solution:
Let y = \(\frac{\left(x^{2}+2\right)^{4}}{\sqrt{x^{2}+5}}\)
Differentiating w.r.t. x, we get

Question 4.
A table of values of f, g, f ‘ and g’ is given

(i) If r(x) = f [g(x)] find r’ (2).
Solution:
r(x) = f[g(x)]
∴ r'(x) = \(\frac{d}{d x}\)f[g(x)]
= f'[g(x)]∙\(\frac{d}{d x}\)[g(x)]
= f'[g(x)∙[g'(x)]
∴ r'(2) = f'[g(2)]∙g'(2)
= f'(6)∙g'(2) … [∵ g(x) = 6, when x = 2]
= -4 × 4 … [From the table]
= -16.

(ii) If R(x) = g[3 + f(x)] find R’ (4).
Solution:
R(x) = g[3 + f(x)]
∴ R'(x) = \(\frac{d}{d x}\){g[3+f(x)]}
= g'[3 + f(x)]∙\(\frac{d}{d x}\)[3 + f(x)]
= g'[3 +f(x)]∙[0 + f'(x)]
= g'[3 + f(x)]∙f'(x)
∴ R'(4) = g'[3 + f(4)]∙f'(4)
= g'[3 + 3]∙f'(4) … [∵ f(x) = 3, when x = 4]
= g'(6)∙f'(4)
= 7 × 5 … [From the table]
= 35.

(iii) If s(x) = f[9− f(x)] find s’ (4).
Solution:
s(x) = f[9− f(x)]
∴ s'(x) = \(\frac{d}{d x}\){f[9 – f(x)]}
= f'[9 – f(x)]∙\(\frac{d}{d x}\)[0 – f(x)]
= f'[9 – f(x)]∙[0 – f'(x)]
= -f'[9 – f(x)] – f'(x)
∴ s'(4) = -f'[9 – f(4)] – f'(4)
= -f'[9 – 3] – f'(4) … [∵ f(x) = 3, when x = 4]
= -f'(6) – f'(4)
= -(-4)(5) … [From the table]
= 20.

(iv) If S(x) = g[g(x)] find S’ (6)
Solution:
S(x) = g[g(x)]
∴ S'(x) = \(\frac{d}{d x}\)g[g(x)]
= g'[g(x)]∙\(\frac{d}{d x}\)[g(x)]
= g'[g(x)]∙g'(x)
∴ S ‘(6) = g'[g'(6)]∙g'(6)
= g'(2)∙g'(6) … [∵ g (x) = 2, when x = 6]
= 4 × 7 … [From the table]
= 28.

Question 5.
Assume that f ‘(3) = -1, g'(2) = 5, g(2) = 3 and y = f[g(x)] then \(\left[\frac{d y}{d x}\right]_{x=2}\) = ?
Solution:
y = f[g(x)]
∴ \(\frac{d y}{d x}\) = \(\frac{d}{d x}\){[g(x)]}

Question 6.
If h(x) = \(\sqrt{4 f(x)+3 g(x)}\), f(1) = 4, g(1) = 3, f ‘(1) = 3, g'(1) = 4 find h'(1).
Solution:
Given f(1) = 4, g(1) = 3, f ‘(1) = 3, g'(1) = 4 …..(1)

Question 7.
Find the x co-ordinates of all the points on the curve y = sin 2x – 2 sin x, 0 ≤ x < 2π where \(\frac{d y}{d x}\) = 0.
Solution:
y = sin 2x – 2 sin x, 0 ≤ x < 2π

= cos2x × 2 – 2cosx
= 2 (2 cos²x – 1) – 2 cosx
= 4 cos²x – 2 – 2 cos x
= 4 cos²x – 2 cos x – 2
If \(\frac{d y}{d x}\) = 0, then 4 cos²x – 2 cos x – 2 = 0
∴ 4cos²x – 4cosx + 2cosx – 2 = 0
∴ 4 cosx (cosx – 1) + 2 (cosx – 1) = 0
∴ (cosx – 1)(4cosx + 2) = 0
∴ cosx – 1 = 0 or 4cosx + 2 = 0
∴ cos x = 1 or cos x = \(-\frac{1}{2}\)
∴ cos x = cos 0

Question 8.
Select the appropriate hint from the hint basket and fill up the blank spaces in the following paragraph. [Activity]
“Let f (x) = x² + 5 and g(x) = e^x + 3 then
f [g(x)] = _ _ _ _ _ _ _ _ and g [f(x)] =_ _ _ _ _ _ _ _.
Now f ‘(x) = _ _ _ _ _ _ _ _ and g'(x) = _ _ _ _ _ _ _ _.
The derivative off [g (x)] w. r. t. x in terms of f and g is _ _ _ _ _ _ _ _.
Therefore \(\frac{d}{d x}\)[f[g(x)]] = _ _ _ _ _ _ _ _ _ and [\(\frac{d}{d x}\)[f[g(x)]]]_{x = 0} = _ _ _ _ _ _ _ _ _ _ _.
The derivative of g[f(x)] w. r. t. x in terms of f and g is _ _ _ _ _ _ __ _ _ _ _.
Therefore \(\frac{d}{d x}\)[g[f(x)]] = _ _ _ _ _ _ _ _ _ and [\(\frac{d}{d x}\)[g[f(x)]]]_{x = 1} = _ _ _ _ _ _ _ _ _ _ _.”
Hint basket : { f ‘[g(x)]·g'(x), 2e^2x + 6e^x, 8, g'[f(x)]·f ‘(x), 2xe^{x2 + 5}, -2e⁶, e^2x + 6e^x + 14, e^{x2 + 5} + 3, 2x, e^x}
Solution:
f[g(x)] = e^2x + 6e^x + 14
g[f(x)] = e^{x2 + 5} + 3
f'(x) = 2x, g’f(x) = e^x
The derivative of f[g(x)] w.r.t. x in terms of and g is f'[g(x)]∙g'(x).
∴ \(\frac{d}{d x}\){f[g(x)]} = 2e^2x + 6e^x and \(\frac{d}{d x}\){f[g(x)]}_{x = 0} = 8
The derivative of g[f(x)] w.r.t. x in terms of f and g is g’f(x)]∙f'(x).
∴ \(\frac{d}{d x}\){g[(f(x)]} = 2xe^{x2 + 5} and
\(\frac{d}{d x}\){g[(f(x)]}_{x = -1} = -2e⁶.

Balbharti 12th Maharashtra State Board Maths Solutions Book Pdf Chapter 2 Applications of Derivatives Ex 2.4 Questions and Answers.

Maharashtra State Board 12th Maths Solutions Chapter 2 Applications of Derivatives Ex 2.4

Question 1.
Test whether the following functions are increasing or decreasing.
(i) f(x) = x³ – 6x² + 12x – 16, x ∈ R.
Solution:
f(x) = x³ – 6x² + 12x – 16
∴ f'(x) = \(\frac{d}{d x}\) (x³ – 6x² + 12x – 16)
= 3x² – 6 × 2x + 12 × 1 – 0
= 3x² – 12x + 12
= 3(x² – 4x + 4)
= 3(x – 2)² ≥ 0 for all x ∈ R
∴ f(x) ≥ 0 for all x ∈ R
∴ f is increasing for all x ∈ R.

(ii) f(x) = 2 – 3x + 3x² – x³, x ∈ R.
Solution:
f(x) = 2 – 3x + 3x² – x³
∴ f'(x) = \(\frac{d}{d x}\) (2 – 3x + 3x² – x³)
= 0 – 3 × 1 + 3 × 2x – 3x²
= -3 + 6x – 3x²
= -3(x² – 2x + 1)
= -3(x – 1)² ≤ 0 for all x ∈ R
∴ f'(x) ≤ 0 for all x ∈ R
∴ f is decreasing for all x ∈ R.

(iii) f(x) = x – \(\frac{1}{x}\), x ∈ R, x ≠ 0.
Solution:
f(x) = x – \(\frac{1}{x}\)
f'(x) = \(\frac{d}{d x}\left(x-\frac{1}{x}\right)=1-\left(\frac{-1}{x^{2}}\right)\)
= \(1+\frac{1}{x^{2}}\) > 0 for all x ∈ R, x ≠ 0
∴ f'(x) > 0 for all x ∈ R, where x ≠ 0
∴ f is increasing for all x ∈ R, where x ≠ 0.

Question 2.
Find the values of x for which the following functions are strictly increasing:
(i) f(x) = 2x³ – 3x² – 12x + 6
Solution:
f(x) = 2x³ – 3x² – 12x + 6
∴ f'(x) = \(\frac{d}{d x}\) (2x³ – 3x² – 12x + 6)
= 2 × 3x² – 3 × 2x – 12 × 1 + 0
= 6x² – 6x – 12
= 6(x² – x – 2)
f is strictly increasing if f'(x) > 0
i.e. if 6(x² – x – 2) > 0
i.e. if x² – x – 2 > 0
i.e. if x² – x > 2
i.e. if x² – x + \(\frac{1}{4}\) > 2 + \(\frac{1}{4}\)
i.e. if \(\left(x-\frac{1}{2}\right)^{2}>\frac{9}{4}\)
i.e. if x – \(\frac{1}{2}\) > \(\frac{3}{2}\) or x – \(\frac{1}{2}\) < \(\frac{-3}{2}\) i.e. if x > 2 or x < -1
∴ f is strictly increasing if x < -1 or x > 2.

(ii) f(x) = 3 + 3x – 3x² + x³
Solution:
f(x) = 3 + 3x – 3x² + x³
∴ f'(x) = \(\frac{d}{d x}\) (3 + 3x – 3x² + x³)
= 0 + 3 × 1 – 3 × 2x + 3x²
= 3 – 6x + 3x²
= 3(x² – 2x + 1)
f is strictly increasing if f'(x) > 0
i.e. if 3(x² – 2x + 1) > 0
i.e. if x² – 2x + 1 > 0
i.e. if (x – 1)² > 0
This is possible if x ∈ R and x ≠ 1
i.e. x ∈ R – {1}
∴ f is strictly increasing if x ∈ R – {1}.

(iii) f(x) = x³ – 6x² – 36x + 7
Solution:
f(x) = x³ – 6x² – 36x + 7
∴ f'(x) = \(\frac{d}{d x}\) (x³ – 6x² – 36x + 7)
= 3x² – 6 × 2x – 36 × 1 + 0
= 3x² – 12x – 36
= 3(x² – 4x – 12)
f is strictly increasing if f'(x) > 0
i.e. if 3(x² – 4x – 12) > 0
i.e. if x² – 4x – 12 > 0
i.e. if x² – 4x > 12
i.e. if x² – 4x + 4 > 12 + 4
i.e. if (x – 2)² > 16
i.e. if x – 2 > 4 or x – 2 < -4 i.e. if x > 6 or x < -2
∴ f is strictly increasing if x < -2 or x > 6.

Question 3.
Find the values of x for which the following functions are strictly decreasiong:
(i) f(x) = 2x³ – 3x² – 12x + 6
Solution:
f(x) = 2x³ – 3x² – 12x + 6
∴ f'(x) = \(\frac{d}{d x}\) (2x³ – 3x² – 12x + 6)
= 2 × 3x² – 3 × 2x – 12 × 1 + 0
= 6x² – 6x – 12
= 6(x² – x – 2)
f is strictly decreasing if f'(x) < 0
i.e. if 6(x² – x – 2) < 0
i.e. if x² – x – 2 < 0
i.e. if x² – x < 2
i.e. if x² – x + \(\frac{1}{4}\) < 2 + \(\frac{1}{4}\)
i.e. if \(\left(x-\frac{1}{2}\right)^{2}<\frac{9}{4}\)
i.e. if \(-\frac{3}{2}<x-\frac{1}{2}<\frac{3}{2}\)
i.e. if \(-\frac{3}{2}+\frac{1}{2}<x-\frac{1}{2}+\frac{1}{2}<\frac{3}{2}+\frac{1}{2}\)
i.e. if -1 < x < 2
∴ f is strictly decreasing if -1 < x < 2.

(ii) f(x) = x + \(\frac{25}{x}\)
Solution:
f(x) = x + \(\frac{25}{x}\), x ≠ 0
∴ f'(x) = \(\frac{d}{d x}\left(x+\frac{25}{x}\right)\)
= 1 + 25(-1) x^-2
= 1 – \(\frac{25}{x^{2}}\)
f is is strictly decreasing if f'(x) < 0
i.e. if 1 – \(\frac{25}{x^{2}}\) < 0
i.e. if 1 < \(\frac{25}{x^{2}}\)
i.e. if x² < 25
i.e. if -5 < x < 5, x ≠ 0
i.e. if x ∈ (-5, 5) – {0}
∴ f is strictly decreasing if x ∈ (-5, 5) – {0}.

(iii) f(x) = x³ – 9x² + 24x + 12
Solution:
f(x) = x³ – 9x² + 24x + 12
∴ f'(x) = \(\frac{d}{d x}\) (x³ – 9x² + 24x + 12)
= 3x² – 9 × 2x + 24 × 1 + 0
= 3x² – 18x + 24
= 3(x² – 6x + 8)
f is strictly decreasing if f'(x) < 0
i.e. if 3(x² – 6x + 8) < 0
i.e. if x² – 6x + 8 < 0
i.e. if x² – 6x < -8
i.e. if x² – 6x + 9 < -8 + 9
i.e. if (x – 3)² < 1
i.e. if -1 < x – 3 < 1
i.e. if -1 + 3 < x – 3 + 3 < 1 + 3
i.e. if 2 < x < 4
i.e., if x ∈ (2, 4)
∴ f is strictly decreasing if x ∈ (2, 4)

Question 4.
Find the values of x for which the function f(x) = x³ – 12x² – 144x + 13
(a) increasing
(b) decreasing.
Solution:
f(x) = x³ – 12x² – 144x + 13
∴ f'(x) = \(\frac{d}{d x}\) (x³ – 12x² – 144x + 13)
= 3x² – 12 × 2x – 144 × 1 + 0
= 3x² – 24x – 144
= 3(x² – 8x – 48)

(a) f is increasing if f'(x) ≥ 0
i.e. if 3(x² – 8x – 48) ≥ 0
i.e. if x² – 8x – 48 ≥ 0
i.e. if x² – 8x ≥ 48
i.e. if x² – 8x + 16 ≥ 48 + 16
i.e. if (x – 4)² ≥ 64
i.e. if x – 4 ≥ 8 or x – 4 ≤ -8
i.e. if x > 12 or x ≤ -4
∴ f is increasing if x ≤ -4 or x ≥ 12,
i.e. x ∈ (-∞, -4] ∪ [12, ∞).

(b) f is decreasing if f'(x) ≤ 0
i.e. if 3(x² – 8x – 48) ≤ 0
i.e. if x² – 8x – 48 ≤ 0
i.e. if x² – 8x ≤ 48
i.e. if x² – 8x + 16 ≤ 48 + 16
i.e. if (x – 4)² ≤ 64
i.e. if -8 ≤ x – 4 ≤ 8
i.e. if -4 ≤ x ≤ 12
∴ f is decreasing if -4 ≤ x ≤ 12, i.e. x ∈ [-4, 12].

Question 5.
Find the values of x for which f(x) = 2x³ – 15x² – 144x – 7 is
(a) strictly increasing
(b) strictly decreasing.
Solution:
f(x) = 2x³ – 15x² – 144x – 7
f'(x) = \(\frac{d}{d x}\) (2x³ – 15x² – 144x – 7)
= 2 × 3x² – 15 × 2x – 144 × 1 – 0
= 6x² – 30x – 144
= 6(x² – 5x – 24)
(a) f is strictly increasing if f'(x) > 0
i.e. if 6(x² – 5x – 24) > 0
i.e. if x² – 5x – 24 > 0
i.e. if x² – 5x > 24
i.e. if x² – 5x + \(\frac{25}{4}\) > 24 + \(\frac{25}{4}\)
i.e. if \(\left(x-\frac{5}{2}\right)^{2}>\frac{121}{4}\)
i.e. if \(x-\frac{5}{2}>\frac{11}{2} \text { or } x-\frac{5}{2}<-\frac{11}{2}\) i.e. if x > 8 or x < -3
∴ f is strictly increasing, if x < -3 or x > 8.

(b) f is strictly decreasing if f'(x) < 0
i.e. if 6(x² – 5x – 24) < 0
i.e. if x² – 5x – 24 < 0
i.e. if x² – 5x < 24
i.e. if x² – 5x + \(\frac{25}{4}\) < 24 + \(\frac{25}{4}\)
i.e. if \(\left(x-\frac{5}{2}\right)^{2}<\frac{121}{4}\)
i.e. if \(-\frac{11}{2}<x-\frac{5}{2}<\frac{11}{2}\)
i.e. if \(-\frac{11}{2}+\frac{5}{2}<x-\frac{5}{2}+\frac{5}{2}<\frac{11}{2}+\frac{5}{2}\)
i.e. if -3 < x < 8
∴ f is strictly decreasing, if -3 < x < 8.

Question 6.
Find the values of x for which f(x) = \(\frac{\boldsymbol{x}}{x^{2}+1}\) is
(a) strictly increasing
(b) strictly decreasing.
Solution:
f(x) = \(\frac{\boldsymbol{x}}{x^{2}+1}\)

(a) f is strictly increasing if f'(x) > 0
i.e. if \(\frac{1-x^{2}}{\left(x^{2}+1\right)^{2}}\) > 0
i.e. if 1 – x² > 0 ……..[∵ (x² + 1)² > 0]
i.e. if 1 > x²
i.e. if x² < 1
i.e. if -1 < x < 1
∴ f is strictly increasing if -1 < x < 1

(b) f is strictly decreasing if f'(x) < 0
i.e. if \(\frac{1-x^{2}}{\left(x^{2}+1\right)^{2}}\) < 0
i.e. if 1 – x² < 0 ……..[∵ (x² + 1)² > 0]
i.e. if 1 < x² i.e. if x² > 1
i.e. if x > 1 or x < -1
∴ f is strictly decreasing if x < -1 or x > 1
i.e. x ∈ (-∞, -1) ∪ (1, ∞).

Question 7.
Show that f(x) = 3x + \(\frac{1}{3 x}\) is increasing in (\(\frac{1}{3}\), 1) and decreasing in (\(\frac{1}{9}\), \(\frac{1}{3}\))
Solution:
f(x) = 3x + \(\frac{1}{3 x}\)

Question 8.
Show that f(x) = x – cos x is increasing for all x.
Solution:
f(x) = x – cos x
∴ f'(x) = \(\frac{d}{d x}\) (x – cos x)
= 1 – (-sin x)
= 1 + sin x
Now, -1 ≤ sin x ≤ 1 for all x ∈ R
∴ -1 + 1 ≤ 1 + sin x ≤ 1 for all x ∈ R
∴ 0 ≤ f'(x) ≤ 1 for all x ∈ R
∴ f'(x) ≥ 0 for all x ∈ R
∴ f is increasing for all x.

Question 9.
Find the maximum and minimum of the following functions:
(i) y = 5x³ + 2x² – 3x
Solution:

(ii) f(x) = 2x³ – 21x² + 36x – 20
Solution:
f(x) = 2x³ – 21x² + 36x – 20
∴ f'(x) = \(\frac{d}{d x}\) (2x³ – 21x² + 36x – 20)
= 2 × 3x² – 21 × 2x + 36 × 1 – 0
= 6x² – 42x + 36
and f”(x) = \(\frac{d}{d x}\) (6x² – 42x + 36)
= 6 × 2x – 42 × 1 + 0
= 12x – 42
f'(x) = 0 gives 6x² – 42x + 36 = 0
∴ x² – 7x + 6 = 0
∴ (x – 1)(x – 6) = 0
the roots of f'(x) = 0 are x₁ = 1 and x₂ = 6.

Method 1 (Second Derivative Test):
(a) f”(1) = 12(1) – 42 = -30 < 0
∴ by the second derivative test, f has maximum at x = 1
and maximum value of f at x = 1
f(1) = 2(1)³ – 21(1)² + 36(1) – 20
= 2 – 21 + 36 – 20
= -3

(b) f”(6) = 12(6) – 42 = 30 > 0
∴ by the second derivative test, f has minimum at x = 6
and minimum value of f at x = 6
f(6) = 2(6)³ – 21(6)² + 36(6) – 20
= 432 – 756 + 216 – 20
= -128.
Hence, the function f has maximum value -3 at x = 1 and minimum value -128 at x = 6.

Method 2 (First Derivative Test):
(a) f'(x) = 6(x – 1)(x – 6)
Consider x = 1
Let h be a small positive number. Then
f'(1 – h) = 6(1 – h – 1)(1 – h – 6)
= 6(-h)(-5 – h)
= 6h(5 + h)> 0
and f'(1 + h) = 6(1 + h – 1)(1 + h – 6)
= 6h(h – 5) < 0, as h is small positive number.
∴ by the first derivative test, f has maximum at x = 1 and maximum value of f at x = 1
f(1) = 2(1)³ – 21(1)² + 36(1) – 20
= 2 – 21 + 36 – 20
= -3

(b) f'(x) = 6(x – 1)(x – 6)
Consider x = 6
Let h be a small positive number. Then
f'(6 – h) = 6(6 – h – 1)(6 – h – 6)
= 6(5 – h)(-h)
= -6h(5 – h) < 0, as h is small positive number
and f'(6 + h) = 6(6 + h – 1)(6 + h – 6) = 6(5 + h)(h) > 0
∴ by the first derivative test, f has minimum at x = 6
and minimum value of f at x = 6
f(6) = 2(6)³ – 21(6)² + 36(6) – 20
= 432 – 756 + 216 – 20
= -128
Hence, the function f has maximum value -3 at x = 1
and minimum value -128 at x = 6.

(iii) f(x) = x³ – 9x² + 24x
Solution:
f(x) = x³ – 9x² + 24x
∴ f'(x) = \(\frac{d}{d x}\) (x³ – 9x² + 24x)
= 3x² – 9 × 2x + 24 × 1
= 3x² – 18x + 24
and f”(x) = \(\frac{d}{d x}\) (3x² – 18x + 24)
= 3 × 2x – 18 × 1 + 0
= 6x – 18
f'(x) = 0 gives 3x² – 18x + 24 = 0
∴ x² – 6x + 8 = 0
∴ (x – 2)(x – 4) = 0
∴ the roots of f'(x) = 0 are x₁ = 2 and x₂ = 4.

(a) f”(2) = 6(2) – 18 = -6 < 0
∴ by the second derivative test, f has maximum at x = 2
and maximum value of f at x = 2
f(2) = (2)³ – 9(2)² + 24(2)
= 8 – 36 + 48
= 20

(b) f”(4) = 6(4) – 18 = 6 > 0
∴ by the second derivative test, f has minimum at x = 4
and minimum value of f at x = 4
f(4) = (4)³ – 9(4)² + 24(4)
= 64 – 144 + 96
= 16
Hence, the function f has maximum value 20 at x = 2 and minimum value 16 at x = 4.

(iv) f(x) = x² + \(\frac{16}{x^{2}}\)
Solution:

(v) f(x) = x log x
Solution:

(vi) f(x) = \(\frac{\log x}{x}\)
Solution:

Question 10.
Divide the number 30 into two parts such that their product is maximum.
Solution:
Let the first part of 30 be x.
Then the second part is 30 – x.
∴ their product = x(30 – x) = 30x – x² = f(x) ……(Say)
∴ f'(x) = \(\frac{d}{d x}\) (30x – x²)
= 30 × 1 – 2x
= 30 – 2x
and f”(x) = \(\frac{d}{d x}\) (30 – 2x)
= 0 – 2 × 1
= -2
The root of the equation f(x) = 0,
i.e. 30 – 2x = 0 is x = 15 and f”(15) = -2 < 0
∴ by the second derivative test, f is maximum at x = 15.
Hence, the required parts of 30 are 15 and 15.

Question 11.
Divide the number 20 into two parts such that the sum of their squares is minimum.
Solution:
Let the first part of 20 be x.
Then the second part is 20 – x.
∴ sum of their squares = x² + (20 – x)² = f(x) …… (Say)
∴ f'(x) = \(\frac{d}{d x}\) [x² + (20 – x)²]
= 2x + 2(20 – x) . \(\frac{d}{d x}\) (20 – x)
= 2x + 2(20 – x) × (0 – 1)
= 2x – 40 + 2x
= 4x – 40
and f”(x) = \(\frac{d}{d x}\) (4x – 40)
= 4 × 1 – 0
= 4
The root of the equation f'(x) = 0,
i.e. 4x – 40 = 0 is x = 10 and f”(10) = 4 > 0
∴ by the second derivative test, f is minimum at x = 10.
Hence, the required parts of 20 are 10 and 10.

Question 12.
A wire of length 36 meters is bent in the form of a rectangle. Find its dimensions if the area of the rectangle is maximum.
Solution:
Let x metres and y metres be the length and breadth of the rectangle.
Then its perimeter is 2(x + y) = 36
x + y = 18
y = 18 – x
Area of the rectangle = xy = x (18 – x)
Let f(x) = x(18 – x) = 18x – x²
∴ f'(x) = \(\frac{d}{d x}\) (18x – x²) = 18 – 2x
and f”(x) = \(\frac{d}{d x}\) (18 – 2x) = 0 – 2 × 1 = -2
Now, f'(x) = 0, if 18 – 2x = 0
i.e. if x = 9
and f”(9) = -2 < 0
∴ by the second derivative test, f has maximum value at x = 9.
When x = 9, y = 18 – 9 = 9
∴ x = 9 cm, y = 9 cm
∴ the rectangle is a square of side 9 metres.

Question 13.
A ball is thrown in the air. Its height at any time t is given by h = 3 + 14t – 5t². Find the maximum height it can reach.
Solution:
The height h at any t is given by h = 3 + 14t – 5t²


Hence, the maximum height the ball can reach = 12.8 units.

Question 14.
Find the largest size of a rectangle that can be inscribed in a semicircle of radius 1 unit, so that two vertices lie on the diameter.
Solution:

Let ABCD be the rectangle inscribed in a semicircle of radius 1 unit such that the vertices A and B lie on the diameter.
Let AB = DC = x and BC = AD = y.
Let O be the centre of the semicircle.
Join OC and OD. Then OC = OD = radius = 1.
Also, AD = BC and m∠A = m∠B = 90°.
∴ OA = OB
∴ OB = \(\frac{1}{2}\) AB = \(\frac{x}{2}\)
In right angled triangle OBC,
OB² + BC² = OC²




Hence, the area of the rectangle is maximum (i.e. rectangle has the largest size) when its length is √2 units and breadth is \(\frac{1}{\sqrt{2}}\) unit.

Question 15.
An open cylindrical tank whose base is a circle is to be constructed of metal sheet so as to contain a volume of πa³ cu cm of water. Find the dimensions so that the quantity of the metal sheet required is minimum.
Solution:
Let x be the radius of the base, h be the height, V be the volume and S be the total surface area of the cylindrical tank.
Then V = πa³ … (Given)
∴ πx²h = πa³
∴ h = \(\frac{a^{3}}{x^{2}}\) ……..(1)
Now, S = 2πxh + πx²

∴ by the second derivative test, S is minimum when x = a
When x = a, from (1)
h = \(\frac{a^{3}}{a^{2}}\) = a
Hence, the quantity of metal sheet is minimum when radius height = a cm.

Question 16.
The perimeter of a triangle is 10 cm. If one of the sides is 4 cm. What are the other two sides of the triangle for its maximum area?
Solution:

Let ABC be the triangle such that the side BC = a = 4 cm.
Also, the perimeter of the triangle is 10 cm.
i.e. a + b + c = 10
∴ 2s = 10
∴ s = 5
Also, 4 + b + c = 10
∴ b + c = 6
∴ b = 6 – c
Let ∆ be the area of the triangle.



∴ by the second derivative test, ∆ is maximum when c = 3.
When c = 3, b = 6 – c = 6 – 3 = 3
Hence, the area of the triangle is maximum when the other two sides are 3 cm and 3 cm.

Question 17.
A box with a square base is to have an open top. The surface area of the box is 192 sq cm. What should be its dimensions in order that the volume is largest?
Solution:
Let x cm be the side of square base and h cm be its height.
Then x² + 4xh = 192
∴ h = \(\frac{192-x^{2}}{4 x}\) …… (1)
Let V be the volume of the box.

∴ by the second derivative test, V is maximum at x = 8.
If x = 8, h = \(\frac{192-64}{4(8)}=\frac{128}{32}\) = 4
Hence, the volume of the box is largest, when the side of square base is 8 cm and its height is 4 cm.

Question 18.
The profit function P (x) of a firm, selling x items per day is given by P(x) = (150 – x)x – 1625. Find the number of items the firm should manufacture to get maximum profit. Find the maximum profit.
Solution:
Profit function P (x) is given by
P(x) = (150 – x)x – 1625 = 150x – x² -1625
∴ P'(x) = \(\frac{d}{d x}\) (150x – x² – 1625)
= 150 × 1 – 2x – 0
= 150 – 2x
and P”(x) = \(\frac{d}{d x}\) (150 – 2x)
= 0 – 2 × 1
= -2
Now, P'(x) = 0 gives, 150 – 2x = 0
∴ x = 75
and P”(75) = -2 < 0
∴ by the second derivative test, P(x) is maximum when x = 75
Maximum profit = P(75)
= (150 – 75)75 – 1625
= 75 × 75 – 1625
= 4000
Hence, the profit will be maximum, if the manufacturer manufactures 75 items and the maximum profit is 4000.

Question 19.
Find two numbers whose sum is 15 and when the square of one multiplied by the cube of the other is maximum.
Solution:
Let the two numbers be x and y.
Then x + y = 15
∴ y = 15 – x
Let P is the product of square of y and cube of x.
Then P = x³y²
= x³(15 – x)²
= x³(225 – 30x + x²)
= x⁵ – 30x⁴ + 225x³
∴ \(\frac{d P}{d x}\) = \(\frac{d}{d x}\) (x⁵ – 30x⁴ + 225x³)
= 5x⁴ – 30 × 4x³ + 225 × 3x²
= 5x⁴ – 120x³ + 675x²
and \(\frac{d^{2} P}{d x^{2}}\) = \(\frac{d}{d x}\) (5x⁴ – 120x³ + 675x²)
= 5 × 4x³ – 120 × 3x² + 675 × 2x
= 20x³ – 360x² + 1350x
= 10x(2x² – 36x + 135)
Now, \(\frac{d P}{d x}\) = 0 gives 5x⁴ – 120x³ + 675x² = 0
∴ 5x²(x² – 24x +135) = 0
∴ 5x²(x² – 15x – 9x + 135) = 0
∴ 5x²[x(x – 15) – 9(x – 35)] = 0
∴ 5x²(x – 15)(x – 9) = 0
∴ the roots of \(\frac{d P}{d x}\) = 0 are x₁ = 0, x₂ = 15 and x₃ = 9
If x = 0, then y = 15 – 0 = 15
If x = 15, then y = 15 – 15 = 0
In both cases, product x³y² is zero, which is not maximum.
∴ x ≠ 0 and x ≠ 15
∴ x = 6
Now, \(\left(\frac{d^{2} P}{d x^{2}}\right)_{\text {at } x=6}\) = 10(6)[2(6)² – 36 × 6 + 135]
= 60[72 – 216 + 135]
= 60(-9)
= -540 < 0
∴ P is maximum when x = 6
If x = 6, then y = 15 – 6 = 9
Hence, the required numbers are 6 and 9.

Question 20.
Show that among rectangles of given area, the square has least perimeter.
Solution:
Let x be the length and y be the breadth of the rectangle whose area is A sq units (which is given as constant).
Then xy = A
∴ y = \(\frac{A}{x}\) ………(1)
Let P be the perimeter of the rectangle.

x = y
∴ rectangle is a square.
Hence, among rectangles of given area, the square has least perimeter.

Question 21.
Show that the height of a closed right circular cylinder of given volume and least surface area is equal to its diameter.
Solution:
Let x be the radius of base, h be the height and S be the surface area of the closed right circular cylinder whose volume is V which is given to be constant.
Then πr²h = V
∴ h = \(\frac{V}{\pi r^{2}}=\frac{A}{x^{2}}\) …….(1)
where A = \(\frac{V}{\pi}\), which is constant.


Hence, the surface area is least when height of the closed right circular cylinder is equal to its diameter.

Question 22.
Find the volume of the largest cylinder that can be inscribed in a sphere of radius ‘r’ cm.
Solution:
Let R be the radius and h be the height of the cylinder which is inscribed in a sphere of radius r cm.
Then from the figure,


Hence, the volume of the largest cylinder inscribed in a sphere of radius ‘r’ cm = \(\frac{4 \pi r^{3}}{3 \sqrt{3}}\) cu cm.

Question 23.
Show that y = log(1 + x) – \(\frac{2 x}{2+x}\), x > -1 is an increasing function on its domain.
Solution:
y = log(1 + x) – \(\frac{2 x}{2+x}\), x > -1

Hence, the given function is increasing function on its domain.

Question 24.
Prove that y = \(\frac{4 \sin \theta}{2+\cos \theta}\) – θ is an increasing function if θ ∈ [0, \(\frac{\pi}{2}\)]
Solution:
y = \(\frac{4 \sin \theta}{2+\cos \theta}\) – θ

Balbharti 12th Maharashtra State Board Maths Solutions Book Pdf Chapter 2 Applications of Derivatives Ex 2.3 Questions and Answers.

Maharashtra State Board 12th Maths Solutions Chapter 2 Applications of Derivatives Ex 2.3

Question 1.
Check the validity of the Rolle’s theorem for the following functions.
(i) f(x) = x² – 4x + 3, x ∈ [1, 3]
Solution:
The function f given as f(x) = x² – 4x + 3 is polynomial function.
Hence, it is continuous on [1, 3] and differentiable on (1, 3).
Now, f(1) = 1² – 4(1) + 3 = 1 – 4 + 3 = 0
and f(3) = 3² – 4(3) + 3 = 9 – 12 + 3 = 0
∴ f(1) = f(3)
Thus, the function f satisfies all the conditions of Rolle’s theorem.

(ii) f(x) = e^-x sin x, x ∈ [0, π].
Solution:
The functions e^-x and sin x are continuous and differentiable on their domains.
∴ f(x) = e^-x sin x is continuous on [0, π] and differentiable on (0, π).
Now, f(0) = e⁰ sin 0 = 1 × 0 = 0
and f(π) = e^-π sin π = e^-π × 0 = 0
∴ f(0) = f(π)
Thus, the function f satisfies all the conditions of the Rolle’s theorem.

(iii) f(x) = 2x² – 5x + 3, x ∈ [1, 3].
Solution:
The function f given as f(x) = 2x² – 5x + 3 is a polynomial function.
Hence, it is continuous on [1, 3] and differentiable on (1, 3).
Now, f(1) = 2(1)² – 5(1) + 3 = 2 – 5 + 3 = 0
and f(3) = 2(3)² – 5(3) + 3 = 18 – 15 + 3 = 6
∴ f(1) ≠ f(3)
Hence, the conditions of Rolle’s theorem are not satisfied.

(iv) f(x) = sin x – cos x + 3, x ∈ [0, 2π].
Solution:
The functions sin x, cos x and 3 are continuous and differentiable on their domains.
∴ f(x) = sin x – cos x + 3 is continuous on [0, 2π] and differentiable on (0, 2π).
Now, f(0) = sin 0 – cos 0 + 3 = 0 – 1 + 3 = 2
and f(2π) = sin 2π – cos 2π + 3 = 0 – 1 + 3 = 2
∴ f(0) = f(2π)
Thus, the function f satisfies all the conditions of the Rolle’s theorem.

(v) f(x) = x², if 0 ≤ x ≤ 2
= 6 – x, if 2 < x ≤ 6.
Solution:
f(x) = x², if 0 ≤ x ≤ 2
= 6 – x, if 2 < x ≤ 6
∴ f(x) = \(\frac{d}{d x}\left(x^{2}\right)\) = 2x, if 0 ≤ x ≤ 2
= \(\frac{d}{d x}(6-x)\) = -1, if 2 < x ≤ 6
∴ Lf'(2) = 2(2) = 4 and Rf'(2) = -1
∴ Lf'(2) ≠ Rf'(2)
∴ f is not differentiable at x = 2 and 2 ∈ (0, 6).
∴ f is not differentiable at all the points on (0, 6).
Hence, the conditions of Rolle’s theorem are not satisfied.

(vi) f(x) = \(x^{\frac{2}{3}}\), x ∈ [-1, 1].
Solution:
f(x) = \(x^{\frac{2}{3}}\)
∴ \(f^{\prime}(x)=\frac{d}{d x}\left(x^{\frac{2}{3}}\right)=\frac{2}{3} x^{-\frac{1}{3}}\) = \(\frac{2}{3 \sqrt[3]{x}}\)
This does not exist at x = 0 and 0 ∈ (-1, 1)
∴ f is not differentiable on the interval (-1, 1).
Hence, the conditions of Rolle’s theorem are not satisfied.

Question 2.
Given an interval [a, b] that satisfies hypothesis of Rolle’s theorem for the function f(x) = x⁴ + x² – 2. It is known that a = -1. Find the value of b.
Solution:
f(x) = x⁴ + x² – 2
Since the hypothesis of Rolle’s theorem are satisfied by f in the interval [a, b], we have
f(a) = f(b), where a = -1
Now, f(a) = f(-1) = (-1)⁴ + (-1)² – 2 = 1 + 1 – 2 = 0
and f(b) = b⁴ + b² – 2
∴ f(a) = f(b) gives
0 = b⁴ + b² – 2 i.e. b⁴ + b² – 2 = 0.
Since, b = 1 satisfies this equation, b = 1 is one of the roots of this equation.
Hence, b = 1.

Question 3.
Verify Rolle’s theorem for the following functions.
(i) f(x) = sin x + cos x + 7, x ∈ [0, 2π]
Solution:
The functions sin x, cos x and 7 are continuous and differentiable on their domains.
∴ f(x) = sin x + cos x + 7 is continuous on [0, 2π] and differentiable on (0, 2π)
Now, f(0) = sin 0 + cos 0 + 7 = 0 + 1 + 7 = 8
and f(2π) = sin 2π + cos 2π + 7 = 0 + 1 + 7 = 8
∴ f(0) = f(2π)
Thus, the function f satisfies all the conditions of Rolle’s theorem.
∴ there exists c ∈ (0, 2π) such that f'(c) = 0.
Now, f(x) = sin x + cos x + 7
∴ f'(x) = \(\frac{d}{d x}\) (sin x + cos x + 7)
= cos x – sin x + 0
= cos x – sin x
∴ f'(c) = cos c – sin c
∴ f'(c) = 0 gives, cos c – sin c = 0
∴ cos c = sin c
∴ c = \(\frac{\pi}{4}, \frac{5 \pi}{4}, \frac{9 \pi}{4}, \ldots\)
But \(\frac{\pi}{4}, \frac{5 \pi}{4}\) ∈ (0, 2π)
∴ c = \(\frac{\pi}{4} \text { or } \frac{5 \pi}{4}\)
Hence, the Rolle’s theorem is verified.

(ii) f(x) = sin(\(\frac{x}{2}\)), x ∈ [0, 2π]
Solution:
The function f(x) = sin(\(\frac{x}{2}\)) is continuous on [0, 2π] and differentiable on (0, 2π).
Now, f(0) = sin 0 = 0
and f(2π) = sin π = 0
∴ f(0) = f(2π)
Thus, the function f satisfies all the conditions of Rolle’s theorem.
∴ there exists c ∈ (0, 2π) such that f'(c) = 0.


Hence, Rolle’s theorem is verified.

(iii) f(x) = x² – 5x + 9, x ∈ [1, 4].
Solution:
The function f given as f(x) = x² – 5x + 9 is a polynomial function.
Hence it is continuous on [1, 4] and differentiable on (1, 4).
Now, f(1) = 1² – 5(1) + 9 = 1 – 5 + 9 = 5
and f(4) = 4² – 5(4) + 9 = 16 – 20+ 9 = 5
∴ f(1) = f(4)
Thus, the function f satisfies all the conditions of the Rolle’s theorem.
∴ there exists c ∈ (1, 4) such that f'(c) = 0.
Now, f(x) = x2 – 5x + 9
∴ f'(x) = \(\frac{d}{d x}\) (x² – 5x + 9)
= 2x – 5 × 1 + 0
= 2x – 5
∴ f'(c) = 2c – 5
∴ f'(c) = 0 gives, 2c – 5 = 0
∴ c = 5/2 ∈ (1, 4)
Hence, the Rolle’s theorem is verified.

Question 4.
If Rolle’s theorem holds for the function f(x) = x³ + px² + qx + 5, x ∈ [1, 3] with c = 2 + \(\frac{1}{\sqrt{3}}\), find the values of p and q.
Solution:
The Rolle’s theorem holds for the function f(x) = x³ + px² + qx + 5, x ∈ [1, 3]
∴ f(1) = f(3)
∴ 1³ + p(1)² + q(1) + 5 = 3³ + p (3)² + q(3) + 5
∴ 1 + p + q + 5 = 27 + 9p + 3q + 5
∴ 8p + 2q = -26
∴ 4p + q = -13 ….. (1)
Also, there exists at least one point c ∈ (1, 3) such that f'(c) = 0.
Now, f'(x) = \(\frac{d}{d x}\) (x³ + px² + qx + 5)
= 3x² + p × 2x + q × 1 + 0
= 3x² + 2px + q

But f'(c) = 0
∴ \(4 p+\frac{2 p}{\sqrt{3}}+q+13+\frac{12}{\sqrt{3}}=0\)
∴ (4√3 + 2)p + √3q + (13√3 + 12) = 0
∴ (4√3 + 2)p + √3q = -13√3 – 12 ……. (2)
Multiplying equation (1) by √3, we get
4√3p + √3q= -13√3
Subtracting this equation from (2), we get
2p = -12 ⇒ p= -6
∴ from (1), 4(-6) + q = -13 ⇒ q = 11
Hence, p = -6 and q = 11.

Question 5.
If Rolle’s theorem holds for the function f(x) = (x – 2) log x, x ∈ [1, 2], show that the equation x log x = 2 – x is satisfied by at least one value of x in (1, 2).
Solution:
The Rolle’s theorem holds for the function f(x) = (x – 2) log x, x ∈ [1, 2].
∴ there exists at least one real number c ∈ (1, 2) such that f'(c) = 0.
Now, f(x) = (x – 2) log x

∴ f'(c) = 0 gives 1 – \(\frac{2}{c}\) + log c = 0
∴ c – 2 + c log c = 0
∴ c log c = 2 – c, where c ∈ (1, 2)
∴ c satisfies the equation x log x = 2 – x, c ∈ (1, 2).
Hence, the equation x log x = 2 – x is satisfied by at least one value of x in (1, 2).

Question 6.
The function f(x) = \(x(x+3) e^{-\frac{x}{2}}\) satisfies all the conditions of Rolle’s theorem on [-3, 0]. Find the value of c such that f'(c) = 0.
Solution:
The function f(x) satisfies all the conditions of Rolle’s theorem, therefore there exist c ∈ (-3, 0) such that f'(c) = 0.

Question 7.
Verify Lagrange’s mean value theorem for the following functions:
(i) f(x) = log x on [1, e].
Solution:
The function f given as f(x) = log x is a logarithmic function that is continuous for all positive real numbers.
Hence, it is continuous on [1, e] and differentiable on (1, e).
Thus, the function f satisfies the conditions of Lagrange’s mean value theorem.
∴ there exists c ∈ (1, e) such that

Hence, Lagrange’s mean value theorem is verified.

(ii) f(x) = (x – 1)(x – 2)(x – 3) on [0, 4].
Solution:
The function f given as
f(x) = (x – 1)(x – 2)(x – 3)
= (x – 1)(x² – 5x + 6)
= x³ – 5x² + 6x – x² + 5x – 6
= x³ – 6x² + 11x – 6 is a polynomial function.
Hence, it is continuous on [0, 4] and differentiable on (0, 4).
Thus, the function f satisfies the conditions of Lagrange’s, mean value theorem.
∴ there exists c ∈ (0, 4) such that


Hence, Lagrange’s mean value theorem is verified.

(iii) f(x) = x² – 3x – 1, x ∈ \(\left[\frac{-11}{7}, \frac{13}{7}\right]\)
Solution:
The function f given as f(x) = x² – 3x – 1 is a polynomial function.
Hence, it is continuous on \(\left[\frac{-11}{7}, \frac{13}{7}\right]\) and differentiable on \(\left(\frac{-11}{7}, \frac{13}{7}\right)\).
Thus, the function f satisfies the conditions of LMVT.
∴ there exists c ∈ \(\left(\frac{-11}{7}, \frac{13}{7}\right)\) such that


Hence, Lagrange’s mean value theorem is verified.

(iv) f(x) = 2x – x², x ∈ [0, 1].
Solution:
The function f given as f(x) = 2x – x² is a polynomial function.
Hence, it is continuous on [0, 1] and differentiable on (0, 1).
Thus, the function f satisfies the conditions of Lagrange’s mean value theorem.
∴ there exists c ∈ (0, 1) such that

Hence, Lagrange’s mean value theorem is verified.

(v) f(x) = \(\frac{x-1}{x-3}\) on [4, 5].
Solution:
The function f given as
f(x) = \(\frac{x-1}{x-3}\) is a rational function which is continuous except at x = 3.
But 3 ∉ [4, 5]
Hence, it is continuous on [4, 5] and differentiable on (4, 5).
Thus, the function f satisfies the conditions of Lagrange’s mean value theorem.
∴ there exists c ∈ (4, 5) such that
f'(c) = \(\frac{f(5)-f(4)}{5-4}\) ……..(1)

Hence, Lagrange’s mean value theorem is verified.

Balbharti 12th Maharashtra State Board Maths Solutions Book Pdf Chapter 1 Differentiation Ex 1.3 Questions and Answers.

Maharashtra State Board 12th Maths Solutions Chapter 1 Differentiation Ex 1.3

Question 1.
Differentiate the following w.r.t. x:
(i) \(\frac{(x+1)^{2}}{(x+2)^{3}(x+3)^{4}}\)
Solution:
Let y = \(\frac{(x+1)^{2}}{(x+2)^{3}(x+3)^{4}}\)
Then, log y = log [latex]\frac{(x+1)^{2}}{(x+2)^{3}(x+3)^{4}}[/latex]
= log (x + 1)² – log (x + 2)³ – log (x + 3)⁴
= 2 log (x +1) – 3 log (x + 2) – 4 log (x + 3)
Differentiating both sides w.r.t. x, we get

(ii) \(\sqrt[3]{\frac{4 x-1}{(2 x+3)(5-2 x)^{2}}}\)
Solution:
Let y = \(\sqrt[3]{\frac{4 x-1}{(2 x+3)(5-2 x)^{2}}}\)
Then log y = log [latex]\sqrt[3]{\frac{4 x-1}{(2 x+3)(5-2 x)^{2}}}[/latex]

Differentiating both sides w.r.t. x, we get

(iii) \(\left(x^{2}+3\right)^{\frac{3}{2}} \cdot \sin ^{3} 2 x \cdot 2^{x^{2}}\)
Solution:
Let y = \(\left(x^{2}+3\right)^{\frac{3}{2}} \cdot \sin ^{3} 2 x \cdot 2^{x^{2}}\)
Then log y = log [latex]\left(x^{2}+3\right)^{\frac{3}{2}} \cdot \sin ^{3} 2 x \cdot 2^{x^{2}}[/latex]

Differentiating both sides w.r.t. x, we get

(iv) \(\frac{\left(x^{2}+2 x+2\right)^{\frac{3}{2}}}{(\sqrt{x}+3)^{3}(\cos x)^{x}}\)
Solution:
Let y = \(\frac{\left(x^{2}+2 x+2\right)^{\frac{3}{2}}}{(\sqrt{x}+3)^{3}(\cos x)^{x}}\)
Then log y = log [latex]\frac{\left(x^{2}+2 x+2\right)^{\frac{3}{2}}}{(\sqrt{x}+3)^{3}(\cos x)^{x}}[/latex]

Differentiating both sides w.r.t. x, we get

(v) \(\frac{x^{5} \cdot \tan ^{3} 4 x}{\sin ^{2} 3 x}\)
Solution:
Let y = \(\frac{x^{5} \cdot \tan ^{3} 4 x}{\sin ^{2} 3 x}\)
Then log y = log [latex]\frac{x^{5} \cdot \tan ^{3} 4 x}{\sin ^{2} 3 x}[/latex]
= log x⁵ + log tan³4x – log sin²3x
= 5 log x+ 3 log (tan 4x) – 2 log (sin 3x)
Differentiating both sides w.r.t. x, we get

(vi) \(x^{\tan ^{-1} x}\)
Solution:
Let y = \(x^{\tan ^{-1} x}\)
Then log y = log (\(x^{\tan ^{-1} x}\)) = (tan^-1 x)(log x)
Differentiating both sides w.r.t. x, we get

(vii) (sin x)^x
Solution:
Let y = (sin x)^x
Then log y = log (sin x)^x = x . log (sin x)
Differentiating both sides w.r.t. x, we get

(viii) sin x^x
Solution:
Let y = (sin x^x)
Then \(\frac{d y}{d x}=\frac{d}{d x}\left[\left(\sin x^{x}\right)\right]\)
\(\frac{d y}{d x}=\cos \left(x^{x}\right) \cdot \frac{d}{d x}\left(x^{x}\right)\) ……. (1)
Let u = x^x
Then log u = log x^x = x . log x
Differentiating both sides w.r.t. x, we get

Question 2.
Differentiate the following w.r.t. x:
(i) x^e + x^x + e^x + e^e
Solution:
Let y = x^e + x^x + e^x + e^e
Let u = x^x
Then log u = log x^x = x log x
Differentiating both sides w.r.t. x, we get
\(\frac{1}{u} \cdot \frac{d u}{d x}=\frac{d}{d x}(x \log x)\)

(ii) \(x^{x^{x}}+e^{x^{x}}\)
Solution:
Let y = \(x^{x^{x}}+e^{x^{x}}\)
Put u = \(x^{x^{x}}\) and v = \(e^{x^{x}}\)
Then y = u + v
∴ \(\frac{d y}{d x}=\frac{d u}{d x}+\frac{d v}{d x}\)
Take u = \(x^{x^{x}}\)
log u = log \(x^{x^{x}}\) = x^x . log x
Differentiating both sides w.r.t. x, we get

(iii) (log x)^x – (cos x)^{cot x}
Solution:
Let y = (log x)^x – (cos x)^{cot x}
Put u = (log x)^x and v = (cos x)^{cot x}
Then y = u – v
∴ \(\frac{d y}{d x}=\frac{d u}{d x}-\frac{d v}{d x}\) ……..(1)
Take u = (log x)^x
∴ log u = log (log x)^x = x . log (log x)
Differentiating both sides w.r.t. x, we get

(iv) \(x^{e^{x}}+(\log x)^{\sin x}\)
Solution:
Let y = \(x^{e^{x}}+(\log x)^{\sin x}\)
Put u = \(x^{e^{x}}\) and v = (log x)^{sin x}
Then y = u + v
∴ \(\frac{d y}{d x}=\frac{d u}{d x}+\frac{d v}{d x}\) ……….(1)
Take u = \(x^{e^{x}}\)
∴ log u = log \(x^{e^{x}}\) = e^x . log x
Differentiating both sides w.r.t. x, we get

Also, v = (log x)^{sin x}
∴ log v = log (log x)^{sin x} = (sin x) . (log log x)
Differentiating both sides w.r.t. x, we get
\(\frac{1}{v} \cdot \frac{d v}{d x}=\frac{d}{d x}[(\sin x) \cdot(\log \log x)]\)
= \((\sin x) \cdot \frac{d}{d x}\left[(\log \log x)+(\log \log x) \cdot \frac{d}{d x}(\sin x)\right]\)

(v) \(e^{\tan x}+(\log x)^{\tan x}\)
Solution:
Let y = \(e^{\tan x}+(\log x)^{\tan x}\)
Put u = (log x)^{tan x}
∴ log u =log(log x)^{tan x} = (tan x).(log log x)
Differentiating both sides w.r.t. x, we get

(vi) (sin x)^{tan x} + (cos x)^{cot x}
Solution:
Let y = (sin x)^{tan x} + (cos x)^{cot x}
Put u = (sin x)^{tan x} and v = (cos x)^{cot x}
Then y = u + v
∴ \(\frac{d y}{d x}=\frac{d u}{d x}+\frac{d v}{d x}\) ………(1)
Take u = (sin x)^{tan x}
∴ log u = log (sin x)^{tan x} = (tan x) . (log sin x)
Differentiating both sides w.r.t. x, we get

(vii) \(10^{x^{x}}+x^{x^{10}}+x^{10^{x}}\)
Solution:
Let y = \(10^{x^{x}}+x^{x^{10}}+x^{10^{x}}\)
Put u = \(10^{x^{x}}\), v = \(x^{x^{10}}\) and w = \(x^{10^{x}}\)
Then y = u + v + w
∴ \(\frac{d y}{d x}=\frac{d u}{d x}+\frac{d v}{d x}+\frac{d w}{d x}\) ………(1)

(viii) \(\left[(\tan x)^{\tan x}\right]^{\tan x}\) at x = \(\frac{\pi}{4}\)
Solution:
Let y = \(\left[(\tan x)^{\tan x}\right]^{\tan x}\)
∴ log y = log [latex]\left[(\tan x)^{\tan x}\right]^{\tan x}[/latex]
= tan x . log(tan x)^{tan x}
= tan x . tan x log (tan x)
= (tan x)² . log (tan x)
Differentiating both sides w.r.t. x, we get

Question 3.
Find \(\frac{d y}{d x}\) if
(i) √x + √y = √a
Solution:
√x + √y = √a
Differentiating both sides w.r.t. x, we get

(ii) x√x + y√y = a√a
Solution:
x√x + y√y = a√a
∴ \(x^{\frac{3}{2}}+y^{\frac{3}{2}}=a^{\frac{3}{2}}\)
Differentiating both sides w.r.t. x, we get

(iii) x + √xy + y = 1
Solution:
x + √xy + y = 1
Differentiating both sides w.r.t. x, we get

(iv) x³ + x²y + xy² + y³ = 81
Solution:
x³ + x²y + xy² + y³ = 81
Differentiating both sides w.r.t. x, we get

(v) x²y² – tan^-1(\(\sqrt{x^{2}+y^{2}}\)) = cot^-1(\(\sqrt{x^{2}+y^{2}}\))
Solution:
x²y² – tan^-1(\(\sqrt{x^{2}+y^{2}}\)) = cot^-1(\(\sqrt{x^{2}+y^{2}}\))
∴ x²y² = tan^-1(\(\sqrt{x^{2}+y^{2}}\)) + cot^-1(\(\sqrt{x^{2}+y^{2}}\))
∴ x²y² = \(\frac{\pi}{2}\) …….[∵ \(\tan ^{-1} x+\cot ^{-1} x=\frac{\pi}{2}\)]
Differentiating both sides w.r.t. x, we get

(vi) xe^y + ye^x = 1
Solution:
xe^y + ye^x = 1
Differentiating both sides w.r.t. x, we get

(vii) e^x+y = cos (x – y)
Solution:
e^x+y = cos (x – y)
Differentiating both sides w.r.t. x, we get

(viii) cos (xy) = x + y
Solution:
cos (xy) = x + y
Differentiating both sides w.r.t. x, we get

(ix) \(e^{e^{x-y}}=\frac{x}{y}\)
Solution:
\(e^{e^{x-y}}=\frac{x}{y}\)
∴ e^x-y = log(\(\frac{x}{y}\)) …….[e^x = y ⇒ x = log y]
∴ e^x-y = log x – log y
Differentiating both sides w.r.t. x, we get

Question 4.
Show that \(\frac{d y}{d x}=\frac{y}{x}\) in the following, where a and p are constants.
(i) x⁷y⁵ = (x + y)¹²
Solution:
x⁷y⁵ = (x + y)¹²
(log x⁷y⁵) = log(x + y)¹²
log x⁷ + log y⁵ = log(x + y)¹²
7 log x + 5 log y = 12 log (x + y)
Differentiating both sides w.r.t. x, we get

(ii) x^py⁴ = (x + y)^p+4, p∈N
Solution:
x^py⁴ = (x + y)^p+4
Taking log
log (x^py⁴) = log(x + y)^p+4
log x^p + log y⁴ = (p + 4) log(x + y)
p log x + 4 log y = (p + 4) log(x + y)
Differentiating both sides w.r.t. x, we get

(iii) \(\sec \left(\frac{x^{5}+y^{5}}{x^{5}-y^{5}}\right)=a^{2}\)
Solution:
\(\sec \left(\frac{x^{5}+y^{5}}{x^{5}-y^{5}}\right)=a^{2}\)

Differentiating both sides w.r.t. x, we get


Differentiating both sides w.r.t. x, we get

(iv) \(\tan ^{-1}\left(\frac{3 x^{2}-4 y^{2}}{3 x^{2}+4 y^{2}}\right)=a^{2}\)
Solution:
\(\tan ^{-1}\left(\frac{3 x^{2}-4 y^{2}}{3 x^{2}+4 y^{2}}\right)=a^{2}\)

Differentiating both sides w.r.t. x, we get

(v) \(\cos ^{-1}\left(\frac{7 x^{4}+5 y^{4}}{7 x^{4}-5 y^{4}}\right)=\tan ^{-1} a\)
Solution:

(vi) \(\log \left(\frac{x^{20}-y^{20}}{x^{20}+y^{20}}\right)=20\)
Solution:
\(\log \left(\frac{x^{20}-y^{20}}{x^{20}+y^{20}}\right)=20\)

Differentiating both sides w.r.t. x, we get

(vii) \(e^{\frac{x^{7}-y^{7}}{x^{7}+y^{7}}}=a\)
Solution:
\(e^{\frac{x^{7}-y^{7}}{x^{7}+y^{7}}}=a\)

Differentiating both sides w.r.t. x, we get

(viii) \(\sin \left(\frac{x^{3}-y^{3}}{x^{3}+y^{3}}\right)=a^{3}\)
Solution:

Differentiating both sides w.r.t. x, we get

Question 5.
(i) If log (x + y) = log (xy) + p, where p is a constant, then prove that \(\frac{d y}{d x}=-\frac{y^{2}}{x^{2}}\).
Solution:
log (x + y) = log (xy) + p
∴ log (x + y) = log x + log y + p
Differentiating both sides w.r.t. x, we get

(ii) If \(\log _{10}\left(\frac{x^{3}-y^{3}}{x^{3}+y^{3}}\right)=2\), show that \(\frac{d y}{d x}=-\frac{99 x^{2}}{101 y^{2}}\)
Solution:
\(\log _{10}\left(\frac{x^{3}-y^{3}}{x^{3}+y^{3}}\right)=2\)

(iii) If \(\log _{5}\left(\frac{x^{4}+y^{4}}{x^{4}-y^{4}}\right)=2\), show that \(\frac{d y}{d x}=-\frac{12 x^{3}}{13 y^{3}}\)
Solution:

Differentiating both sides w.r.t. x, we get

(iv) If e^x + e^y = e^x+y, then show that \(\frac{d y}{d x}=-e^{y-x}\)
Solution:
e^x + e^y = e^x+y ……(1)
Differentiating both sides w.r.t. x, we get

(v) If \(\sin ^{-1}\left(\frac{x^{5}-y^{5}}{x^{5}+y^{5}}\right)=\frac{\pi}{6}\), show that \(\frac{d y}{d x}=\frac{x^{4}}{3 y^{4}}\)
Solution:
\(\sin ^{-1}\left(\frac{x^{5}-y^{5}}{x^{5}+y^{5}}\right)=\frac{\pi}{6}\)
\(\frac{x^{5}-y^{5}}{x^{5}+y^{5}}=\sin \frac{\pi}{6}=\frac{1}{2}\)
2x⁵ – 2y⁵ = x⁵ + y⁵
3y⁵ = x⁵
Differentiating both sides w.r.t. x, we get
\(3 \times 5 y^{4} \frac{d y}{d x}=5 x^{4}\)
∴ \(\frac{d y}{d x}=\frac{x^{4}}{3 y^{4}}\)

(vi) If x^y = e^x-y, then show that \(\frac{d y}{d x}=\frac{\log x}{(1+\log x)^{2}}\)
Solution:
x^y = e^x-y
log x^y = log e^x-y
y log x = (x – y) log e
y log x = (x – y) ….. [∵ log e = 1]
y + y log x = x – y
y + y log x = x
y(1 + log x) = x
y = \(\frac{x}{1+\log x}\)

(vii) If \(y=\sqrt{\cos x+\sqrt{\cos x+\sqrt{\cos x+\ldots \infty}}}\), then show that \(\frac{d y}{d x}=\frac{\sin x}{1-2 y}\)
Solution:
\(y=\sqrt{\cos x+\sqrt{\cos x+\sqrt{\cos x+\ldots \infty}}}\)
y² = cos x + \(\sqrt{\cos x+\sqrt{\cos x+\ldots \infty}}\)
y² = cos x + y
Differentiating both sides w.r.t. x, we get

(viii) If \(y=\sqrt{\log x+\sqrt{\log x+\sqrt{\log x+\ldots \infty}}}\), then show that \(\frac{d y}{d x}=\frac{1}{x(2 y-1)}\)
Solution:
\(y=\sqrt{\log x+\sqrt{\log x+\sqrt{\log x+\ldots \infty}}}\)

(ix) If \(y=x^{x^{x^{-\infty}}}\), then show that \(\frac{d y}{d x}=\frac{y^{2}}{x(1-\log y)}\)
Solution:
\(y=x^{x^{x^{-\infty}}}\)

(x) If e^y = y^x, then show that \(\frac{d y}{d x}=\frac{(\log y)^{2}}{\log y-1}\)
Solution:
e^y = y^x
log e^y = log y^x
y log e = x log y
y = x log y …… [∵log e = 1] ……….(1)
Differentiating both sides w.r.t. x, we get
\(\frac{d y}{d x}=x \frac{d}{d x}(\log y)+(\log y) \cdot \frac{d}{d x}(x)\)

Balbharti 12th Maharashtra State Board Maths Solutions Book Pdf Chapter 3 Indefinite Integration Ex 3.2(A) Questions and Answers.

Maharashtra State Board 12th Maths Solutions Chapter 3 Indefinite Integration Ex 3.2(A)

I. Integrate the following functions w.r.t. x:

Question 1.
\(\frac{(\log x)^{n}}{x}\)
Solution:

Question 2.
\(\frac{\left(\sin ^{-1} x\right)^{\frac{3}{2}}}{\sqrt{1-x^{2}}}\)
Solution:
Let I = \(\int \frac{\left(\sin ^{-1} x\right)^{\frac{3}{2}}}{\sqrt{1-x^{2}}} d x\)

Question 3.
\(\frac{1+x}{x \cdot \sin (x+\log x)}\)
Solution:

Question 4.
\(\frac{x \cdot \sec ^{2}\left(x^{2}\right)}{\sqrt{\tan ^{3}\left(x^{2}\right)}}\)
Solution:

Question 5.
\(\frac{e^{3 x}}{e^{3 x}+1}\)
Solution:

Question 6.
\(\frac{\left(x^{2}+2\right)}{\left(x^{2}+1\right)} \cdot a^{x+\tan ^{-1} x}\)
Solution:

Question 7.
\(\frac{e^{x} \cdot \log \left(\sin e^{x}\right)}{\tan \left(e^{x}\right)}\)
Solution:

Question 8.
\(\frac{e^{2 x}+1}{e^{2 x}-1}\)
Solution:

Question 9.
sin⁴x . cos³x
Solution:

Question 10.
\(\frac{1}{4 x+5 x^{-11}}\)
Solution:

Question 11.
x⁹ . sec²(x¹⁰)
Solution:

Question 12.
\(e^{3 \log x} \cdot\left(x^{4}+1\right)^{-1}\)
Solution:

Question 13.
\(\frac{\sqrt{\tan x}}{\sin x \cdot \cos x}\)
Solution:
Let I = \(\int \frac{\sqrt{\tan x}}{\sin x \cdot \cos x} d x\)
Dividing numerator and denominator by cos²x, we get

Question 14.
\(\frac{(x-1)^{2}}{\left(x^{2}+1\right)^{2}}\)
Solution:

Question 15.
\(\frac{2 \sin x \cos x}{3 \cos ^{2} x+4 \sin ^{2} x}\)
Solution:

Question 16.
\(\frac{1}{\sqrt{x}+\sqrt{x^{3}}}\)
Solution:

Question 17.
\(\frac{10 x^{9}+10^{x} \cdot \log 10}{10^{x}+x^{10}}\)
Solution:

Question 18.
\(\frac{x^{n-1}}{\sqrt{1+4 x^{n}}}\)
Solution:

Question 19.
(2x + 1) \(\sqrt{x+2}\)
Solution:

Question 20.
\(x^{5} \sqrt{a^{2}+x^{2}}\)
Solution:

Question 21.
\((5-3 x)(2-3 x)^{-\frac{1}{2}}\)
Solution:

Question 22.
\(\frac{7+4 x+5 x^{2}}{(2 x+3)^{\frac{3}{2}}}\)
Solution:

Question 23.
\(\frac{x^{2}}{\sqrt{9-x^{6}}}\)
Solution:

Question 24.
\(\frac{1}{x\left(x^{3}-1\right)}\)
Solution:

Question 25.
\(\frac{1}{x \cdot \log x \cdot \log (\log x)}\)
Solution:

II. Integrate the following functions w.r.t x:

Question 1.
\(\frac{\cos 3 x-\cos 4 x}{\sin 3 x+\sin 4 x}\)
Solution:

Question 2.
\(\frac{\cos x}{\sin (x-a)}\)
Solution:

Question 3.
\(\frac{\sin (x-a)}{\cos (x+b)}\)
Solution:

Question 4.
\(\frac{1}{\sin x \cdot \cos x+2 \cos ^{2} x}\)
Solution:
Let I = \(\int \frac{1}{\sin x \cdot \cos x+2 \cos ^{2} x} d x\)
Dividing numerator and denominator of cos²x, we get

Question 5.
\(\frac{\sin x+2 \cos x}{3 \sin x+4 \cos x}\)
Solution:
Let I = \(\int \frac{\sin x+2 \cos x}{3 \sin x+4 \cos x} d x\)
Put, Numerator = A (Denominator) + B [\(\frac{d}{d x}\) (Denominator)]
∴ sin x+ 2 cos x = A(3 sin x + 4 cos x) + B [\(\frac{d}{d x}\) (3 sin x + 4 cos x)]
= A(3 sin x + 4 cos x) + B (3 cos x – 4 sin x)
∴ sin x + 2 cos x = (3A – 4B) sin x + (4A + 3B) cos x
Equating the coefficients of sin x and cos x on both the sides, we get
3A – 4B = 1 …… (1)
and 4A + 3B = 2 …… (2)
Multiplying equation (1) by 3 and equation (2) by 4, we get
9A – 12B = 3
16A + 12B = 8
On adding, we get

Question 6.
\(\frac{1}{2+3 \tan x}\)
Solution:
Let I = \(\int \frac{1}{2+3 \tan x} d x\)

Numerator = A (Denominator) + B [\(\frac{d}{d x}\) (Denominator)]
∴ cos x = A(2 cos x + 3 sin x) + B [\(\frac{d}{d x}\) (2 cos x + 3 sin x)]
= A (2 cos x + 3 sin x) + B (-2 sin x + 3 cos x)
∴ cos x = (2A + 3B) cos x + (3A – 2B) sin x
Equating the coefficients of cosx and sinx on both the sides, we get
2A + 3B = 1 …… (1)
and 3A – 2B = 0 ……. (2)
Multiplying equation (1) by 2 and equation (2) by 3, we get
4A + 6B = 2
9A – 6B = 0
On adding, we get

Question 7.
\(\frac{4 e^{x}-25}{2 e^{x}-5}\)
Solution:
Let I = \(\int \frac{4 e^{x}-25}{2 e^{x}-5} d x\)
Put, Numerator = A (Denominator) + B [\(\frac{d}{d x}\) (Denominator)]
∴ 4ex – 25 = A(2ex – 5) + B[\(\frac{d}{d x}\) (2ex – 5)]
= A(2ex – 5) + B(2ex – 0)
∴ 4ex – 25 = (2A + 2B) ex – 5A
Equating the coefficient of ex and constant on both sides, we get
2A + 2B = 4 …….(1)
and 5A = 25
∴ A = 5
from (1), 2(5) + 2B = 4
∴ 2B = -6
∴ B = -3

Question 8.
\(\frac{20+12 e^{x}}{3 e^{x}+4}\)
Solution:

Question 9.
\(\frac{3 e^{2 x}+5}{4 e^{2 x}-5}\)
Solution:
Let I = \(\int \frac{3 e^{2 x}+5}{4 e^{2 x}-5} d x\)
Put, Numerator = A (Denominator) + B [\(\frac{d}{d x}\) (Denominator)]
∴ 3e2x + 5 = A(4e2x – 5) + B [\(\frac{d}{d x}\) (4e2x – 5)]
= A(4e2x – 5) + B(4 . e2x × 2 – 0)
∴ 3e2x + 5 = (4A + 8B) e2x – 5A
Equating the coefficient of e2x and constant on both sides, we get
4A + 8B = 3 …….. (1)
and -5A = 5
∴ A = -1
∴ from (1), 4(-1) + 8B = 3
∴ 8B = 7
∴ B = \(\frac{7}{8}\)

Question 10.
cos⁸ x . cot x
Solution:

Question 11.
tan⁵x
Solution:
Let I = ∫ tan⁵x dx

Question 12.
cos⁷x
Solution:

Question 13.
tan 3x tan 2x tan x
Solution:
Let I = ∫ tan 3x tan 2x tan x dx
Consider tan 3x = tan (2x + x) = \(\frac{\tan 2 x+\tan x}{1-\tan 2 x \tan x}\)
tan 3x (1 – tan 2x tan x) = tan 2x + tan x
tan 3x – tan 3x tan 2x tan x = tan 2x + tan x
tan 3x – tan 2x – tan x = tan 3x tan 2x tan x
I = ∫(tan 3x – tan 2x – tan x) dx
= ∫tan3x dx – ∫tan 2x dx – ∫tan x dx
= \(\frac{1}{3}\) log | sec 3x| – \(\frac{1}{2}\) log |sec 2x| – log |sec x| + c.

Question 14.
sin⁵x cos⁸x
Solution:

Question 15.
\(3^{\cos ^{2} x \cdot} \sin 2 x\)
Solution:

Question 16.
\(\frac{\sin 6 x}{\sin 10 x \sin 4 x}\)
Solution:

Question 17.
\(\frac{\sin x \cos ^{3} x}{1+\cos ^{2} x}\)
Solution:

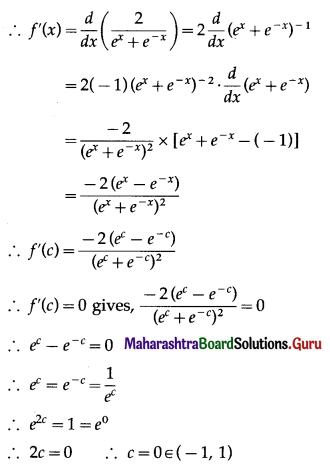
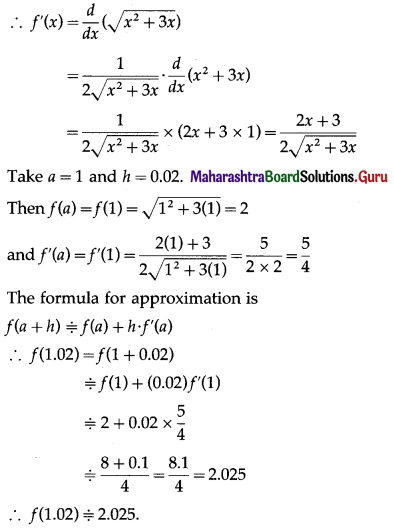
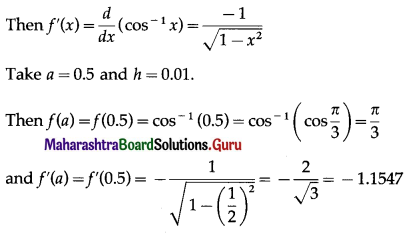
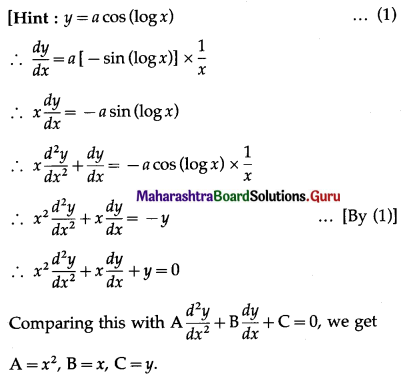
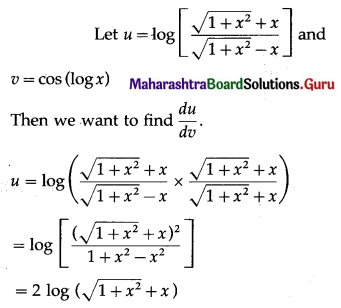
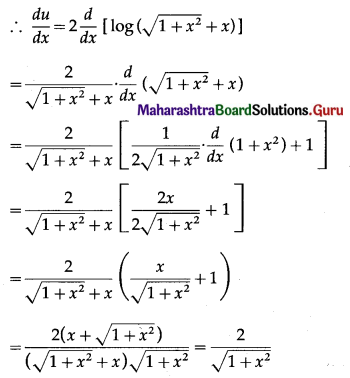
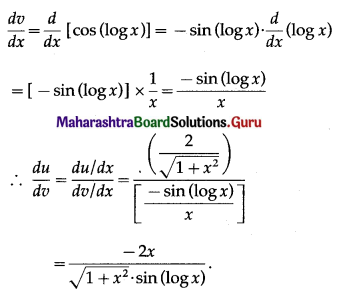
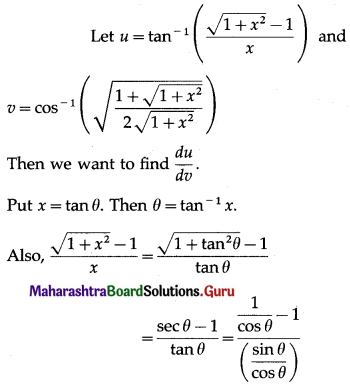
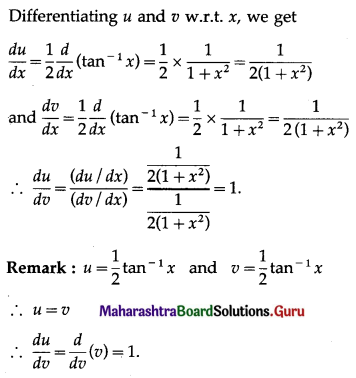
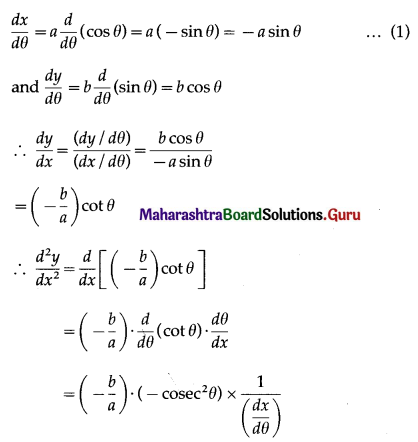
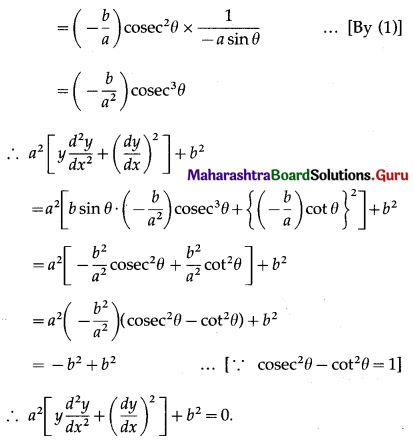
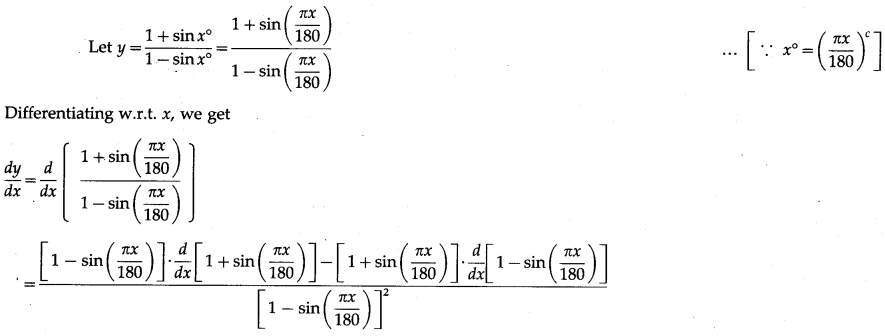
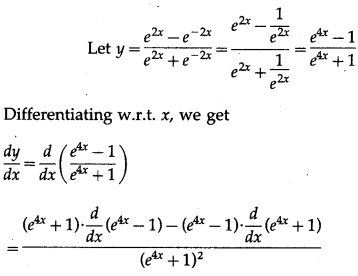
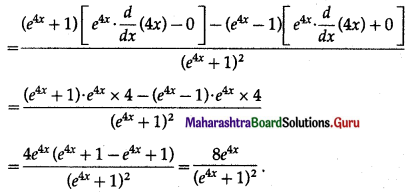
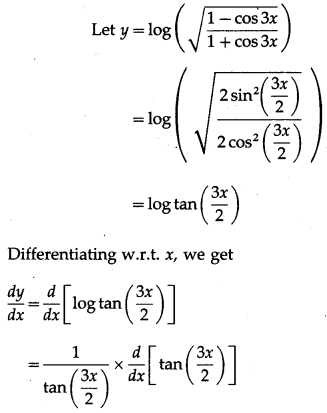
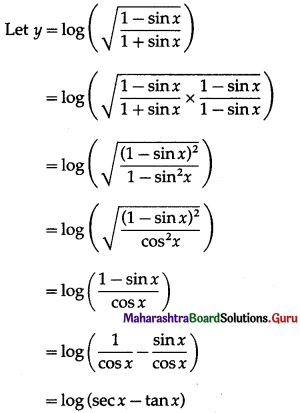
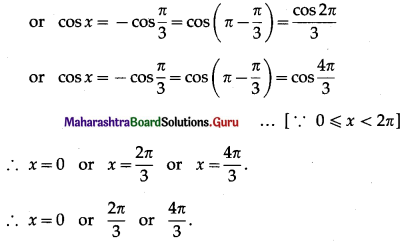
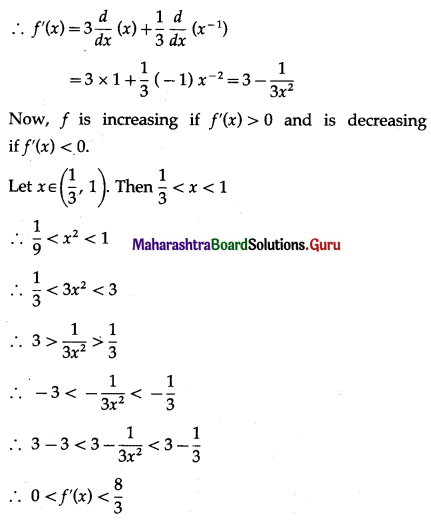
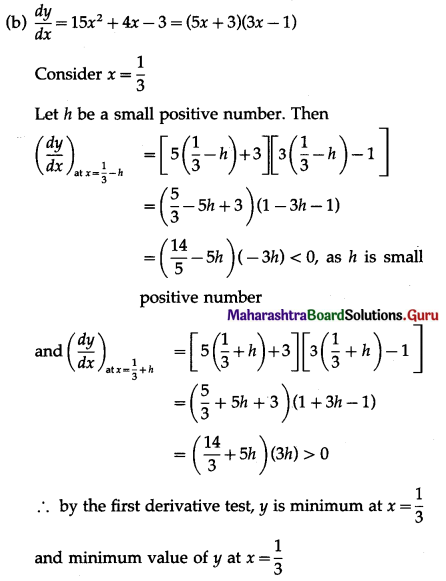
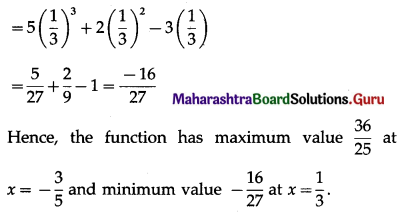
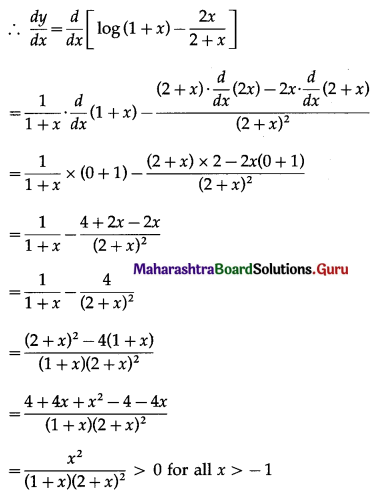
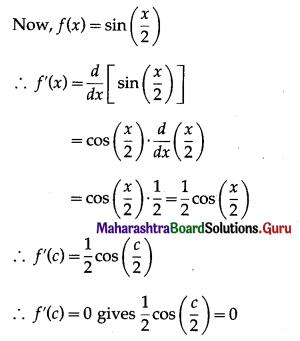
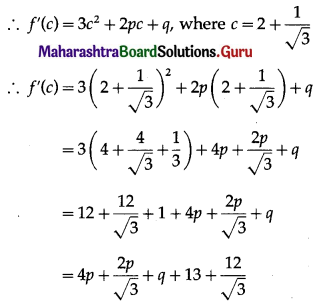
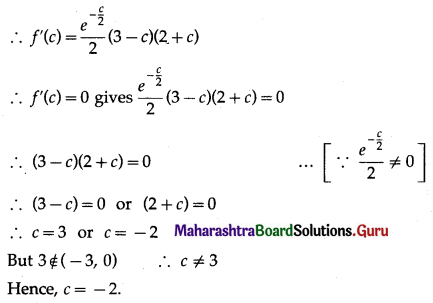
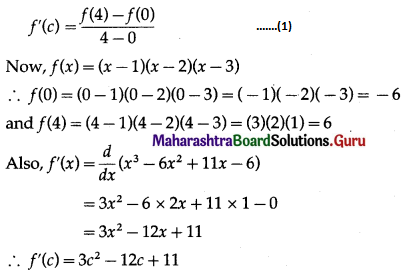
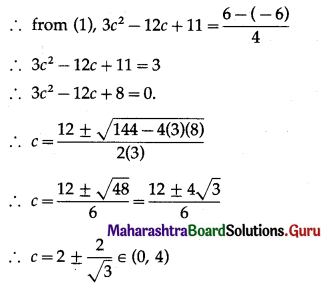
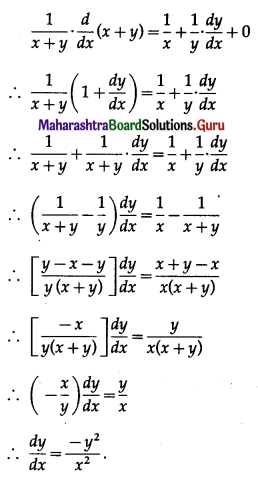
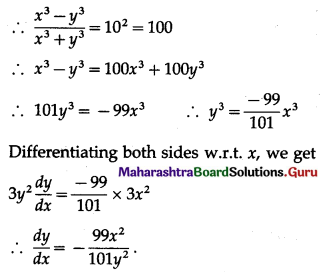
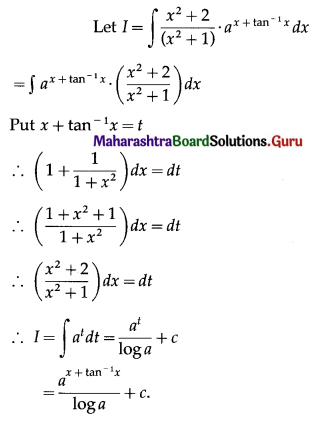
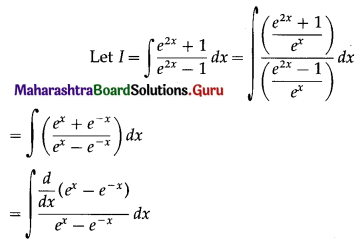
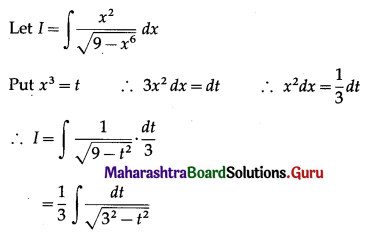
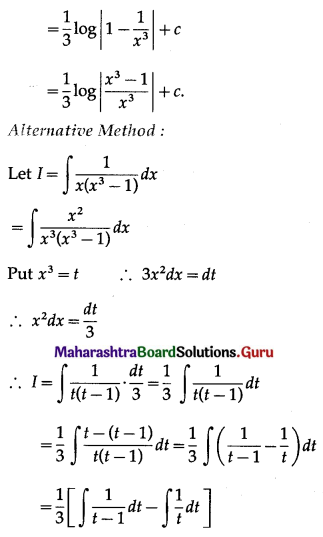
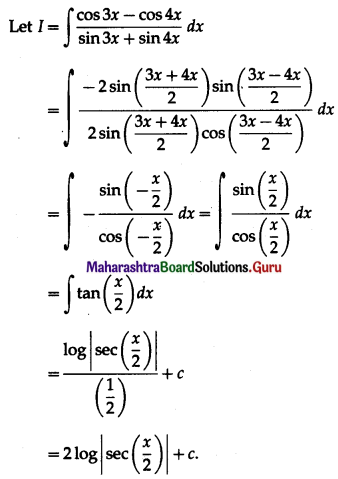
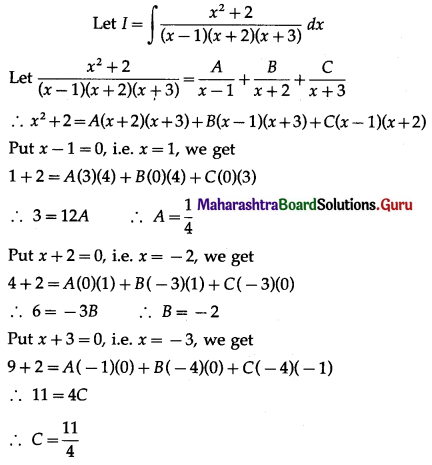
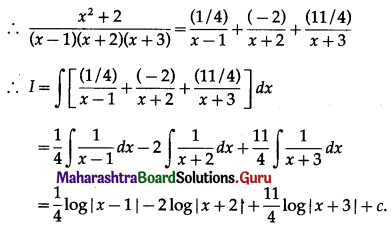
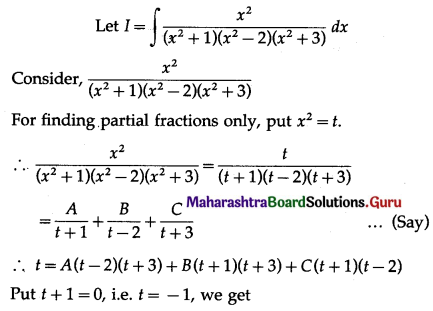
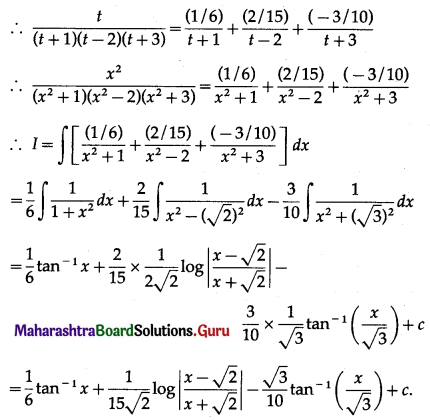
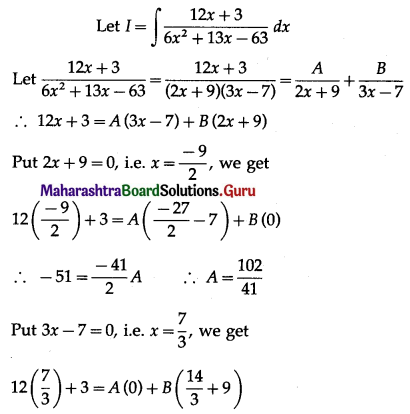
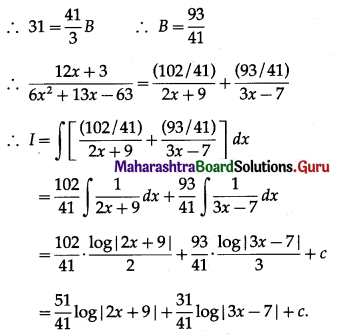
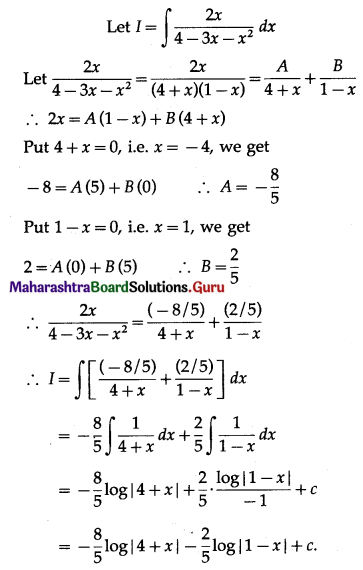
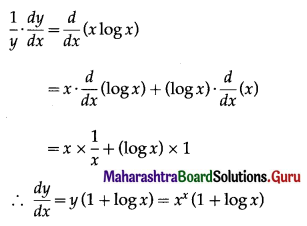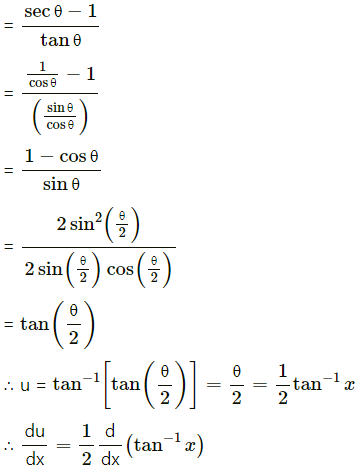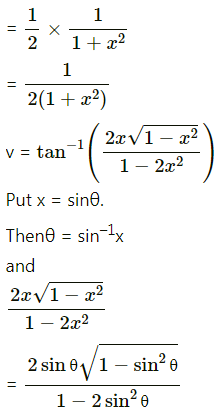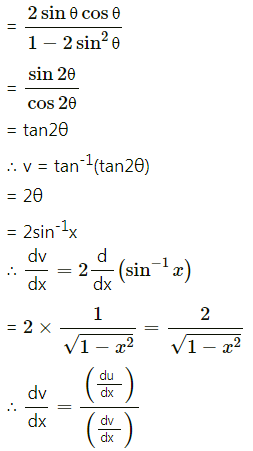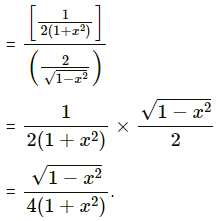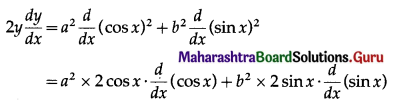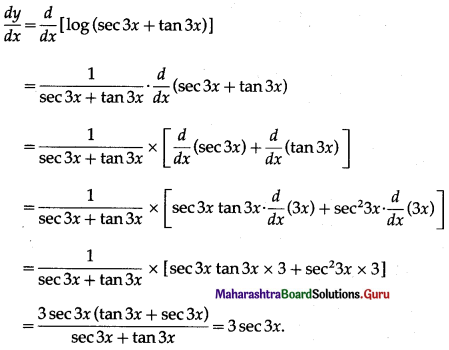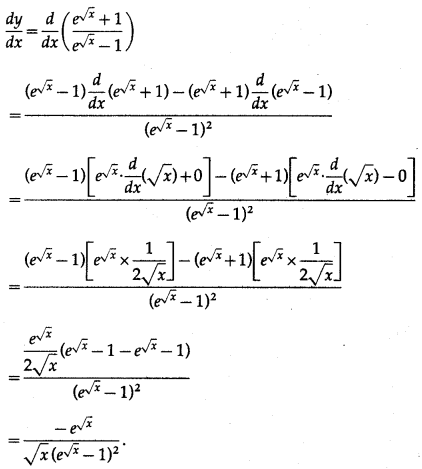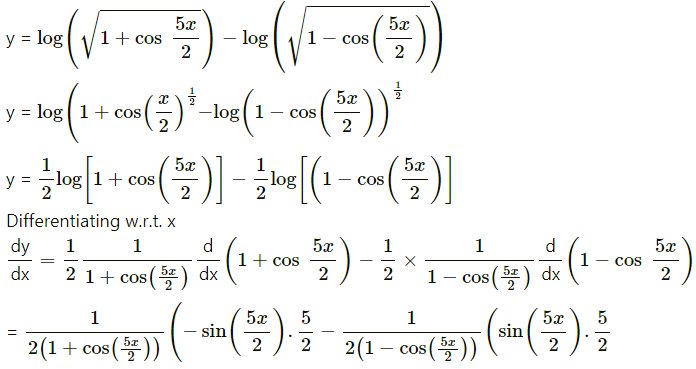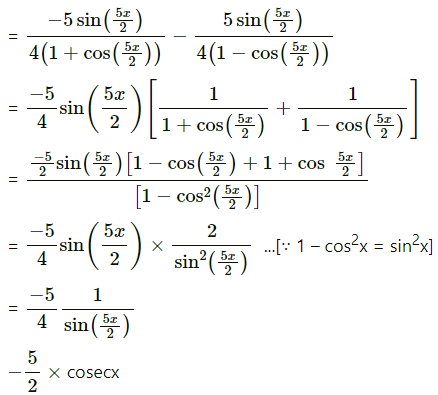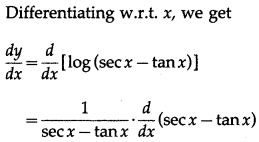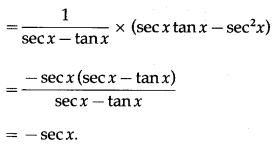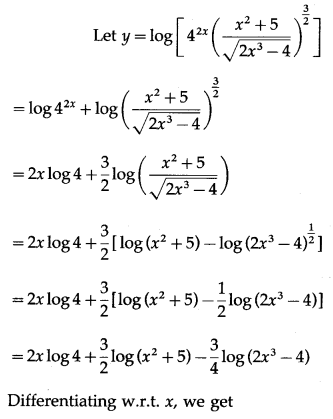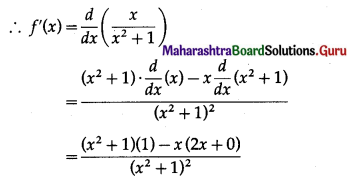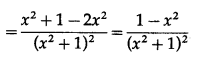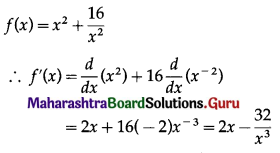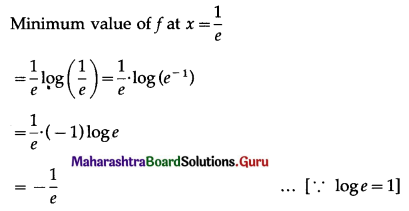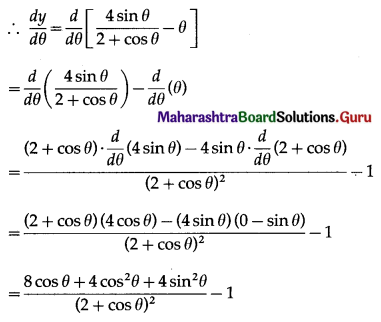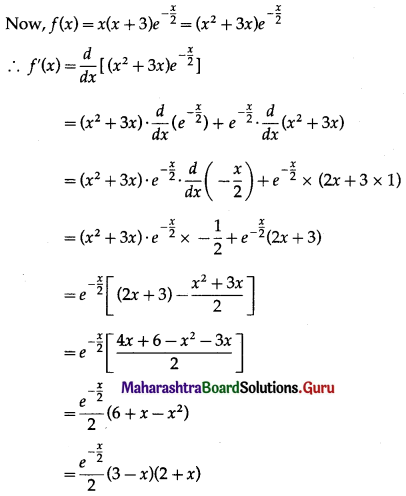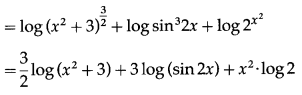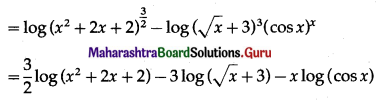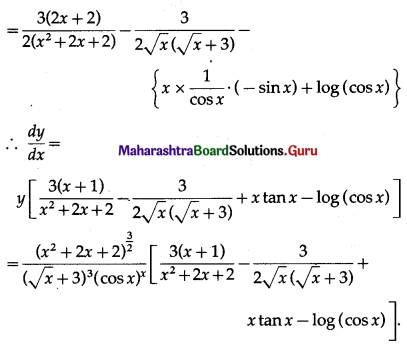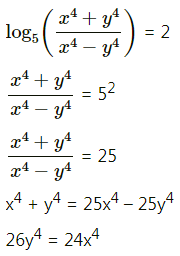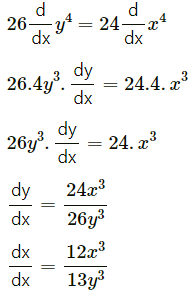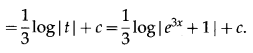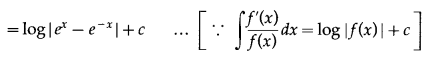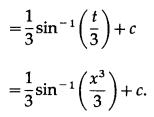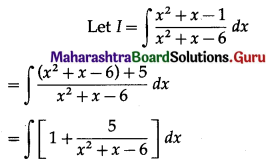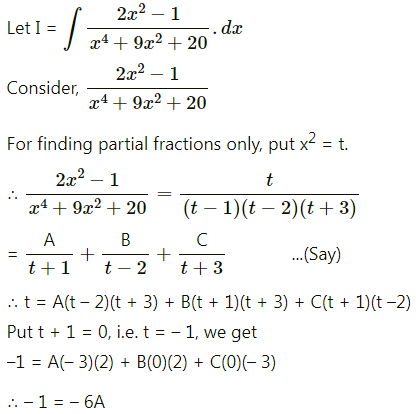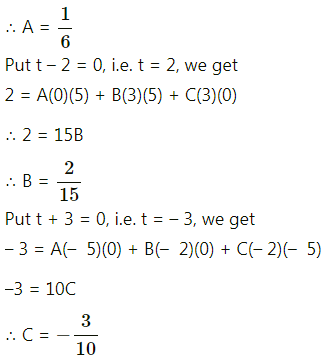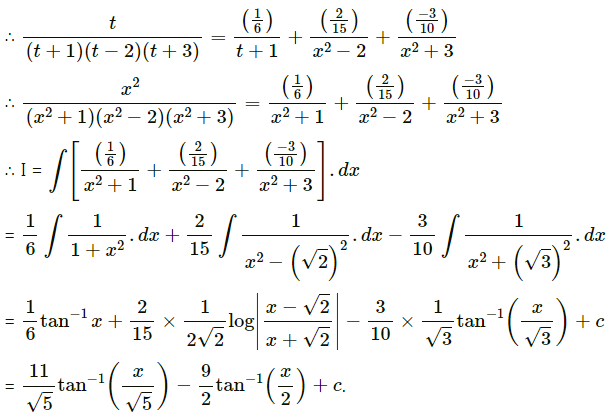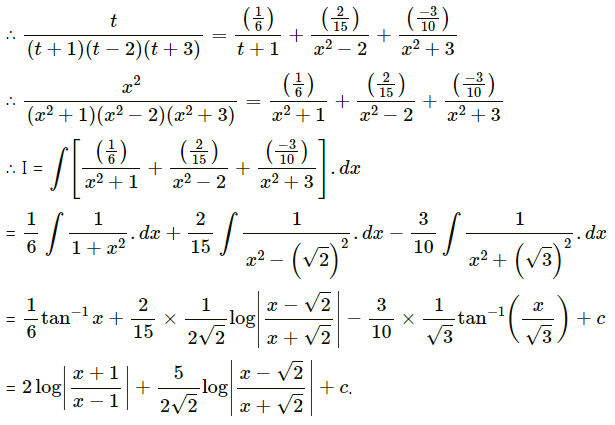Balbharti 12th Maharashtra State Board Maths Solutions Book Pdf Chapter 7 Linear Programming Miscellaneous Exercise 7 Questions and Answers.

Maharashtra State Board 12th Maths Solutions Chapter 7 Linear Programming Miscellaneous Exercise 7

I) Select the appropriate alternatives for each of the following :
Question 1.
The value of objective function is maximum under linear constraints _______.
(A) at the centre of feasible region
(B) at (0, 0)
(C) at a vertex of feasible region
(D) the vertex which is of maximum distance from (0, 0)
Solution:
(C) at a vertex of feasible region

Question 2.
Which of the following is correct _______.
(A) every L.P.P. has an optimal solution
(B) a L.P.P. has unique optimal solution
(C) if L.P.P. has two optimal solutions then it has infinite number of optimal solutions
(D) the set of all feasible solution of L.P.P. may not be convex set
Solution:
(C) if L.P.P. has two optimal solutions then it has infinite number of optimal solutions

Question 3.
Objective function of L.P.P. is _______.
(A) a constraint
(B) a function to be maximized or minimized
(C) a relation between the decision variables
(D) equation of a straight line
Solution:
(B) a function to be maximized or minimized

Question 4.
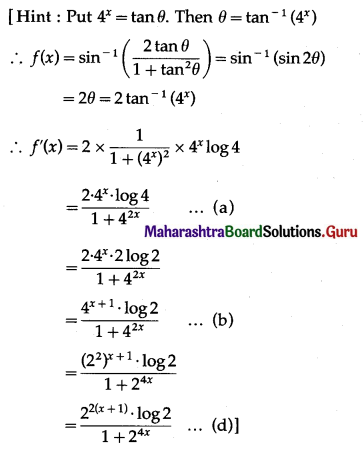
The maximum value of z = 5x + 3y subjected to the constraints 3x + 5y ≤ 15, 5x + 2y ≤ 10, x, y≥ 0 is _______.
(A) 235
(B) \(\frac{235}{9}\)
(C) \(\frac{235}{19}\)
(D) \(\frac{235}{3}\)
Solution:
(C) \(\frac{235}{19}\)

Question 5.
The maximum value of z = 10x + 6y subjected to the constraints 3x + y ≤ 12, 2x + 5y ≤ 34, x ≥ 0, y≥ 0. _______.
(A) 56
(B) 65
(C) 55
(D) 66
Solution:
(A) 56

Question 6.
The point at which the maximum value of x + y subject to the constraints x + 2y ≤ 70, 2x + y ≤ 95, x ≥ 0, y ≥ 0 is obtained at _______.
(A) (30, 25)
(B) (20, 35)
(C) (35, 20)
(D) (40, 15)
Solution:
(D) (40, 15)

Question 7.
Of all the points of the feasible region, the optimal value ofz obtained at the point lies _______.
(A) inside the feasible region
(B) at the boundary of the feasible region
(C) at vertex of feasible region
(D) outside the feasible region
Solution:
(C) at vertex of feasible region

Question 8.
Feasible region is the set of points which satisfy _______.
(A) the objective function
(B) all of the given constraints
(C) some of the given constraints
(D) only one constraint
Solution:
(B) all of the given constraints

Question 9.
Solution of L.P.P. to minimize z = 2x + 3y such that x ≥ 0, y ≥ 0, 1 ≤ x + 2y ≤ 10 is _______.
(A) x = 0, y = \(\frac{1}{2}\)
(B) x = \(\frac{1}{2}\), y = 0
(C) x = 1, y = 2
(D) x = \(\frac{1}{2}\), y = \(\frac{1}{2}\)
Solution:
(A) x = 0, y = \(\frac{1}{2}\)

Question 10.
The corner points of the feasible solution given by the inequation x + y ≤ 4, 2x + y ≤ 7, x ≥ 0, y ≥ 0 are _______.
(A) (0, 0), (4, 0), (7, 1), (0, 4)
(B) (0, 0), (\(\frac{7}{2}\), 0), (3, 1), (0, 4)
(C) (0, 0), (\(\frac{7}{2}\), 0), (3, 1), (0, 7)
(D) (0, 0), (4, 0), (3, 1), (0, 7)
Solution:
(B) (0, 0), (\(\frac{7}{2}\), 0), (3, 1), (0, 4)

Question 11.
The corner points of the feasible solution are (0, 0), (2, 0), (\(\frac{12}{7}\), \(\frac{3}{7}\)), (0, 1). Then z = 7x + y is maximum at _______.
(A) (0, 0)
(B) (2, 0)
(C) (\(\frac{12}{7}\), \(\frac{3}{7}\))
(D) (0, 1)
Solution:
(B) (2, 0)

Question 12.
If the corner points of the feasible solution are (0, 0), (3, 0), (2, 1) and (0, \(\frac{7}{3}\) ), the maximum value of z = 4x + 5y is _______.
(A) 12
(B) 13
(C) 35
(D) 0
Solution:
(B) 13

Question 13.
If the corner points of the feasible solution are (0, 10), (2, 2) and (4, 0) then the point of minimum z = 3x + 2y is _______.
(A) (2, 2)
(B) (0, 10)
(C) (4, 0)
(D) (3 ,4)
Solution:
(A) (2, 2)

Question 14.
The half plane represented by 3x + 2y < 8 contains the point _______.
(A) (1, \(\frac{5}{2}\))
(B) (2, 1)
(C) (0, 0)
(D) (5, 1)
Solution:
(C) (0, 0)

Question 15.
The half plane represented by 4x + 3y > 14 contains the point _______.
(A) (0, 0)
(B) (2, 2)
(C) (3, 4)
(D) (1, 1)
Solution:
(C) (3, 4)

II) Solve the following :
Question 1.
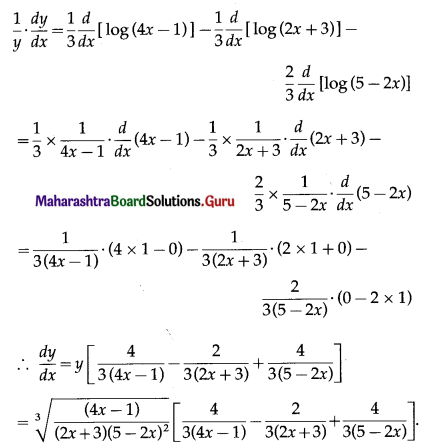
Solve each of the following inequations graphically using X Y plane.
(i) 4x – 18 ≥ 0
Solution:
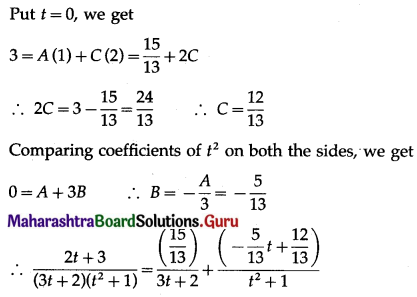
Consider the line whose equation is 4x – 18 ≥ 0 i.e. x = \(\frac{18}{4}=\frac{9}{2}\) = 4.5
This represents a line parallel to Y-axis passing3through the point (4.5, 0)
Draw the line x = 4.5
To find the solution set we have to check the position of the origin (0, 0).
When x = 0, 4x – 18 = 4 × 0 – 18 = -18 > 0
∴ the coordinates of the origin does not satisfy thegiven inequality.
∴ the solution set consists of the line x = 4.5 and the non-origin side of the line which is shaded in the graph.

(ii) -11x – 55 ≤ 0
Solution:
Consider the line whose equation is -11x – 55 ≤ 0 i.e. x = -5
This represents a line parallel to Y-axis passing3through the point (-5, 0)
Draw the line x = – 5
To find the solution set we have to check the position of the origin (0, 0).
When x = 0, -11x – 55 = – 11(0) – 55 = -55 > 0
∴ the coordinates of the origin does not satisfy thegiven inequality.
∴ the solution set consists of the line x = -5 and the non-origin side of the line which is shaded in the graph.

(iii) 5y – 12 ≥ 0
Solution:
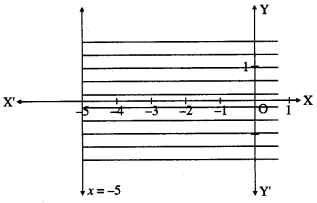
Consider the line whose equation is 5y – 12 ≥ 0 i.e. y = \(\frac{12}{5}\)
This represents a line parallel to X-axis passing through the point (o, \(\frac{12}{5}\))
Draw the line y = \(\frac{12}{5}\)
To find the solution set, we have to check the position of the origin (0, 0).
When y = 0, 5y – 12 = 5(0) – 12 = -12 > 0
∴ the coordinates of the origin does not satisfy the given inequality.
∴ the solution set consists of the line y = \(\frac{12}{5}\) and the non-origin side of the line which is shaded in the graph.

(iv) y ≤ -3.5
Solution:
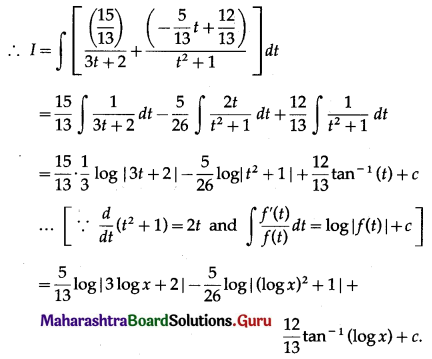
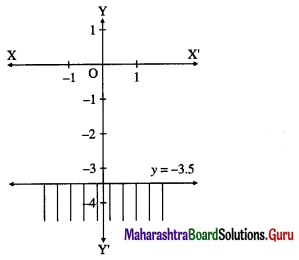
Consider the line whose equation is y ≤ – 3.5 i.e. y = – 3.5
This represents a line parallel to X-axis passing3through the point (0, -3.5)
Draw the line y = – 3.5
To find the solution set, we have to check the position of the origin (0, 0).
∴ the coordinates of the origin does not satisfy the given inequality.
∴ the solution set consists of the line y = – 3.5 and the non-origin side of the line which is shaded in the graph.

Question 2.
Sketch the graph of each of following inequations in XOY co-ordinate system.
(i) x ≥ 5y
Solution:

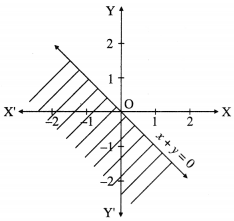
(ii) x + y≤ 0
Solution:

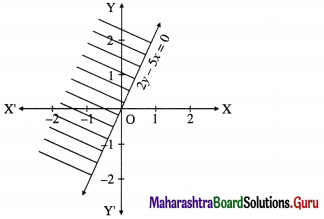
(iii) 2y – 5x ≥ 0
Solution:

(iv) |x + 5| ≤ y
Solution:
|x + 5| ≤ y
∴ -y ≤ x + 5 ≤ y
∴ -y ≤ x + 5 and x + 5 ≤ y
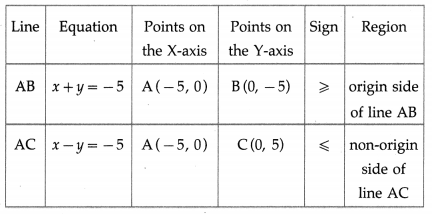
∴ x + y ≥ -5 and x – y ≤ -5
First we draw the lines AB and AC whose equations are
x + y= -5 and x – y = -5 respectively.

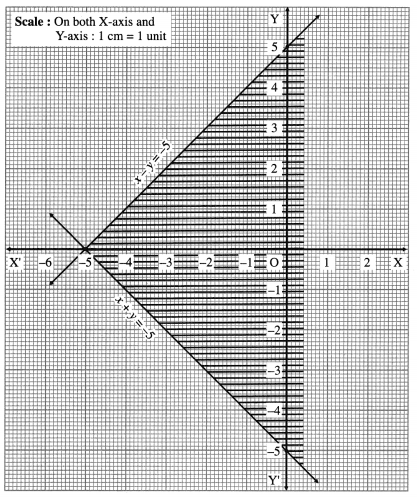
The graph of |x + 5| ≤ y is as below:

Question 3.
Find graphical solution for each of the following system of linear inequation.
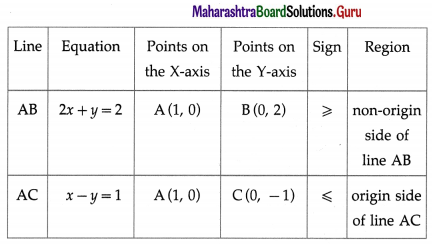
(i) 2x + y ≥ 2, x – y ≤ 1
Solution:
First we draw the lines AB and AC whose equations are 2x + y = 2 and x – y = 1 respectively.


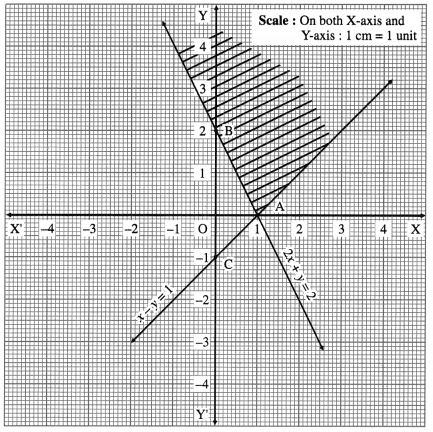
The solution set of the given system of inequalities is shaded in the graph.

(ii) x + 2y ≥ 4, 2x – y ≤ 6
Solution:

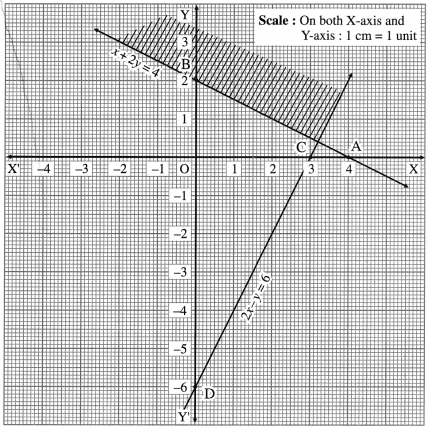
(iii) 3x + 4y ≤ 12, x – 2y ≥ 2, y ≥ -1
Solution:
First we draw the lines AB, CD and ED whose equations are 3x + 4y = 12, x – 2y = 2 and y = -1 respectively.


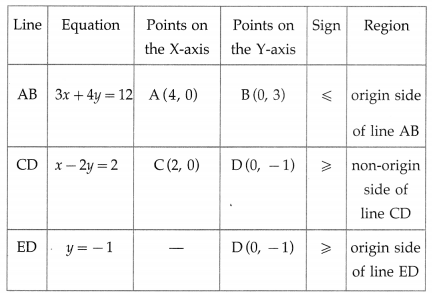
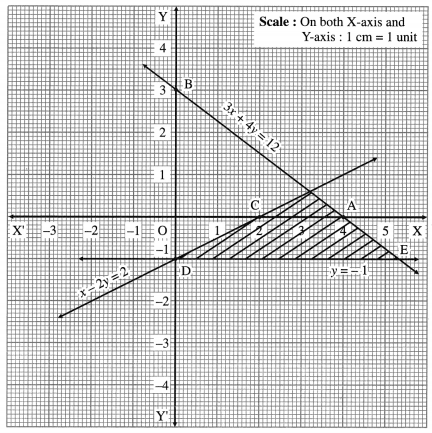
The solution set of given system of inequation is shaded in the graph.

Question 4.
Find feasible solution for each of the following system of linear inequations graphically.
(i) 2x + 3y ≤ 12, 2x + y ≤ 8, x ≥ 0, y ≥ 0
Solution:

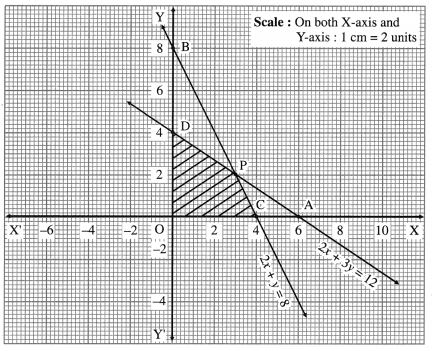
The feasible solution is OCPBO.

(ii) 3x + 4y ≥ 12, 4x + 7y ≤ 28, x ≥ 0, y ≥ 0
Solution:

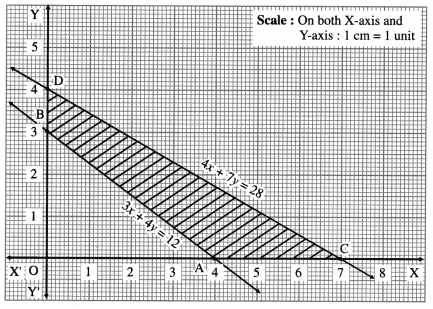
The feasible solution is ACDBA.

Question 5.
Solve each of the following L.P.P.
(i) Maximize z = 5x₁ + 6x₂ subject to 2x₁ + 3x₂ ≤ 18, 2x₁ + x₂ ≤ 12, x₁ ≥ 0, x₂ ≥ 0
Solution:
First we draw the lines AB and CD whose equations are 2x₁ + 3x₂ = 18 and 2x₁ + x₂ = 12 respectively.


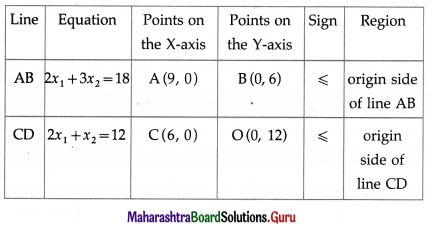
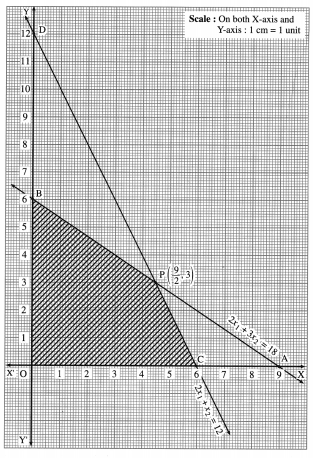
The feasible region is OCPBO which is shaded in the graph. The vertices of the feasible region are O(0, 0), C(6, 0), P and B (0,6).
P is the point of intersection of the lines
2x₁ + 3x₂ = 18 ….(1)
and 2x₁ + x₂ = 12
On subtracting, we get
2x₂ = 6 ∴ x₂ = 3
Substituting x₂ = 3 in (2), we get
2x₁ + 3 = 12 ∴ x₂ = 9
∴ P is (\(\frac{9}{2}\), 3)
The values of objective function z = 5x₁ + 6x₂ at these vertices are
z(O) = 5(0) + 6(0) = 0 + 0 = 0
z(C) = 5(6) + 6(0) = 30 + 0 = 30
z(P) = 5(\(\frac{9}{2}\)) + 6(3) = \(\frac{45}{2}\) + 18 = \(\frac{45+36}{2}=\frac{81}{2}\) = 40.5
z(B) = 5(0) + 6(3) = 0 + 18 = 18
Maximum value of z is 40.5 when x₁ = 9/2, y = 3.

(ii) Maximize z = 4x + 2y subject to 3x + y ≥ 27, x + y ≥ 21
Question is modified.
Maximize z = 4x + 2y subject to 3x + y ≤ 27, x + y ≤ 21, x ≥ 0, y ≥ 0
Solution:
First we draw the lines AB and CD whose equations are 3x + y = 27 and x + y = 21 respectively.


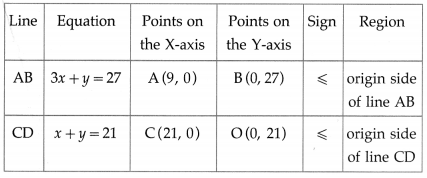
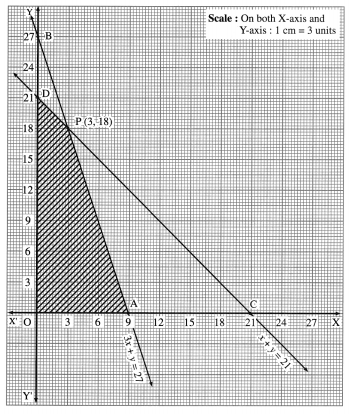
The feasible region is OAPDO which is shaded region in the graph. The vertices of the feasible region are 0(0, 0), A (9, 0), P and D(0, 21). P is the point of intersection of lines
3x + y = 27 … (1)
and x + y = 21 … (2)
On substracting, we get 2x = 6 ∴ x = 3
Substituting x = 3 in equation (1), we get
9 + y = 27 ∴ y = 18
∴ P = (3, 18)
The values of the objective function z = 4x + 2y at these vertices are
z(O) = 4(0) + 2(0) = 0 + 0 = 0
z(a) = 4(9) + 2(0) = 36 + 0 = 36
z(P) = 4(3) + 2(18) = 12 + 36 = 48
z (D) = 4(0) + 2(21) = 0 + 42 = 42
∴ 2 has minimum value 48 when x = 3, y = 18.

(iii) Maximize z = 6x + 10y subject to 3x + 5y ≤ 10, 5x + 3y ≤ 15, x ≥ 0, y ≥ 0
Solution:
First we draw the lines AB and CD whose equations are 3x + 5y = 10 and 5x + 3y = 15 respectively.


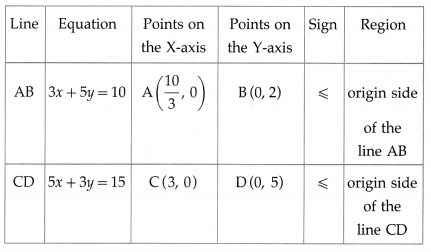
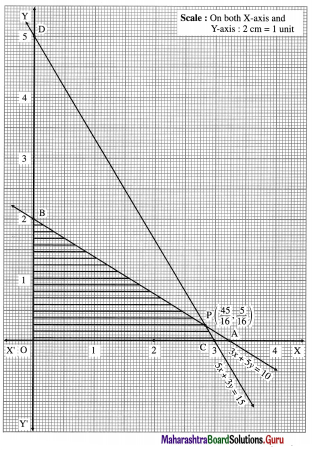
The feasible region is OCPBD which is shaded in the graph.
The vertices of the feasible region are 0(0, 0), C(3, 0), P and B (0, 2).
P is the point of intersection of the lines
3x + 5y = 10 … (1)
and 5x + 3y = 15 … (2)
Multiplying equation (1) by 5 and equation (2) by 3, we get
15x + 25y = 50
15x + 9y = 45
On subtracting, we get
16y = 5 ∴ y = \(\frac{5}{16}\)
Substituting y = \(\frac{5}{16}\) in equation (1), we get
3x + \(\frac{25}{16}\) = 10 ∴ 3x = 10 – \(\frac{25}{16}=\frac{135}{16}\)
∴ x = \(\frac{45}{16}\) ∴ P ≡ \(\left(\frac{45}{16}, \frac{5}{16}\right)\)
The values of objective function z = 6x + 10y at these vertices are
z(O) = 6(0) + 10(0) = 0 + 0 = 0
z(C) = 6(3) + 10(0) = 18 + 0 = 18
z(P) = 6\(\left(\frac{45}{16}\right)\) + 10\(\left(\frac{5}{10}\right)\) = \(\frac{270}{16}+\frac{50}{16}=\frac{320}{16}\) = 20
z(B) = 6(0) + 10(2) = 0 + 20 = 20
The maximum value of z is 20 at P\(\left(\frac{45}{16}, \frac{5}{16}\right)\) and B (0, 2) two consecutive vertices.
∴ z has maximum value 20 at each point of line segment PB where B is (0, 2) and P is \(\left(\frac{45}{16}, \frac{5}{16}\right)\).
Hence, there are infinite number of optimum solutions.

(iv) Maximize z = 2x + 3y subject to x – y ≥ 3, x ≥ 0, y ≥ 0
Solution:
First we draw the lines AB whose equation is x – y = 3.


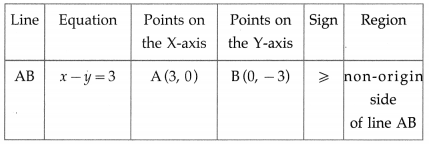
The feasible region is shaded which is unbounded.
Therefore, the value of objective function can be in- j creased indefinitely. Hence, this LPP has unbounded solution.

Question 6.
Solve each of the following L.P.P.
(i) Maximize z = 4x₁ + 3x₂ subject to 3x₁ + x₂ ≤ 15, 3x₁ + 4x₂ ≤ 24, x₁ ≥ 0, x₂ ≥ 0
Solution:
We first draw the lines AB and CD whose equations are 3x₁ + x₂ = 15 and 3x₁ + 4x₂ = 24 respectively.


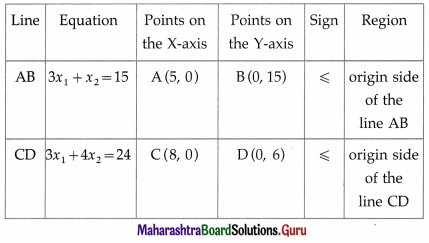
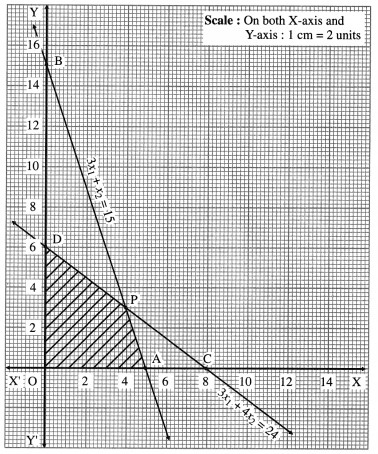
The feasible region is OAPDO which is shaded in the graph.
The Vertices of the feasible region are 0(0, 0), A(5, 0), P and D(0, 6).
P is the point of intersection of lines.
3x₁ + 4x₂ = 24 … (1)
and 3x₁ + x₂ = 15 … (2)
On subtracting, we get
3x₂ = 9 ∴ x₂ = 3
Substituting x₂ = 3 in (2), we get
3x₁ + 3 = 15
∴ 3x₁ = 12 ∴ x₁ = 4 ∴ P is (4, 3)
The values of objective function z = 4x₁ + 3x₂ at these vertices are
z(O) = 4(0) + 3(0) = 0 + 0 = 0
z(a) = 4(5) + 3(0) = 20 + 0 = 20
z(P) = 4(4) + 3(3) = 16 + 9 = 25
z(D) = 4(0) + 3(6) = 0 + 18 = 18
∴ z has maximum value 25 when x = 4 and y = 3.

(ii) Maximize z = 60x + 50y subject to x + 2y ≤ 40, 3x + 2y ≤ 60, x ≥ 0, y ≥ 0
Solution:
We first draw the lines AB and CD whose equations are x + 2y = 40 and 3x + 2y = 60 respectively.


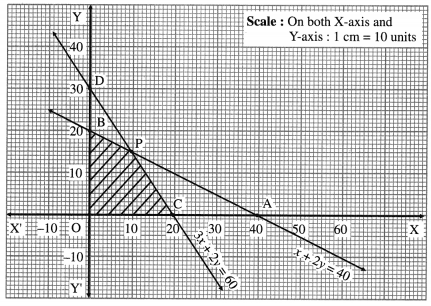
The feasible region is OCPBO which is shaded in the graph.
The vertices of the feasible region are O (0, 0), C (20, 0), P and B (0, 20).
P is the point of intersection of the lines.
3x + 2y = 60 … (1)
and x + 2y = 40 … (2)
On subtracting, we get
2x = 20 ∴ x = 10
Substituting x = 10 in (2), we get
10 + 2y = 40
∴ 2y = 30 ∴ y = 15 ∴ P is (10, 15)
The values of the objective function z = 60x + 50y at these vertices are
z(O) = 60(0) + 50(0) = 0 + 0 = 0
z(C) = 60(20) + 50(0) = 1200 + 0 = 1200
z(P) = 60(10) + 50(15) = 600 + 750 = 1350
z(B) = 60(0) + 50(20) = 0 + 1000 = 1000 .
∴ z has maximum value 1350 at x = 10, y = 15.

(iii) Maximize z = 4x + 2y subject to 3x + y ≥ 27, x + y ≥ 21, x + 2y ≥ 30; x ≥ 0, y ≥ 0
Solution:
We first draw the lines AB, CD and EF whose equations are 3x + y = 27, x + y = 21, x + 2y = 30 respectively.


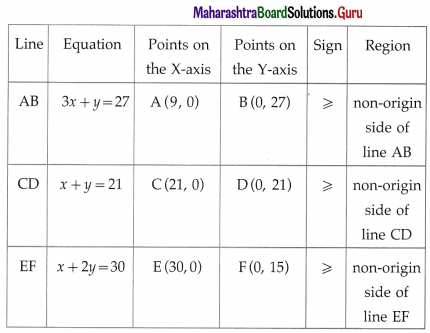
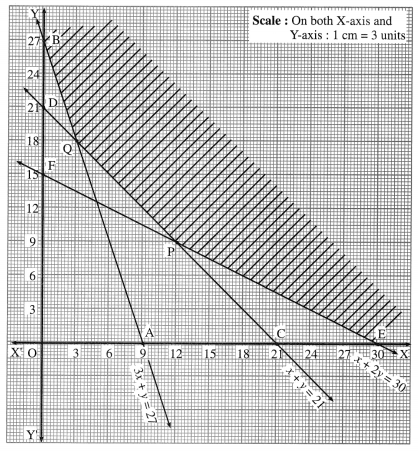
The feasible region is XEPQBY which is shaded in the graph.
The vertices of the feasible region are E (30,0), P, Q and B (0,27).
P is the point of intersection of the lines
x + 2y = 30 … (1)
and x + y = 21 … (2)
On subtracting, we get
y = 9
Substituting y = 9 in (2), we get
x + 9 = 21 ∴ x = 12
∴ P is (12, 9)
Q is the point of intersection of the lines
x + y = 21 … (2)
and 3x + y = 27 … (3)
On subtracting, we get
2x = 6 ∴ x = 3
Substituting x = 3 in (2), we get
3 + y = 21 ∴ y = 18
∴ Q is (3, 18).
The values of the objective function z = 4x + 2y at these vertices are
z(E) = 4(30) + 2(0) = 120 + 0 = 120
z(P) = 4(12) + 2(9) = 48 + 18 = 66
z(Q) = 4(3) + 2(18) = 12 + 36 = 48
z(B) = 4(0) + 2(27) = 0 + 54 = 54
∴ z has minimum value 48, when x = 3 and y = 18.

Question 7.
A carpenter makes chairs and tables. Profits are ₹140/- per chair and ₹ 210/- per table. Both products are processed on three machines : Assembling, Finishing and Polishing. The time required for each product in hours and availability of each machine is given by following table:

Formulate the above problem as L.P.P. Solve it graphically to get maximum profit.
Solution:
Let the number of chairs and tables made by the carpenter be x and y respectively.
The profits are ₹ 140 per chair and ₹ 210 per table.
∴ total profit z = ₹ (140x + 210y) This is the objective function which is to be maximized.
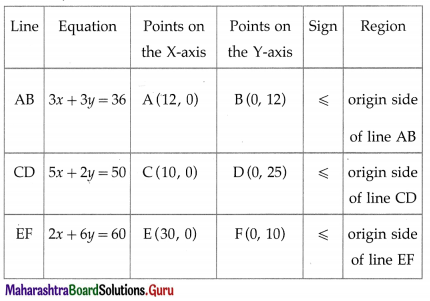
The constraints are as per the following table :

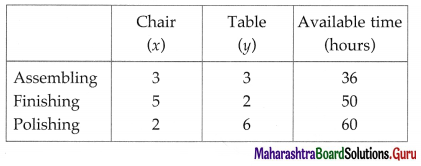
From the table, the constraints are
3x + 3y ≤ 36, 5x + 2y ≤ 50, 2x + 6y ≤ 60.
The number of chairs and tables cannot be negative.
∴ x ≥ 0, y ≥ 0
Hence, the mathematical formulation of given LPP is :
Maximize z = 140x + 210y, subject to
3x + 3y ≤ 36, 5x + 2y ≤ 50, 2x + 6y ≤ 60, x ≥ 0, y ≥ 0.
We first draw the lines AB, CD and EF whose equations are 3x + 3y = 36, 5x + 2y = 50 and 2x + 6y = 60 respectively.


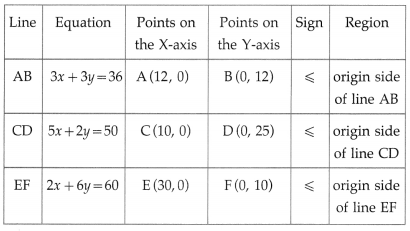
The feasible region is OCPQFO which is shaded in the graph.
The vertices of the feasible region are O (0, 0), C (10, 0), P, Q and F (0, 10).
P is the point of intersection of the lines
5x + 2y = 50 … (1)
and 3x + 3y = 36 … (2)
Multiplying equation (1) by 3 and equation (2) by 2, we get
15x + 6y = 150
6x + 6y = 72
On subtracting, we get 26
9x = 78 ∴ x = \(\frac{26}{3}\)
Substituting x = \(\frac{26}{3}\) in (2), we get
3\(\left(\frac{26}{3}\right)\) + 3y = 36
3y = 1o y = \(\frac{10}{3}\)
Q is the point of intersection of the lines
3x + 3y = 36 … (2)
and 2x + 6y = 60 … (3)
Multiplying equation (2) by 2, we get
6x + 6 y = 72
Subtracting equation (3) from this equation, we get
4x = 12 ∴ x = 3
Substituting x = 3 in (2), we get
3(3) + 3y = 36
∴ 3y = 27 ∴ y = 9
∴ Q is (3, 9).
Hence, the vertices of the feasible region are O (0, 0),
C(10, 0), P\(\left(\frac{26}{3}, \frac{10}{3}\right)\), Q(3, 9) and F(0, 10).
The values of the objective function z = 140x + 210y at these vertices are
z(O) = 140(0) + 210(0) = 0 + 0 = 0
z(C) = 140 (10) + 210(0) = 1400 + 0 = 1400
z(P) = 140\(\left(\frac{26}{3}\right)\) + 210\(\left(\frac{10}{3}\right)\) = \(\frac{3640+2100}{3}=\frac{5740}{3}\) = 1913.33
z(Q) = 140(3) + 210(9) = 420 + 1890 = 2310
z(F) = 140(0) + 210(10) = 0 + 2100 = 2100
∴ z has maximum value 2310 when x = 3 and y = 9 Hence, the carpenter should make 3 chairs and 9 tables to get the maximum profit of ₹ 2310.

Question 8.
A company manufactures bicycles and tricycles, each of which must be processed through two machines A and B. Maximum availability of Machine A and B is respectively 120 and 180 hours. Manufacturing a bicycle requires 6 hours on Machine A and 3 hours on Machine B. Manufacturing a tricycles requires 4 hours on Machine A and 10 hours on Machine B. If profits are ₹180/- for a bicycle and ₹220/- for a tricycle. Determine the number of bicycles and tricycles that should be manufactured in order to maximize the profit.
Solution:
Let x bicycles and y tricycles are to be manu¬factured. Then the total profit is z = ₹ (180x + 220y)
This is a linear function which is to be maximized. Hence, it is the objective function. The constraints are as per the following table :

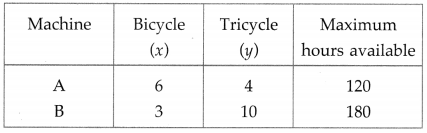
From the table, the constraints are
6x + 4y ≤ 120, 3x +10y ≤ 180
Also, the number of bicycles and tricycles cannot be i negative.
∴ x ≥ 0, y ≥ 0.
Hence, the mathematical formulation of given LPP is :
Maximize z = 180x + 220y, subject to
6x + 4y ≤ 120, 3x + 10y ≤ 180, x ≥ 0, y ≥ 0.
First we draw the lines AB and CD whose equations are 6x + 4y = 120 and 3x + 10y = 180 respectively.


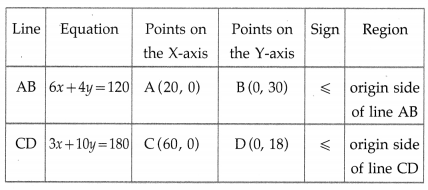
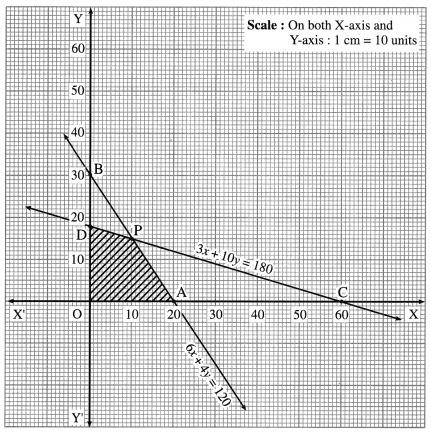
The feasible region is OAPDO which is shaded in the graph.
The vertices of the feasible region are O(0, 0), A(20, 0) P and D(0, 18).
P is the point of intersection of the lines
3x + 10y = 180 … (1)
and 6x + 4y = 120 … (2)
Multiplying equation (1) by 2, we get
6x + 20y = 360
Subtracting equation (2) from this equation, we get
16y = 240 ∴ y = 15
∴ from (1), 3x + 10(15) = 180
∴ 3x = 30 ∴ x = 10
∴ P = (10, 15)
The values of the objective function z = 180x + 220y at these vertices are
z(O) = 180(0) + 220(0) = 0 + 0 = 0
z(a) = 180(20) + 220(0) = 3600 + 0 = 3600
z(P) = 180(10) + 220(15) = 1800 + 3300 = 5100
z(D) = 180(0) +220(18) = 3960
∴ the maximum value of z is 5100 at the point (10, 15).
Hence, 10 bicycles and 15 tricycles should be manufactured in order to have the maximum profit of ₹ 5100.

Question 9.
A factory produced two types of chemicals A and B. The following table gives the units of ingredients P and Q (per kg) of chemicals A and B as well as minimum requirements of P and Q and also cost per kg. chemicals A and B :

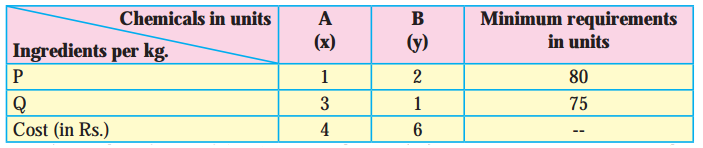
Find the number of units of chemicals A and B should be produced so as to minimize the cost.
Solution:
Let the factory produce x units of chemical A and y units of chemical B. Then the total cost is z = ₹ (4x + 6y). This is the objective function which is to be minimized.
From the given table, the constraints are
x + 2y ≥ 80, 3x + y ≥ 75.
Also, the number of units x and y of chemicals A and B cannot be negative.
∴ x ≥ 0, y ≥ 0.
∴ the mathematical formulation of given LPP is
Minimize z = 4x + 6y, subject to
x + 2y ≥ 80, 3x + y ≥ 75, x ≥ 0, y ≥ 0.
First we draw the lines AB and CD whose equations are x + 2y = 80 and 3x + y = 75 respectively.


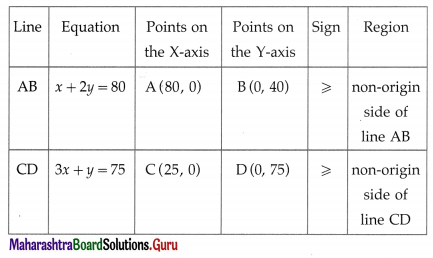
The feasible region is shaded in the graph.
The vertices of the feasible region are A (80, 0), P and D (0, 75).
P is the point of intersection of the lines
x + 2y = 80 … (1)
and 3x + y = 75 … (2)
Multiplying equation (2) by 2, we get
6x + 2 y = 150
Subtracting equation (1) from this equation, we get
5x = 70 ∴ x = 14
∴ from (2), 3(14) + y = 75
∴ 42 + y = 75 ∴ y = 33
∴ P = (14, 33)
The values of the objective function z = 4x + 6y at these vertices are
z(a) = 4(80)+ 6(0) =320 + 0 = 320
z(P) = 4(14)+ 6(33) = 56+ 198 = 254
z(D) = 4(0) + 6(75) = 0 + 450 = 450
∴ the minimum value of z is 254 at the point (14, 33).
Hence, 14 units of chemical A and 33 units of chemical B are to be produced in order to have the j minimum cost of ₹ 254.

Question 10.
A company produces mixers and food processors. Profit on selling one mixer and one food processor is ₹ 2,000/- and ₹ 3,000/- respectively. Both the products are processed through three Machines A, B, C. The time required in hours by each product and total time available in hours per week on each machine are as follows :

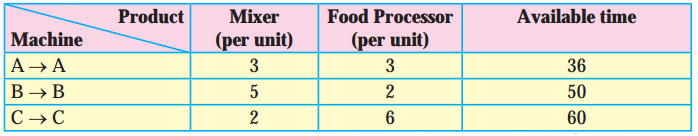
How many mixers and food processors should be produced to maximize the profit?
Solution:
Let the company produce x mixers and y food processors.
Then the total profit is z = ₹ (2000x + 3000y)
This is the objective function which is to be maximized. From the given table in the problem, the constraints are 3x + 3y ≤ 36, 5x + 2y ≤ 50, 2x + 6y ≤ 60
Also, the number of mixers and food processors cannot be negative,
∴ x ≥ 0, y ≥ 0.
∴ the mathematical formulation of given LPP is
Maximize z = 2000x + 3000y, subject to 3x + 3y ≤ 36, 5x + 2y ≤ 50, 2x + 6y ≤60, x ≥ 0, y ≥ 0.
First we draw the lines AB, CD and EF whose equations are 3x + 3y = 36, 5x + 2y = 50 and 2x + 6y = 60 respectively.


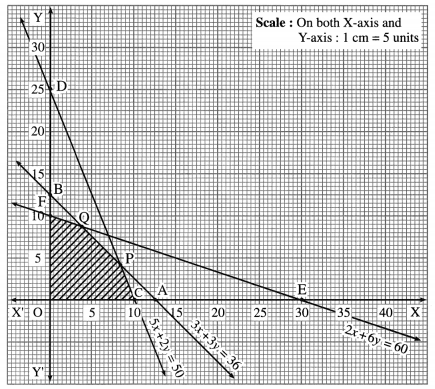
The feasible region is OCPQFO which is shaded in the graph.
The vertices of the feasible region are O(0, 0), C(10, 0), P, Q and F(0,10).
P is the point of intersection of the lines
3x + 3y = 36 … (1)
and 5x + 2y = 50 … (2)
Multiplying equation (1) by 2 and equation (2) by 3, we get
6x + 6y = 72
15x + 6y = 150
On subtracting, we get
9x = 78
∴ x = \(\frac{26}{3}\)
∴ from (1), 3\(\left(\frac{26}{3}\right)\) + 3y = 36
∴ 3y = 10
∴ y = \(\frac{10}{3}\)
∴ P = \(\left(\frac{26}{3}, \frac{10}{3}\right)\)
Q is the point of intersection of the lines
3x + 3y = 36 … (1)
and 2x + 6y = 60 … (3)
Multiplying equation (1) by 2, we get
6x + 6y = 72
Subtracting equation (3), from this equation, we get
4x = 12
∴ x = 3
∴ from (1), 3(3) + 3y = 36
∴ 3y = 27
∴ y = 9
∴ Q = (3, 9)
The values of the objective function z = 2000x + 3000y at these vertices are
z(O) = 2000(0) + 3000(0) = 0 + 0 = 0
z(C) = 2000(10) + 3000(0) = 20000 + 0 = 20000
z(P) = 2000\(\left(\frac{26}{3}\right)\) + 3000\(\left(\frac{10}{3}\right)\) = \(\frac{52000}{3}+\frac{30000}{3}=\frac{82000}{3}\)
z(Q) = 2000(3) + 3000(9) = 6000 + 27000 = 33000
z(F) = 2000(0) + 3000(10) = 30000 + 0 = 30000
∴ the maximum value of z is 33000 at the point (3, 9).
Hence, 3 mixers and 9 food processors should be produced in order to get the maximum profit of ₹ 33,000.

Question 11.
A chemical company produces a chemical containing three basic elements A, B, C so that it has at least 16 liters of A, 24 liters of B and 18 liters of C. This chemical is made by mixing two compounds I and II. Each unit of compound I has 4 liters of A, 12 liters of B, 2 liters of C. Each unit of compound II has 2 liters of A, 2 liters of B and 6 liters of C. The cost per unit of compound I is ₹ 800/- and that of compound II is ₹ 640/-. Formulate the problem as L.P.P. and solve it to minimize the cost.
Solution:
Let the company buy x units of compound I and y units of compound II.
Then the total cost is z = ₹(800x + 640y).
This is the objective function which is to be minimized.
The constraints are as per the following table :

From the table, the constraints are
4x + 2y ≥ 16, 12x + 2y ≥ 24, 2x + 6y ≥ 18.
Also, the number of units of compound I and compound II cannot be negative.
∴ x ≥ 0, y ≥ 0.
∴ the mathematical formulation of given LPP is
Minimize z = 800x + 640y, subject to 4x + 2y ≥ 16, 12x + 2y ≥ 24, 2x + 6y ≥ 18, x ≥ 0, y ≥ 0.
First we draw the lines AB, CD and EF whose equations are 4x + 2y = 16, 12x + 2y = 24 and 2x + 6y = 18


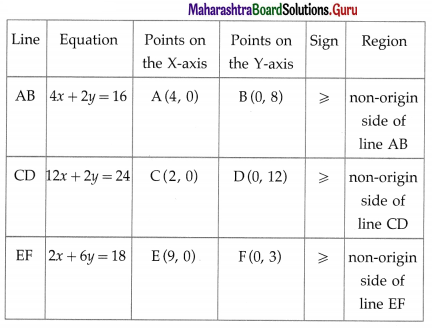
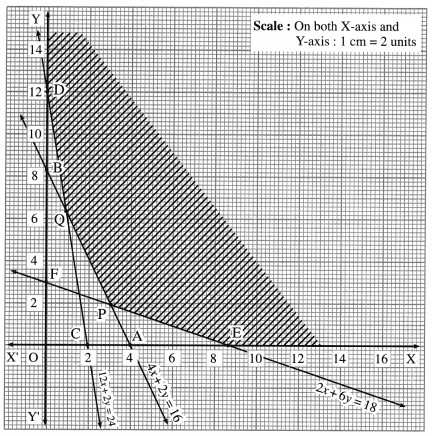
The feasible region is shaded in the graph.
The vertices of the feasible region are E(9, 0), P, Q, and D(0, 12).
P is the point of intersection of the lines
2x + 6y = 18 … (1)
and 4x + 2y = 16 … (2)
Multiplying equation (1) by 2, we get 4x + 12y = 36
Subtracting equation (2) from this equation, we get
10y = 20
∴ y = 2
∴ from (1), 2x + 6(2) = 18
∴ 2x = 6
∴ x = 3
∴ P = (3, 2)
Q is the point of intersection of the lines
12x + 2y = 24 … (3)
and 4x + 2y = 16 … (2)
On subtracting, we get
8x = 8 ∴ x = 1
∴ from (2), 4(1) + 2y = 16
∴ 2y = 12 ∴ y = 6
∴ Q = (1, 6)
The values of the objective function z = 800x + 640y at these vertices are
z(E) = 800(9)+ 640(0) =7200 + 0 = 7200
z(P) = 800(3) + 640(2) = 2400 + 1280 = 3680
z(Q) = 800(1) + 640(6) =800 + 3840 =4640
z(D) = 800(0) + 640(12) = 0 + 7680 = 7680
∴ the minimum value of z is 3680 at the point (3, 2).
Hence, the company should buy 3 units of compound I and 2 units of compound II to have the minimum cost of ₹ 3680.

Question 12.
A person makes two types of gift items A and B requires the services of a cutter and a finisher. Gift item A requires 4 hours of cutter’s time and 2 hours of finisher’s time. B requires 2 hours of cutter’s time and 4 hours of finisher’s time. The cutter and finisher have 208 hours and 152 hours available times respectively every month. The profit of one gift item of type A is ₹ 75/- and on gift item B is ₹ 125/-. Assuming that the person can sell all the gift items produced, determine how many gift items of each type should he make every month to obtain the best returns?
Solution:
Let x: number of gift item A
y: number of gift item B
As numbers of the items are never negative
x ≥ 0; y ≥ 0

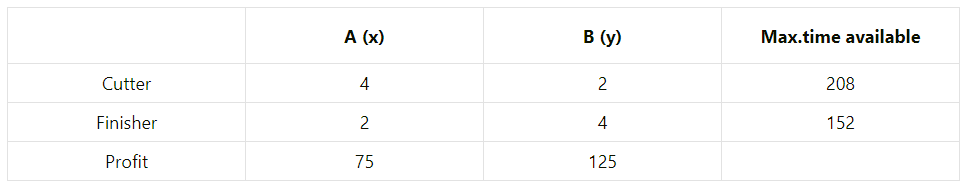
Total time required for the cutter = 4x + 2y
Maximum available time 208 hours
∴ 4x+ 2y ≤ 208
Total time required for the finisher 2x +4y
Maximum available time 152 hours
2x + 4y ≤ 152
Total Profit is 75x + 125y
∴ L.P.P. of the above problem is
Minimize z = 75x + 125y
Subject to 4x+ 2y ≤ 208
2x + 4y ≤ 152
x ≥ 0; y ≥ 0
Graphical solution

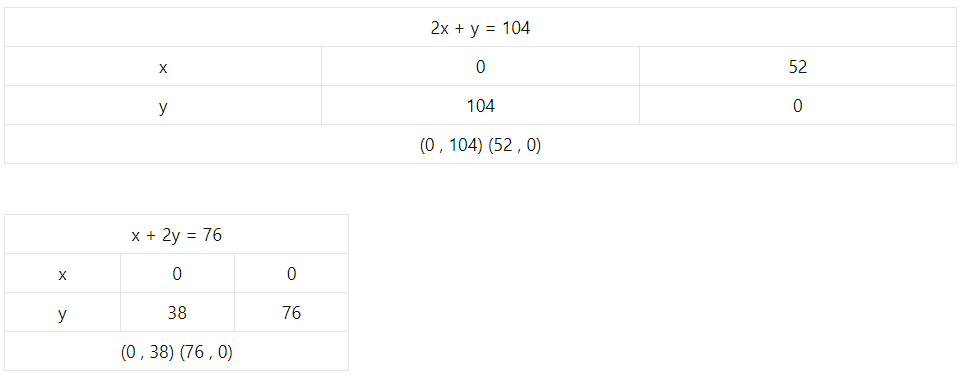
Corner points
Now, Z at
x = (75x + 125y)
O(0, 0) = 75 × 0 + 125 × 0 = 0
A(52,0) = 75 × 52 + 125 × 0 = 3900
B(44, 16) = 75 × 44 + 125 × 16 = 5300
C(0, 38) = 75 × 0 + 125 × 38 = 4750
A person should make 44 items of type A and 16 Uems of type Band his returns are ₹ 5,300.

Question 13.
A firm manufactures two products A and B on which profit earned per unit ₹3/- and ₹4/- respectively. Each product is processed on two machines M₁ and M₂. The product A requires one minute of processing time on M₁ and two minute of processing time on M₂, B requires one minute of processing time on M₁ and one minute of processing time on M₂. Machine M₁ is available for use for 450 minutes while M₂ is available for 600 minutes during any working day. Find the number of units of product A and B to be manufactured to get the maximum profit.
Solution:
Let the firm manufactures x units of product
A and y units of product B.
The profit earned per unit of A is ₹3 and B is ₹ 4.
Hence, the total profit is z = ₹ (3x + 4y).
This is the linear function which is to be maximized.
Hence, it is the objective function.
The constraints are as per the following table :

From the table, the constraints are
x + y ≤ 450, 2x + y ≤ 600
Since, the number of gift items cannot be negative, x ≥ 0, y ≥ o.
∴ the mathematical formulation of LPP is,
Maximize z = 3x + 4y, subject to x + y ≤ 450, 2x + y ≤ 600, x ≥ 0, y ≥ 0.
Now, we draw the lines AB and CD whose equations are x + y = 450 and 2x + y — 600 respectively.


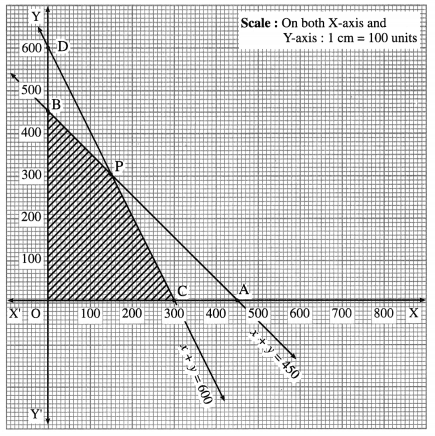
The feasible region is OCPBO which is shaded in the graph.
The vertices of the feasible region are O(0, 0), C(300, 0), P and B (0, 450).
P is the point of intersection of the lines
2x + y = 600 … (1)
and x + y = 450 … (2)
On subtracting, we get
∴ x = 150
Substituting x = 150 in equation (2), we get
150 + y = 450
∴ y = 300
∴ P = (150, 300)
The values of the objective function z = 3x + 4y at these vertices are
z(O) = 3(0) + 4(0) = 0 + 0 = 0
z(C) = 3(300) + 4(0) = 900 + 0 = 900
z(P) = 3(150) + 4(300) = 450 + 1200 = 1650
z(B) = 3(0) + 4(450) = 0 + 1800 = 1800
∴ z has the maximum value 1800 when x = 0 and y = 450 Hence, the firm gets maximum profit of ₹ 1800 if it manufactures 450 units of product B and no unit product A.

Question 14.
A firm manufacturing two types of electrical items A and B, can make a profit of ₹ 20/- per unit of A and ₹ 30/- per unit of B. Both A and B make use of two essential components a motor and a transformer. Each unit of A requires 3 motors and 2 transformers and each units of B requires 2 motors and 4 transformers. The total supply of components per month is restricted to 210 motors and 300 transformers. How many units of A and B should the manufacture per month to maximize profit? How much is the maximum profit?
Solution:
Let the firm manufactures x units of item A and y units of item B.
Firm can make profit of ₹ 20 per unit of A and ₹ 30 per unit of B.
Hence, the total profit is z = ₹ (20x + 30y).
This is the objective function which is to be maximized. The constraints are as per the following table :

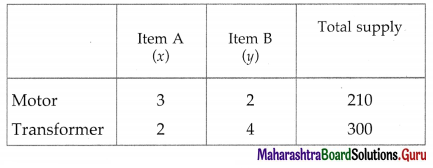
From the table, the constraints are
3x + 2y ≤ 210, 2x + 4y ≤ 300
Since, number of items cannot be negative, x ≥ 0, y ≥ 0.
Hence, the mathematical formulation of given LPP is :
Maximize z = 20x + 30y, subject to 3x + 2y ≤ 210, 2x + 4y ≤ 300, x ≥ 0, y ≥ 0.
We draw the lines AB and CD whose equations are 3x + 2y = 210 and 2x + 4y = 300 respectively.

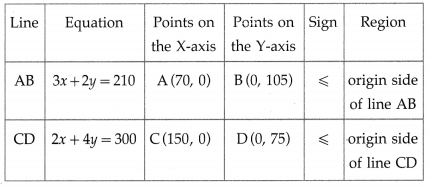
The feasible region is OAPDO which is shaded in the graph.
The vertices of the feasible region are O (0, 0), A (70, 0), P and D (0, 75).
P is the point of intersection of the lines
2x + 4y = 300 … (1)
and 3x + 2y = 210 … (2)
Multiplying equation (2) by 2, we get
6x + 4y = 420
Subtracting equation (1) from this equation, we get
∴ 4x = 120 ∴ x = 30
Substituting x = 30 in (1), we get
2(30) + 4y = 300
∴ 4y = 240 ∴ y = 60
∴ P is (30, 60)
The values of the objective function z = 20x + 30y at these vertices are
z(O) = 20(0) + 30(0) = 0 + 0 = 0
z(A) = 20(70) + 30(0) = 1400 + 0 = 1400
z(P) = 20(30) + 30(60) = 600 + 1800 = 2400
z(D) = 20(0) + 30(75) = 0 + 2250 = 2250
∴ z has the maximum value 2400 when x = 30 and y = 60. Hence, the firm should manufactured 30 units of item A and 60 units of item B to get the maximum profit of ₹ 2400.

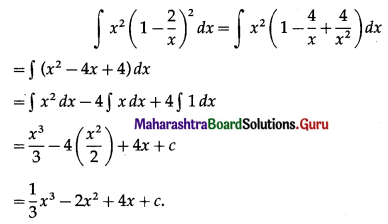
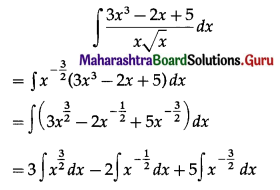
Balbharti 12th Maharashtra State Board Maths Solutions Book Pdf Chapter 3 Indefinite Integration Ex 3.4 Questions and Answers.

Maharashtra State Board 12th Maths Solutions Chapter 3 Indefinite Integration Ex 3.4

I. Integrate the following w. r. t. x:

Question 1.
\(\frac{x^{2}+2}{(x-1)(x+2)(x+3)}\)
Solution:

Question 2.
\(\frac{x^{2}}{\left(x^{2}+1\right)\left(x^{2}-2\right)\left(x^{2}+3\right)}\)
Solution:

Question 3.
\(\frac{12 x+3}{6 x^{2}+13 x-63}\)
Solution:

Question 4.
\(\frac{2 x}{4-3 x-x^{2}}\)
Solution:

Question 5.
\(\frac{x^{2}+x-1}{x^{2}+x-6}\)
Solution:

Question 6.
\(\frac{6 x^{3}+5 x^{2}-7}{3 x^{2}-2 x-1}\)
Solution:

Question 7.
\(\frac{12 x^{2}-2 x-9}{\left(4 x^{2}-1\right)(x+3)}\)
Solution:

Question 8.
\(\frac{1}{x\left(x^{5}+1\right)}\)
Solution:

Question 9.
\(\frac{2 x^{2}-1}{x^{4}+9 x^{2}+20}\)
Solution:

Question 10.
\(\frac{x^{2}+3}{\left(x^{2}-1\right)\left(x^{2}-2\right)}\)
Solution:

Question 11.
\(\frac{2 x}{\left(2+x^{2}\right)\left(3+x^{2}\right)}\)
Solution:

Question 12.
\(\frac{2^{x}}{4^{x}-3 \cdot 2^{x}-4}\)
Solution:

Question 13.
\(\frac{3 x-2}{(x+1)^{2}(x+3)}\)
Solution:

Question 14.
\(\frac{5 x^{2}+20 x+6}{x^{3}+2 x^{2}+x}\)
Solution:
Let I = ∫\(\frac{5 x^{2}+20 x+6}{x^{3}+2 x^{2}+x}\) dx

Question 15.
\(\frac{1}{x\left(1+4 x^{3}+3 x^{6}\right)}\)
Solution:

Question 16.
\(\frac{1}{x^{3}-1}\)
Solution:

Question 17.
\(\frac{(3 \sin x-2) \cdot \cos x}{5-4 \sin x-\cos ^{2} x}\)
Solution:

Question 18.
\(\frac{1}{\sin x+\sin 2 x}\)
Solution:

Question 19.
\(\frac{1}{2 \sin x+\sin 2 x}\)
Solution:

Question 20.
\(\frac{1}{\sin 2 x+\cos x}\)
Solution:

Question 21.
\(\frac{1}{\sin x \cdot(3+2 \cos x)}\)
Solution:

Question 22.
\(\frac{5 \cdot e^{x}}{\left(e^{x}+1\right)\left(e^{2 x}+9\right)}\)
Solution:

Question 23.
\(\frac{2 \log x+3}{x(3 \log x+2)\left[(\log x)^{2}+1\right]}\)
Solution: