Class 11

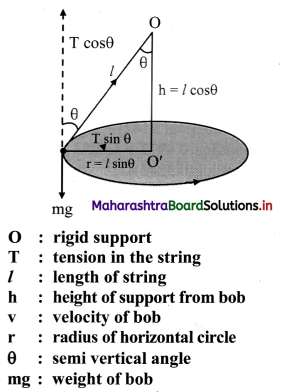
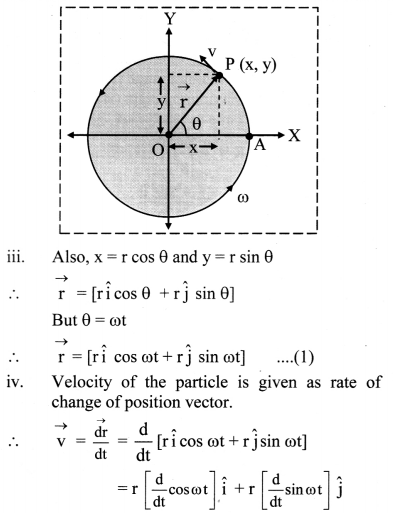
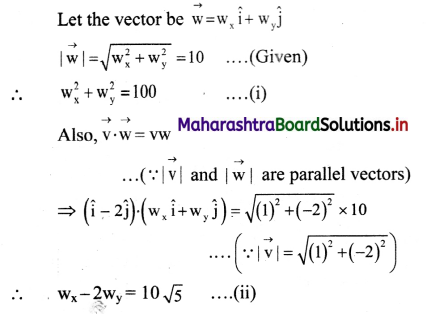
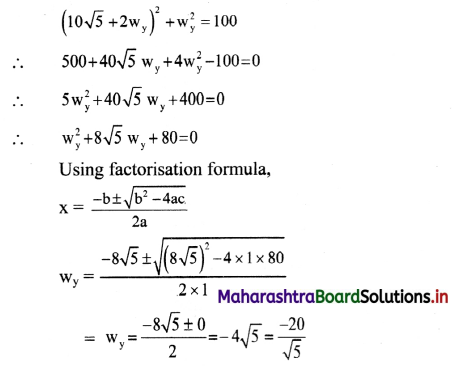
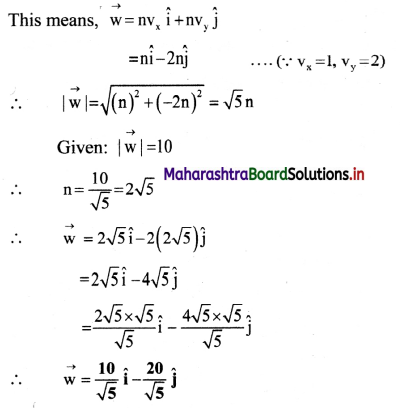
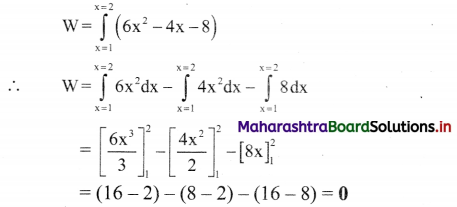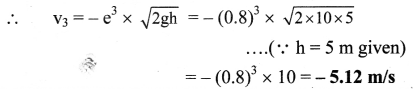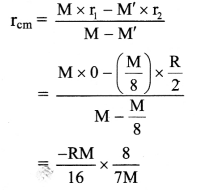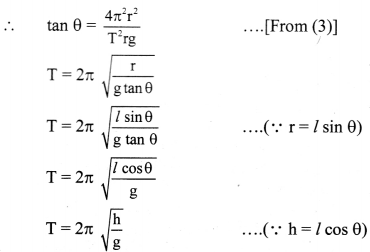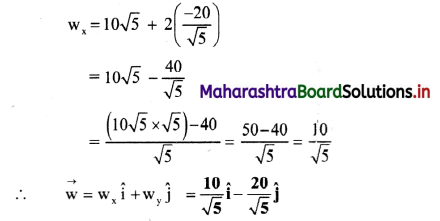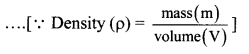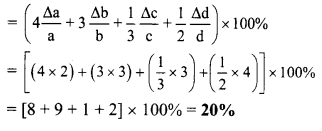Balbharti Maharashtra State Board 11th Physics Textbook Solutions Chapter 5 Gravitation Textbook Exercise Questions and Answers.

Maharashtra State Board 11th Physics Solutions Chapter 5 Gravitation

1. Choose the correct option.

Question 1.
The value of acceleration due to gravity is maximum at
(A) the equator of the Earth
(B) the centre of the Earth.
(C) the pole of the Earth.
(D) slightly above the surface of the Earth.
Answer:
(C) the pole of the Earth.

Question 2.
The weight of a particle at the centre of the Earth is _________
(A) infinite.
(B) zero.
(C) same as that at other places.
(D) greater than at the poles.
Answer:
(B) zero.

Question 3.
The gravitational potential due to the Earth is minimum at
(A) the centre of the Earth.
(B) the surface of the Earth.
(C) a points inside the Earth but not at its centre.
(D) infinite distance.
Answer:
(A) the centre of the Earth.

Question 4.
The binding energy of a satellite revolving around planet in a circular orbit is 3 × 10⁹ J. Its kinetic energy is _________
(A) 6 × 10⁹ J
(B) -3 × 10⁹ J
(C) -6 × 10⁺⁹ J
(D) 3 × 10⁺⁹J
Answer:
(D) 3 × 10⁺⁹J

2. Answer the following questions.

Question 1.
State Kepler’s law equal of area.
Answer:
The line that joins a planet and the Sun sweeps equal areas in equal intervals of time.

Question 2.
State Kepler’s law of period.
Answer:
The square of the time period of revolution of a planet around the Sun is proportional to the cube of the semimajor axis of the ellipse traced by the planet.

Question 3.
What are the dimensions of the universal gravitational constant?
Answer:
The dimensions of universal gravitational constant are: [L³M^-1T^-2].

Question 4.
Define binding energy of a satellite.
Answer:
The minimum energy required by a satellite to escape from Earth ‘s gravitational influence is the binding energy of the satellite.

Question 5.
What do you mean by geostationary satellite?
Answer:
Some satellites that revolve around the Earth in equatorial plane have same sense of rotation as that of the Earth. The also have the same period of rotation as that of the Earth i.e.. 24 hours. Due to this, these satellites appear stationary from the Earth’s surface and are known as geostationary satellites.

Question 6.
State Newton’s law of gravitation.
Answer:
Statement:
Every particle of matter attracts every other particle of matter with a force which is directly proportional to the product of their masses and inversely proportional to the square of the distance between them.

Question 7.
Define escape velocity of a satellite.
Answer:
The minimum velocity with which a both’ should he thrown vertically upwards from the surface of the Earth so that it escapes the Earth ‘s gravitational field, is called the escape velocity (v_e) of the body.

Question 8.
What is the variation in acceleration due to gravity with altitude?
Answer:
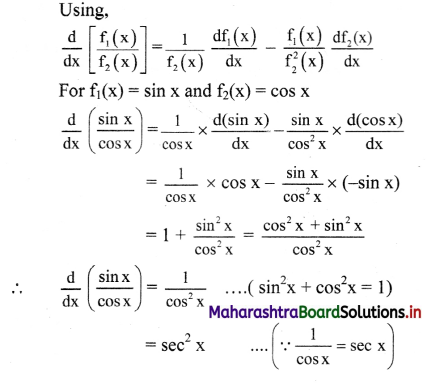
Variation in acceleration due to gravity due to altitude is given by, g_h = g\(\left(\frac{R}{R+h}\right)^{2}\)
where,
g_h = acceleration due to gravity of an object placed at h altitude
g = acceleration due to gravity on surface of the Earth
R = radius of the Earth
h = attitude height of the object from the surface of the Earth.
Hence, acceleration due to gravity decreases with increase in altitude.

Question 9.
On which factors does the escape speed of a body from the surface of Earth depend?
Answer:
The escape speed depends only on the mass and radius of the planet.
[Note: Escape velocity does not depend upon the mass of the body]

Question 10.
As we go from one planet to another planet, how will the mass and weight of a body change?
Answer:

1. As we go from one planet to another, mass of a body remains unaffected.
2. However, due to change in mass and radius of planet, acceleration due to gravity acting on the body changes as, g ∝ \(\frac{\mathrm{M}}{\mathrm{R}^{2}}\).
   Hence, weight of the body also changes as, W ∝ \(\frac{\mathrm{M}}{\mathrm{R}^{2}}\)

Question 11.
What is periodic time of a geostationary satellite?
Answer:
The periodic time of a geostationary satellite is same as that of the Earth i.e., one day or 24 hours.

Question 12.
State Newton’s law of gravitation and express it in vector form.
Answer:

1. Statement:
   Every particle of matter attracts every other particle of matter with a force which is directly proportional to the product of their masses and inversely proportional to the square of the distance between them.
2. In vector form, it can be expressed as,
   \(\overrightarrow{\mathrm{F}}_{21}=\mathrm{G} \frac{\mathrm{m}_{1} \mathrm{~m}_{2}}{\mathrm{r}^{2}}\left(-\hat{\mathrm{r}}_{21}\right)\)
   where, \(\hat{\mathbf{r}}_{21}\) is the unit vector from m₁ to m₂.
   The force \(\overrightarrow{\mathrm{F}}_{21}\) is directed from m₂ to m₁.

Question 13.
What do you mean by gravitational constant? State its SI units.
Answer:

1. From Newton’s law of gravitation,
   F = G \(\frac{\mathrm{m}_{1} \mathrm{~m}_{2}}{\mathrm{r}^{2}}\)
   where, G = constant called universal gravitational constant Its value is 667 X 10^-11 N m²/kg².
2. G = \(\frac{\mathrm{Fr}^{2}}{\mathrm{~m}_{1} \mathrm{~m}_{2}}\)
   If m₁ = m₂ = 1 kg, r = 1 m thenF = G.
   Hence, the universal gravitational constant is the force of gravitation between two particles of unit mass separated by unit distance.
3. Unit: N m²/kg² in SI system.

Question 14.
Why is a minimum two stage rocket necessary for launching of a satellite?
Answer:

1. For the projection of an artificial satellite, it is necessary for the satellite to have a certain velocity.
2. In a single stage rocket, when the fuel in first stage of rocket is ignited on the surface of the Earth, it raises the satellite vertically.
3. The velocity of projection of satellite normal to the surface of the Earth is the vertical velocity.
4. If this vertical velocity is less than the escape velocity (v_e), the satellite returns to the Earth’s surface. While, if the vertical velocity is greater than or equal to the escape velocity, the satellite will escape from Earth’s gravitational influence and go to infinity.
5. Hence, minimum two stage rocket, one to raise the satellite to desired height and another to provide required hori7ontal velocity, is necessary for launching of a satellite.

Question 15.
State the conditions for various possible orbits of a satellite depending upon the horizontal speed of projection
Answer:
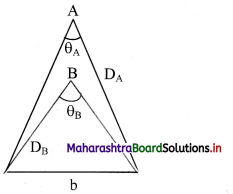
The path of the satellite depends upon the value of horizontal speed of projection v_h relative to critical velocity v_c and escape velocity v_e.
Case (I) v_h < v_c:
The orbit of satellite is an ellipse with point of projection as apogee and Earth at one of the foci. During this elliptical path, if the satellite passes through the Earth’s atmosphere. it experiences a nonconservative force of air resistance. As a result it loses energy and spirals down to the Earth.
Case (II) v_h = v_c:
The satellite moves in a stable circular orbit around the Earth.
Case (III) v_c < v_h < v_e:
The satellite moves in an elliptical orbit round the Earth with the point of projection as perigee.
Case (IV) v_h = v_e
The satellite travels along parabolic path and never returns to the point of projection. Its speed will be zero at infinity.
Case (V) v_h > v_e:
The satellite escapes from gravitational influence of Earth traversing a hyperbolic path.

3. Answer the following questions in detail.

Question 1.
Derive an expression for critical velocity of a satellite.
Answer:
Expression for critical velocity:

1. Consider a satellite of mass m revolving round the Earth at height h above its surface. Let M be the mass of the Earth and R be its radius.
2. If the satellite is moving in a circular orbit of radius (R + h) = r, its speed must be equal to the magnitude of critical velocity v_c.
3. The centripetal force necessary for circular motion of satellite is provided by gravitational force exerted by the satellite on the Earth.
   ∴ Centripetal force = Gravitational force
   
   This is the expression for critical speed at the orbit of radius (R + h).
4. The critical speed of a satellite is independent of the mass of the satellite. It depends upon the mass of the Earth and the height at which the satellite is revolving or gravitational acceleration at that altitude.

Question 2.
State any four applications of a communication satellite.
Answer:
Applications of communication satellite:

1. For the transmission of television and radiowave signals over large areas of Earth’s surface.
2. For broadcasting telecommunication.
3. For military purposes.
4. For navigation surveillance.

Question 3.
Show that acceleration due to gravity at height h above the Earth’s surface is g_h = g(\(\frac{R}{R+h}\))²
Answer:
Variation of g due to altitude:

1. Let,
   R = radius of the Earth,
   M = mass of the Earth.
   g = acceleration due to gravity at the surface of the Earth.
2. Consider a body of mass m on the surface of the Earth. The acceleration due to gravity on the Earth’s surface is given by,
   
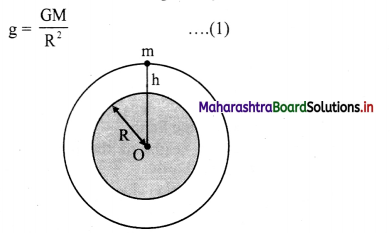
3. The body is taken at height h above the surface of the Earth as shown in figure. The acceleration due to gravity now changes to,
   g_h = \(\frac{\mathrm{GM}}{(\mathrm{R}+\mathrm{h})^{2}}\) …………. (2)
4. Dividing equation (2) by equation (1), we get,
   
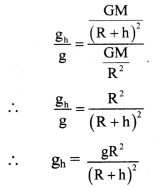
   We can rewrite,
   
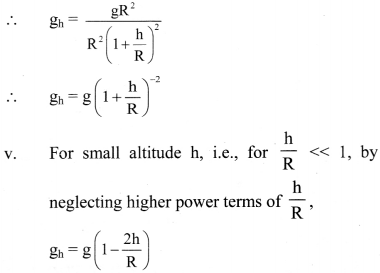
   This expression can be used to calculate the value of g at height h above the surface of the Earth as long as h<< R.

Question 4.
Draw a labelled diagram to show different trajectories of a satellite depending upon the tangential projection speed.
Answer:

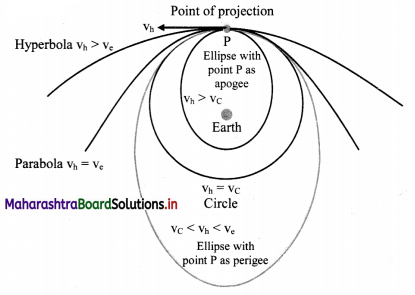
v_h = horizontal speed of projection
v _c = critical velocity
v_e = escape velocity

Question 5.
Derive an expression for binding energy of a body at rest on the Earth’s surface.
Answer:

1. Let,
   M = mass of the Earth
   m = mass of the satellite
   R = radius of the Earth.
2. Since the satellite is at rest on the Earth, v = 0
   ∴ Kinetic energy of satellite.
   K.E = \(\frac{1}{2}\) mv² = 0
3. Gravitational potential at the Earth’s surface
   = – \(\frac{\mathrm{GM}}{\mathrm{R}}\)
   ∴ Potential energy of satellite = Gravitational potential × mass of satellite
   = –\(\frac{\mathrm{GMm}}{\mathrm{R}}\)
4. Total energy of sitellite = T.E = P.E + K.E
   ∴ T.E. = –\(\frac{\mathrm{GMm}}{\mathrm{R}}\) + 0 = –\(\frac{\mathrm{GMm}}{\mathrm{R}}\)
5. Negative sign in the energy indicates that the satellite is bound to the Earth, due to gravitational force of attraction.
6. For the satellite to be free form Earth’s gravitational influence, its total energy should become positive. That energy is the binding energy of the satellite at rest on the surface of the Earth.
   ∴ B.E. = \(\frac{\mathrm{GMm}}{\mathrm{R}}\)

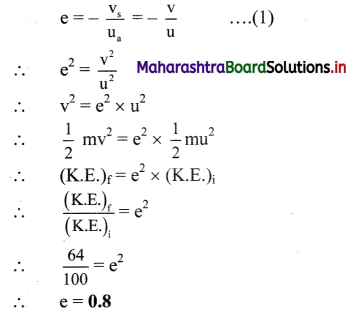
Question 6.
Why do astronauts in an orbiting satellite have a feeling of weightlessness?
Answer:

1. For an astronaut, in a satellite, the net force towards the centre of the Earth will always be, F = mg – N.
   where, N is the normal reaction.
2. In the case of a revolving satellite, the satellite is performing a circular motion. The acceleration for this motion is centripetal, which is provided by the gravitational acceleration g at the location of the satellite.
3. In this case, the downward acceleration, a_d = g, or the satellite (along with the astronaut) is in the state of free fall.
4. Thus, the net force acting on astronaut will be, F = mg – ma_d i.e., the apparent weight will be zero, giving the feeling of total weightlessness.

Question 7.
Draw a graph showing the variation of gravitational acceleration due to the depth and altitude from the Earth’s surface.
Answer:

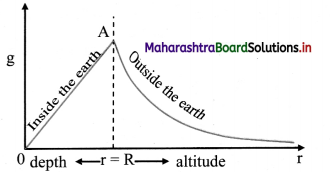
Question 8.
At which place on the Earth’s surface is the gravitational acceleration maximum? Why?
Answer:

1. Gravitational acceleration on the surface of the Earth depends on latitude of the place as well as rotation and shape of the Earth.
2. At poles, latitude θ = 90°.
   ∴ g’ = g
   i.e., there is no reduction in acceleration due to gravity at poles.
3. Also, shape of the Earth is actually an ellipsoid, bulged at equator. The polar radius of the Earth is 6356 km which minimum. As g ∝ \(\frac{1}{\mathrm{R}^{2}}\), acceleration due to gravity is maximum at poles i.e., 9.8322 m/s².

Question 9.
At which place on the Earth surface the gravitational acceleration minimum? Why?
Answer:

1. Gravitational acceleration on the surface of the Earth depends on latitude of the place as well as rotation and shape of the Earth.
2. At equator, latitude θ = 0°.
   ∴ g’ = g – Rω²
   i.e., the acceleration due to gravity ¡s reduced by amount Rω²(≈ 0.034 m/s²) at equator.
3. Also, shape of the Earth is actually an ellipsoid, bulged at equator. The equatorial radius of the Earth is 6378 km, which is maximum. As g ∝ \(\frac{1}{\mathrm{R}^{2}}\) acceleration due to gravity is minimum on equator i.e., 9.7804 m/s².

Question 10.
Define the binding energy of a satellite. Obtain an expression for binding energy of a satellite revolving around the Earth at certain attitude.
Answer:
The minimum energy required by a satellite to escape from Earth ‘s gravitational influence is the binding energy of the satellite.
Expression for binding energy of satellite revolving in circular orbit round the Earth:

1. Consider a satellite of mass m revolving at height h above the surface of the Earth in a circular orbit. It possesses potential energy as well as kinetic energy.
2. Let M be the mass of the Earth, R be the Radius of the Earth, v_c be critical velocity of satellite, r = (R + h) be thc radius of the orbit.
3. Kinetic energy of satellite = \(\frac{1}{2} \mathrm{mv}_{\mathrm{c}}^{2}=\frac{1}{2} \frac{\mathrm{GMm}}{\mathrm{r}}\)
4. The gravitational potential at a distance r from the centre of the Earth is –\(\frac{\mathrm{GM}}{\mathrm{r}}\)
   ∴ Potential energy of satellite = Gravitational potential × mass of satellite
   = –\(\frac{\mathrm{GMm}}{\mathrm{r}}\)
5. The total energy of satellite is given as T.E. = KF. + P.E.
   = \(\frac{1}{2} \frac{\mathrm{GMm}}{\mathrm{r}}-\frac{\mathrm{GMm}}{\mathrm{r}}=-\frac{1}{2} \frac{\mathrm{GMm}}{\mathrm{r}}\)
6. Total energy of a circularly orbiting satellite is negative. Negative sign indicates that the satellite is bound to the Earth, due to gravitational force of attraction. For the satellite to be free from the Earth’s gravitational influence its total energy should become zero or positive.
7. Hence the minimum energy to be supplied to unbind the satellite is +\(\frac{1}{2} \frac{\mathrm{GMm}}{\mathrm{r}}\). This is the binding energy of a satellite.

Question 11.
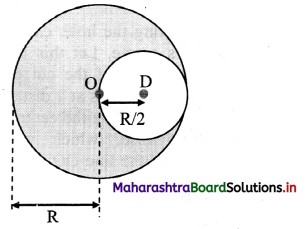
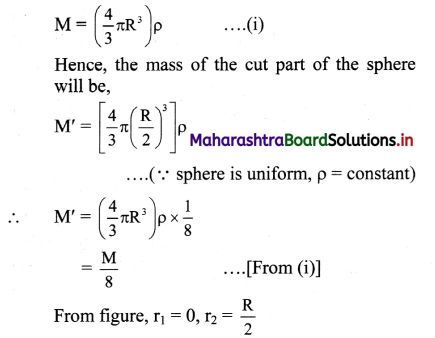
Obtain the formula for acceleration due to gravity at the depth ‘d’ below the Earth’s surface.
Answer:

1. The Earth can be considered to be a sphere made of large number of concentric uniform spherical shells.
2. When an object is on the surface of the Earth it experiences the gravitational force as if the entire mass of the Earth is concentrated at its centre.
3. The acceleration due to gravity on the surface of the Earth is, g = \(\frac{\mathrm{GM}}{\mathrm{R}^{2}}\)
4. Assuming that the density of the Earth is uniform, mass of the Earth is given by
   M = volume x density = \(\frac{4}{3}\) πR³ρ
   ∴ g = \(\frac{\mathrm{G} \times \frac{4}{3} \pi \mathrm{R}^{3} \rho}{\mathrm{R}^{2}}\) = \(\frac{4}{3}\) πRρG ………….. (1)
5. Consider a body at a point P at the depth d below the surface of the Earth as shown in figure.
   
   Here the force on a body at P due to outer spherical shell shown by shaded region, cancel out due to symmetry.
   The net force on P is only due to the inner sphere of radius OP = R – d.
6. Acceleration due to gravity because of this sphere is,
   
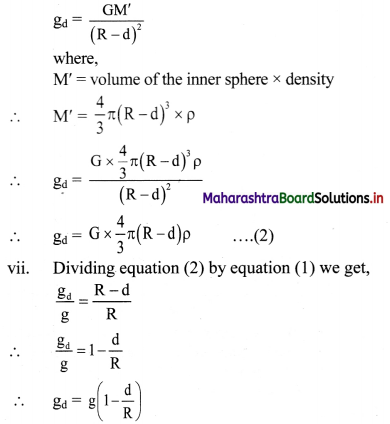
   This equation gives acceleration due to gravity at depth d below the Earth’s surface.

Question 12.
State Kepler’s three laws of planetary motion.
Answer:

• All planets move in elliptical orbits around the Sun with the Sun at one of the foci of the ellipse.
• The line that joins a planet and the Sun sweeps equal areas in equal intervals of time.
• The square of the time period of revolution of a planet around the Sun is proportional to the cube of the semimajor axis of the ellipse traced by the planet.

Question 13.
State the formula for acceleration due to gravity at depth ‘d’ and altitude ‘h’ Hence show that their ratio is equal to \(\left(\frac{R-d}{R-2 h}\right)\) by assuming that the altitude is very small as compared to the radius of the Earth.
Answer:

1. For an object at depth d, acceleration due to gravity of the Earth is given by,
   g_d = g(1 – \(\frac{\mathrm{d}}{\mathrm{R}}\)) ………………. (1)
2. Also, the acceleration due to gravity at smaller altitude h is given by,
   g_h = g(1 – \(\frac{2 \mathrm{~h}}{\mathrm{R}}\)) ……………. (2)
3. Hence, dividing equation (1) by equation (2),
   we get,
   

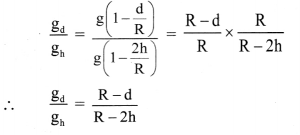
Question 14.
What is critical velocity? Obtain an expression for critical velocity of an orbiting satellite. On what factors does it depend?
Answer:
The exact horizontal velocity of projection that must be given to a satellite at a certain height so that it can revolve in a circular orbIt round the Earth is called the critical velocity or orbital velocity (v_c).
Expression for critical velocity:

1. Consider a satellite of mass m revolving round the Earth at height h above its surface. Let M be the mass of the Earth and R be its radius.
2. If the satellite is moving in a circular orbit of radius (R + h) = r, its speed must be equal to the magnitude of critical velocity v_c.
3. The centripetal force necessary for circular motion of satellite is provided by gravitational force exerted by the satellite on the Earth.
   ∴ Centripetal force = Gravitational force
   
   This is the expression for critical speed at the orbit of radius (R + h).
4. The critical speed of a satellite is independent of the mass of the satellite. It depends upon the mass of the Earth and the height at which the satellite is revolving or gravitational acceleration at that altitude.

Question 15.
Define escape speed. Derive an expression for the escape speed of an object from the surface of the earth.
Answer:

1. The minimum velocity with which a both’ should he thrown vertically upwards from the surface of the Earth so that it escapes the Earth ‘s gravitational field, is called the escape velocity (v_e) of the body.
2. As the gravitational force due to Earth becomes zero at infinite distance, the object has to reach infinite distance in order to escape.
3. Let us consider the kinetic and potential energies of an object thrown vertically upwards with escape velocity v_e.
4. On the surface of the Earth,
   K.E.= \(\frac{1}{2} \mathrm{mv}_{\mathrm{e}}^{2}\)
   P.E. = –\(\frac{\mathrm{GMm}}{\mathrm{R}}\)
   Total energy = P.E. + K.E.
   ∴ T.E. = \(\frac{1}{2} \mathrm{mv}_{\mathrm{e}}^{2}-\frac{\mathrm{GMm}}{\mathrm{R}}\) ………………. (1)
5. The kinetic energy of the object will go on decreasing with time as it is pulled back by Earth’s gravitational force. It will become zero when it reaches infinity. Thus, at infinite distance from the Earth,
   K.E. = 0
   Also,
   P.E. = –\(\frac{\mathrm{GMm}}{\infty}\) = 0
   ∴ Total energy = P.E. + K.E. = 0
6. As energy is conserved
   \(\frac{1}{2} \mathrm{mv}_{\mathrm{e}}^{2}-\frac{\mathrm{GMm}}{\mathrm{R}}=0\) ……[From(1)]
   or, v_e = \(\sqrt{\frac{2 \mathrm{GM}}{\mathrm{R}}}\)

Question 16.
Describe how an artificial satellite using two stage rocket is launched in an orbit around the Earth.
Answer:

1. Launching of a satellite in an orbit around the Earth cannot take place by use of single stage rocket. It requires minimum two stage rocket.
2. With the help of first stage of rocket, satellite can be taken to a desired height above the surface of the Earth.
3. Then the launcher is rotated in horizontal direction i.e.. through 900 using remote control and the first stage of the rocket is detached.
4. With the help of second stage of rocket, a specific horizontal velocity (v_h) is given to satellite so that it can revolve in a circular path around the Earth.
5. The satellite follows different paths depending upon the horizontal velocity provided to it.

4. Solve the following problems.

Question 1.
At what distance below the surface of the Earth, the acceleration due to gravity decreases by 10% of its value at the surface, given radius of Earth is 6400 km.
Solution:
Given: g_d = 90% of g i.e., \(\frac{\mathrm{g}_{\mathrm{d}}}{\mathrm{g}}\) = 0.9,
R = 6400km = 6.4 × 10⁶ m
To find: Distance below the Earth’s surface (d)

At distance 640 km below the surface of the Earth, value of acceleration due to gravity decreases by 10%.

Question 2.
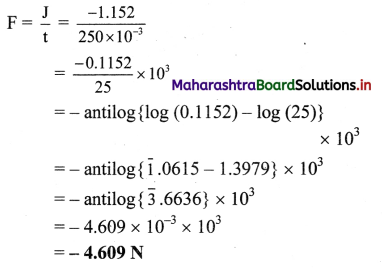
If the Earth were made of wood, the mass of wooden Earth would have been 10% as much as it is now (without change in its diameter). Calculate escape speed from the surface of this Earth.
Solution:

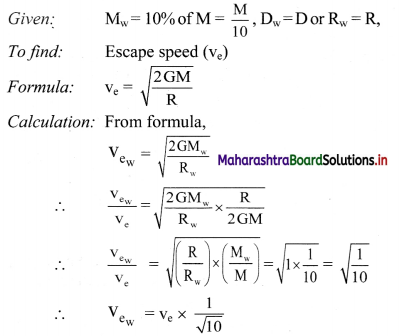
As, we know that the escape speed from surface of the Earth is 11.2 km/s, Substituting value of v_e = 11.2 km/s
V_ew = 11.2 × \(\frac{1}{\sqrt{10}}=\frac{11.2}{3.162}\)
= 11.2 × \(\frac{1}{3.162}\)
…………… [Taking square root value]
= antilog {log(1 1.2) –  Log(3.162)}
= antilog {1.0492 – 0.5000}
= antilog {0.5492} = 3.542
∴ V_ew = 3.54km/s
The escape velocity from the surface of wooden Earth is 3.54 km/s.

Question 3.
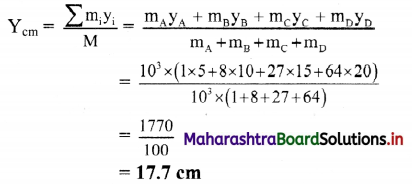
Calculate the kinetic energy, potential energy, total energy and binding energy of an artificial satellite of mass 2000 kg orbiting at a height of 3600 km above the surface of the Earth.
Given:- G = 6.67 × 10^-11 Nm²/kg²
R = 6400 km
M = 6 × 10²⁴ kg
Solution:
Given:- m = 2000 kg, h = 3600 km = 3.6 × 10⁶ m,
G = 6.67 × 10^-11 Nm²/kg²
R = 6400 km
M = 6 × 10²⁴ kg

To find: i) Kninetic energy (K.E.)
ii) Potential Energy (P.E.)
iii) Total Energy (T.E.)
iv) Binding Energy (B.E.)

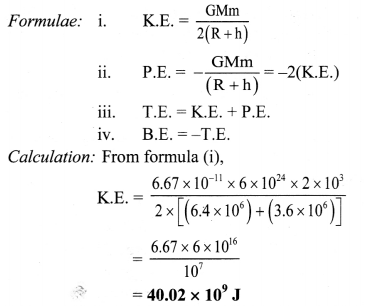
From formula (ii),
P.E. = -2 × 40.02 × 10⁹
= -80.04 × 10⁹ J
From formula (iii),
T.E. = (40.02 × 10⁹) + (-80.02 × 10⁹)
= -40.02 × 10⁹ J
From formula (iv),
B.E.= -(-40.02 × 10⁹)
= 40.02 × 10⁹ J
Kinetic energy of the satellite is 40.02 × 10⁹ J, potential energy is -80.04 × 10⁹ J, total energy is -40.02 × 10⁹ J and binding energy is 40.02 × 10⁹ J.
[Note: Total energy of orbiting satellite is negative.]

Question 4.
Two satellites A and B are revolving around a planet. Their periods of revolution are 1 hour and 8 hours respectively. The radius of orbit of satellite B is 4 × 10⁴ km. find radius of orbit of satellite A .
Solution:
Given: T_A = 1 hour, T_B = 8 hour,
r_B = 4 × 10⁴ km
To find: Radius of orbit of satellite A (r_A)

Radius of orbit of satellite A will be 1 × 10⁴ km.

Question 5.
Find the gravitational force between the Sun and the Earth.
Given Mass of the Sun = 1.99 × 10³⁰ kg
Mass of the Earth = 5.98 × 10²⁴ kg
The average distance between the Earth and the Sun = 1.5 × 10¹¹ m.
Solution:
Given: M_S = 1.99 × 10³⁰ kg
M_E = 5.98 × 10²⁴ kg, R = 1.5 × 10¹¹ m.
To find: Gravitational force between the Sun and the Earth (F)
Formula: F = \(\frac{\mathrm{Gm}_{1} \mathrm{~m}_{2}}{\mathrm{r}^{2}}\)
Calculation:As, we know, G = 6.67 × 10^-11 N m²/kg²
From formula,

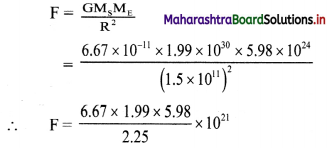
= antilog {(log(6.67) + log( 1.99) + log(5.98) – log(2.25)} × 10²¹
= antilog {(0.8241) + (0.2989) + (0.7767) – (0.3522)} × 10²¹
= antilog {1.5475} × 10²¹
= 35.28 × 10²¹
= 3.5 × 10²² N
The gravitational force between the Sun and the Earth is 3.5 × 10²² N.

Question 6.
Calculate the acceleration due to gravity at a height of 300 km from the surface of the Earth. (M = 5.98 × 10²⁴ kg, R = 6400 km).
Solution:
Given: h = 300 km = 0.3 × 10⁶ m,
M = 5.98 × 10²⁴ kg,
R = 6400km = 6.4 × 10⁶ m
G = 6.67 × 10^-11 Nm²/kg²
To find: Acceleration due to gravity at height (g_h)
Formula: g_h = \(\frac{G M}{(R+h)^{2}}\)

Calculation: From formula,
g_h = \(\frac{6.67 \times 10^{-11} \times 5.98 \times 10^{24}}{\left[\left(6.4 \times 10^{6}\right)+\left(0.3 \times 10^{6}\right)\right]^{2}}\)
= \(\frac{6.67 \times 5.98 \times 10^{13}}{(6.7)^{2} \times 10^{12}}\)
6.67 X 10” x 5.98 X iO
= antilog {log(6.67) + log(5.98) – 2log(6.7)} × 10
= antilog{0.8241 + 0.7767 – 2(0.8261)} × 10
= antilog {1.6008 – 1.6522} × 10
= antilog {\(\overline{1}\) .9486} × 10
= 0.8884 × 10 = 8.884 m/s²
Acceleration due to gravity at 300 km will be 8.884 m/s².

Question 7.
Calculate the speed of a satellite in an orbit at a height of 1000 km from the Earth’s surface. M_E = 5.98 × 10²⁴ kg, R = 6.4 × 10⁶ m.
Solution:
Given: h = 1000 km = 1 × 10⁶ m,
ME = 5.98 × 10²⁴ kg, R = 6.4 × 10⁶ m,
G = 6.67 × 10^-11 N m²/kg²
To find: Speed of satellite (v_c)

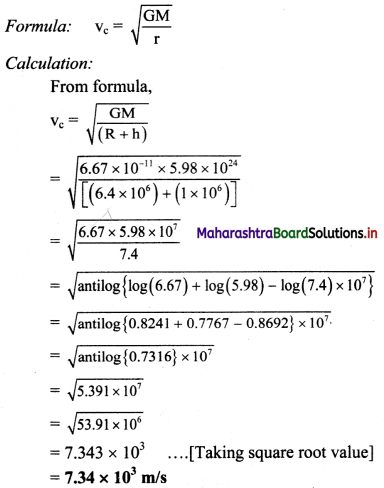
Speed of the satellite at height 1000 km is 7.34 × 10³ m/s.

Question 8.
Calculate the value of acceleration due to gravity on the surface of Mars if the radius of Mars = 3.4 × 10³ km and its mass is 6.4 × 10²³ kg.
Solution:
Given:
M = 6.4 × 10²³ kg
R = 3.4 × 10³ = 3.4 × 10⁶ m,
To find: Acceleration due to gravity on the surface of the Mars (g_M)
Formula: g = \(\frac{\mathrm{GM}}{\mathrm{R}^{2}}\)
Calculation: As, G = 6.67 × 10^-11 N m²/kg²
From formula,
g_M = \(\frac{6.67 \times 10^{-11} \times 6.4 \times 10^{23}}{\left(3.4 \times 10^{6}\right)^{2}}=\frac{6.67 \times 6.4}{3.4 \times 3.4}\)
= antilog {log(6.67) + log(6.4) – log(3.4) – log(3.4)}
= antilog {(0.8241) + (0.8062) – (0.5315) – (0.53 15)}
= antilog {0.5673}
= 3.693 m/s²
Acceleration due to gravity on the surface of Mars is 3.693 m/s².

Question 9.
A planet has mass 6.4 × 10²⁴ kg and radius 3.4 × 10⁶ m. Calculate energy required to remove on object of mass 800 kg from the surface of the planet to infinity.
Solution:
Given: M = 6.4 × 10²⁴ kg, R = 3.4 × 10⁶ m, m = 800 kg
To find:   Energy required to remove the object from surface of planet to infinity = B.E.
Formula:    B.E. = \(\frac{\mathrm{GMm}}{\mathrm{R}}\)
Calculation: We know that,
G = 6.67 × 10^-11 N m²/kg²
From formula,

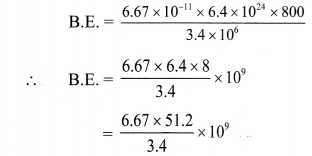
= antilog{log(6.67) + log(51.2) – log(3.4)} × 10⁹
= antilog{0.8241 + 1.7093 – 0.5315} × 10⁹
= antilog {2.0019} × 10⁹
= 1.004 × 10² × 10⁹
= 1.004 × 10¹¹ J
Energy required to remove the object from the surface of the planet is 1.004 × 10¹¹ J.
[Note: Answer calculated above ¡s in accordance with retual methods of calculation.]

Question 10.
Calculate the value of the universal gravitational constant from the given data. Mass of the Earth = 6 × 10²⁴ kg, Radius of the Earth = 6400 km and the acceleration due to gravity on the surface = 9.8 m/s²
Solution:
Given: M = 6 × 10²⁴ kg,
R = 6400km = 6.4 × 10⁶ m,
g = 9.8 m/s²
To find: Gravitational constant (G)
Formula. g = \(\frac{\mathrm{GM}}{\mathrm{R}^{2}}\)
Calculation: From formula,
G = \(\frac{\mathrm{gR}^{2}}{\mathrm{M}}\)
G = \(\frac{9.8 \times\left(6.4 \times 10^{6}\right)^{2}}{6 \times 10^{24}}=\frac{401.4 \times 10^{12}}{6 \times 10^{24}}\)
∴ G = 6.69 × 10^-11 N m²/kg²
The value of gravitational constant is 6.69 × 10^-11 N m²/kg².

Question 11.
A body weighs 5.6 kg wt on the surface of the Earth. How much will be its weight on a planet whose mass is 1/7 times the mass of the Earth and radius twice that of the Earth’s radius.
Solution:
Given: W_E = 5.6 kg-wt.,
\(\frac{\mathrm{M}_{\mathrm{p}}}{\mathrm{M}_{\mathrm{E}}}=\frac{1}{7}, \frac{\mathrm{R}_{\mathrm{p}}}{\mathrm{R}_{\mathrm{E}}}\) = 2
To find: Weight of the body on the surface of planet (W_p)
Formula: W = mg = \(\frac{\mathrm{GMm}}{\mathrm{R}^{2}}\)
Calculation: From formula,

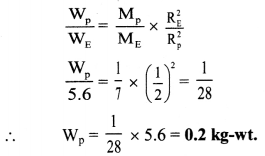
Weight of the body on the surface of a planet will be 0.2 kg-wt.
[Note: The answer given above is calculated in accordance with textual method considering the given data].

Question 12.
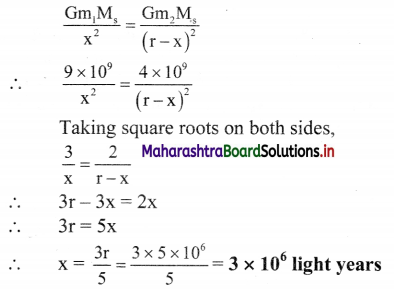
What is the gravitational potential due to the Earth at a point which is at a height of 2R_E above the surface of the Earth, Mass of the Earth is 6 × 10²⁴ kg, radius of the Earth = 6400 km and G = 6.67 × 10^-11 Nm² kg^-2.
Solution:
Given: M = 6 × 10²⁴ kg,
R_E = 6400km = 6.4 × 10⁶ m,
G = 6.67 × 10^-11 Nm²/kg²,
h = 2R_E
To find: Gravitational potential (V)
Formula: V = – \(\frac{\mathrm{GM}}{\mathrm{r}}\)
Calculation: From formula,

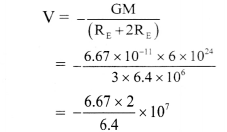
= -2.08 × 10⁷ J kg^-1
Negative sign indicates the attractive nature of gravitational potential.
Gravitational potential due to Earth will be 2.08 × 10⁷ J kg^-1 towards the centre of the Earth.
[Note: According lo definition of gravitational potential its SI unit is J/kg.]

11th Physics Digest Chapter 5 Gravitation Intext Questions and Answers

Can you recall? (Textbook Page No. 78)

Question 1.
i) What are Kepler’s laws?
ii)What is the shape of the orbits of planets?
Answer:

1. The Kepler’s laws are:
   • Kepler’s first law: The orbit of a planet is an ellipse with the Sun at one of the foci.
   • Kepler’s second law: The line joining the planet and the Sun sweeps equal areas in equal intervals of time.
   • Kepler’s third law: The square of orbital period of revolution of a planet around the Sun is directly proportional to the cube of the mean distance of the planet from the Sun.
2. The orbits of the planet are elliptical in shape.

Question 2.
When released from certain height why do objects tend to fall vertically downwards?
Answer:
When released from certain height, objects tend to fall vertically downwards because of the gravitational force exerted by the Earth.

Balbharti Maharashtra State Board 11th Physics Textbook Solutions Chapter 4 Laws of Motion Textbook Exercise Questions and Answers.

Maharashtra State Board 11th Physics Solutions Chapter 4 Laws of Motion

1. Choose the correct answer.

Question 1.
Consider the following pair of forces of equal magnitude and opposite directions:
(P) Gravitational forces are exerted on each other by two-point masses separated by a distance.
(Q) Couple of forces are used to rotate a water tap.
(R) Gravitational force and normal force are experienced by an object kept on a table.
For which of these pair/pairs the two forces do NOT cancel each other’s translational
effect?
(A) Only P
(B) Only P and Q
(C) Only R
(D) Only Q and R
Answer:
(A) Only P

Question 2.
Consider the following forces: (w) Force due to tension along a string, (x) Normal force given by a surface, (y) Force due to air resistance, and (z) Buoyant force or upthrust given by a fluid.
Which of these are electromagnetic forces?
(A) Only w, y, and z
(B) Only w, x, and y
(C) Only x, y and z
(D) All four.
Answer:
(D) All four.

Question 3.
At a given instant three-point masses m, 2m, and 3m are equidistant from each other. Consider only the gravitational forces between them. Select correct statement/s for this instance only:
(A) Mass m experiences maximum force.
(B) Mass 2m experiences a maximum force.
(C) Mass 3m experiences a maximum force.
(D) All masses experience a force of the same magnitude.
Answer:
(C) Mass 3m experiences a maximum force.

Question 4.
The rough surface of a horizontal table offers a definite maximum opposing force to initiate the motion of a block along with the table, which is proportional to the resultant normal force given by the table. Forces F₁ and F₂ act at the same angle θ with the horizontal and both are just initiating the sliding motion of the block along with the table. Force F₁ is a pulling force while the force F₂ is a pushing force. F₂ > F₁, because
(A) Component of F₂ adds up to weight to increase the normal reaction.
(B) Component of F₁ adds up to weight to increase the normal reaction.
(C) Component of F₂ adds up to the opposing force.
(D) Component of F₁ adds up to the opposing force.
Answer:
(A) Component of F2 adds up to weight to increase the normal reaction.

Question 5.
A mass 2m moving with some speed is directly approaching another mass m moving with double speed. After some time, they collide with coefficient of restitution 0.5. Ratio of their respective speeds after collision is
(A) 2/3
(B) 3/2
(C) 2
(D) ½
Answer:
(B) 3/2

Question 6.
A uniform rod of mass 2m is held horizontal by two sturdy, practically inextensible vertical strings tied at its ends. A boy of mass 3m hangs himself at one third length of the rod. Ratio of the tension in the string close to the boy to that in the other string is
(A) 2
(B) 1.5
(C) 4/3
(D) 5/3
Answer:
(B) 1.5

Question 7.
Select WRONG statement about centre of mass:
(A) Centre of mass of a ‘C’ shaped uniform rod can never be a point on that rod.
(B) If the line of action of a force passes through the centre of mass, the moment of that force is zero.
(C) Centre of mass of our Earth is not at its geometrical centre.
(D) While balancing an object on a pivot, the line of action of the gravitational force of the earth passes through the centre of mass of the object.
Answer:
(D) While balancing an object on a pivot, the line of action of the gravitational force of the earth passes through the centre of mass of the object.

Question 8.
For which of the following objects will the centre of mass NOT be at their geometrical centre?
(I) An egg
(II) a cylindrical box full of rice
(III) a cubical box containing assorted sweets
(A) Only (I)
(B) Only (I) and (II)
(C) Only (III)
(D) All, (I), (II) and (III).
Answer:
(D) All, (I), (II) and (III).

2. Answer the following questions.

Question 1.
In the following table, every entry on the left column can match with any number of entries on the right side. Pick up all those and write respectively against A, B, C and D.

Answer:

1. Force due to tension in string: Electromagnetic (EM) force, reaction force, non-conservative force.
2. Normal force: Electromagnetic (EM) force, non-conservative force. Reaction force
3. Frictional force: Electromagnetic (EM) force, reaction force, non-conservative force.
4. Resistive force offered by air or water for objects moving through it: Electromagnetic (EM) force, non-conservative force.

Question 2.
In real life objects, never travel with uniform velocity, even on a horizontal surface, unless something is done? Why
is it so? What is to be done?
Answer:

1. According to Newton’s first law, for a body to achieve uniform velocity, the net force acting on it should be zero.
2. In real life, a body in motion is constantly being acted upon by resistive or opposing force like friction, in the direction opposite to that of the motion.
3. To overcome these opposing forces, an additional external force is required. Thus, the net force is not maintained at zero, making it hard to achieve uniform velocity.

Question 3.
For the study of any kind of motion, we never use Newton’s first law of motion directly. Why should it be studied?
Answer:

1. Newton’s first law shows an equivalence between the ‘state of rest’ and ‘state of uniform motion along a straight line.’
2. Newton’s first law of motion defines force as a physical quantity that brings about a change in ‘state of rest’ or ‘state of .uniform motion along a straight line’ of a body.
3. Newton’s first law of motion defines inertia as a fundamental property of every physical object by which the object resists any change in its state of rest or of uniform motion along a straight line. Due to all these reasons, Newton’s first law should be studied.

Question 4.
Are there any situations in which we cannot apply Newton’s laws of motion? Is there any alternative for it?
Answer:

1. Limitation: Newton’s laws of motion cannot be applied for objects moving in non-inertial (accelerated) frame of reference.
   Alternative solution: For non-inertial (accelerated) frame of reference, pseudo force needs to be considered along with all the other forces.
2. Limitation: Newton’s laws of motion are applicable to point objects and rigid bodies. Alternative solution: Body needs to be approximated as a particle as the laws can be applied to individual particles in a rigid body and then summed up over the body.
3. Limitation: Newton’s laws of motion cannot be applied for objects moving with speeds comparable to that of light.
   Alternative solution: Einstein’s special theory of relativity has to be used.
4. Limitation: Newton’s laws of motion cannot be applied for studying the behaviour and interactions of objects having atomic or molecular sizes.
   Alternative solution: Quantum mechanics has to be used.

Question 5.
You are inside a closed capsule from where you are not able to see anything about the outside world. Suddenly you feel that you are pushed towards your right. Can you explain the possible cause (s)? Is it a feeling or a reality? Give at least one more situation like this.
Answer:

1. In a capsule, if we suddenly feel a push towards the right it is because the capsule is in motion and taking a turn towards the left.
2. The push towards the right is a feeling. In reality, when the capsule is beginning its turning motion towards the left, we continue in a straight line.
3. This happens because we try to maintain our direction of motion while the capsule takes a turn towards the left.
4. An external force is required to change our direction of motion. In accordance with one of the inferences from Newton’s first law of motion, in the absence of any external force, we continue to move in a straight line at constant speed and feel the sudden push in the direction opposite to the motion of the capsule.
5. Example: While travelling by bus, when the bus takes a sudden turn we feel the push in the opposite direction.

Question 6.
Among the four fundamental forces, only one force governs your daily life almost entirely. Justify the statement by stating that force.
Answer:

1. Electromagnetic force is the attractive and repulsive force between electrically charged particles.
2. Since electromagnetic force is much stronger than the gravitational force, it dominates all the phenomena on atomic and molecular scales.
3. Majority of the forces experienced in our daily life like friction, normal reaction, tension in strings, elastic forces, viscosity etc. are electromagnetic in nature.
4. The structure of atoms and molecules, the dynamics of chemical reactions etc. are governed by electromagnetic forces.

Thus, out of the four fundamental forces, electromagnetic force governs our daily life almost entirely.

Question 7.
Find the odd man out:
(i) Force responsible for a string to become taut on stretching
(ii) Weight of an object
(iii) The force due to which we can hold an object in hand.
Answer:
Weight of an object.
Reason: Weight of an object (force due to gravity) is a non-contact force while force responsible for a string to become taut (tension force) and force due to which we can hold an object in hand (normal force) are contact forces.

Question 8.
You are sitting next to your friend on ground. Is there any gravitational force of attraction between you two? If so, why are you not coming together naturally? Is any force other than the gravitational force of the earth coming in picture?
Answer:

1. Yes, there exists a gravitational force between me and my friend sitting beside each other.
2. The gravitational force between any two objects is given by, \(\overrightarrow{\mathrm{F}}=\mathrm{G} \frac{\mathrm{m}_{1} \mathrm{~m}_{2}}{\mathrm{r}^{2}}\) Where,
   G = universal gravitational constant, m₁ and m₂ = mass of the two objects, r = distance between centres of the two objects
3. Thus, me and my friend attract each other. But due to our small masses, we exert a force on each other, which is too small as compared to the gravitational force of the earth. Hence, me and my friend don’t move towards each other.
4. Apart from gravitational force of the earth, there is the normal force and frictional force acting on both me and my friend.

In Chapter 5, you will study about force of gravitation in detail.

Question 9.
Distinguish between:
(A) Real and pseudo forces,
(B) Conservative and non-conservative forces,
(C) Contact and non-contact forces,
(D) Inertial and non-inertial frames of reference.
Answer:
(A) Real and pseudo forces,

No	Real force	Pseudo Force
i.	A force which is produced due to interaction between the objects is called real force.	A pseudo force is one which arises due to the acceleration of the observer’s frame of reference.
ii.	Real forces obey Newton’s laws of motion.	Pseudo forces do not obey Newton’s laws of motion.
iii.	Real forces are one of the four fundamental forces.	Pseudo forces are not among any of the four fundamental forces.
	Example: The earth revolves around the sun in circular path due to gravitational force of attraction between the sun and the earth.	Example: Bus is moving with an acceleration (a) on a straight road in forward direction, a person of mass ‘m’ experiences a backward pseudo force of magnitude ‘ma’.

(B) Conservative and non-conservative forces,

No	Conservative	Non-conservative forces
i.	If work done by or against a force is independent’ of the actual path, the force is said to be a conservative force.	If work done by or against a force is dependent of the actual path, the force is said to be a non- conservative force.
ii.	During work done by a conservative force, the mechanical energy is conserved.	During work done by a non­ conservative force, the mechanical energy may not be conserved.
iii.	Work done is completely recoverable.	Work done is not recoverable.
	Example: gravitational force, magnetic force etc.	Example: Frictional force, air drag etc.

(C) Contact and non-contact forces,

No	Contact forces	Non-contact forces
i.	The forces experienced by a body due to physical contact are called contact forces.	The forces experienced by a body without any physical contact are called non-contact forces.
ii.	Example: gravitational force, electrostatic force, magnetostatic force etc.	Example: Frictional force, force exerted due to collision, normal reaction etc.

(D) Inertial and non-inertial frames of reference.

No.	Inertial frame of reference	Non-inertial frame of reference
i	The body moves with a constant velocity (can be zero).	The body moves with variable velocity.
ii.	Newton’s laws are	Newton’s laws are
iii.	The body does not accelerate.	The body undergoes acceleration.
iv.	In this frame, force acting on a body is a real force.	The acceleration of the frame gives rise to a pseudo force.
	Example: A rocket in inter-galactic space (gravity free space between galaxies) with all its engine shut.	Example: If a car just starts its motion from rest, then during the time of acceleration the car will be in a non- inertial frame of reference.

Question 10.
State the formula for calculating work done by a force. Are there any conditions or limitations in using it directly? If so, state those clearly. Is there any mathematical way out for it? Explain.
Answer:

1. Suppose a constant force \(\overrightarrow{\mathrm{F}}\) acting on a body produces a displacement \(\overrightarrow{\mathrm{S}}\) in the body along the positive X-direction. Then the work done by the force is given as,
   W = F.s cos θ
   Where θ is the angle between the applied force and displacement.
2. If displacement is in the direction of the force applied, θ = 0°
   W = \(\overrightarrow{\mathrm{F}}\).\(\overrightarrow{\mathrm{s}}\)

Conditions/limitations for application of work formula:

1. The formula for work done is applicable only if both force \(\overrightarrow{\mathrm{F}}\) and displacement \(\overrightarrow{\mathrm{s}}\) are constant and finite i.e., it cannot be applied when the force is variable.
2. The formula is not applicable in several real- life situations like lifting an object through several thousand kilometres since the gravitational force is not constant. It is not applicable to viscous forces like fluid resistance as they depend upon speed and thus are often not constant with time.
3. The method of integration has to be applied to find the work done by a variable force.

Integral method to find work done by a variable force:

1. Let the force vary non-linearly in magnitude between the points A and B as shown in figure (a).
2. In order to calculate the total work done during the displacement from s₁ to s₂, we need to use integration. For integration, we need to divide the displacement into large numbers of infinitesimal (infinitely small) displacements.
3. Let at P₁, the magnitude of force be F = P₁P₁‘. Due to this force, the body displaces through infinitesimally small displacement ds, in the direction of force.
   It moves from P₁ to P₂.
   ∴ \(\mathrm{d} \overrightarrow{\mathrm{s}}=\overrightarrow{\mathrm{P}_{1} \mathrm{P}_{2}}\)
4. But direction of force and displacement are same, we have
   \(\mathrm{d} \overrightarrow{\mathrm{s}}=\mathrm{P}_{1}{ }^{\prime} \mathrm{P}_{2}^{\prime}\)
5. \(\mathrm{d} \overrightarrow{\mathrm{s}}\) is so small that the force F is practically constant for the displacement. As the force is constant, the area of the strip \(\overrightarrow{\mathrm{F}} \cdot \mathrm{d} \overrightarrow{\mathrm{s}}\) is the work done dW for this displacement.
6. Hence, small work done between P₁ to P₂ is dW and is given by
   dW = \(\overrightarrow{\mathrm{F}} \cdot \mathrm{d} \overrightarrow{\mathrm{s}}\) = \(\mathrm{P}_{1} \mathrm{P}_{1}^{\prime} \times \mathrm{P}_{1}^{\prime} \mathrm{P}_{2}^{\prime}\)
   = Area of the strip P₁P₂P₂‘P₁‘.
7. The total work done can be found out by dividing the portion AB into small strips like P₁P₂P₂‘P₁‘ and taking sum of all the areas of the strips.
   ∴ W = \int_{s_{1}}^{s 2} \vec{F} \cdot d \vec{s}=\text { Area } A B B^{\prime} A^{\prime}\(\)
8. Method of integration is applicable if the exact way of variation in \(\overrightarrow{\mathrm{F}}\) and \(\overrightarrow{\mathrm{s}}\) is known and that function is integrable.
9. The work done by the non-linear variable force is represented by the area under the portion of force-displacement graph.
10. Similarly, in case of a linear variable force, the area under the curve from s₁ to s₂ (trapezium APQB) gives total work done W [figure (b)].
    

Question 11.
Justify the statement, “Work and energy are the two sides of a coin”.
Answer:

1. Work and energy both are scalar quantities.
2. Work and energy both have the same dimensions i.e., [M¹L²T^-2].
3. Work and energy both have the same units i.e., SI unit: joule and CGS unit: erg.
4. Energy refers to the total amount of work a body can do.
5. A body capable of doing more work possesses more energy and vice versa.
6. Work done on a body by a conservative force is equal to the change in its kinetic energy.

Thus, work and energy are the two sides of the same

Question 12.
From the terrace of a building of height H, you dropped a ball of mass m. It reached the ground with speed v. Is the relation mgH = \(\frac{1}{2} m \mathrm{v}^{2}\) applicable exactly? If not, how can you account for the difference? Will the ball bounce to the same height from where it was dropped?
Answer:

1. Let the ball dropped from the terrace of a building of height h have mass m. During free fall, the ball is acted upon by gravity (accelerating conservative force).
2. While coming down, the work that is done is equal to the decrease in the potential energy.
3. This work done however is not entirely converted into kinetic energy but some part of it is used in overcoming the air resistance (retarding non-conservative force). This part of energy appears in some other forms such as heat, sound, etc.
4. Thus, in this case of an accelerating conservative force along with a retarding non-conservative force, the work-energy theorem is given as, Decrease in the gravitational
   P.E. = Increase in the kinetic energy + work done against non-conservative forces.
5. Thus, the relation mgh = \(\frac{1}{2} \mathrm{mv}^{2}[latex] is not applicable when non-conservative forces are considered. The part of the energy converted to heat, sound etc also needs to be added to the equation,
6. The ball will not bounce to the same height from where it was dropped due to the loss in kinetic energy during the collision making it an inelastic collision.

Question 13.
State the law of conservation of linear momentum. It is a consequence of which law? Given an example from our daily life for conservation of momentum. Does it hold good during burst of a cracker?
Answer:

1. Statement: The total momentum of an isolated system is conserved during any interaction.
2. The law of conservation of linear momentum is a consequence of Newton’s second law of motion, (in combination with Newton’s third law)
3. Example: When a nail is driven into a wall by striking it with a hammer, the hammer is seen to rebound after striking the nail. This is because the hammer imparts a certain amount of momentum to the nail and the nail imparts an equal and opposite amount of momentum to the hammer.
   Linear momentum conservation during the burst of a cracker:
   • The law of conservation of linear momentum holds good during bursting of a cracker.
   • When a cracker is at rest before explosion, the linear momentum of the cracker is zero.
   • When cracker explodes into number of pieces, scattered in different directions, the vector sum of linear momentum of these pieces is also zero. This is as per the law of conservation of linear momentum.

Question 14.
Define coefficient of restitution and obtain its value for an elastic collision and a perfectly inelastic collision.
Answer:

i. For two colliding bodies, the negative of ratio of relative velocity of separation to relative velocity of approach is called the coefficient of restitution.

ii. Consider an head-on collision of two bodies of masses m₁ and m₂ with respective initial velocities u₁ and u₂. As the collision is head on, the colliding masses are along the same line before and after the collision. Relative velocity of approach is given as,
u_a = u₂ – u₁
Let v₁ and v₂ be their respective velocities after the collision. The relative velocity of recede (or separation) is then v_s = v₂ – v₁

iii. For a head on elastic collision, According to the principle of conservation of linear momentum,
Total initial momentum = Total final momentum

iv. For a perfectly inelastic collision, the colliding bodies move jointly after the collision, i.e., v₁ = v₂
∴ v₁ – v₂ = 0
Substituting this in equation (1), e = 0.

Question 15.
Discuss the following as special cases of elastic collisions and obtain their exact or approximate final velocities in terms
of their initial velocities.
(i) Colliding bodies are identical.
(ii) A very heavy object collides on a lighter object, initially at rest.
(iii) A very light object collides on a comparatively much massive object, initially at rest.
Answer:
The final velocities after a head-on elastic collision is given as,

i. Colliding bodies are identical
If m₁ = m₂, then v₁ = u₂ and v₂ = u₁
Thus, objects will exchange their velocities after head on elastic collision.

ii. A very heavy object collides with a lighter object, initially at rest.
Let m₁ be the mass of the heavier body and m₂ be the mass of the lighter body i.e., m₁ >> m₂; lighter particle is at rest i.e., u₂ = 0 then,

i.e., the heavier colliding body is left unaffected and the lighter body which is struck, travels with double the speed of the massive striking body.

iii. A very light object collides on a comparatively much massive object, initially at rest.
If m₁ is mass of a light body and m₂ is mass of heavy body i.e., m₁ << m₂ and u₂ = 0. Thus, m₁ can be neglected.
Hence v₁ ≅ -u₁, and v₂ ≅ 0.
i.e., the tiny (lighter) object rebounds with same speed while the massive object is unaffected.

Question 16.
A bullet of mass m₁ travelling with a velocity u strikes a stationary wooden block of mass m₂ and gets embedded into it. Determine the expression for loss in the kinetic energy of the system. Is this violating the principle of conservation of energy? If not, how can you account for this loss?
Answer:

1. A bullet of mass m₁ travelling with a velocity u, striking a stationary wooden block of mass m₂ and getting embedded into it is a case of perfectly inelastic collision.
2. In a perfectly inelastic collision, although there is a loss in kinetic energy, the principle of conservation of energy is not violated as the total energy of the system is conserved.

Loss in the kinetic energy during a perfectly inelastic head on collision:

1. Let two bodies A and B of masses m₁ and m₂ move with initial velocity [latex]\overrightarrow{\mathrm{u}_{1}}\), and \(\overrightarrow{\mathrm{u}_{2}}\) respectively such that particle A collides head- on with particle B i.e., u₁ > u₂.
2. If the collision is perfectly inelastic, the particles stick together and move with a common velocity \(\overrightarrow{\mathbf{V}}\) after the collision along the same straight line.
   loss in kinetic energy = total initial kinetic energy – total final kinetic energy,
3. By the law of conservation of momentum, m₁u₁ + m₂u₂ = (m₁ + m₂) v
   ∴ v = \(\frac{\mathrm{m}_{1} \mathrm{u}_{1}+\mathrm{m}_{2} \mathrm{u}_{2}}{\mathrm{~m}_{1}+\mathrm{m}_{2}}\)
4. Loss of Kinetic energy,
   
5. Both the masses and the term (u₁ – u₂)² are positive. Hence, there is always a loss in a perfectly inelastic collision. For a perfectly inelastic collision, as e = 0, the loss is maximum.

Question 17.
One of the effects of a force is to change the momentum. Define the quantity related to this and explain it for a variable
force. Usually when do we define it instead of using the force?
Answer:

1. Impulse is the quantity related to change in momentum.
2. Impulse is defined as the change of momentum of an object when the object is acted upon by a force for a given time interval.

Need to define impulse:

1. In cases when time for which an appreciable force acting on an object is extremely small, it becomes difficult to measure the force and time independently.
2. In such cases, however, the effect of the force i.e, the change in momentum due to the force is noticeable and can be measured.
3. For such cases, it is convenient to define impulse itself as a physical quantity.
4. Example: Hitting a ball with a bat, giving a kick to a foot-ball, hammering a nail, bouncing a ball from a hard surface, etc.

Impulse for a variable force:

1. Consider the collision between a bat and ball. The variation of the force as a function of time is shown below. The force axis is starting from zero.
2. From the graph, it can be seen that the force is zero before the impact. It rises to a maximum during the impact and decreases to zero after the impact.
3. The shaded area or the area under the curve of the force -time graph gives the product of force against the corresponding time (∆t) which is the impulse of the force.
   Area of ABCDE = F. ∆t = impulse of force
4. For a constant force, the area under the curve is a rectangle.
5. In case of a softer tennis ball, the collision time becomes larger and the maximum force becomes less keeping the area under curve of the (F – t) graph same.
   Area of ABCDE = Area of PQRST

In chapter 3, you have studied the concept of using area under the curve.

Question 18.
While rotating an object or while opening a door or a water tap we apply a force or forces. Under which conditions is this process easy for us? Why? Define the vector quantity concerned. How does it differ for a single force and for two opposite forces with different lines of action?
Answer:

1. Opening a door can be done with ease if the force applied is:
   • proportional to the mass of the object
   • far away from the axis of rotation and the direction of force is perpendicular to the line joining the axis of rotation with the point of application of force.
2. This is because, the rotational ability of a force depends not only upon the magnitude and direction of force but also on the point where the force acts with respect to the axis of rotation.
3. Rotating an object like a water tap can be done with ease if the two forces are equal in magnitude but opposite in direction are applied along different lines of action.
4. The ability of a force to produce rotational motion is measured by its turning effect called ‘moment of force’ or ‘torque’.
5. However, a moment of couple or rotational effect of a couple is also called torque.
6. For differences in the two vector quantities.

No.	Moment of a force	Moment of a couple
i.	Moment of a force is given as, \(\vec{\tau}=\vec{r} \times \vec{F}\)	Moment of a couple is given as, \(\vec{\tau}=\vec{r}_{12} \times \vec{F}_{1}=\vec{r}_{21} \times \vec{F}_{2}\)
ii.	It depends upon the axis of rotation and the point of application of the force.	It depends only upon the two forces, i.e., it is independent of the axis of rotation or the points of application of forces.
iii.	It can produce translational acceleration also, if the axis of rotation is not fixed or if friction is not enough.	Does not produce any translational acceleration, but produces only rotational or angular acceleration.
iv.	Its rotational effect can be balanced by a proper single force or by a proper couple.	Its rotational effect can be balanced only by another couple of equal and opposite torque.

Question 19.
Why is the moment of a couple independent of the axis of rotation even if the axis is fixed?
Answer:

1. Consider a rectangular sheet free to rotate only about a fixed axis of rotation, perpendicular to the plane.
2. A couple of forces \(\overrightarrow{\mathrm{F}}\) and –\(\overrightarrow{\mathrm{F}}\) is acting on the sheet at two different locations.
3. Consider the torque of the couple as two torques due to individual forces causing rotation about the axis of rotation.
4. Case 1: The axis of rotation is between the lines of action of the two forces constituting the couple. Let x and y be the perpendicular distances of the axis of rotation from the forces \(\overrightarrow{\mathrm{F}}\) and –\(\overrightarrow{\mathrm{F}}\) respectively.
   In this case, the pair of forces cause anticlockwise rotation. As a result, the direction of individual torques due to the two forces is the same.
   
5. Case 2: Lines of action of both the forces are on the same side of the axis of rotation. Let q and p be the perpendicular distances of the axis of rotation from the forces \(\overrightarrow{\mathrm{F}}\) and –\(\overrightarrow{\mathrm{F}}\)

Question 20.
Explain balancing or mechanical equilibrium. Linear velocity of a rotating fan as a whole is generally zero. Is it in
mechanical equilibrium? Justify your answer.
Answer:

1. The state in which the momentum of a system is constant in the absence of an external unbalanced force is called mechanical equilibrium.
2. A particle is said to be in mechanical equilibrium, if no net force is acting upon it.
3. In case of a system of bodies to be in mechanical equilibrium, the net force acting on any part of the system should be zero i.e., the velocity or linear momentum of all parts of the system must be constant or zero. There should be no acceleration in any part of the system.
4. Mathematically, for a system in mechanical equilibrium, \(\sum \vec{F}\) = 0.
5. In case of rotating fan, if linear velocity is zero, then the linear momentum is zero. That means there is no net force acting on the fan. Hence, the fan is in mechanical equilibrium.

Question 21.
Why do we need to know the centre of mass of an object? For which objects, its position may differ from that of the centre of gravity?
Use g = 10 m s^-2, unless, otherwise stated.
Answer:

1. Centre of mass of an object allows us to apply Newton’s laws of motion to finite objects (objects of measurable size) by considering these objects as point objects.
2. For objects in non-uniform gravitational field or whose size is comparable to that of the Earth (size at least few thousand km), the position of centre of mass will differ than that of centre of gravity.

3. Solve the following problems.

Question 1.
A truck of mass 5 ton is travelling on a horizontal road with 36 km hr^-1 stops on travelling 1 km after its engine fails suddenly. What fraction of its weight is the frictional force exerted by the road? If we assume that the story repeats for a car of mass 1 ton i.e., can moving with same speed stops in similar distance same how much will the fraction be?
[Ans: \(\frac{1}{200}\) in the both]
Solution:
Given: m_truck = 5 ton = 5000 kg,
m_car = 1 ton = 1000 kg,
u = 36km/hr = 10 m/s,
v = 0 m/s, s = 1 km = 1000 m
To find: Ratio of force of friction to the weight of vehicle
Formulae:
i. v² = u² + 2as
ii. F = ma
Calculation: From formula (i),
2 × a_truck × s = v² – u²
∴ 2 × a_truck × 1000 = 0² – 10²
∴ 200a_truck = -100
∴ a_truck = -0.05 m/s²
Negative sign indicates that velocity is decreasing
From formula (ii),
F_truck = m_truck × a_truck = 5000 × 0.05
= 250 N

Answer:
The frictional force acting on both the truck and the car is \(\frac{1}{200}\) of their weight.

Question 2.
A lighter object A and a heavier object B are initially at rest. Both are imparted the same linear momentum. Which will start with greater kinetic energy: A or B or both will start with the same energy?
[Ans: A]
Solution:

1. Let m₁ and m₂ be the masses of light object A and heavy object B and v₁ and v₂ be their respective velocities.
2. Since both are imparted with the same linear momentum,
   m₁ v₁ = m₂ v₂
3. Kinetic energy of the lighter object A
   
4. As m₁ < m₂, therefore K.E._A > K.E._B, i.e, the lighter body A has more kinetic energy.

Question 3.
As i was standing on a weighing machine inside a lift it recorded 50 kg wt. Suddenly for few seconds it recorded 45 kg wt. What must have happened during that time? Explain with complete numerical analysis. [Ans: Lift must be coming down with acceleration \(\frac{\mathrm{g}}{10}\) = 1 ms^-2]
Solution:
The weight recorded by weighing machine is always apparent weight and a measure of reaction force acting on the person. As the apparent weight (45 kg-wt) in this case is less than actual weight (50 kg-wt) the lift must be accelerated downwards during that time.

Numerical Analysis

1. Weight on the weighing machine inside the lift is recorded as 50 kg-wt
   ∴ mg = 50 kg-wt
   
2. This weight acts on the weighing machine which offers a reaction R given by the reading of the weighing machine
   ∴ R = 45kg-wt = \(\frac{9}{10}\)mg
3. The forces acting on person inside lift are as follows:
   • Weight mg downward (exerted by the earth)
   • Normal reaction (R) upward (exerted by the floor)
4. As, R < mg, the net force is in downward direction and given as,
   mg – R=ma
   But R = \(\frac{9}{10}\)mg.
   ∴ mg – \(\frac{9}{10}\)mg = ma
   ∴ \(\frac{mg}{10}\) = ma
   ∴ a = g/10
   ∴ a = 1 m/s² (∵ g = 1 m/s²)
5. Therefore, the elevator must be accelerated downwards with an acceleration of 1 m/s² at that time.

Question 4.
Figure below shows a block of mass 35 kg resting on a table. The table is so rough that it offers a self adjusting resistive force 10% of the weight of the block for its sliding motion along the table. A 20 kg wt load is attached to the block and is passed over a pulley to hang freely on the left side. On the right side there is a 2 kg wt pan attached to the block and hung freely. Weights of 1 kg wt each, can be added to the pan. Minimum how many and maximum how many such weights can be added into the pan so that the block does not slide along the table? [Ans: Min 15, maximum 21].

Solution:
Frictional (resistive) force f = 10% (weight)
= \(\frac{10}{100}\) × 35 × 10 = 35N 100

i. Consider FBD for 20 kg-wt load. Initially, the block kept on the table is moving towards left, because of the movement of block of mass 20 kg in downward direction.
Thus, for block of mass 20 kg,
ma = mg – T₁ …. (1)
Consider the forces acting on the block of mass 35 kg in horizontal direction only as shown in figure (b). Thus, the force equation for this block is, m₁a = T₁ – T₂ – f ….(2)
To prevent the block from sliding across the table,
m₁a = ma = 0
∴ T₁ = mg = 200 N ….[From (1)]
T₁ = T₂ + f ….[From (2)]
∴ T₂ + f = 200
∴ T₂ = 200 – 35 = 165 N
Thus, the total force acting on the block from right hand side should be 165 N.
∴ Total mass = 16.5 kg
∴ Minimum weight to be added = 16.5 – 2 = 14.5 kg
≈ 15 weights of 1 kg each

ii. Now, considering motion of the block towards right, the force equations for the masses in the pan and the block of mass 35 kg can be determined from FBD shown

From figure (c)
m₁a = T₂ – T₁ – f ….(iii)
From figure (d),
m₂a = m₂g – T₂ … .(iv)
To prevent the block of mass 35 kg from sliding across the table, m₁a = m₂a = 0
From equations (iii) and (iv),
T₂ = T₁ + f
T₂ = m₂g
∴ m₂g = 200 + 35 = 235 N
∴ The maximum mass required to stop the sliding = 23.5 – 2 = 21.5kg ≈ 21 weights of 1 kg
Answer:
The minimum 15 weights and maximum 21 weights of 1 kg each are required to stop the block from sliding.

Question 5.
Power is rate of doing work or the rate at which energy is supplied to the system. A constant force F is applied to a body
of mass m. Power delivered by the force at time t from the start is proportional to
(a) t
(b) t²
(c) \(\sqrt{t}\)
(d) t⁰
Derive the expression for power in terms of F, m and t.
[Ans: p = \(\frac{F^{2} t}{m}\), ∴ p ∝ t]
Solution:
Derivation for expression of power:

i. A constant force F is applied to a body of mass (m) initially at rest (u = 0).

ii. We have,
v = u + at
∴ v = 0 + at
∴ v = at …. (1)

iii. Now, power is the rate of doing work,
∴ P = \(\frac{\mathrm{d} \mathrm{W}}{\mathrm{d} \mathrm{t}}\)
∴ P = F. \(\frac{\mathrm{d} \mathrm{s}}{\mathrm{dt}}\) [∵ dW = F. ds]

iv. But \(\frac{\mathrm{d} \mathrm{s}}{\mathrm{dt}}\) = v, the instantaneous velocity of the particle.
∴ P = F.V … (2)

v. According to Newton’s second law,
F = ma … (3)

vi. Substituting equations (1) and (3) in equation (2)
P = (ma) (at)
∴ P = ma²t
∴ P = \(\frac{m^{2} a^{2}}{m}\) × t
∴ P = \(\frac{\mathrm{F}^{2}}{\mathrm{~m}} \mathrm{t}\)

vii. As F and m are constant, therefore, P ∝ t.

Question 6.
40000 litre of oil of density 0.9 g cc is pumped from an oil tanker ship into a storage tank at 10 m higher level than the ship in half an hour. What should be the power of the pump? [Ans: 2 kW]
Solution:
h = 10 m, ρ = 0.9 g/cc = 900 kg/m³, g = 10 m/s²,
V = 40000 litre = 40000 × 10³ × 10^-6 m³
= 40 m³
T = 30 min = 1800 s
To find: Power(P)
Formula: P = \(\frac{\mathrm{W}}{\mathrm{t}}=\frac{\mathrm{h} \rho \mathrm{gV}}{\mathrm{t}}\)
Calculation: From formula,
P = \(\frac{10 \times 900 \times 10 \times 40}{1800}\)
∴ P = 2000 W
∴ P = 2 kW
Answer:
The power of the pump is 2 kW.

Question 7.
Ten identical masses (m each) are connected one below the other with 10 strings. Holding the topmost string, the system is accelerated upwards with acceleration g/2. What is the tension in the 6th string from the top (Topmost string being the first string)? [Ans: 6 mg]
Solution
Consider the 6^th string from the top. The number of masses below the 6^th string is 5. Thus, FBD for the 6^th mass is given in figure (b).

Answer:
Tension in the 6^th string is 7.5 mg.
[Note: The answer given above is modified considering the correct textual concepts.]

Question 8.
Two galaxies of masses 9 billion solar mass and 4 billion solar mass are 5 million light-years apart. If, the Sun has to cross the line joining them, without being attracted by either of them, through what point it should pass? [Ans: 3 million light-years from the 9 billion solar mass]
Solution:
The Sun can cross the line joining the two galaxies without being attracted by either of them if it passes from a neutral point. A neutral point is a point on the line joining two objects where the effect of gravitational forces acting due to both the objects is nullified.
Given that;
m₁ = 9 × 10⁹ M_s
m₂ = 4 × 10⁹ M_s
r = 5 × 10⁶ light years
Let the neutral point be at distance x from mi. If sun is present at that point,

Answer: The Sun has to cross the line from a point at a distance 3 million light years from the galaxy of mass 9 billion solar mass.

Question 9.
While decreasing linearly from 5 N to 3 N, a force displaces an object from 3 m to 5 m. Calculate the work done by this force during this displacement. [Ans: 8 N]
Solution:
For a variable force, work done is given by area under the curve of force v/s displacement graph. From given data, graph can be plotted as follows:

= 8 J
Ans: Work done is 8 J.
[Note: According to the definition of work done, S.J. unit of wõrk done is joule (J)]

Alternate solution:
Work done, w = Area of trapezium ADCB
∴ W = \(\frac{1}{2}\)(AD + CB) × DC
∴ W = 1 (5N + 3N) × (5m – 3m)
= \(\frac{1}{2}\) × 8 × 2 = 8J

Question 10.
Variation of a force in a certain region is given by F = 6x² – 4x – 8. It displaces an object from x = 1 m to x = 2 m in this region. Calculate the amount of work
done. [Ans: Zero]
Solution:

Answer:
The work done is zero.

Question 11.
A ball of mass 100 g dropped on the ground from 5 m bounces repeatedly. During every bounce 64% of the potential energy is converted into kinetic energy. Calculate the following:
(a) Coefficient of restitution.
(b) Speed with which the ball comes up from the ground after third bounce.
(c) Impulse given by the ball to the ground during this bounce.
(d) Average force exerted by the ground if this impact lasts for 250 ms.
(e) Average pressure exerted by the ball on the ground during this impact if the contact area of the ball is 0.5 cm².
[Ans: 0.8, 5.12 m/s, 1.152N s, 4.608 N, 9.216 × 104 N/m²]
Solution:
Given that, for every bounce, 64% of the initial energy is converted to final energy.
i. Coefficient of restitution in case of inelastic collision is given by,

ii. From equation (1),
∴ v = – eu
∴ After first bounce,
v₁ = – eu
after second bounce,
v₂ = -ev₁ = -e(-eu)= e²u
and after third bounce,
v₃ = – ev₂ = – e(e²u) = – e³u
But u = \(\sqrt{2 \mathrm{gh}}\)

iii. Impulse given by the ball during third bounce, is,

iv. Average force exerted in 250 ms,

v. Average pressure for area
0.5 cm² = 0.5 × 10^-4m²
P = \(\frac{\mathrm{F}}{\mathrm{A}}=\frac{4.608}{0.5 \times 10^{-4}}\) = 9.216 × 10⁴ N/m²

Question 12.
A spring ball of mass 0.5 kg is dropped from some height. On falling freely for 10 s, it explodes into two fragments of
mass ratio 1:2. The lighter fragment continues to travel downwards with speed of 60 m/s. Calculate the kinetic energy supplied during explosion. [Ans: 200 J]
Solution:
m₁ + m₂ = 0.5 kg, m₁ : m₂ = 1 : 2,
m₁ = \(\frac{1}{6}\) kg,
∴ m₂ = \(\frac{1}{3}\) kg
Initially, when the ball is falling freely for 10s,
v = u + at = 0 + 10(10)
∴ v = 100 m/s = u₁ = u₂
(m₁ + m₂)v = m₁v₁ + m₂v₂
∴ 0.5 × 100 = \(\frac{1}{6}\)(60) + \(\frac{1}{3}\)v₂
∴ 50 = 10 + \(\frac{1}{3}\)v₂
∴ 40 = \(\frac{1}{3}\)v₂
∴ v₂ = 120m/s

∴ K.E. = 200J.
Ans: Kinetic energy supplied is 200 J.

Question 13.
A marble of mass 2m travelling at 6 cm/s is directly followed by another marble of mass m with double speed. After collision, the heavier one travels with the average initial speed of the two. Calculate the coefficient of restitution. [Ans: 0.5]
Solution:
Given: m₁ = 2m, m₂ = m, u₁ = 6 cm/s,
u₂ = 2u₁ = 12 cm/s,
v₁ = \(\frac{\mathrm{u}_{1}+\mathrm{u}_{2}}{2}\) = 9cm/s
To find: Coefficient of restitution(e)
Formulae:

i. m₁u₁ +m₂u₂ = m₁v₁ + m₂v₂
ii. e = \(\frac{v_{2}-v_{1}}{u_{1}-u_{2}}\)
Calculation: From formula (i),
[(2m) × 6] + (m × 12) = (2m × 9) + mv₂
∴ v₂ = 6cm/s

From formula (ii),
e = \(\frac{6-9}{6-12}\) = \(\frac{-3}{-6}\) = 0.5

Answer: The coefficient of restitution is 0.5

Question 14.
A 2 m long wooden plank of mass 20 kg is pivoted (supported from below) at 0.5 m from either end. A person of mass 40 kg starts walking from one of these pivots to the farther end. How far can the person walk before the plank topples? [Ans: 1.25 m]
Solution:
Let the person starts walking from pivot P₂ as shown in the figure.

Assume the person can walk up to distance x from P₁ before the plank topples. The plank will topple when the moment exerted by the person about P₁ is not balanced by a moment of force due to plank about P₂.

∴ For equilibrium,
40 × x = 20 × 0.5
∴ x = \(\frac{1}{4}\) = 0.25 m
Hence, the total distance walked by the person is 1.25 m.

Question 15.
A 2 m long ladder of mass 10 kg is kept against a wall such that its base is 1.2 m away from the wall. The wall is smooth but the ground is rough. The roughness of the ground is such that it offers a maximum horizontal resistive force (for sliding motion) half that of normal reaction at the point of contact. A monkey of mass 20 kg starts climbing the ladder. How far can it climb along the ladder? How much is the horizontal reaction at the wall? [Ans: 1.5 m, 15 N]
Solution:

From the figure,
Given that, AC = length of ladder = 2 m
BC= 1.2m
From Pythagoras theorem,
AB = \(\sqrt{\mathrm{AC}^{2}-\mathrm{BC}^{2}}\) = 1.6 m … (i)
Also, ∆ABC ~ ∆DD’C
∴ \(\frac{\mathrm{AB}}{\mathrm{DD}^{\prime}}\) = \(\frac{\mathrm{BC}}{\mathrm{D}^{\prime} \mathrm{C}}\) = \(\frac{\mathrm{AC}}{\mathrm{DC}}\)
∴ \(\frac{1.2}{\mathrm{D}^{\prime} \mathrm{C}}=\frac{2}{1}\)
∴ D’C = 0.6 m … (ii)

The ladder exerts horizontal force \(\overrightarrow{\mathrm{H}}\) on the wall at A and \(\overrightarrow{\mathrm{F}}\) is the force exerted on the ground at C.
As |\(\overrightarrow{\mathrm{F}}\)| = \(|\overrightarrow{\mathrm{H}}|=|\overrightarrow{\mathrm{F}}|=\frac{\mathrm{N}}{2}\) … (iii)

Let monkey climb upto distance x along BC (Horizontal) i.e., CM’ = x .. . .(iv)
Then, the net normal reaction at point C will be, N = 100 + 200 = 300N
From equation (iii),
H = \(\frac{\mathrm{N}}{2}=\frac{300}{2}\) = 150N
By condition of equilibrium, taking moments about C,
(-H × AB) + (W₁ × CD’) + (W₂ × CM’) + (F × 0)’0
∴ (-150 × 1.6) + (100 × 0.6) + (200 × x) = 0
∴ 60 + 200x = 240
∴ 200x = 180
∴ x = 0.9

From figure, it can be shown that,
∆ABC ~ ∆MM’C
∴ \(\frac{\mathrm{BC}}{\mathrm{CM}^{\prime}}\) = \(\frac{\mathrm{AC}}{\mathrm{CM}^{\prime}}\) ∴ \(\frac{\mathrm{1.2}}{\mathrm{0.9}^{\prime}}\) = \(\frac{\mathrm{2}}{\mathrm{CM}^{\prime}}\)
∴ CM = 1.5 m

Answer:

1. The monkey can climb upto 1.5 m along the ladder.
2. The horizontal reaction at wall is 150 N.

Question 16.
Four uniform solid cubes of edges 10 cm, 20 cm, 30 cm and 40 cm are kept on the ground, touching each other in order. Locate centre of mass of their system. [Ans: 65 cm, 17.7 cm]
Solution:

The given cubes are arranged as shown in figure. Let one of the comers of smallest cube lie at the origin.
As the cubes are uniform, let their centre of masses lie at their respective centres.
r_A \(\equiv\) (5, 5), r_B \(\equiv\) (20, 10), r_C \(\equiv\) = (45, 15) and r_D \(\equiv\) – (80, 20)
Also, masses of the cubes are,
m_A = \(l_{\mathrm{A}}^{3} \times \rho=10^{3} \rho\)
m_B = (20)³ρ
m_C = (30)³ρ
m_D = (40)³ρ
As the cubes are uniform, p is same for all of them.
∴ For X – co-ordinate of centre of mass of the system,

Similarly,
Y – co-ordinate of centre of mass of system is,

Answer: Centre of mass of the system is located at point (65 cm, 17.7 cm)

Question 17.
A uniform solid sphere of radius R has a hole of radius R/2 drilled inside it. One end of the hole is at the centre of the
sphere while the other is at the boundary. Locate centre of mass of the remaining sphere. [Ans: -R/14 ]
Solution:

Let the centre of the sphere be origin O. Then, r₁ be the position vector of centre of mass of uniform solid sphere and r₂ be the position vector of centre of mass of the cut-out part of the sphere.
Now, mass of the sphere is given as,

∴ Position vector of centre of mass of remaining part,

r_CM = \(\frac{-\mathbf{R}}{14}\)
(Negative sign indicates the distance is on left side of the origin.)
Ans: Position of centre of mass of remaining sphere \(\frac{-\mathbf{R}}{14}\)

Question 18.
In the following table, every item on the left side can match with any number of items on the right hand side. Select all those.

Answer:
i. Elastic collision: (b)
ii. Inelastic collision: (a), (c), (f), (e)
iii. Perfectly inelastic collision: (d)
iv. Head-on collision: (c), (f)

11th Physics Digest Chapter 4 Laws of Motion Intext Questions and Answers

Can you recall? (Textbook Page No. 47)

Question 1.
What are different types of motions?
Answer:
The various types of motion are linear, uniform linear, non-uniform linear, oscillatory, circular, periodic, and random motion.

Question 2.
What do you mean by kinematical equations and what are they?
Answer:
A set of three equations that analyses rectilinear motion of the uniformly accelerated body and help to predict the position of body are called kinematical equations.

1. Equation for velocity-time relation: v = u + at
2. Equation for position-time relation: s = ut + \(\frac{1}{2}\)at²
3. Position-velocity relation: v² = u² + 2as

Can you tell? (Textbook Page No. 48)

Question 1.
Was Aristotle correct? If correct, explain his statement with an illustration.
Answer:
Aristotle was not correct in stating that an external force is required to keep a body in uniform motion.

Question 2.
If wrong, give the correct modified version of his statement.
Answer:
For an uninterrupted motion of a body, an additional external force is required for overcoming opposing/resistive forces.

Can you tell? (Textbook Page No. 48)

Question 1.
What is then special about Newton’s first law if it is derivable from Newton’s second law?
Answer:

1. Newton’s first law shows an equivalence between the ‘state of rest’ and ‘state of uniform motion along a straight line.’
2. Newton’s first law of motion defines force as a physical quantity that brings about a change in ‘state of rest’ or ‘state of .uniform motion along a straight line’ of a body.
3. Newton’s first law of motion defines inertia as a fundamental property of every physical object by which the object resists any change in its state of rest or of uniform motion along a straight line.
4. Due to all these reasons, Newton’s first law should be studied.

Balbharti Maharashtra State Board 11th Physics Textbook Solutions Chapter 3 Motion in a Plane Textbook Exercise Questions and Answers.

Maharashtra State Board 11th Physics Solutions Chapter 3 Motion in a Plane

1. Choose the correct option.

Question 1.
An object thrown from a moving bus is on example of __________
(A) Uniform circular motion
(B) Rectilinear motion
(C) Projectile motion
(D) Motion in one dimension
Answer:
(C) Projectile motion

Question 2.
For a particle having a uniform circular motion, which of the following is constant ____________.
(A) Speed
(B) Acceleration
(C) Velocity
(D) Displacement
Answer:
(A) Speed

Question 3.
The bob of a conical pendulum undergoes ___________
(A) Rectilinear motion in horizontal plane
(B) Uniform motion in a horizontal circle
(C) Uniform motion in a vertical circle
(D) Rectilinear motion in vertical circle
Answer:
(B) Uniform motion in a horizontal circle

Question 4.
For uniform acceleration in rectilinear motion which of the following is not correct?
(A) Velocity-time graph is linear
(B) Acceleration is the slope of velocity time graph
(C) The area under the velocity-time graph equals displacement
(D) Velocity-time graph is nonlinear
Answer:
(D) Velocity-time graph is nonlinear

Question 5.
If three particles A, B and C are having velocities \(\overrightarrow{\mathrm{v}}_{A}\), \(\overrightarrow{\mathrm{v}}_{B}\) and \(\overrightarrow{\mathrm{v}}_{C}\) which of the following formula gives the relative velocity of A with respect to B
(A) \(\overrightarrow{\mathrm{v}}_{A}\) + \(\overrightarrow{\mathrm{v}}_{B}\)
(B) \(\overrightarrow{\mathrm{v}}_{A}\) – \(\overrightarrow{\mathrm{v}}_{C}\) + \(\overrightarrow{\mathrm{v}}_{B}\)
(C) \(\overrightarrow{\mathrm{v}}_{A}\) – \(\overrightarrow{\mathrm{v}}_{B}\)
(D) \(\overrightarrow{\mathrm{v}}_{C}\) – \(\overrightarrow{\mathrm{v}}_{A}\)
Answer:
(C) \(\overrightarrow{\mathrm{v}}_{A}\) – \(\overrightarrow{\mathrm{v}}_{B}\)

2. Answer the following questions.

Question 1.
Separate the following in groups of scalar and vectors: velocity, speed, displacement, work done, force, power, energy, acceleration, electric charge, angular velocity.
Answer:
Scalars
Speed, work done, power, energy, electric charge.

Vectors
Velocity, displacement, force, acceleration, angular velocity (pseudo vector).

Question 2.
Define average velocity and instantaneous velocity. When are they same?
Answer:
Average velocity:

1. Average velocity (\(\overrightarrow{\mathrm{v}}_{\mathrm{av}}\)) of an object is the displacement (\(\Delta \overrightarrow{\mathrm{x}}\)) of the object during the time interval (∆t) over which average velocity is being calculated, divided by that time interval.
2. Average velocity = (\(\frac{\text { Displacement }}{\text { Time interval }}\))
   \(\overrightarrow{\mathrm{V}_{\mathrm{av}}}=\frac{\overrightarrow{\mathrm{x}}_{2}-\overrightarrow{\mathrm{x}}_{1}}{\mathrm{t}_{2}-\mathrm{t}_{1}}=\frac{\Delta \overrightarrow{\mathrm{x}}}{\Delta \mathrm{t}}\)
3. Average velocity is a vector quantity.
4. Its SI unit is m/s and dimensions are [M⁰L¹T^-1]
5. For example, if the positions of an object are x +4 m and x = +6 m at times t = O and t = 1 minute respectively, the magnitude of its average velocity during that time is V_av = (6 – 4)1(1 – 0) = 2 m per minute and its direction will be along the positive X-axis.
   ∴ \(\overrightarrow{\mathrm{v}}_{\mathrm{av}}\) = 2 i m/min
   Where, i = unit vector along X-axis.

Instantaneous velocity:

1. The instantaneous velocity (\(\overrightarrow{\mathrm{V}}\)) is the limiting value of ¡he average velocity of the object over a small time interval (∆t) around t when the value of lime interval goes to zero.
2. It is the velocity of an object at a given instant of time.
3. \(\overrightarrow{\mathrm{v}}=\lim _{\Delta t \rightarrow 0} \frac{\Delta \overrightarrow{\mathrm{x}}}{\Delta \mathrm{t}}=\frac{\mathrm{d} \overrightarrow{\mathrm{x}}}{\mathrm{dt}}\)
   where \(\frac{\mathrm{d} \overrightarrow{\mathrm{x}}}{\mathrm{dt}}\) derivative of \(\overrightarrow{\mathrm{x}}\) with respect to t.

In case of uniform rectilinear motion, i.e., when an object is moving with constant velocity along a straight line, the average and instantaneous velocity remain same.

Question 3.
Define free fall.
Answer:
The motion of any object under the influence of gravity alone is called as free fall.

Question 4.
If the motion of an object is described by x = f(t) write formulae for instantaneous velocity and acceleration.
Answer:

1. Instantaneous velocity of an object is given as,
   \(\overrightarrow{\mathrm{v}}=\lim _{\Delta t \rightarrow 0} \frac{\Delta \overrightarrow{\mathrm{x}}}{\Delta \mathrm{t}}=\frac{\mathrm{d} \overrightarrow{\mathrm{x}}}{\mathrm{dt}}\)
2. Motion of the object is given as, x = f(t)
3. The derivative f ‘(f) represents the rate of change of the position f (t) at time t, which is the instantaneous velocity of the object.
   ∴ \(\vec{v}=\frac{\mathrm{d} \overrightarrow{\mathrm{x}}}{\mathrm{dt}}\) = f'(t)
4. Acceleration is defined as the rate of change of velocity with respect to time.
5. The second derivative of the position function f “(t) represents the rate of change of velocity i.e., acceleration.
   ∴ \(\overrightarrow{\mathrm{a}}=\frac{\Delta \overrightarrow{\mathrm{v}}}{\Delta \mathrm{t}}=\frac{\mathrm{d}^{2} \mathrm{x}}{\mathrm{dt}^{2}}\) = f”(t)

Question 5.
Derive equations of motion for a particle moving in a plane and show that the motion can be resolved in two independent motions in mutually perpendicular directions.
Answer:

1. Consider an object moving in an x-y plane. Let the initial velocity of the object be \(\overrightarrow{\mathrm{u}}\) at t = 0 and its velocity at time t be \(\overrightarrow{\mathrm{v}}\).
2. As the acceleration is constant, the average acceleration and the instantaneous acceleration will be equal.
   
   This is the first equation of motion in vector form.
3. Let the displacement of the object from time t
   = 0 to t be \(\overrightarrow{\mathrm{s}}\)
   For constant acceleration, \(\overrightarrow{\mathrm{v}}_{\mathrm{av}}=\frac{\overrightarrow{\mathrm{v}}+\overrightarrow{\mathrm{u}}}{2}\)
   
   This is the second equation of motion in vector form.
4. Equations (1) and (2) can be resolved into their x and y components so as to get corresponding scalar equations as follows.
   v_x = u_x + a_xt ………….. (3)
   v_y = u_y + a_y t …………… (4)
   s_x = u_xt + \(\frac{1}{2}\) a_xt² ………….. (5)
   s_y = u_yt + \(\frac{1}{2}\) a_yt² ………..(6)
5. It can be seen that equations (3) and (5) involve only the x components of displacement, velocity and acceleration while equations (4) and (6) involve only the y components of these quantities.
6. Thus, the motion along the x direction of the object is completely controlled by the x components of velocity and acceleration while that along the y direction is completely controlled by the y components of these quantities.
7. This shows that the two sets of equations are independent of each other and can be solved independently.

Question 6.
Derive equations of motion graphically for a particle having uniform acceleration, moving along a straight line.
Answer:

1. Consider an object starting from position x = 0 at time t = 0. Let the velocity at time (t = 0) and t be u and v respectively.
2. The slope of line PQ gives the acceleration. Thus
   ∴ a = \(\frac{\mathrm{v}-\mathrm{u}}{\mathrm{t}-0}=\frac{\mathrm{v}-\mathbf{u}}{\mathrm{t}}\)
   ∴ v = u + at …………… (1)
   This is the first equation of motion.
3. The area under the curve in velocity-time graph gives the displacement of the object.
   ∴ s = area of the quadrilateral OPQS = area of rectangle OPRS + area of triangle PQR.
   = ut + \(\frac{1}{2}\) (v – u) t
   But, from equation (1)
   at = v – u
   ∴ s = ut + \(\frac{1}{2}\) at²
   This is the second equation of motion,
4. The velocity is increasing linearly with time as acceleration is constant. The displacement is given as,
   
   ∴ s = (v² – u²) / (2a)
   ∴ v² – u² = 2as
   This is the third equation of motion.

Question 7.
Derive the formula for the range and maximum height achieved by a projectile thrown from the origin with initial velocity \(\vec{u}\) at an angel θ to the horizontal.
Answer:
Expression for range:

1. Consider a body projected with velocity \(\vec{u}\), at an angle θ of projection from point O in the co-ordinate system of the XY- plane, as shown in figure.
2. The initial velocity \(\vec{u}\) can be resolved into two rectangular components:
   
   u_x = u cos θ (Horizontal component)
   u_y = u sin θ (Vertical component)
3. The horizontal component remains constant throughout the motion due to the absence of any force acting in that direction, while the vertical component changes according to
   v_y = u_y + a_yt
   with a_y = -g and u_y = u sinθ
4. Thus, the components of velocity of the projectile at time t are given by,
   v_x = u_x = u cos θ
   v_y = u_x – gt = usin θ – gt
5. Similarly, the components of displacements of the projectile in the horizontal and vertical directions at time t are given by,
   s = (u cosθ) t
   s_y = (usinθ)t – \(\frac{1}{2}\) gt²
6. At the highest point, the time of ascent of the projectile is given as,
   t_A = \(\frac{u \sin \theta}{g}\) …………..(2)
7. The total time in air i.e., time of flight is given as, T = 2t_A = \(\frac{2u \sin \theta}{g}\) …… (3)
8. The total horizontal distance travelled by the particle in this time T is given as,
   R = u_x ∙ T
   R = u cos θ ∙ (2t_A)
   R = u cos θ ∙ \(\frac{2 \mathrm{u} \sin \theta}{\mathrm{g}}\) ……………[From (3)]
   R = \(\frac{u^{2}(2 \sin \theta \cdot \cos \theta)}{g}\)
   R = \(\frac{\mathrm{u}^{2} \sin 2 \theta}{\mathrm{g}}\) ………..[∵ sin 2θ = 2sin∙cosθ]
   This is required expression for horizontal range of the projectile.

Expression for maximum height of a projectile:
The maximum height H reached by the projectile is the distance travelled along the vertical (y) direction in time t_A.

Substituting s_y = H and t = t_a in equation (1),
we have,
H = (u sin θ)t_A – \(\frac{1}{2}\) gt_A²

This equation represents maximum height of projectile.

Question 8.
Show that the path of a projectile is a parabola.
Answer:

1. Consider a body projected with velocity initial velocity \(\vec{u}\) , at an angle θ of projection from point O in the co-ordinate system of the XY-plane. as shown in figure.
   
2. The initial velocity \(\vec{u}\) can be resolved into two rectangular components:
   u_x = u cos θ (Horizontal component)
   u_y = u sin θ (Vertical component)
3. The horizontal component remains constant throughout the motion due to the absence of any force acting in that direction, while the vertical component changes according to,
   v_y = u_y + a_y t
   with a_y, = -g and u_y = u sinθ
4. Thus, the components of velocity of the projectile at time t are given by,
   v_x = u_x = u cosθ
   v_y = u_y – gt = u sinθ – gt
5. Similarly, the components of displacements of the projectile in the horizontal and vertical directions at time t are given by,
   s_x = (u cosθ)t …………..(1)
   s_y = (u sinθ)t – \(\frac{1}{2}\) gt² ………………. (2)
6. As the projectile starts from x = O, we can use
   s_x = x and s_y = y.
   Substituting s_x = x in equation (1),
   x = (u cosθ) t
   ∴ t = \(\frac{\mathrm{X}}{(\mathrm{u} \cos \theta)}\) …………….. (3)
   Substituting, s_y in equation (2),
   y = (u sinθ)t – \(\frac{1}{2}\) gt² …………… (4)
   Substituting equation (3) in equation (4), we have,
   y = u sin θ (\(\frac{\mathrm{X}}{(\mathrm{u} \cos \theta)}\)) – \(\frac{1}{2}\) (\(\frac{\mathrm{X}}{(\mathrm{u} \cos \theta)}\))² g
   ∴ y = x (tan θ) – (\(\frac{g}{2 u^{2} \cos ^{2} \theta}\))x² ………………. (5)
   Equation (5) represents the path of the projectile.
7. If we put tan θ = A and g/2u²cos²θ = B then equation (5) can be written as y = Ax – Bx² where A and B are constants. This is equation of parabola. Hence, path of projectile is a parabola.

Question 9.
What is a conical pendulum? Show that its time period is given by 2π \(\sqrt{\frac{l \cos \theta}{g}}\), where l is the length of the string, θ is the angle that the string makes with the vertical and g is the acceleration due to gravity.
Answer:
A simple pendulum, Ch i given such a motion that the bob describes a horizontal circle and the string making a constant angle with the vertical describes a cone, is called a conical pendulum.

1. Consider a bob of mass m tied to one end of a string of length ‘P and other end is fixed to rigid support.
2. Let the bob be displaced from its mean position and whirled around a horizontal circle of radius ‘r’ with constant angular velocity ω, then the bob performs U.C.M.
3. During the motion, string is inclined to the vertical at an angle θ as shown in the figure above.
4. In the displaced position, there are two forces acting on the bob.
   • The weight mg acting vertically downwards.
   • The tension T acting upward along the string.
5. The tension (T) acting in the string can be resolved into two components:
   • T cosθ acting vertically upwards.
   • T sinθ acting horizontally towards centre of the circle.
6. Since, there is no net force, vertical component T cosθ balances the weight and horizontal component T sinθ provides the necessary centripetal force.
   ∴ T cos θ = mg ……………. (1)
   T sin θ = \(\frac{\mathrm{mv}^{2}}{\mathrm{r}}\) = mrω² ……….. (2)
7. Dividing equation (2) by (1),
   tan θ = \(\frac{\mathrm{v}^{2}}{\mathrm{rg}}\) ……….. (3)
   Therefore, the angle made by the string with the vertical is θ = tan^-1 (\(\frac{\mathrm{v}^{2}}{\mathrm{rg}}\))
8. Since we know v = \(\frac{2 \pi \mathrm{r}}{\mathrm{T}}\)
   
   where l is length of the pendulum and h is the vertical distance of the horizontal circle from the fixed point O.

Question 10.
Define angular velocity. Show that the centripetal force on a particle undergoing uniform circular motion is -mω²\(\vec{r}\).
Answer:
Angular velocity of a particle is the rate of change of angular displacement.

Expression for centripetal force on a particle undergoing uniform circular motion:
i) Suppose a particle is performing U.C.M in anticlockwise direction.
The co-ordinate axes are chosen as shown in the figure.
Let,
A = initial position of the particle which lies on positive X-axis
P = instantaneous position after time t
θ = angle made by radius vector
ω = constant angular speed
\(\overrightarrow{\mathrm{r}}\) = instantaneous position vector at time t

ii) From the figure,
\(\overrightarrow{\mathrm{r}}=\hat{\mathrm{i}} \mathrm{x}+\hat{\mathrm{j}} \mathrm{y}\)
where, \(\hat{\mathrm{i}}\) and \(\hat{\mathrm{j}}\) are unit vectors along X-axis and Y-axis respectively.


Negative sign shows that direction of acceleration is opposite to the direction of position vector. Equation (3) is the centripetal acceleration.
vii) Magnitude of centripetal acceleration is given by a = ω²r

viii) The force providing this acceleration should also be along the same direction, hence centripetal.
∴ \(\overrightarrow{\mathrm{F}}\) = m\(\overrightarrow{\mathrm{a}}\) = -mω²\(\overrightarrow{\mathrm{r}}\)
This is the expression for the centripetal force on a particle undergoing uniform circular motion.

ix) Magnitude of F = mω²r = \(\frac{\mathrm{mv}^{2}}{\mathrm{r}}\) = mωv

[Note: The definition of angular velocity is not mentioned in this chapter but is in Ch.2 Mathematical Methods.]

3. Solve the following problems.

Question 1.
An aeroplane has a run of 500 m to take off from the runway. It starts from rest and moves with constant acceleration to cover the runway in 30 sec. What is the velocity of the aeroplane at the take off ?
Answer:
Given: Length of runway (s) = 500 m, t = 30 s
To find: Velocity (y)
Formulae. i) s = ut + \(\frac{1}{2}\) at²
ii) v = u + at
Calculation: As the plane was initially at rest, u = 0
From formula (1),
500 = 0 + \(\frac{1}{2}\) × a × (30)²
∴ 500 = 450 a
∴ a = \(\frac{10}{9}\) m/s²
From formula (ii),
v = 0 + \(\frac{10}{9}\) × 30
∴ v = \(\frac{100}{3}\) m/s = (\(\frac{100}{3} \times \frac{18}{5}\)) km/hr
∴ v = 120 km/hr
The velocity of the aeroplane at the take off is 120 km/hr.

Question 2.
A car moving along a straight road with a speed of 120 km/hr, is brought to rest by applying brakes. The car covers a distance of 100 m before it stops. Calculate
(i) the average retardation of the car
(ii) time taken by the car to come to rest.
Answer:
Given: u = 120 kmh^-1 = 120 × \(\frac{5}{18}\) = \(\frac{100}{3}\) ms^-1
s = 100 m, v = 0
To find: i) Average retardation of the car (a)
ii) Time taken by car (t)

Formulae: i) v² – u² = 2as
ii) v = u + at
Calculation: From formula (i),

i) Average retardation of the car is \(\frac{50}{9}\) ms² (in magnitude).
ii) Time taken by the car to come to rest is 6 s.

Question 3.
A car travels at a speed of 50 km/hr for 30 minutes, at 30 km/hr for next 15 minutes and then 70 km/hr for next 45 minutes. What is the average speed of the car?
Answer:
Given: v₁ = 50 km/hr. t₁ = 30 minutes = 0.5 hr,
v₂ = 30 km/hr, t₂ = 15 minutes = 0.25 hr,
v₃ = 70 km/hr, t₃ = 45 minutes 0.75 hr
To find: Average speed of car (v_av)
Formula v_av = \(\frac{\text { total path length }}{\text { total time interval }}\)
Calculation:
Path length,
x₁ = v₁ × t₁ = 50 × 0.5 = 25km
x₂ = v₂ × t₂ = 30 × 0.25 = 7.5 km
x₃ = v₃ × t₃ = 70 × 0.75 = 52.5 km
From formula,
v_av = \(\frac{x_{1}+x_{2}+x_{3}}{t_{1}+t_{2}+t_{3}}\)
∴ v_av = \(\frac{25+7.5+52.5}{0.5+0.25+0.75}=\frac{85}{1.5}\)
∴ v_av = 56.66 km/hr

Question 4.
A velocity-time graph is shown in the adjoining figure.

Determine:

1. initial speed of the car
2. maximum speed attained by the car
3. part of the graph showing zero acceleration
4. part of the graph showing constant retardation
5. distance travelled by the car in first 6 sec.

Answer:

1. Initial speed is at origin i.e. 0 m/s.
2. Maximum speed attained by car, v_max = speed from A to B = 20 m/s.
3. The part of the graph which shows zero acceleration is between t = 3 s and t = 6 s i.e., AB. This is because, during AB there is no change in velocity.
4. The graph shows constant retardation from t = 6 s to t = 8 s i.e., BC.
5. Distance travelled by car in first 6 s
   = Area of OABDO
   = A(△OAE) + A(rect. ABDE)
   = \(\frac{1}{2}\) × 3 × 20 + 3 × 20
   = 30 + 60
   ∴ Distance travelled by car in first 6 s = 90 m

Question 5.
A man throws a ball to maximum horizontal distance of 80 meters. Calculate the maximum height reached.
Answer:
Given: R = 80m
To find: Maximum height reached (H_max)
Formula: R_max = 4H_max
Calculation: From formula,
∴ H_max = \(\frac{\mathrm{R}_{\max }}{4}=\frac{80}{4}\) = 20 m
The maximum height reached by the ball is 20m.

Question 6.
A particle is projected with speed v₀ at angle θ to the horizontal on an inclined surface making an angle Φ (Φ < θ) to the horizontal. Find the range of the projectile along the inclined surface.
Answer:

i) The equation of trajectory of projectile is given by,
y(tan θ)x – (\(\frac{\mathrm{g}}{2 \mathrm{u}^{2} \cos ^{2} \theta}\))x² …………..(1)

ii) In this case to find R substitute,
y = R sinΦ ………….. (2)
x = R cosΦ ………….. (3)

iii) From equations (1), (2) and (3),
we have,

Question 7.
A metro train runs from station A to B to C. It takes 4 minutes in travelling from station A to station B. The train halts at station B for 20 s. Then it starts from station B and reaches station C in the next 3 minutes. At the start, the train accelerates for 10 sec to reach the constant speed of 72 km/hr. The train moving at the constant speed is brought to rest in 10 sec. at next station.
(i) Plot the velocity-time graph for the train travelling from station A to B to C.
(ii) Calculate the distance between stations A, B, and C.
Answer:

The metro train travels from station A to station B in 4 minutes = 240 s.
The trains halts at station B for 20 s.
The train travels from station B’ to station C in 3 minutes= 180 s.
∴ Total time taken by the metro train in travelling from station A to B to C
= 240 + 20 + 180 = 440 s.
At start, the train accelerates for 10 seconds to reach a constant speed of 72 km/hr = 20 m/s.
The train moving is brought to rest in 10 s at next station.
The velocity-time graph for the train travelling from station A to B to C is as follows:
Distance travelled by the train from station A to station B
= Area of PQRS
= A ( △PQQ’) A (☐QRR’) + A(SRR’)
= (\(\frac{1}{2}\) × 10 × 20 + (220 × 20) + (\(\frac{1}{2}\) 10 × 20)
= 100 + 4400 + 100
= 4600m = 4.6km

Distance travelled by the train from station B’ to station C
= Area of EFGD
= A(△EFF’) + A(☐F’FGG’) + A(△DGG’)
= (\(\frac{1}{2}\) × 10 × 20) × (160 × 20) + (\(\frac{1}{2}\) × 10 × 20)
= 100 + 3200 + 100
= 3400m = 3.4km

Question 8.
A train is moving eastward at 10 m/sec. A waiter is walking eastward at 1.2m/sec; and a fly is flying toward the north across the waiter’s tray at 2 m/s. What is the velocity of the fly relative to Earth.
Answer:
Given

Question 9.
A car moves in a circle at the constant speed of 50 m/s and completes one revolution in 40 s. Determine the magnitude of the acceleration of the car.
Answer:
Given: v = 50 m/s, t = 40 s, s = 2πr
To find: acceleration (a)
Formulae: i) v = \(\frac{\mathrm{s}}{\mathrm{t}}\)
ii) a = \(\frac{\mathrm{v}^{2}}{\mathrm{r}}\)
Calculation: From formula (i),
50 = \(\frac{2 \pi \mathrm{r}}{40}\)
∴ r = \(\frac{50 \times 40}{2 \pi}\)
∴ r = \(\frac{1000}{\pi}\) cm
From formula (ii),
a = \(\frac{v^{2}}{r}=\frac{50^{2}}{1000 / \pi}\)
∴ a = \(\frac{5 \pi}{2}\) = 7.85 m/s²
The magnitude of acceleration of the car is 7.85 m/s.

Alternate method:
Given: v = 50 m/s, t = 40 s,
To find: acceleration (a)
Formula: a = rω² = vω
Calculation: From formula,
a = vω
= v(\(\frac{2 \pi}{\mathrm{t}}\))
= 50(\(\frac{2 \times 3.142}{40}\))
= \(\frac{5}{2}\) × 3.142
∴ a = 7.85m/s²

Question 10.
A particle moves in a circle with constant speed of 15 m/s. The radius of the circle is 2 m. Determine the centripetal acceleration of the particle.
Answer:
Given: v = 15 m/s, r = 2m
To find: Centripetal acceleration (a)
Formula: a = \(\frac{\mathrm{v}^{2}}{\mathrm{r}}\)
Calculation: From formula,
a = \(\frac{(15)^{2}}{2}=\frac{225}{2}\)
∴ a = 112.5m/s²
The centripetal acceleration of the particle is 112.5 m/s².

Question 11.
A projectile is thrown at an angle of 30° to the horizontal. What should be the range of initial velocity (u) so that its range will be between 40m and 50 m? Assume g = 10 m s^-2.
Answer:
Given: 40 ≤ R ≤ 50, θ = 300, g = 10 m/s²
To find: Range of initial velocity (u)
Formula: R = \(\frac{\mathrm{u}^{2} \sin (2 \theta)}{\mathrm{g}}\)
Calculation: From formula,
The range of initial velocity,

∴ 21.49m/s ≤ u ≤ 24.03m/s
The range of initial velocity should be between 21.49 m/s ≤ u ≤ 24.03 m/s.

11th Physics Digest Chapter 3 Motion in a Plane Intext Questions and Answers

Can you recall? (Textbook Page No. 30)

Question 1.
What ¡s meant by motion?
Answer:
The change ¡n the position of an object with respect to its surroundings is called motion.

Question 2.
What Is rectilinear motion?
Answer:
Motion in which an object travels along a straight line is called rectilinear motion.

Question 3.
What is the difference between displacement and distance travelled?
Answer:

• Displacement is the shortest distance between the initial and final points of movement.
• Distance is the actual path followed by a body between the points in which it moves.

Question 4.
What is the difference between uniform and non-uniform motion?
Answer:

• A body is said to have uniform motion if it covers equal distances in equal intervals of time.
• A body is said to have non-uniform motion if it covers unequal distances in equal intervals of time.

Internet my friend (Textbook Page No. 44)

i. hyperphysics.phy-astr.gsu.eduJhbase/mot.html#motcon
ii. www .college-physics.comlbook/mechanics
[Students are expected to visit the above mentioned webs ires and collect more information.]

Balbharti Maharashtra State Board 11th Physics Textbook Solutions Chapter 2 Mathematical Methods Textbook Exercise Questions and Answers.

Maharashtra State Board 11th Physics Solutions Chapter 2 Mathematical Methods

1. Choose the correct option.

Question 1.
The resultant of two forces 10 N and 15 N acting along + x and – x-axes respectively, is
(A) 25 N along + x-axis
(B) 25 N along – x-axis
(C) 5 N along + x-axis
(D) 5 N along – x-axis
Answer:
(D) 5 N along – x-axis

Question 2.
For two vectors to be equal, they should have the
(A) same magnitude
(B) same direction
(C) same magnitude and direction
(D) same magnitude but opposite direction
Answer:
(C) same magnitude and direction

Question 3.
The magnitude of scalar product of two unit vectors perpendicular to each other is
(A) zero
(B) 1
(C) -1
(D) 2
Answer:
(A) zero

Question 4.
The magnitude of vector product of two unit vectors making an angle of 60° with each other is
(A) 1
(B) 2
(C) \(\frac{3}{2}\)
(D) \(\frac{\sqrt{3}}{2}\)
Answer:
(D) \(\frac{\sqrt{3}}{2}\)

Question 5.
If \(\overrightarrow{\mathrm{A}}\), \(\overrightarrow{\mathrm{B}}\), and \(\overrightarrow{\mathrm{C}}\) are three vectors, then which of the following is not correct?
(A) \(\overrightarrow{\mathrm{A}}\) . (\(\overrightarrow{\mathrm{B}}\) + \(\overrightarrow{\mathrm{C}}\)) = \(\overrightarrow{\mathrm{A}}\) . \(\overrightarrow{\mathrm{B}}\) + \(\overrightarrow{\mathrm{A}}\) . \(\overrightarrow{\mathrm{C}}\)
(B) \(\overrightarrow{\mathrm{A}}\) . \(\overrightarrow{\mathrm{B}}\) = \(\overrightarrow{\mathrm{B}}\) . \(\overrightarrow{\mathrm{A}}\)
(C) \(\overrightarrow{\mathrm{A}}\) × \(\overrightarrow{\mathrm{B}}\) = \(\overrightarrow{\mathrm{B}}\) × \(\overrightarrow{\mathrm{A}}\)
(D) \(\overrightarrow{\mathrm{A}}\) × (\(\overrightarrow{\mathrm{B}}\) × \(\overrightarrow{\mathrm{C}}\)) = \(\overrightarrow{\mathrm{A}}\) × \(\overrightarrow{\mathrm{B}}\) + \(\overrightarrow{\mathrm{B}}\) × \(\overrightarrow{\mathrm{C}}\)
Answer:
(C) \(\overrightarrow{\mathrm{A}}\) × \(\overrightarrow{\mathrm{B}}\) = \(\overrightarrow{\mathrm{B}}\) × \(\overrightarrow{\mathrm{A}}\)

2. Answer the following questions.

Question 1.
Show that \(\overrightarrow{\mathrm{A}}\) = \(\frac{\hat{i}-\hat{j}}{\sqrt{2}}\) is a unit vector.
Solution:

Question 2.
If \(\overrightarrow{\mathbf{v}_{1}}\) = 3\(\hat{i}\) + 4\(\hat{j}\) + \(\hat{k}\) and \(\overrightarrow{\mathbf{v}_{2}}\) = \(\hat{i}\) – \(\hat{j}\) – \(\hat{k}\), determine the magnitude of \(\overrightarrow{\mathbf{v}_{1}}\) + \(\overrightarrow{\mathbf{v}_{2}}\).
Solution:
\(\overrightarrow{\mathbf{v}_{1}}\) + \(\overrightarrow{\mathbf{v}_{2}}\) = (3\(\hat{i}\) + 4\(\hat{j}\) + \(\hat{k}\)) + (\(\hat{i}\) – \(\hat{j}\) – \(\hat{k}\))
= 3\(\hat{i}\) + 3\(\hat{i}\) + 4\(\hat{j}\) – \(\hat{j}\) + \(\hat{k}\) – \(\hat{k}\)
= 4\(\hat{i}\) + 3\(\hat{j}\)
∴ Magnitude of (\(\overrightarrow{\mathbf{v}_{1}}\) + \(\overrightarrow{\mathbf{v}_{2}}\)),
|\(\overrightarrow{\mathbf{v}_{1}}\) + \(\overrightarrow{\mathbf{v}_{2}}\)| = \(\sqrt{4^{2}+3^{2}}\) = \(\sqrt{25}\) = 5 units.
Answer:
Magnitude of \(\overrightarrow{\mathbf{v}_{1}}\) + \(\overrightarrow{\mathbf{v}_{2}}\) = 5 units.

Question 3.
For \(\overline{\mathrm{v}_{1}}\) = 2\(\hat{i}\) – 3\(\hat{j}\) and \(\overline{\mathrm{v}_{2}}\) = -6\(\hat{i}\) + 5\(\hat{j}\), determine the magnitude and direction of \(\overline{\mathrm{v}_{1}}\) + \(\overline{\mathrm{v}_{2}}\).
Answer:
\(\overline{\mathrm{v}_{1}}\) + \(\overline{\mathrm{v}_{2}}\) = (2\(\hat{i}\) – 3\(\hat{j}\)) + (-6\(\hat{i}\) + 5\(\hat{j}\))
= (2\(\hat{i}\) – 6\(\hat{i}\)) + (-3\(\hat{j}\) + 5\(\hat{j}\))
= -4\(\hat{i}\) + 2\(\hat{j}\)
∴ |\(\overline{\mathrm{v}_{1}}\) + \(\overline{\mathrm{v}_{2}}\)| = \(\sqrt{(-4)^{2}+2^{2}}\) = \(\sqrt{20}\) = \(\sqrt{4 \times 5}\) = 2\(\sqrt{5}\)
Comparing \(\overline{\mathrm{v}_{1}}\) + \(\overline{\mathrm{v}_{2}}\), with \(\overrightarrow{\mathrm{R}}\) = R_x\(\hat{i}\) + R_y\(\hat{j}\)
⇒ R_x = -4 and R_y = 2
Taking θ to be angle made by \(\overrightarrow{\mathrm{R}}\) with X-axis,

Answer:
Magnitude and direction of \(\overline{\mathrm{v}_{1}}\) + \(\overline{\mathrm{v}_{2}}\), is
respectively 2\(\sqrt{5}\) and and tan^-1\(\left(-\frac{1}{2}\right)\) with X – axis.

Question 4.
Find a vector which is parallel to \(\overrightarrow{\mathrm{v}}\) = \(\hat{i}\) – 2\(\hat{j}\) and has a magnitude 10.
Answer:

Substituting for w_x in (i) using equation (ii),

Using equation (ii),

Answer:
Required vector is \(\frac{10}{\sqrt{5}} \hat{\mathbf{i}}\) – \(\frac{20}{\sqrt{5}} \hat{\mathbf{j}}\)

Alternate method:

When two vectors are parallel, one vector is scalar multiple of another,
i.e., if \(\overrightarrow{\mathrm{v}}\) and \(\overrightarrow{\mathrm{w}}\) are parallel then, \(\overrightarrow{\mathrm{w}}\) = n\(\overrightarrow{\mathrm{v}}\) where, n is scalar.

Question 5.
Show that vectors \(\vec{a}\) = 2\(\hat{\mathbf{i}}\) + 5\(\hat{\mathbf{j}}\) – 6\(\hat{\mathbf{k}}\) and \(\vec{b}\) = \(\hat{\mathbf{i}}\) + \(\frac{5}{2}\)\(\hat{\mathbf{j}}\) – 3\(\hat{\mathbf{k}}\) are parallel.
Answer:
Let angle between two vectors be θ.

⇒ Two vectors are parallel.

Alternate method:

\(\vec{a}\) = 2(\(\hat{\mathbf{i}}\) + \(\frac{5}{2}\)\(\hat{\mathbf{j}}\) + \(\hat{\mathbf{k}}\)) = 2\(\vec{b}\)
Since \(\vec{a}\) is a scalar multiple of \(\vec{b}\), the vectors are parallel.

3. Solve the following problems.

Question 1.
Determine \(\vec{a}\) × \(\vec{b}\), given \(\vec{a}\) = 2\(\hat{\mathbf{i}}\) + 3\(\hat{\mathbf{j}}\) and \(\vec{b}\) = 3\(\hat{\mathbf{i}}\) + 5\(\hat{\mathbf{j}}\).
Answer:
Using determinant for vectors in two dimensions,

Answer:
\(\vec{a}\) × \(\vec{b}\) gives \(\hat{\mathbf{k}}\)

Question 2.
Show that vectors \(\overrightarrow{\mathbf{a}}\) = 2\(\hat{\mathbf{i}}\) + 3\(\hat{\mathbf{j}}\) + 6\(\hat{\mathbf{k}}\), \(\overrightarrow{\mathbf{b}}\) = 3\(\hat{\mathbf{i}}\) – 6\(\hat{\mathbf{j}}\) + 2\(\hat{\mathbf{k}}\) and \(\overrightarrow{\mathbf{c}}\) = 6\(\hat{\mathbf{i}}\) + 2\(\hat{\mathbf{j}}\) – 3\(\hat{\mathbf{k}}\) are mutually perpendicular.
Solution:
As dot product of two perpendicular vectors is zero. Taking dot product of \(\vec{a}\) and \(\vec{b}\)


Combining two results, we can say that given three vectors \(\vec{a}\), \(\vec{b}\), and \(\vec{c}\) are mutually perpendicular to each other.

Question 3.
Determine the vector product of \(\overrightarrow{\mathrm{v}_{1}}\) = 2\(\hat{i}\) + 3\(\hat{j}\) – \(\hat{k}\) and \(\overrightarrow{\mathrm{v}_{2}}\) = \(\hat{i}\) + 2\(\hat{j}\) – 3\(\hat{k}\) are perpendicular to each other, determine the value of a.
Solution:

Answer:
Required vector product is -7\(\hat{i}\) + 5\(\hat{j}\) + \(\hat{k}\)

Question 4.
Given \(\bar{v}_{1}\) = 5\(\hat{i}\) + 2\(\hat{j}\) and \(\bar{v}_{2}\) = a\(\hat{i}\) – 6\(\hat{j}\) are perpendicular to each other, determine the value of a.
Solution:
As \(\bar{v}_{1}\) and \(\bar{v}_{2}\) are perpendicular to each other, θ = 90°

Answer:
Value of a is \(\frac{12}{5}\).

Question 5.
Obtain derivatives of the following functions:
(i) x sin x
(ii) x⁴ + cos x
(iii) x/sin x
Answer:
(i) x sin x
Solution:
\(\frac{d}{d x}\)[f₁(x) × f₂(x)] = f₁(x)\(\frac{\mathrm{df}_{2}(\mathrm{x})}{\mathrm{dx}}\) + \(\frac{\mathrm{df}_{1}(\mathrm{x})}{\mathrm{dx}}\)f₂(x)
For f₁(x) = x and f₂(x) = sin x
\(\frac{d}{d x}\)(x sin x) = x\(\frac{\mathrm{d}(\sin \mathrm{x})}{\mathrm{dx}}\) + \(\frac{d(x)}{d x}\) sin x
= x cos x + 1 × sin x
= sin x + x cos x

(ii) x⁴ + cos x
Solution:

(iii) \(\frac{\mathbf{x}}{\sin x}\)
Solution:

[Note: As derivative of (sin x) is cos x, negative sign that occurs in rule for differentiation for quotient of two functions gets retained in final answer]

Question 6.
Using the rule for differentiation for quotient of two functions, prove that \(\frac{d}{d x}\left(\frac{\sin x}{\cos x}\right)\) = sec²x
Solution:

Question 7.
Evaluate the following integral:
(i) \(\int_{0}^{\pi / 2} \sin x d x\)
(ii) \(\int_{1}^{5} x d x\)
Answer:
(i) \(\int_{0}^{\pi / 2} \sin x d x\)
Solution:

(ii) \(\int_{1}^{5} x d x\)
Solution:

11th Physics Digest Chapter 2 Mathematical Methods Intext Questions and Answers

Can you recall? (Textbook Page No. 16)

Question 1.
Define scalars and vectors.
Answer:

1. Physical quantities which can be completely described b their magnitude (a number and unit) are called scalars.
2. Physical quantities which need magnitude, as well as direction for their complete description, are called vectors.

Question 2.
Which of the following are scalars or vectors?
Displacements, distance travelled, velocity, speed, force, work done, energy
Answer:

1. Scalars: Distance travelled, speed, work done, energy.
2. Vectors: Displacement, velocity, force.

Question 3.
What is the difference between a scalar and a vector?
Answer:

No.	Scalars	Vectors
i.	It has magnitude only	It has magnitude as well as direction.
ii.	Scalars can be added or subtracted according to the rules of the algebra.	Vectors are added or subtracted by the geometrical (graphical) method or vector algebra.
iii.	It has no specific representation.	It is represented by the symbol (→) arrow.
iv.	The division of a scalar by another scalar is valid.	The division of a vector by another vector is not valid.
	Example: Length, mass, time, volume, etc.	Example: Displacement, velocity, acceleration, force, etc.

Internet my friend (Textbook page no. 28)

1. 1. hyperphysics.phy-astr.gsu.edu/hbase/vect. html#veccon
   2. hyperphysics.phy-astr.gsu.edu/hbase/ hframe.html

Answer:
[Students can use links given above as a reference and collect information about mathematical methods]

Balbharti Maharashtra State Board 11th Physics Textbook Solutions Chapter 1 Units and Measurements Textbook Exercise Questions and Answers.

Maharashtra State Board 11th Physics Solutions Chapter 1 Units and Measurements

1. Choose the correct option.

Question 1.
[L¹M¹T^-2] is the dimensional formula for
(A) Velocity
(B) Acceleration
(C) Force
(D) Work
Answer:
(C) Force

Question 2.
The error in the measurement of the sides of a rectangle is 1%. The error in the measurement of its area is
(A) 1%
(B) \(\frac{1}{2}\)%
(C) 2%
(D) None of the above.
Answer:
(C) 2%

Question 3.
Light year is a unit of
(A) Time
(B) Mass
(C) Distance
(D) Luminosity
Answer:
(C) Distance

Question 4.
Dimensions of kinetic energy are the same as that of
(A) Force
(B) Acceleration
(C) Work
(D) Pressure
Answer:
(C) Work

Question 5.
Which of the following is not a fundamental unit?
(A) cm
(B) kg
(C) centigrade
(D) volt
Answer:
(D) volt

2. Answer the following questions.

Question 1.
Star A is farther than star B. Which star will have a large parallax angle?
Answer:

i). ‘b’ is constant for the two stars
∴ θ ∝ \(\frac{1}{D}\)

ii) As star A is farther i.e., D_A > D_B
⇒ θ_A < θ_B
Hence, star B will have larger parallax angle than star A.

Question 2.
What are the dimensions of the quantity l \(\sqrt{l / g}\), l being the length and g the acceleration due to gravity?
Answer:

[Note: When power of symbol expressing fundamental quantity appearing in the dimensional formula is not given, ills taken as 1.]

Question 3.
Define absolute error, mean absolute error, relative error and percentage error.
Answer:
Absolute error:
a. For a given set of measurements of a quantity, the magnitude of the difference between mean value (Most probable value) and each individual value is called absolute error (∆a) in the measurement of that quantity.
b. absolute error = |mean value – measured value|
∆a₁ = |a_mean – a₁|
Similarly, ∆a₂ = |a_mean – a₂|
. . . ..
. . . .
. . . .
∆a_n = |a_mean – a_n|

Mean absolute error:
For a given set of measurements of a same quantity the arithmetic mean of all the absolute errors is called mean absolute error in the measurement of that physical quantity.
∆a_mean = \(\frac{\Delta \mathrm{a}_{1}+\Delta \mathrm{a}_{2}+\ldots \ldots .+\Delta \mathrm{a}_{n}}{\mathrm{n}}=\frac{1}{\mathrm{n}} \sum_{\mathrm{i}=1}^{n} \Delta \mathrm{a}_{\mathrm{i}}\)

Relative error:
The ratio of the mean absolute error in the measurement of a physical quantity to its arithmetic mean value is called relative error.
Relative error = \(\frac{\Delta \mathrm{a}_{\mathrm{mean}}}{\mathrm{a}_{\mathrm{mean}}}\)

Percentage error:
The relative error represented by percentage (i.e., multiplied by 100) is called the percentage error.
Percentage error = \(\frac{\Delta \mathrm{a}_{\mathrm{mean}}}{\mathrm{a}_{\mathrm{mean}}}\) × 100%
[Note: Considering conceptual conventions question is modified to define percentage error and not mean percentage error.]

Question 4.
Describe what is meant by significant figures and order of magnitude.
Answer:
Significant figures:

1. Significant figures in the measured value of a physical quantity is the sum of reliable digits and the first uncertain digit.
   OR
   The number of digits in a measurement about which we are certain, plus one additional digit, the first one about which we are not certain is known as significant figures or significant digits.
2. Larger the number of significant figures obtained in a measurement, greater is the accuracy of the measurement. The reverse is also true.
3. If one uses the instrument of smaller least count, the number of significant digits increases.

Rules for determining significant figures:

1. All the non-zero digits are significant, for example if the volume of an object is 178.43 cm³, there are five significant digits which are 1,7,8,4 and 3.
2. All the zeros between two nonzero digits are significant, eg., m = 165.02 g has 5 significant digits.
3. If the number is less than 1, the zero/zeroes on the right of the decimal point and to the left of the first nonzero digit are not significant e.g. in 0.001405, significant. Thus the above number has four significant digits.
4. The zeroes on the right hand side of the last nonzero number are significant (but for this, the number must be written with a decimal point), e.g. 1.500 or 0.01500 both have 4 significant figures each.
   On the contrary, if a measurement yields length L given as L = 125 m = 12500 cm = 125000 mm, it has only three significant digits.

Order of magnitude:
The magnitude of any physical quantity can be expressed as A × 10ⁿ where ‘A’ is a number such that 0.5 ≤ A < 5 then, ‘n’ is an integer called the order of magnitude.
Examples:

1. Speed of light in air = 3 × 10⁸ m/s
   ∴ order of magnitude = 8
2. Mass of an electron = 9.1 × 10^-31 kg
   = 0.91 × 10³⁰ kg
   ∴ order of magnitude = -30

Question 5.
If the measured values of two quantities are A ± ∆A and B ± ∆B, ∆A and ∆B being the mean absolute errors. What is the maximum possible error in A ± B? Show that if Z = \(\frac{A}{B}\)
\(\frac{\Delta Z}{Z}=\frac{\Delta A}{A}+\frac{\Delta B}{B}\)
Answer:
Maximum possible error in (A ± B) is (∆A + ∆B).
Errors in divisions:
i) A
Suppose, Z = \(\frac{A}{B}\) and measured values of A and B are (A ± ∆A) and (B ± ∆B) then,


∴ Maximum relative error of \(\frac{\Delta Z}{Z}=\pm\left(\frac{\Delta \mathrm{A}}{\mathrm{A}}+\frac{\Delta \mathrm{B}}{\mathrm{B}}\right)\)

ii) Thus, when two quantities are divided, the maximum relative error in the result is the sum of relative errors in each quantity.

Question 6.
Derive the formula for kinetic energy of a particle having mass m and velocity v using dimensional analysis
Answer:
Kinetic energy of a body depends upon mass (m) and velocity (v) of the body.
Let K.E. ∝ m^x v^y
∴ K.E. = km^x v^y …….. (1)
where,
k = dimensionless constant of proportionality. Taking dimensions on both sides of equation (1),
[L²M¹T^-2] – [L⁰M¹T⁰]^x [L¹M⁰T^-1]^y
= [L⁰M^xT⁰] [L^yM⁰T^-y]
= [L^0+yM^x+0T^0-y]
[L²M¹T²] = [L^yM^xT^-y] …………. (2)
Equating dimensions of L, M, T on both sides of equation (2),
x = 1 and y = 2 ,
Substituting x, y in equation (1), we have
K.E. = kmv²

3. Solve numerical examples.

Question 1.
The masses of two bodies are measured to be 15.7 ± 0.2 kg and 27.3 ± 0.3 kg. What is the total mass of the two and the error in it?
Answer:
Given: A ± ∆A = 15.7 ± 0.2kg and
B ± ∆B = 27.3 ± 0.3 kg.
To find: Total mass (Z), and total error (∆Z)
Formulae: i. Z = A + B

ii) ±∆Z = ±∆A ± ∆B
Calculation: From formula (i),
Z = 15.7 + 27.3 = 43 kg
From formula (ii),
± ∆Z (± 0.2) + (± 0.3)
=±(0.2 + 0.3)
= ± 0.5 kg
Total mass is 43 kg and total error is ± 0.5 kg.

Question 2.
The distance travelled by an object in time (100 ± 1) s is (5.2 ± 0.1) m. What is the speed and it’s relative error?
Answer:
Given: Distance (D ± ∆D) = (5.2 ± 0.1) m,
time(t ± ∆t) = (100 ± 1)s.
To find: Speed (v), maximum relative error \(\left(\frac{\Delta \mathrm{v}}{\mathrm{v}}\right)\)

Formulae: i. v = \(\frac{\mathrm{D}}{\mathrm{t}}\)
ii. \(\frac{\Delta \mathrm{v}}{\mathrm{v}}=\pm\left(\frac{\Delta \mathrm{D}}{\mathrm{D}}+\frac{\Delta \mathrm{t}}{\mathrm{t}}\right)\)

Calculation: From formula (i),
v = \(\frac{5.2}{100}\) = 0.052 m/s
From formula (ii),
\(\frac{\Delta \mathrm{v}}{\mathrm{v}}=\pm\left(\frac{0.1}{5.2}+\frac{1}{100}\right)\)
= \(\pm\left(\frac{1}{52}+\frac{1}{100}\right)=\pm \frac{19}{650}\)
= ± 0.029 rn/s
The speed is 0.052 m/s and its maximum relative error is ± 0.029 m/s.
[Note: Framing of numerical is modified to make it specific and meaningful.]

Question 3.
An electron with charge e enters a uniform. magnetic field \(\vec{B}\) with a velocity \(\vec{v}\). The velocity is perpendicular to the magnetic field. The force on the charge e is given by
|\(\vec{F}\)| = Bev Obtain the dimensions of \(\vec{B}\).
Answer:
Given: |\(\vec{F}\)| = B e v
Considering only magnitude, given equation is simplified to,
F = B e v

∴ B = [L⁰M¹T^-2I^-1]
[Note: The answer given above is calculated in accordance with textual method considering the given data.]

Question 4.
A large ball 2 m in radius is made up of a rope of square cross section with edge length 4 mm. Neglecting the air gaps in the ball, what is the total length of the rope to the nearest order of magnitude?
Answer:
Volume of ball = Volume enclosed by rope.
\(\frac{4}{3}\) π (radius)³ = Area of cross-section of rope × length of rope.
∴ length of rope l = \(\frac{\frac{4}{3} \pi r^{3}}{A}\)
Given:
r = 2 m and
Area = A = 4 × 4 = 16 mm²
= 16 × 10^-6 m²
∴ l = \(\frac{4 \times 3.142 \times 2^{3}}{3 \times 16 \times 10^{-6}}\)
= \(\frac{3.142 \times 2}{3}\) × 10^-6 m
≈ 2 × 10⁶ m.
Total length of rope to the nearest order of magnitude = 10⁶ m = 10³ km

Question 5.
Nuclear radius R has a dependence on the mass number (A) as R = 1.3 × 10^-16 A^{\(\frac{1}{3}\)} m. For a nucleus of mass number A=125, obtain the order of magnitude of R expressed in metre.
Answer:
R= 1.3 × 10^-16 × A^{\(\frac{1}{3}\)} m
For A = 125
R= 1.3 × 10^-16 × (125)^{\(\frac{1}{3}\)}
= 1.3 × 10^-16 × 5
= 6.5 × 10^-16
= 0.65 × 10^-15 m
∴ Order of magnitude = -15
[Note: Taking the standard value of nuclear radius R = 1.3 × 10^-155 m, the order of magnitude comes to be 10^-14 m.]

Question 6.
In a workshop a worker measures the length of a steel plate with a Vernier callipers having a least count 0.01 cm. Four such measurements of the length yielded the following values: 3.11 cm, 3.13 cm, 3.14 cm, 3.14 cm. Find the mean length, the mean absolute error and the percentage error in the measured value of the length.
Answer:
Given: a₁ = 3.11 cm, a₂ = 3.13 cm,
a₃ = 3.14 cm. a₄ = 3.14cm
Least count L.C. = 0.01 cm.
To find.
i. Mean length (a_mean)
ii. Mean absolute error (∆a_mean)
iii. Percentage error.

Formulae: i. a_mean = \(\frac{a_{1}+a_{2}+a_{3}+a_{4}}{4}\)
ii. ∆a_n = |a_mean – a_n|
iii. ∆a_mean = \(\frac{\Delta \mathrm{a}_{1}+\Delta \mathrm{a}_{2}+\Delta \mathrm{a}_{3}+\Delta \mathrm{a}_{4}}{4}\)
iv. Percentage error = \(\frac{\Delta \mathrm{a}_{\mathrm{mean}}}{\mathrm{a}_{\text {mean }}}\) × 100

Calculation: From formula (i),
a_mean = \(\frac{3.11+3.13+3.14+3.14}{4}\)
= 3.13 cm
From formula (ii),
∆a₁ = |3.13 – 3.11| = 0.02 cm
∆a₂ = |3.13 – 3.13| = 0
∆a₃ = |3.13 – 3.14| = 0.01 cm
∆a₄ = |3.13 – 3.14| = 0.01 cm
From formula (iii),
∆a_mean = \(\frac{0.02+0+0.01+0.01}{4}\) = 0.01 cm
From formula (iii).
% error = \(\frac{0.01}{3.13}\) × 100
= \(\frac{1}{3.13}\)
= 0.3196
……..(using reciprocal table)
= 0.32%

i. Mean length is 3.13 cm.
ii. Mean absolute error is 0.01 cm.
iii. Percentage error is 0.32 %.
[Note: As per given data of numerical, percentage error calculation upon rounding off yields percentage error as 0.32%]

Question 7.
Find the percentage error in kinetic energy of a body having mass 60.0 ± 0.3 g moving with a velocity 25.0 ± 0.1 cm/s.
Answer:
Given: m = 60.0 g, v = 25.0 cm/s.
∆m = 0.3 g, ∆v = 0.1 cm/s
To find: Percentage error in E
Formula: Percentage error in E
\(\left(\frac{\Delta \mathrm{m}}{\mathrm{m}}+2 \frac{\Delta \mathrm{v}}{\mathrm{v}}\right)\) × 100%

Calculation: From formula,
Percentage error in E
= \(\left(\frac{0.3}{60.0}+2 \times \frac{0.1}{25.0}\right)\) × 100%
= 1.3%
The percentage error in energy is 1.3%.

Question 8.
In Ohm’s experiments, the values of the unknown resistances were found to be 6.12 Ω , 6.09 Ω, 6.22 Ω, 6.15 Ω. Calculate the mean absolute error, relative error and percentage error in these measurements.
Answer:
Given: a₁ = 6.12 Ω, a₂ = 6.09 Ω, a₃ = 6.22 Ω, a₄ = 6.15 Ω,

To find:

i) Absolute error (∆a_mean)
ii) Relative error
iii) Percentage error

Formulae:

i) a_mean = \(\frac{a_{1}+a_{2}+a_{3}+a_{4}}{4}\)
ii) ∆a_n = |a_mean – a_n|
iii) ∆a_mean = \(\frac{\Delta \mathrm{a}_{1}+\Delta \mathrm{a}_{2}+\Delta \mathrm{a}_{3}+\Delta \mathrm{a}_{4}}{4}\)
iv) Percentage error = \(\frac{\Delta \mathrm{a}_{\mathrm{mean}}}{\mathrm{a}_{\text {mean }}}\) × 100

From formula (ii),
∆a₁ = |6.145 – 6.12|= 0.025
∆a₂ = |6.145 – 6.09| = 0.055
∆a₃ = |6.145 – 6.22| = 0.075
∆a₄ = |6.l45 – 6.15| = 0.005
From formula (iii),
∆a_mean = \(\frac{0.025+0.055+0.075+0.005}{4}=\frac{0.160}{4}\)
= 0.04 Ω
From formula (iv),
Relative error = \(\frac{0.04}{6.145}\) = 0.0065 Ω
From formula (v).
Percentage error = 0.0065 \frac{0.04}{6.145} 100 = 0.65%

i. The mean absolute error is 0.04 Ω.
ii. The relative error is 0.0065 Ω.
iii. The percentage error is 0.65%.
[Note: Framing of numerical is modified to reach the answer given to the numerical.]

Question 9.
An object is falling freely under the gravitational force. Its velocity after travelling a distance h is v. If v depends upon gravitational acceleration g and distance, prove with dimensional analysis that v = k\(\sqrt{g h}\) where k is a constant.
Answer:
Given = v = k\(\sqrt{g h}\)

k being constant is assumed to be dimensionless.
Dimensions of L.H.S. = [v] = [L¹T^-1]
Dimension of R.H.S. = [latex]\sqrt{g h}[/latex]
= [L¹T^-2]^{\(\frac{1}{2}\)} × [L¹]^{\(\frac{1}{2}\)}
= [L²T^-2]^{\(\frac{1}{2}\)}
= [L¹T^-1]
As, [L.H.S.] = [R.H.S.],
=> v = k\(\sqrt{g h}\)is dimensionally correct equation.

Question 10.
v = at + \(\frac{b}{t+c}\) + v₀ is a dimensionally valid equation. Obtain the dimensional formula for a, b and c where v is velocity, t is time and v₀ is initial velocity.
Answer:
Solution: Given: y = at + \(\frac{b}{t+c}\) + + v₀

As only dimensionally identical quantities can be added together or subtracted from each other, each term on R.H.S. has dimensions of L.H.S. i.e., dimensions of velocity.

∴ [LH.S.] = [v] = [L¹T^-1]
This means, [at] = [v] = [L¹T^-1]
Given, t = time has dimension [T^-1]
∴ [a] = \(\frac{\left[\mathrm{L}^{1} \mathrm{~T}^{-1}\right]}{[\mathrm{t}]}=\frac{\left[\mathrm{L}^{1} \mathrm{~T}^{-1}\right]}{\left[\mathrm{T}^{1}\right]}\) = [L¹T^-2] = L¹M⁰T^-2]
Similarly, [c] = [t] = [T¹] = [L⁰M⁰T¹]
∴ \(\frac{[\mathrm{b}]}{\left[\mathrm{T}^{1}\right]}\) = [v] = [L¹T^-1]
∴ [b] = [L¹T^-1] × [T¹] = [L¹] = [L¹M⁰T⁰]

Question 11.
The length, breadth and thickness of a rectangular sheet of metal are 4.234 m, 1.005 m, and 2.01 cm respectively. Give the area and volume of the sheet to correct significant figures.
Answer:
Given: l = 4.234 m, b = 1.005 m,
t = 2.01 cm = 2.01 × 10^-2 m = 0.0201 m

To find:
i) Area of sheet to correct significant figures (A)
ii) Volume of sheet to correct significant figures (V)
Formulae: i. A = 2(lb + bt + tl)
iii) V = l × b × t

Calculation: From formula (i),
A = 2(4.234 × 1.005 + 1.005 × 0.0201 +0.0201 × 4.234)
= 2 |[antilog(log 4.234 + logl .005) + antiiog(log 1.005 + log0.0201) + antilog(log 0.0201 + log 4.234)]}
= 2{[antilog(0.6267 + 0.0021) + antilog(0.0021 + \(\overline{2}\) .3010) + antilog (\(\overline{2}\) .3010 + 0.6267)]}
= 2 {[antilog(0.6288) + antilog (\(\overline{2}[/latex .3031) +antilog([latex]\overline{2}\) .9277)]}
= 2 [4.254 + 0.02009 + 0.08467]
= 2 [4.35876]
= 8.71752m²

In correct significant figure,
A = 8.72 m2 From formula (ii),
V =4.234 × 1.005 × 0.0201
= antilog [log (4.234) + log (1.005) + log (0.0201)]
= antilog [0.6269 – 0.0021 – \(\overline{2}\).3032]
= antilog [0.6288 – \(\overline{2}\).3032]
= antilog [ 2 .9320]
= 8.551 × 10^-2
= 0.08551 m³
In correct significant figure (rounding off),
V = 0.086 m³

i.) Area of sheet to correct significant figures is 8.72 m².
ii) Volume of sheet to correct significant figures is 0.086 m³.
[Note: The given solution is arrived to by considering a rectangular sheet.]

Question 12.
If the length of a cylinder is l = (4.00 ± 0.001) cm, radius r = (0.0250 ± 0.001) cm and mass m = (6.25 ± 0.01) gm. Calculate the percentage error in the determination of density.
Answer:
Given: l = (4.00 ± 0.001) cm,
In order to have same precision, we use, (4.000 ± 0.001),
r = (0.0250 ± 0.00 1) cm,
In order to have same precision, we use, (0.025 ± 0.001)
m = (6.25 ± 0.01) g
To find: percentage error in density

Formulae:
i) Relative error in volume, \(\frac{\Delta \mathrm{V}}{\mathrm{V}}=\frac{2 \Delta \mathrm{r}}{\mathrm{r}}+\frac{\Delta l}{l}\)
….(∵ Volume of cylinder, V = πr²l)

ii) Releative error \(\frac{\Delta \rho}{\rho}=\frac{\Delta m}{m}+\frac{\Delta V}{V}\)

iii) Percentage error= Relative error × 100%

Calculation.
From formulae (i) and (ii),
∴ \(\frac{\Delta \rho}{\rho}=\frac{\Delta \mathrm{m}}{\mathrm{m}}+\frac{2 \Delta \mathrm{r}}{\mathrm{r}}+\frac{\Delta l}{l}\)
= \(\frac{0.01}{6.25}+\frac{2(0.001)}{0.025}+\frac{0.001}{4.000}\)
= 0.00 16 + 0.08 + 0.00025
= 0.08 185
From formula (iii).
% error in density = \(\frac{\Delta \rho}{\rho}\) × 100
= 0.08185 × 100
= 8.185%
Percentage error in density is 8.185%.

Question 13.
When the planet Jupiter is at a distance of 824.7 million kilometers from the Earth, its angular diameter is measured to be 35.72″ of arc. Calculate the diameter of the Jupiter.
Answer:
Given: Angular diameter (a) = 35.72″
= 35.72″ × 4.847 × 10^-6 rad
1.73 × 10^-4 rad

Distance from Earth (D)
= 824.7 million km
= 824.7 × 10⁶ km
= 824.7 × 10⁹ m.

To find: Diameter of Jupiter (d)
Formula: d = α D
Calculation: From formula,
d = 1.73 × 10^-4 × 824.7 × 10⁹
= 1.428 × 10⁸ m
= 1.428 × 10⁵ km
Diameter of Jupiter is 1.428 × 10⁵ km.

Question 14.
If the formula for a physical quantity is X = \(\frac{a^{4} b^{3}}{c^{1 / 3} d^{1 / 2}}\) and if the percentage error in the measurements of a, b, c and d are 2%, 3%, 3% and 4% respectively. Calculate percentage error in X.
Answer:
Given X = \(\frac{a^{4} b^{3}}{c^{1 / 3} d^{1 / 2}}\)
Percentage error in a, b, c, d is respectively 2%, 3%, 3% and 4%.
Now, Percentage error in X

Question 15.
Write down the number of significant figures in the following: 0.003 m², 0.1250 gm cm^-2, 6.4 × 10⁶ m, 1.6 × 10^-19 C, 9.1 × 10^-31 kg.
Answer:

Question 16.
The diameter of a sphere is 2.14 cm. Calculate the volume of the sphere to the correct number of significant figures.
Answer:
Volume of sphere = \(\frac{4}{3}\) πr³
= \(\frac{4}{3}\) × 3.142 × (\(\frac{2.14}{2}\))³ ………….. (∵ r = \(\frac{d}{2}\))
= \(\frac{4}{3}\) × 3.142 × (1.07)³
= 1.333 × 3.142 × (1.07)³
= {antilog [log (1.333) + log(3.142)+3 log(1.07)]}
= {antilog [0.1249 + 0.4972 + 3 (0.0294)])
= {antilog [0.6221 + 0.0882]}
= {antilog [0.7103]}
= 5.133cm³
In multiplication or division, the final result should retain as many significant figures as there are in the original number with the least significant figures.
Volume in correct significant figures
∴ 5.13 cm³

11th Physics Digest Chapter 1 Units and Measurements Intext Questions and Answers

Can you recall (Textbook Page No. 1)

Question 1.
i) What is a unit?
ii) Which units have you used in the laboratory for measuring
a. length
b. mass
c. time
d. temperature?
iii. Which system of units have you used?
Answer:

1. The standard measure of any quantity is called the unit of that quantity.
2. 
3. MKS or SI system is used mostly. At times. even CGS system is used.

Can you tell? (Textbook Page No. 8)

Question 1.
If ten students are asked to measure the length of a piece of cloth upto a mm, using a metre scale, do you think their answers will be identical? Give reasons.
Answer:
Answers of the students are likely to be different. Length of cloth needs to be measured up to a millimetre (mm) length. Hence, to obtain accurate and precise reading one must use measuring instrument having least count smaller than 1 mm.

But least count of metre scale is 1 mm. As a result, even smallest uncertainty in reading would vary reading significantly. Also, skill of students doing measurement may also introduce uncertainty in observation.
Hence, their answers are likely to be different.

Activity (Textbook Page No. 10)

Perform an experiment using a Vernier callipers of least count 0.01cm to measure the external diameter of a hollow cylinder. Take 3 readings at different positions on the cylinder and find (i) the mean diameter (ii) the absolute mean error and (iii) the percentage error in the measurement of diameter.
Answer:
Given: L.C. = 0.01 cm
To measure external diameter of hollow cylinder readings are taken as follows:

[Note: The above table is made assuming zero error in Vernier calipers. If caliper has positive or negative zero error, the zero error correction needs to be introduced into observed reading.]

Internet my friend (Textbook Page No. 12)

i. ideoiectures.net/mit801f99_lewin_lec0l/
ii. hyperphysicsphy-astr.gsu.ed u/libase/hfra me. html

[Students can use links given above as a reference and collect information about units and measurements]

Balbharti Maharashtra State Board Hindi Yuvakbharati 11th Digest रचना पत्र लेखन Notes, Questions and Answers.

Maharashtra State Board 11th Hindi रचना पत्र लेखन

पत्रलेखन एक कला है आजकल इसका साहित्यिक महत्त्व भी स्वीकारा जाने लगा है। एक अच्छे पत्र की पाँच विशेषताएँ होती हैं।

1. सरल भाषा शैली।
2. विचारों की सुस्पष्टता।
3. संक्षेप एवं संपूर्णता।
4. प्रभावान्विति।
5. बाहरी सजावट।

पत्र लिखते समय निम्नलिखित वातों को ध्यान में रखना चाहिए –

1. जहाँ तक संभव हो, पत्र में स्वाभाविकता का निर्वाह होना चाहिए। पत्र में कहीं बनावटीपन नहीं होना चाहिए।
2. साधारण संबंधियों या अधिकारी या अपरिचित व्यक्तियों को लिखे पत्रों में कहीं भी अनावश्यक विस्तार या भावुकता नहीं होनी चाहिए।
3. सरकारी और कामकाजी पत्रों में कहीं अनावश्यक विस्तार या भावुकता नहीं होनी चाहिए।
4. निकट संबंधियों के लिखे पत्रों में पूर्ण आत्मीयता और स्वाभाविकता होनी चाहिए।
5. पत्र को उपयुक्त परिच्छेदों में विभाजित करके लिखना चाहिए।
6. पत्र की भाषा शुद्ध, सरल व प्रवाहपूर्ण होनी चाहिए। वर्तनी (Spelling) एवं विराम चिह्नों का समुचित प्रयोग होना चाहिए।
7. पत्र संक्षिप्त, सुव्यवस्थित, सुस्पष्ट एवं हेतुपूर्ण होना चाहिए। अनावश्यक बातों के लिए पत्र में कोई जगह नहीं होती।

पत्र के प्रकार:

1. व्यक्तिगत या पारिवारिक पत्र
2. सामाजिक पत्र
3. व्यावसायिक अथवा व्यापारिक पत्र
4. कार्यालयीन पत्र

मुख्य रूप से औपचारिक और अनौपचारिक दो तरह के पत्र माने गए है।

औपचारिक पत्र : इस पत्र में संदेश, कथ्य, अपरिचित व्यक्ति एवं अधिकारी को लिखा जाता है इसमें प्राय: कार्यालयीन पत्र, सरकारी पत्र, व्यावसायिक व व्यासपीठ पत्र तथा शिकायती पत्र आते हैं। अनौपचारिक पत्र : इसमें व्यक्तिगत; सगे संबंधियों के पत्र, घरेलू या पारिवारिक पत्र आते हैं। अनौपचारिक पत्रों में पत्र लेखक और जिसे पत्र लिखा जाता है, उसके संबंध के अनुसार अभिवादन या अभिनिवेदन में भिन्नता होती। है निम्नलिखित तालिका में सारी बातें स्पष्ट की गई हैं।

विशेष : जो संबंध छोटे-बड़े नहीं हैं या जिन संबंधो में व्यक्तिगत पत्रों जैसी नितांत आत्मीयता नहीं है बल्कि मात्र व्यावहारिकता है वहाँ ‘प्रणाम’ या ‘शुभाशीष’ जैसे किसी अभिवादन की आवश्यकता नहीं होती हैं।

पत्र का प्रारूप

अनौपचारिक पत्र

दिनांक : ……………………………….. संबोधन : ……………………………….. अभिवादन : ……………………………….. प्रारंभ : ………………………………..विषय विवेचन : …………………………………………………………………………………………………………………………………….. …………………………………………………………………………………………………………………………………………………………….. ……………………………………………………………………………………………………………………………………………………………..तुम्हारा / तुम्हारी : ……………………………….. नाम : ……………………………….. पता : ……………………………….. ई-मेल आईडी : ………………………………..

औपचारिक पत्र

दिनांक : ……………………………….. प्रति, ……………………………….. ………………………………..विषय : ……………………………….. संदर्भ : ……………………………….. महोदय : ……………………………….. विषय विवेचन : …………………………………………………………………………………………………………………………………….. …………………………………………………………………………………………………………………………………………………………….. ……………………………………………………………………………………………………………………………………………………………..भवदीय/भवदीया, हस्ताक्षर : ……………………………….. नाम : ……………………………….. पता : ……………………………….. ई-मेल आईडी : ………………………………..

पत्र के नमूने

1. व्यक्ति गत / अनौपचारिक पत्र

दिनांक : 7 सितंबर, 2019. आदरणीय पिताजी, सादर प्रणाम।आपको यह जानकर खुशी होगी कि मैं यहाँ आनंद से हूँ। मैं अपने महाविद्यालय में कई सहपाठियों को मित्र बना चुका हूँ, जो अच्छे . स्वभाव के, परिश्रमी और अध्ययनशील है। मैं यहाँ अभी नया हूँ फिर भी सब का स्नेह प्राप्त है। यहाँ के प्राचार्य और प्राध्यापक सभी अच्छे हैं। उनका हम पर पूरा ध्यान रहता है। मैं विज्ञान परिषद का मंत्री चुना गया हूँ।यहाँ जीवन अत्यंत व्यस्त है। हर क्षण कीमती है। सब में एक तरह की प्रतियोगिता है। सभी एक-दूसरे से आगे निकलना चाहते हैं। मैं आप को विश्वास दिलाता हूँ कि जीतोड़ परिश्रम करके मैं परीक्षा में अच्छे अंक लाऊँगा। शेष कुशल है। पूजनीय माता जी को प्रणाम व प्रिया को आशीर्वाद।आपका स्नेहाकांक्षी, शरद नाम : शरद देशमुख पता : बी- 212, साई कृपा, महात्मा गांधी रोड, विलेपार्ले (पूर्व), मुंबई – 400 057 ई-मेल आईडी : sharad2000@gmail.com

2. वधाई पत्र :

दिनांक. 15 जून, 2017 प्रिय सविता सप्रेम नमस्ते।यह जानकर प्रसन्नता हुई कि तुम बारहवीं कक्षा में प्रथम श्रेणी में उत्तीर्ण हुई हो और तुम्हें 86 प्रतिशत अंक मिले हैं तुम्हारी इस सफलता पर मैं तुम्हें हार्दिक बधाई देती हूँ। आशा करती हूँ कि तुम्हें आगे की परीक्षा में भी ऐसी ही सफलता मिलती रहे।वैद्यकीय या अभियांत्रिकी शिक्षा में तुम अपनी रुचि के अनुसार ही प्रवेश लो, तुम्हें अवश्य सफलता मिलेगी। तुम्हारे माता-पिता को प्रणाम।तुम्हारी कुशलता की कामना के साथ। तुम्हारी सहेली, प्रभा नाम : प्रभा शर्मा पता : डी, 107, साकेत, नवघर रोड, ठाणे (पू.) ई-मेल आईडी : psharma.2017@gmail.com

3. निमंत्रण पत्र:

दिनांक : 15 अप्रैल, 2019, प्रिय भाई भावेश, सप्रेम नमस्ते।आपको यह जानकर प्रसन्नता होगी कि अगामी 5 मई, 2019, रविवार के दिन मेरे नए घर का गृहप्रवेश है! इस शुभ अवसर पर सपरिवार उपस्थित होकर हमे कृतार्थ करें।आशा है कि आप हमें अनुग्रहित करेंगे।आपका शुभाकांक्षी, अनिल कुमार। नाम : अनिल कुमार पाठक पता : 70/क, कलासागर, खार (प.), मंबई-400 052. ई-मेल आईडी : anilpathale@yahoo.com

4. भावी योजना हेतु मित्र को पत्र

दिनांक : 20 मार्च, 2019. प्रिय मित्र अशोक, नमस्ते।तुम्हारा पत्र मिला। समाचार पाकर प्रसन्नता हुई। पिछले हप्ते ही मेरी परीक्षा समाप्त हुई है। अगले हप्ते सी इ टी की परीक्षा भी है, जिसकी तैयारी कर रहा हूँ। मेरे प्रश्न पत्र अच्छे गए हैं। बारहवीं में 85% अंक पाने की उम्मीद है।माता जी और पिता जी चाहते हैं कि मैं अभियंता (इंजीनियर) बनू किंतु मेरी रुचि डॉक्टरी में है। बचपन से ही एक सपना देखा है। डॉक्टरी में अर्थलाभ के साथ मानव-सेवा का सुअवसर भी प्राप्त होगा। यह किसी अन्य व्यवसाय में संभव नहीं है।यदि तुम्हारी सलाह भी मुझे शीघ्र मिले तो बेहतर होगा। चाचा और चाची को मेरा प्रणाम। शेष कुशल है।तुम्हारा मित्र, सतीश नाम :- सतीश ठाकुर, पता : 105, कलाकुंज, शनिवार पेठ, पुणे – 7. ई-मेल आई.डी. : satish.thakur@gmail.com

(5) कार्यालयीन पत्र :

दिनांक : 20 अक्टूबर, 2019 प्रति, श्री.पुलिस इंस्पेक्टर, शहर पुलिस थाना, वर्धा। विषय : पटाखे असमय फोडने पर प्रतिबंध लगाने हेतु अनुरोधन-पत्रमान्यवर महोदय, दीवाली के इस शुभ अवसर पर रंग में भंग डालने की मेरी कोई मनिषा नहीं है। पर्व त्योहार मनाने की स्वतंत्रता में मैं बाधा नहीं डालना| चाहता हूँ। परंतु दीवाली के पटाखों से मुहल्ले में दिन-रात शोर-शराबा चलता है। घर में बुजुर्ग और छोटे बच्चे भी होते हैं। उनपर इसका बुरा प्रभाव पड़ता है।ऊपर से प्रदूषण भी बढ़ता है। मैं सुरेश भोसले आपको विनम्र अनुरोध करता हूँ कि आप इन पटाखों के फोड़ने पर कुछ-कुछ प्रतिबंध लगाएँ। एक निश्चित समय पर ही फोड़ने की इजाजत दें। ज्यादा आवाज करनेवाले पटाखों पर प्रतिबंध डाल दें। इस से ध्वनि प्रदूषण कम होगा और सबकी परेशानी मिटेगी।उम्मीद करता हूँ कि आप हमारी परेशानी को गंभीरता से लेंगे और अपने अधिकारों का उपयोग कर ठोस कदम उठाएँगे। तसदी के लिए माफी चाहता हूँ।भवदीय, सुरेश भोसले। नाम : सुरेश भोसले, पता : 50, सेवा सदन, गोखले नगर, वर्धा। ई-मेल आई.डी : sureshb1978@gmail.com

(6)

दिनांक : 30 मई, 2019. सेवा में, श्रीमान प्रधानाध्यापक, वैद्यनाथ विद्यालय, परली। विषय : पाँचवी कक्षा में प्रवेश दिलाने के लिए प्रार्थना पत्रमान्यवर महोदय, वैद्यनाथ विद्यालय परली में ही नहीं बल्कि महाराष्ट्र के सबसे अच्छे विद्यालयों में से एक है। मैं चाहती हूँ कि मेरा छोटा भाई कमलेश आगे की पढ़ाई आपके विद्यालय में करे। पिछले वर्ष चौथी कक्षा में उसे अस्सी प्रतिशत अंक आए हैं। वह पढ़ाई के साथ-साथ खेल में भी अच्छा है।दौड़ प्रतियोगिता में उसने राज्यस्तर पर कांस्य पदक प्राप्त किया है। नृत्य और अभिनय जैसी कलाओं में भी निपुण है। उसके इन सभी गुणों का आपके विद्यालय में और विकास होगा। उम्मीद करती हूँ कि आप मना नहीं करेंगे।इस पत्र के साथ मैं चौथी के अंक-पत्र की प्रतिलिपि भेज रही हूँ। आपसे नम्र निवेदन है कि आप मेरे भाई को पाँचवी कक्षा में प्रवेश देने की कृपा करें।धन्यवाद। प्रार्थी, शैलजा पाठक। नाम : शैलजा पाठक, पता : 460, आसरा, नेताजी मार्ग, परली। ई-मेल आई.डी.: shaila.pathale@gmail.com

7. व्यावसायिक पत्र :

दिनांक : 16 जून, 2019 सेवा में, मा. व्यवस्थापक, क्वालिटी स्पोर्टस् अप्पा बळवंत चौक, पुणे। विषय : खेल सामग्री की माँग संदर्भ : अखबार में छपा विज्ञापनमान्यवर महोदय, जन-जागरण में प्रकाशित आपके विज्ञापन से ज्ञात हुआ कि आपके यहाँ सभी प्रकार की खेल सामग्री उपलब्ध है। मैं माधव बाग के क्रीड़ा-मंडल का अध्यक्ष होने के नाते आपको यह पत्र लिख रहा हूँ। जल्द ही हमारे यहाँ वार्षिक खेल उत्सव शुरू होगा। इसके लिए मुझे निम्नलिखित खेल-सामग्री की आवश्यकता है। अनु. क्र.  1.  2.  3.  4. सामग्री  फुटबॉल  बास्केटबॉल  हॉकी स्टिक्स  नेट नग  10  10  08  02 नियमानुसार पाँच सौ रुपए का पोस्टल आर्डर आपको भेज रहा हूँ। शेष रकम वी.पी.पी. छुड़ाते समय अदा की जाएगी। उचित कमीशन देने की कृपा करें। खेल सामग्री जल्द से जल्द ऊपर लिखे पते पर भेजने की कोशिश करें। धन्यवाद, भवदीय, अरूण पाटील। पता : माधव बाग, सांगली। ई-मेल आई.डी.: arunp-2898@gmail.com

8. सामाजिक पत्र :

दिनांक : 20 जून, 2019 सेवा में, मा. स्वास्थ्य अधिकारी, नगर परिषद, कोल्हापुर। विषय : मुहल्ले की अस्वच्छता दूर कराने के लिए निवेदनमहोदय, मैं कोल्हापुर की नागरिक हूँ और शिवनेरी, खासबाग मैदान के पास रहती हूँ। मैं मुहल्ले के नागरिकों के प्रतिनिधि के रूप में आपका ध्यान एक महत्त्वपूर्ण समस्या की ओर आकर्षित करना चाहती हूँ।पिछले कई दिनों से मुहल्ले की सफाई ठीक से नहीं हुई है। जगह-जगह गंदगी फैली हुई है। कचरे की पेटियाँ बहुत छोटी हैं और उनकी संख्या भी पर्याप्त नहीं है। उचित मात्रा में कीटनाशक औषधियों का छिड़काव भी नहीं किया जाता। भयंकर बदबू के कारण आने-जाने वालों को भारी परेशानी का सामना करना पड़ता है। मुहल्ले में मच्छरों का प्रकोप भी बढ़ा है। इसके कारण संक्रामक रोगों के फैलने की आशंका उत्पन्न हो गई है।आकस्मिक रूप से हुई भारी वर्षा ने जनता के कष्ट और भी बढ़ा दिए हैं।अत: आप से विनम्र निवेदन है कि तत्काल सफाई का उचित प्रबंध किया जाए। नई कचरा पेटियाँ रखी जाएँ और कीटनाशक दवाएँ छिड़की जाएँ।आशा है, इस दिशा में तत्काल उचित कार्रवाई करेंगे। तसदी के लिए क्षमस्व, भवदीया, संगीता कोटणीस। नाम : सांगीत कोटणीस पता : 46, शिवनेरी, शाहू नगर, खासबाग मैदान, कोल्हापुर। ई-मेल आई.डी.: sangeeta-2010@gmail.com

Balbharti Maharashtra State Board Hindi Yuvakbharati 11th Digest अपठित काव्यांश Notes, Questions and Answers.

Maharashtra State Board 11th Hindi अपठित काव्यांश

1. पद्यांश पढ़कर दी गई सूचनाओं के अनुसार कृतियाँ कीजिए :

सच है, विपत्ति जब आती है,
कायर को ही दहलाती है,
सूरमा नहीं विचलित होते,
क्षण एक नहीं धीरज खोते,

विघ्नों को गले लगाते हैं,
काँटों में राह बनाते हैं।

मुख से न कभी उफ कहते हैं,
संकट का चरण न गहते हैं,
जो आ पड़ता सब सहते हैं,
उद्योग निरत नित रहते हैं,

शूलों का मूल नशाने को,
बढ़ खुद विपत्ति पर छाने को।

है कौन विघ्न ऐसा जग में,
टिक सके आदमी के मग में?
खम ठोंक ठेलता है जब नर,
पर्वत के जाते पाँव उखड़।

प्रश्न 1.
संजाल पूर्ण कीजिए –

उत्तर:

पद्यांश- सच है विपत्ति जब ……………………………………………… पर्वत के जाते पाँव उखड़। (पाठ्यपुस्तक पृष्ठ क्र.111)

प्रश्न 2.
उत्तर लिखिए –
(i) विपत्ति में ऐसा साहस नहीं
(ii) वीर पुरुष के ताल ठोकने का परिणाम –
उत्तरः
(i) विपत्ति में ऐसा साहस नहीं – वीर पुरुष की राह में अवरोध बनकर खड़ रहने का
(ii) वीर पुरुष के ताल ठोकने का परिणाम – पर्वत का घमंड चकनाचूर हो जाता है

प्रश्न 3.
पद्यांश का भावार्थ सरल हिंदी में लिखिए
उत्तर :
प्रस्तुत पद्यांश राष्ट्रकवि ‘दिनकर’जी की कविता ‘सच्चा वीर’ से लिया गया है। इस पद्यांश में कवि ने सच्चे वीर के लक्षण बताए हैं।

विपत्ति केवल डरपोक व्यक्ति को ही भयभीत करती है। कायर विपत्ति से डरकर अपने पाँव मार्ग से पीछे खींच लेता है। लेकिन वीर पुरुष विपत्ति के सामने डटे रहते हैं। संकट में ही वीर पुरुष के धैर्य की परीक्षा होती है। बड़ा से बड़ा संकट आने पर भी वीर पुरुष घबराते नहीं।

वे अपना धैर्य और संयम बनाए रखते हैं। वे आने वाली विघ्न – बाधाओं के सामने चट्टान की तरह अडिग खड़े रहते हैं। वीर पुरुष विकट परिस्थितियों में भी संकटों से संघर्ष करते हैं और उनसे बाहर निकलने का रास्ता ढूँढ़ निकालते हैं। वे संकटों पर विजय हासिल करके ही दम लेते हैं।

वीर पुरुष संकटों से कभी प्रभावित नहीं होते। वे संकटों में न तो कभी ऊफ करते हैं और न ही संकटों के सामने कभी झुकते हैं। मंजिल की राह में आने वाली विघ्न – बाधाओं को जड़ से समाप्त कर उन पर विजय पाने के लिए वे निरंतर प्रयत्न करते रहते हैं।

संसार में ऐसी कोई भी बाधा नहीं है, जो वीर पुरुष की राह में अवरोध बनकर खड़ी होने का साहस कर सके। वीर पुरुष जब ताल ठोंककर साहस से आगे बढ़ते हैं, तो उनके सामने पर्वत भी नहीं ठहर पाते। वे पर्वत के घमंड को चकना चूर कर आगे बढ़ते हैं।

2. पद्यांश पढ़कर दी गई सूचनाओं के अनुसार कृतियाँ कीजिए:

बहुत दिनों के बाद
अब की मैंने जी भर देखी
पकी-सुनहली फसलों की मुसकाने

– बहुत दिनों के बाद

बहुत दिनों के बाद
अब की मैं जी भर सुन पाया
ध्यान कूटती किशोरियों की कोकिल कंठी तान

– बहुत दिनों के बाद

बहुत दिनों के बाद
अब की मैंने जी भर सूंघे
मौलसिरी के ढेर-ढेर से ताज़े-टटके फूल

– बहुत दिनों के बाद

प्रश्न 1.
आकृति पूर्ण कीजिए –

उत्तरः

(ii) पद्यांश में आया फूल का नाम –
उत्तरः
पद्यांश में आया फूल का नाम – मौलसिरी

पद्यांश: बहुत दिनों के बाद ………………………………….. ताज़े-टटके फूल। (पाठ्यपुस्तक पृष्ठ क्र. 115)

प्रश्न 2.
उत्तर लिखिए –
(i) फसलों की विशेषता –
(ii) फूलों की विशेषता –
उत्तर:
(i) फसलों की विशेषता – पकी-सुनहली
(ii) फूलों की विशेषता – ढेर सारे और ताज़े-टटके

प्रश्न 3.
पद्यांश में वर्णित प्राकृतिक सुषमा का वर्णन कीजिए।
उत्तरः
प्रस्तुत पद्यांश कवि नागार्जुन की कविता ‘बहुत दिनों के बाद’ कविता से लिया गया है। कवि बहुत दिनों के बाद अपने गाँव लौटा। गाँव की प्राकृतिक सुषमा देखकर कवि का मन झूम उठा। कवि ने गाँव के खेतों में पकी-सुनहली फसलें देखी। गाँव की किशोरियाँ धान कूट रही थीं।

उनके कंठ से निकले मधुर गीत कोकिल के मधुर तान की तरह प्रतीत हो रहे थे। इन मधुर गीतों को सुनकर वह संतुष्ट हो गए। कवि ने अनुभव किया कि शहरी बनावटी जीवन की अपेक्षा प्राकृतिक सुषमा से युक्त इस ग्रामीण जीवन की सादगी कितनी सुकून देती है। गाँव के मौलसिरी के ताजे-ताजे सुगंधित फूलों के ढेर देखकर वह प्रफुल्लित हुआ।

इस प्रकार गाँव में चारों ओर प्राकृतिक सुषमा बिखरी हुई थी जो कवि को तृप्ति और आनंद प्रदान कर रही थी।

3. पद्यांश पढ़कर दी गई सूचनाओं के अनुसार कृतियाँ कीजिए

तू क्यों बैठ गया है पथ पर?
ध्येय न हो, पर है मग आगे,
बस धरता चल तू पग आगे,
बैठ न चलने वालों के दल में तू आज तमाशा बनकर!

तू क्यों बैठ गया है पथ पर?
मानव का इतिहास रहेगा
कहीं, पुकार-पुकार कहेगा –
निश्चय था गिर मर जाएगा चलता किंतु रहा जीवन भर!

तू क्यों बैठ गया है पथ पर?
जीवित भी तू आज मरा-सा
पर मेरी तो यह अभिलाषा
चितानिकट भी पहुँच सकूँ अपने पैरों-पैरों चलकर!

प्रश्न 1.
संजाल पूर्ण कीजिए –

उत्तरः

पद्यांशः तू क्यों बैठ गया ………………………………… अपने पैरों-पैरों चलकर! (पाठ्पुस्तक पृष्ठ क्र. 118)

प्रश्न 2.
उत्तर लिखिए –
(i) कवि द्वारा मनुष्य को पूछा गया सवाल –
(ii) कवि की अभिलाषा –
उत्तरः
(i) कवि द्वारा मनुष्य को पूछा गया सवाल – ‘तू क्यों बैठ गया है पथ पर’
(ii) कवि की अभिलाषा – ‘चलते-चलते अपनी चितानिकट पहुँचने की’

प्रश्न 3.
पद्यांश का संदेश अपने शब्दों में लिखिए।
उत्तर:
‘तू क्यों बैठ गया है पथ पर’ कविता में कवि ने जीवन-पथ पर चलते – चलते हताश और निराश हो बैठ जाने वालों से कहा है कि जीवन में सतत् क्रियाशील बने रहना आवश्यक है। लक्ष्य से विमुख होकर कायरों की तरह निष्क्रिय बैठे रहना अनुचित है। ऐसे व्यक्ति जीवित रहते मृतक के समान हैं।

समाज में उपहास के पात्र बने ऐसे लोग निरर्थक जीवन जीते हैं। इसके विपरीत उत्साही व्यक्ति दृढ़ संकल्प और मजबूत इरादे के साथ निरंतर आगे बढ़ता है और अपना लक्ष्य प्राप्त करता है। उसे न तो मृत्यु का भय होता है, न ही पथ से गिरने की चिंता।

ऐसे शूरवीर और साहसी का गुणगान इतिहास भी करता है। वे सदा के लिए इतिहास में अमर हो जाते हैं। उनके आदर्श हमेशा जीवित रहते हैं। आने वाली पीढ़ी उनका अनुसरण करती है।

इस तरह प्रस्तुत कविता के द्वारा कवि ने मनुष्य को हताशा और निराशा त्यागकर लक्ष्य के प्रति आस्थावान बने रहने और जीवन पथ की चुनौतियों से संघर्ष करते हुए निरंतर आगे बढ़ते रहने का संदेश दिया है।

4. पद्यांश पढ़कर दी गई सूचनाओं के अनुसार कृतियाँ कीजिए:

हो-हो भैया पानी दो,
पानी दो गड़धानी दो;
जलती है धरती पानी दो
मरती है धरती पानी दो

हो मेरे भैया..!!

अंकुर फूटे रेत में
सोना उपजे खेत में,
बैल पियासा, भूखी है गैया,
नीचे न अंगना में सोन-चिरैया,
फसल-बुवैया की उठे मुडैया,
मिट्टी को चूनर धानी दो

हो मेरे भैया…!!

प्रश्न 1.
संजाल पूर्ण कीजिए –

उत्तर:

प्रश्न 2.
उत्तर लिखिए –
(i) पानी बरसने का धरती पर परिणाम –
(ii) पानी बरसने पर मिट्टी को मिलेगी –
उत्तर:
(i) पानी बरसने का धरती पर परिणाम – तपती धरती को राहत मिलेगी, फसलें उगेंगी।
(ii) पानी बरसने पर मिट्टी को मिलेगी – धानी चूनर

प्रश्न 3.
पद्यांश का भावार्थ सरल हिंदी में लिखिए।
उत्तरः
प्रस्तुत पद्यांश, गोपालदास सक्सेना ‘नीरज’ जी की कविता ‘पानी दो’ से लिया गया है। कवि बादल भैया से पानी की माँग कर रहे है।

नीरज जी बादल भैया से कह रहे हैं, ‘हे बादल भैया, तुम धरती पर जल बरसाओ, धरतीवासियों को गुड़धानी दो। पानी के अभाव में सबकुछ सूना है। तुम जल बरसाकर तपती धरती और सूखती वनस्पतियों को जीवनदान दो। तुम्हारे जल बरसाने से रेत में अंकुर फूटेंगे, खेतों में हरियाली छा जाएगी।

खेतों में फसलें लहलहा उठेगी। हे बादल भैया, पानी के बिना किसान का बैल प्यासा है और गाय भूखी है। किसान का आँगन सूना हो चुका है। अब उसमें सोन-चिरैया फुदकने नहीं आती। हे बादल, जल बरसाओ, जिससे किसान के घर में खुशहाली आए। उसकी टूटी-फूटी मडैया पर छाजन पड़ सके। हे बादल, पानी बरसाकर तुम धरती को हरी-भरी कर दो, मिट्टी को हरी चुनरिया पहना दो।

5. पद्यांश पढ़कर दी गई सूचनाओं के अनुसार कृतियाँ कीजिए:

आज सड़कों पर लिखे हैं सैकड़ों नारे न देख,
घर अँधेरा देख तू, आकाश के तारे न देख!

एक दरिया है यहाँ पर, दूर तक फैला हुआ,
आज अपने बाजुओं को देख, पतवारें न देख।

अब यकीनन ठोस है धरती, हकीक़त की तरह,
यह हकीकत देख, लेकिन खौफ़ के मारे न देख।

प्रश्न 1.
चौखट पूर्ण कीजिए –
कवि ने देखने के लिए कहा है – कवि ने न देखने के लिए कहा है
(1) ………………………………. – (1) ……………………………….
(2) ………………………………. – (2) ……………………………….
(3) ………………………………. – (3) ……………………………….
उत्तरः
कवि ने देखने के लिए कहा है। – कवि ने न देखने के लिए कहा है
(1) घर के अँधेरे को – (1) आकाश के तारे
(2) अपनी बाजुओं को – (2) सड़कों पर लिखे नारे
(3) हकीकत को – (3) पतवार

प्रश्न 2.
उत्तर लिखिए –
(i) आकाश के तारे’ देखने से कवि का तात्पर्य –
(ii) धरती के बारे में कवि की राय –
उत्तरः
(i) ‘आकाश के तारे’ देखने से कवि का तात्पर्य है सच्चाई से दूर भागना।
(ii) धरती के बारे में कवि की राय है कि धरती ठोस है।

प्रश्न 3.
पद्यांश द्वारा मिलने वाली प्रेरणा अपने शब्दों में लिखिए।
उत्तरः
प्रस्तुत पद्यांश कवि दुष्यंत कुमार जी की लिखी गजल ‘दीवारें न देख’ से लिया गया है। कवि मनुष्य के जीवन की हताशा, निराशा को समाप्त कर उसे एक नई दृष्टि देना चाहता है। आम तौर पर मनुष्य का झुकाव चमक-दमक की ओर अधिक होता है। उसमें जीवन के यथार्थ से टकराने की हिम्मत नहीं होती।

अपने घर के अँधेरे को वह नजरअंदाज कर देता है। स्वयं को शक्तिहीन मानकर अपनी जीवन नौका पतवार के भरोसे छोड़ देता है।

कवि कहते हैं इस जीवन रूपी समुंदर में मुसीबतों के तूफान तो आते रहते हैं। मनुष्य को चाहिए कि वह अपनी बाजुओं के बल पर, स्वयं पर भरोसा रखकर जीवन सागर को पार करें। मनुष्य अपनी बाजुओं को देखें और पतवार की बैसाखी के सहारे चलना छोड़ दे।

जीवन का यथार्थ धरती की तरह ठोस है। इस वास्तविकता से जुड़े रहकर ही जीवन – संग्राम लड़ा जा सकता है। जीवन की सच्चाई को जानते हुए खौफ में क्यों जीए मनुष्य? उसे एक योद्धा की तरह यथार्थ से लड़कर विजय प्राप्त करने की प्रेरणा कवि ने दी है।

Balbharti Maharashtra State Board Hindi Yuvakbharati 11th Digest अपठित गद्यांश Notes, Questions and Answers.

Maharashtra State Board 11th Hindi अपठित गद्यांश

प्रश्न 1.
गद्यांश पढ़कर दी गई सूचनाओं के अनुसार कृतियाँ कीजिए:
उत्तरः
साहब सन्नाटे में आ गए। फतहचंद की तरफ डर और क्रोध की दृष्टि से देखकर काँप उठे! फतहचंद के चेहरे पर पक्का इरादा झलक रहा था। साहब समझ गए, यह मनुष्य इस समयं मरने-मारने के लिए तैयार होकर आया है। ताकत में फतहचंद उनके पासंग भी नहीं था।

लेकिन यह निश्चय था कि वह ईंट का जवाब पत्थर से नहीं, बल्कि लोहे से देने को तैयार है। यदि वह फतहचंद को बुरा-भला कहते हैं, तो क्या आश्चर्य है कि वह डंडा लेकर पिल पड़े। हाथापाई करने में यद्यपि उन्हें जीतने में जरा भी संदेह नहीं था; लेकिन बैठे-बिठाये डंडे खाना भी तो कोई बुद्धिमानी नहीं है।

कुत्ते को आप डंडे से मारिए, ठुकराइए, जो चाहे कीजिए, मगर उसी समय तक, जब तक वह गुर्राता नहीं। एक बार गुर्राकर दौड़ पड़े, तो फिर देखें, आपकी हिम्मत कहाँ जाती है? यही हाल उस वक्त साहब बहादुर का था। जब तक यकीन था कि फतहचंद घुड़की, घुरकी, हंटर, ठोकर सब कुछ खामोशी से सह लेगा, तब तक आप शेर थे; अब वह त्योरियाँ बदले, डंडा सँभाले, बिल्ली की तरह घात लगाए खड़ा है।

ज़बान से कोई कड़ा शब्द निकला और उसने डंडा चलाया। वह अधिक-से-अधिक उसे बर्खास्त कर सकते हैं। अगर मारते हैं, तो मार खाने का भी डर।

उसपर फौजदारी में मुकदमा दायर हो जाने का अंदेशा-माना कि वह अपने प्रभाव और ताकत से अंत में फतहचंद को जेल में डलवा देंगे; परंतु परेशानी और बदनामी से किसी तरह न बच सकते थे। एक बुद्धिमान और दूरंदेश आदमी की तरह उन्होंने यह कहा –

‘ओहो, हम समझ गया, आप हमसे नाराज हैं। हमने क्या आपको कुछ कहा है? आप क्यों हमसे नाराज हैं।’ फतहचंद ने तनकर कहा – ‘तुमने अभी आधा घंटा पहले मेरे कान पकड़े थे और मुझे सैकड़ों ऊलजलूल बातें कही थीं। क्या इतनी जल्दी भूल गए?’

प्रश्न 2.
संजाल पूर्ण कीजिए :

उत्तरः

प्रश्न 3.
चौखट में उत्तर लिखिए?

उत्तरः

प्रश्न 4.
(i) गद्यांश से शब्दयुग्म ढूँढ़कर लिखिए –
(1) ……………………………………
(2) ……………………………………
उत्तरः
(i) बुरा – भला
(ii) बैठे – बिठाए

(ii) लिंग परिवर्तन कीजिए –
(i) शेर – ……………………………………
(ii) नौकर – ……………………………………
उत्तरः
(i) शेर – शेरनी
(ii) नौकर – नौकरानी

प्रश्न 5.
‘ईंट का जवाब पत्थर से’ इस मुहावरे को चरितार्थ करता हुआ कोई प्रसंग 10-12 पंक्तियों में लिखिए।
उत्तरः
‘ईंट का जवाब पत्थर से देना’ एक प्रचलित हिंदी मुहावरा है। जिसका अर्थ है कड़ा प्रतिरोध करना या मुँहतोड़ जवाब देना। दुष्ट लोगों के साथ दुष्टता से पेश आना। भारतीय सेना के जाबाज सिपाही सीमा पर अपने दुश्मनों को मुँहतोड़ जवाब देकर उन्हें सबक सिखाते हैं। हमारी रोजमर्रा की जिंदगी में भी ऐसे प्रसंग देखने को मिलते हैं। एक बार सिग्नल पर एक बाइक पर सवार युवक साइकिल पर सँवार लड़की के साथ ऊलजलूल बातें कर रहा था।

लड़की उसकी गुस्ताखी के शालीनता से जवाब दे रही थी। इतने में सिग्नल हुआ और बाइक सँवार चल पड़ा। अब लड़की ने उसका पीछा किया और ऐसा सबक सिखाया कि वह जिंदगी में कभी किसी लड़की को नहीं छेड़ेगा। हाँ, लड़की के विरोध करने पर उसकी मदद के लिए अन्य लोग भी आए और अंत में पुलिस भी आई। लेकिन पहल लड़की ने की और बड़ी हिम्मत दिखाई। उस बाईक सँवार को उसने सड़क के किनारे रोककर दो तमाचे जड़ दिए।

भीड़ जमा हो गई और सब लड़की की ओर से होने के कारण लड़के को शर्मिंदा होना पड़ा। पुलिस ने उसपर एफआयआर कर दी और उसका लाईसेन्स ले लिया। जुर्माना भरना पड़ा, शर्मिंदगी उठानी पड़ी, ये हुई न ‘ईंट का जवाब पत्थर से’ वाली बात।

प्रश्न 6.
गद्यांश पढ़कर दी गई सूचनाओं के अनुसार कृतियाँ कीजिए :
उत्तरः
एक बार शरीर के अंगों में लड़ाई हो गई। इसका आरंभ पैरों ने किया। वे बोले: लड्डू लाना हो या पेड़ा, कचौरी लानी हो या आलू की टिकिया, हमें ही दौड़ना पड़ता है, पर चीज़ लेते ही हाथ उसे थाम लेते हैं, मुँह चट कर जाता है, आँखें देखती हैं, पेट खा जाता है, नाक सूंघती है, हमें क्या मिलता है- हम क्यों बेगार करें! आज से हम नहीं चलेंगे, तो खाते हैं, लेते हैं, वे ही जाएँ, वे ही दौडें।

बस, पैरों की देखा-देखी औरों को भी सूझी। हाथों ने कहा: तुम चलकर जाते हो तो क्या, ढोकर तो हमीं लाते हैं, पर हमें क्या मिलता है, यह अकेला मुँह सब कुछ चट कर जाता है। उन्होंने भी अपना काम छोड़ दिया और इस तरह एक के बाद एक सभी ने छुट्टी की, पर पेट खाली रहा तो शाम को ही सब पर सुती की छाया पड़ी। दूसरे दिन बेचैनी हुई और तीसरे दिन तो सबके सब दम ही तोड़ने लगे।

हँसकर पेट ने कहा: क्यों भाई, कुछ आया मज़ा? तुम समझते थे कि सब कुछ मैं अकेला ही अपने थैले में रख लेता हूँ। अरे भोले भाइयो, यह तो सहकार की बात है। तुम सब अपना काम करके मुझ तक कुछ पहुँचाते हो और मैं अपना काम करके तुम तक कुछ पहुँचाता हूँ और यों हम सब एक-दूसरे को जीवित रखते हैं।

इसी का नाम सहकार भावना है। अंगों ने समझा और उठकर अपने-अपने काम में लगे। बस, जो हाल शरीर का है, वही समाज का है। यहाँ भी सब अपना-अपना काम करते हैं, तो समाज ठीक चलता है। नहीं तो समाज के संगठन में शिथिलता आ जाती है। अब यह बात साफ़ है कि जिसमें सहकारभावना नहीं है, वह समाज का शत्रु है और उसे समाज से जीवनशक्ति ग्रहण करने का कोई अधिकार नहीं है।

प्रश्न 7.
संजाल पूर्ण कीजिए :

उत्तरः

(i) सही विकल्प चुनकर लिखिए –
(1) अरे भोले भाइयो, …………………………………..
(अ) यह तो परोपकार की बात है।
(ब) यह तो सहकार की बात है।
(क) यह तो समझदारी की बात है।
उत्तर :
अरे भोले भाइयो, यह तो सहकार की बात हैं।

(2) जिसमें सहकार भावना नहीं है, वह …………………………………..
(अ) समाज का प्रतिनिधि है।
(ब) समाज का काँटा है।
(क) समाज का शत्रु है।
उत्तर :
जिसमें सहकार भावना नहीं है, वह समाज का शत्रु है।

(ii) उत्तर लिखिए
पेट के खाली रहने के परिणाम

उत्तरः

प्रश्न 8.
(i) निम्नलिखित शब्दों के विलोम लिखिए –
(1) सुस्ती x
(2) सहकार x
उत्तरः
(1) सुस्ती x फुर्ती
(2) सहकार x असहकार

(ii) शरीर के अंगों पर गढ़े मुहावरे लिखिए –
जैसे : पाँव – उलटे पाँव लौटना वैसे
(1) मुँह ……………………………
(2) नाक ……………………………
उत्तरः
(1) मुँह – मुँह की खाना।
(2) नाक – नाक पर मक्खी भी बैठने न देना।

प्रश्न 9.
घर में माँ छुट्टी पर चली गई तो होने वाले परिणाम 10 से 12 वाक्यों में लिखिए
उत्तरः
परिच्छेद में जो हाल सभी अवयवों का हुआ था वैसा ही कुछ मन में आ रहा है। माँ ने अगर घर में ध्यान देना बंद कर दिया तो वक्त पर कुछ भी नहीं हो पाएगा। परिवार की रेलगाड़ी ही पटरी से उतर जाएगी। घर में हाहाकार मच जाएगा। सुबह जगाने से लेकर रात सोने तक हमारी चिंता कौन करेगा?

हम सब का भोजन आदि का बंदोबस्त तो होटल से हो पाएगा और एकाध दिन मजा भी आएगा। लेकिन रोज-रोज न स्वास्थ्य के लिए और न जेब के लिए अच्छा रहेगा। माँ के बनाए भोजन में उसका प्यार जो मिला होता है वह होटल के भोजन में कहाँ से मिलेगा?

हमारी बीमारी में सबसे अधिक चिंता वहीं करती है। अब वह छुट्टी पर चली गई तो हम तो उसके बिना बीमार हो जाएँगे और हमारी देखभाल करने वाली, हमें चैन की नींद मिले इसलिए स्वयं जागने वाली नर्स तो मिलने से रही।

हमें स्कूल कॉलेजों में, पिताजी को दफ्तर में कम-से-कम इतवार की छुटटी तो मिलती ही है लेकिन माँ सप्ताह के सभी दिन और जरूरत पड़ने पर दिन के 24 घंटे हमारी सेवा शुश्रूषा में लगी रहती है। हम सब इस बात के इतने आदी हो गए हैं कि हम नहीं सह पाएँगे माँ की छुट्टी।

प्रश्न 10.
गद्यांश पढ़कर दी गई सूचनाओं के अनुसार कृतियाँ कीजिए:
उत्तरः
तुलसी : फर्माइए

प्राण : (नपी-तुली आवाज में) आप फर्माइए।

तुलसी : जी साब तो…..
प्राण : साहब की ऐसी-तैसी। तुम रास्ते से हट जाओ-आदमी हो या चीन दीवार? (भीतर आकर) क्यों जनाब, यह क्या बदतमीजी है कि कोई दस मील पैदल चलकर हुजूर के दर्शन करने आए और आगे से जवाब मिलता है, (मुँह बनाकर) फर्माइए।

पति : ओह, नहीं-नहीं। आओ-आओ, कहाँ से आ रहे हो?

प्राण : जहन्नुम से- नमस्ते भाभी! (हाथ जोड़ता है और मोढ़ा सरकाकर सोफे के करीब बैठता है। पति-पत्नी भी सोफे पर बैठ जाते हैं।)

प्राण : क्या मैं पूछ सकता हूँ कि हुजूर कल पिकनिक में क्यों तशरीफ नहीं लाए?

पति : अरे क्या बताऊँ भाई, बस यों ही- कुछ देर हो गई- मैंने सोचा….

प्राण : भाभी! मैं तुम्हें बताए देता हूँ कि इन महानुभाव को, जिन्हें तुम्हारा पति होने का सौभाग्य प्राप्त है, बड़ी मजबूत नकेल की जरूरत है।

पति : अरे यार, मजाक छोड़ो। यह बताओ, कहाँ से आ रहे हो इस वक्त?

प्राण : कहाँ से आ रहा हूँ। कमाल है? तो क्या जनाब समझते हैं, मैं आपकी तरह किसी क्लब, किसी होटल, किसा बालरूम या रेसकोर्स से आ रहा हूँ। ये सब गुलछर्रे आप ही को मुबारक हों। शरीफ आदमी हूँ, शरीफों की तरह सीधा दफ्तर से आ रहा हूँ।

पति : अरे, मैं तो इसीलिए पूछ रहा था कि….. खैर, कुछ चाय-वाय पियोगे?

पत्नी : जी हाँ, चाय पीजिएगा?

प्रश्न 11.
संजाल पूर्ण कीजिए

उत्तरः

प्रश्न 12.
(i) कारण लिखिए
(1) प्राणनाथ को नौकर बदतमीज लगा।

(2) प्राणनाथ ने मित्र की पत्नी को सलाह दी कि उसके पति को मजबूत नकेल की जरूरत है।
उत्तरः
(1) क्योंकि प्राणनाथ लंबी दूरी पैदल चलकर अपने मित्र को देखने आए थे और नौकर ने दरवाजे पर उनसे पूछा था फर्माइए।
(2) क्योंकि उनका मित्र पिकनिक में नहीं आया था और न आने का उचित कारण भी नहीं बता सका।

(ii) परिच्छेद के आधार पर दो ऐसे प्रश्न बनाइए जिनके उत्तर निम्न शब्द हो –
(1) दर्शन
(2) मजाक
उत्तरः
(1) दर्शन – प्राणनाथ पैदल चलकर क्यों आए थे?
(2) मजाक – प्राणनाथ को क्या छोड़ने को कहा?

प्रश्न 13.
(i) परिच्छेद से उपसर्गयुक्त शब्द ढूँढकर लिखिए :
(1) …………………………………..
(2) …………………………………..
उत्तरः
(1) बदतमीजी
(2) सौभाग्य

(ii) अनेक शब्दों के लिए एक शब्द लिखिए
(1) ऊँट, बैल आदि की नाक में बँधी हुई रस्सी –
(2) कोई बड़ा आदरणीय व्यक्ति –
उत्तरः
(1) ऊँट, बैल आदि की नाक में बँधी हुई रस्सी – नकेल
(2) कोई बड़ा आदरणीय व्यक्ति – महानुभाव

प्रश्न 14.
‘अतिथि देवो भव’ भारतीय संस्कृति है, इसे १० – १२ पंक्तियों में स्पष्ट कीजिए :
उत्तरः
भारतीय संस्कृति की कई विशेषताएँ हैं। “अतिथि देवो भव'” भारतीय संस्कृति की एक विशेषता है। जब अतिथि को देवता ही मान लिया तो उसके लिए बड़े से बड़ी कुर्बानी भी देने को तैयार हो जाते हैं हम। पुराणों में इसके कई उदाहरण मिलते हैं।

राजा मयुरध्वज अतिथि के स्वागत के लिए खुद को आरे से चिरवाने को भी तैयार हो गए थे। यही परंपरा हम आज भी निभाते हैं। अनेक कठिनाइयों का सामना करते हए भी हम अतिथि का स्वागत करते हैं।

अतिथि सत्कार के संस्कार हम भूल नहीं सकते। अपनी इच्छाओं का समर्पण करने के लिए हम सदैव तैयार रहते हैं। यह हमारा अतिथि प्रेम ही हैं।

आज इस परंपरा में कमी जरूर आई हैं। क्योंकि पहले अतिथि छठे -छमासे आते थे। समय,धन और जगह की कमी नहीं थी और मनोरंजन के साधन भी सुलभ नहीं थे। उस समय अतिथि के पधारने पर मन आनंदित हो उठता था। आज की महानगरीय सभ्यता में समय, स्थान और धन का अभाव है।

ऐसे में अतिथि पधारने पर कई कठिनाइयों का सामना करना पड़ता हैं। फिर भी हम अतिथि का सत्कार करते ही हैं। अपनी संस्कृति को भूल नहीं सकते। और हमें भी तो कभी किसी का अतिथि बनना पड़ता हैं।

प्रश्न 15.
गद्यांश पढ़कर दी गई सूचनाओं के अनुसार कृतियाँ कीजिए:
उत्तरः
छोटे गोल मुख की तुलना में कुछ अधिक चौड़ा लगनेवाला, पर दो काली रूखी लटों से सीमित ललाट, बचपन और प्रौढ़ता को एक साथ अपने भीतर बंद कर लेने का प्रयास-सा करती हुई, लंबी बरौनियोंवाली भारी पलकें और उनकी छाया में डबडबाती हुई-सी आँखें, उस छोटे मुख के लिए भी कुछ छोटी सीधी-सी नाक और मानो अपने ऊपर छुपी हुई हँसी से विस्मित होकर कुछ खुले रहनेवाले होंठ समय के प्रवाह से फीके भर हो सके हैं, धुल नहीं सके।

घर के सब उजले-मैले, सहज-कठिन कामों के कारण, मलिन रेखाजाल से गुंथी और अपनी शेष लाली को कहीं छिपा रखने का प्रयत्न-सा करती हुई कहीं कोमल, कहीं कठोर हथेलियाँ, काली रेखाओं में जड़े कांतिहीन नखों से कुछ भारी जान पड़ने वाली पतली ऊंगलियाँ, हाथों का बोझ सँभालने में भी असमर्थ-सी दुर्बल, रूखी पर गौर बाँहें और मारवाड़ी लहँगे के भारी घेर से थकित-से, एक सहज-सुकुमारता का आभास देते हुए, कुछ लंबी उँगलियों वाले दो छोटे-छोटे पैर, जिनकी एड़ियों में आँगन की मिट्टी की रेखा मटमैले महावर-सी लगती थी, भुलाए भी कैसे जा सकते हैं!

उन हाथों ने बचपन में न जाने कितनी बार मेरे उलझे बाल सुलझाकर बड़ी कोमलता से बाँध दिए थे। वे पैर न जाने कितनी बार, अपनी सीखी हुई गंभीरता भूलकर मेरे लिए द्वार खोलने, आँगन में एक ओर से दूसरी ओर दौड़े थे। किस तरह मेरी अबोध अष्टवर्षीय बुद्धि ने उससे भाभी का संबंध जोड़ लिया था, यह अब बताना कठिन है।

मेरी अनेक सहपाठिनियों के बहुत अच्छी भाभियाँ थीं; कदाचित् उन्हीं की चर्चा सुन-सुनकर मेरे मन ने, जिसने अपनी तो क्या दूर के संबंध की भी कोई भाभी न देखी थी, एक ऐसे अभाव की सृष्टि कर ली, जिसको वह मारवाड़ी विधवा वधू दूर कर सकी।

बचपन का वह मिशन स्कूल मुझे अब तब स्मरण है, जहाँ प्रार्थना और पाठ्यक्रम की एकरसता से मैं इतनी रुआँसी हो जाती थी कि प्रतिदिन घर लौटकर नींद से बेसुध होने तक सबेरे स्कूल न जाने का बहाना सोचने से ही अवकाश न मिलता था।

प्रश्न 16.
चौखट पूर्ण कीजिए :

उत्तरः

प्रश्न 17.
(i) कारण लिखिए –
(1) भाभी की हथेलियाँ मलिन रेखाओं से गुंथी कठोर हो गई थी।
(2) लेखिका स्कूल न जाने का बहाना सोचती रहती थी।
उत्तरः
(1) क्योंकि घर के सब उजले – मैले, सहज – कठिन काम भाभी को ही करने पड़ते थे।
(2) क्योंकि मिशन स्कूल में प्रार्थना और पाठ्यक्रम की एकरसता उन्हें अच्छी नहीं लगती थी।

(ii) निम्नलिखित विधान सही है या गलत लिखिए –
(1) भाभी ने लेखिका के उलझे बाल सुलझाकर कसकर बाँध दिए थे।
(2) लेखिका की अनेक सहपाठिनियों के बहुत अच्छी भाभियाँ थीं।
उत्तरः
(1) भाभी ने लेखिका के उलझे बाल सुलझाकर कसकर बाँध दिए थे। – गलत
(2) लेखिका की अनेक सहपाठिनियों के बहुत अच्छी भाभियाँ थीं। – सही

प्रश्न 18.
(i) परिच्छेद से विलोम शब्द की जोड़ियाँ ढूँढ़कर लिखिए –
जैसे – कोमल x कठोर
वैसे – (1) ………………………………….
(2) ………………………………….
(3) ………………………………….
(4) ………………………………….
उत्तरः
(1) बचपन x प्रौढ़ता
(2) उजले x मैले
(3) सहज x कठिन
(4) उलझे x सुलझे

(ii) ‘आभास’ शब्द से नए अर्थपूर्ण शब्द बनाइए।
(1) ………………………………….
(2) ………………………………….
(3) ………………………………….
(4) ………………………………….
उत्तरः
(1) आस
(2) भास
(3) आभा
(4) सभा

प्रश्न 19.
‘विधवा समाज और परिवार से प्रताड़ित जीवन जीने पर मजबूर होती है इस तथ्य पर अपने विचार लिखिए।
उत्तरः
हमारे समाज में सामाजिक रूढियों एवं परंपराओं की बेड़ियों में जकड़ी विधवाओं की स्थिति बड़ी दयनीय है। विधवा होते ही उन पर तमाम बंदिशे लग जाती हैं। न तो वह कहीं आ जा सकती हैं न मन माफिक खा और पहन सकती है। परिवार और समाज से प्रताड़ित विधवा का जीवन घोर निराशता से भर जाता है। रंगीन वस्त्र पहनना वर्जित हो जाता है और सफेद लिबास में लिपटी रहना उसकी नियती।

दूसरा विवाह कर सुनहरे भविष्य की आशा से भी उसे वंचित कर दिया जाता है। बिना रोशनदान, बिना झरोखा, बिना नौकर चाकर और बिना पशु पक्षियों वाले अँधेरे घर में घुट – घुटकर जीने को उसे विवश किया जाता है। समाज विधवा पर संयम और अनुशासन से रहने की बंदिशें तो लगाता है पर उसके आहार – विहार, मनोरंजन एवं स्वास्थ के प्रति कठोर और उदासीन रहता है।

पति के जीवित रहते जो घर की स्वामिनी थी ,मृत्यु के बाद उसे दासी समझा जाने लगता है। बाल विधवा के साथ तो समाज और क्रूरता का व्यवहार करता है। छोटी छोटी भूलों पर उसे मारा-पिटा और दागा जाता है । उसे पशु से भी बदतर जीवन जीने को विवश किया जाता है।

समाज की घिनौनी पाशविक प्रवृत्ति के चलते बाल-विधवा को छोटी उम्र में ही प्रौढ़ और वृद्ध बनने पर मजबूर कर दिया जाता है। इस तरह विधवाओं को समाज की संकीर्ण और विकृत मानसिकता का शिकार होना पडता है।

प्रश्न 20.
परिच्छेद पढ़कर दी गई सूचनाओं के अनुसार कृतियाँ कीजिए:
उत्तरः
सन 1947 में भारत आजाद हुआ। वास्तव में व्यापार और उद्योग देश की रीढ़ की हड्डी के समान होते हैं, परंतु … समाजवादी समाज रचना का लक्ष्य होने से सरकार ने इनके विकास की ओर ध्यान नहीं दिया। मुक्त और उदार अर्थव्यवस्था से … ही आर्थिक और औद्योगिक विकास संभव है- इस बात को समझने में हमारे नेताओं को चौंतीस वर्ष लगे।

शंतनुराव जी आरंभ से ही इस नीति के समर्थक थे। उनके विचारों के अनुसार ‘सादा रहन-सहन’ ही बेरोजगारी की जड़ है। रोजगारी से निर्माण हुई वस्तुओं का प्रयोग किए बिना रोजगारी कैसे चलेगी? यदि कोई शानदार बंगला, श्रेष्ठ संगीत, बढ़िया कपड़ा या साड़ी इस्तेमाल ही न करे, तो देश में गरीबी और बेरोजगारी बढ़ती ही जाएगी। इनको रोकने के लिए हर एक को अपनी जरूरतें बढ़ानी होंगी।

उद्यमकर्ता कामगारों का शोषण नहीं करता, उल्टे-उन्हें काम देकर गरीबी की खाई से बाहर निकालता है।

आधुनिक जेटयुग के इस महापुरुष ने किर्लोस्कर ब्रदर्स कंपनी के अंतर्गत विभिन्न उत्पादन, व्यवसाय करने वाली लगभग चालीस कंपनियाँ खोलकर उसे किर्लोस्कर उद्योग समूह में परिवर्तित किया। वे कहा करते, “जो भी काम करो, बढ़िया ढंग से करो और उसमें सफलता पाने के लिए मुसीबतों की परवाह न करते हुए, अंत तक मन को थकने न दो।”

आपने इंजीनियरी क्षेत्र के अलावा होटल, परामर्शसेवा (कन्सलटन्सी), संगणक, लीजिंग तथा फाइनान्स आदि क्षेत्रो में भी भरसक योगदान दिया।

आपको 1965 में पद्मश्री, सन 1984 में ‘मराठा चेंबर ऑफ कॉमर्स’ की मानद सदस्यता और सन 1988 में पुणे विश्वविद्यालय की डी. लिट. उपाधि से सम्मानित किया गया। इनके अलावा इंजीनियरी क्षेत्र में उल्लेखनीय कार्य करने के उपलक्ष्य में उन्हें विभिन्न पुरस्कार मिले। औद्योगिक क्षेत्र में उनका जो महत्त्वपूर्ण अंशदान रहा, उसी के कारण आपको औद्योगिक क्षेत्र के भीष्माचार्य’ कहा जाता है।

प्रश्न 21.
संजाल पूर्ण कीजिए।

उत्तरः

प्रश्न 2.
(i) कारण लिखिए –
(1) सादा रहन-सहन ही बेरोजगारी की जड़ है।
(2) शंतनुराव को औद्योगिक क्षेत्र के भीष्माचार्य कहा जाता है।
उत्तरः
(1) क्योंकि शानदार बंगला, श्रेष्ठ संगीत, बढ़िया कपडे इस्तेमाल ही न करेंगे तो देश में गरीबी और बेरोजगारी बढ़ती ही जाएगी।
(2) क्योंकि उनका औद्योगिक क्षेत्र में बहुत बड़ा योगदान रहा है।

(ii) सही विकल्प चुनकर लिखिए –
(1) व्यापार और उद्योग …………………………… की रीढ़ की हड्डी के समान होते हैं। (व्यक्ति / देश / समाज)
(2) मुक्त और उदार …………………………… से ही आर्थिक और औद्योगिक विकास संभव है। (अर्थव्यवस्था / नीति संस्कार)
उत्तरः
(1) व्यापार और उद्योग देश की रीढ़ की हड्डी के समान होते है।
(2) मुक्त और उदार अर्थव्यवस्था से ही आर्थिक और औद्योगिक विकास संभव है।

प्रश्न 3.
(i) अंग्रेजी शब्दों के हिंदी अर्थ लिखिए :
(1) फाइनान्स – ……………………………
(2) कॉमर्स – ……………………………
(3) इंजीनियरी – ……………………………
(4) चेंबर – ……………………………
उत्तरः
(1) फायनान्स – वित्त
(2) कॉमर्स – वाणिज्य
(3) इंजीनियरी – तकनिकी
(4) चेंबर- कक्ष

(ii) निम्नलिखित शब्दों के विलोम लिखिए :
(1) आजाद x ……………………………
(2) समर्थक x ……………………………
(3) पुरस्कार x ……………………………
(4) सम्मानित x ……………………………
उत्तरः
(1) आजाद x गुलाम
(2) समर्थक x विरोधक
(3) पुरस्कार x दंड
(4) सम्मानित x अपमानित

प्रश्न 4.
सफल उद्योजक के गुण 10-12 वाक्यों में लिखिए।
उत्तरः
सफल उद्योजक बनने के लिए चुनौतियों से भरी राह पर निरंतर गतिशील रहते हुए आगे बढना होगा। उत्पादन के लिए महत्त्वपूर्ण है कच्चा माल, मशीनें और कर्मचारी जो प्रशिक्षित हो। इन तीनों के अभाव में उत्पादन संभव नहीं। ये तीनों हैं और उत्पादन भी अच्छी तरह से हो गया तो उत्पादन को बेचने के लिए बाजार भी चाहिए। उद्योजक को चाहिए कि वह अपने उत्पादन का स्तर हर हाल में उच्च कोटी का रखे, जो भी उत्पादन हो वह बढ़िया से बढ़िया हो।

हर समस्या को बारिकी से जानने समझने की जिज्ञासा उसमें हो, कठिनाइयों से जूझने की दृढ़ता उसमें हो। जोखिम स्वीकारने के लिए वह सदैव तत्पर रहे। वह दूरदर्शी होना चाहिए, अगले 50-100 वर्षों का अनुमान लगाने की क्षमता उसमें हो। उसका दृष्टिकोण व्यावहारिक हो।

अपने कर्मचारियों के प्रति विश्वास और स्वयं पर भरोसा होना चाहिए। बाजार की प्रतिस्पर्धा में टिकने के लिए अनगिनत कष्ट उठाने की उसकी तैयारी होनी चाहिए। वह पहले दर्जे का संयोजक एवं प्रबंधक होना चाहिए और सबसे महत्त्वपूर्ण बात वह महत्त्वाकांक्षी होना चाहिए। इतने सारे गुण जिस उद्योजक के पास है वह नाम और शोहरत कमाएगा और सफलता की चोटी पर पहुँचेगा।

Balbharti Maharashtra State Board Hindi Yuvakbharati 11th Digest रचना निबंध लेखन Notes, Questions and Answers.

Maharashtra State Board 11th Hindi रचना निबंध लेखन

निबंध लेखन :

गद्य लिखना अगर कवियों की कसौटी है तो निबंध लिखना गद्यकारों की कसौटी है। निबंध शब्द दो शब्दों से मिलकर बना है नि-बंध। बंध का अर्थ है बाँधना या बंधा हुआ इसमें लगे ‘नि’ उपसर्ग का अर्थ होता है अच्छी तरह से। अत: निबंध का तात्पर्य उस रचना से है जिसे अच्छी तरह बाँधा गया हो।

किसी भी विषय पर अपने भाव, विचार, अनुभव जानकारी इत्यादि को अपनी शैली में क्रमबद्ध कर अभिव्यक्त करना ही निंबध है। निबंध कैसे लिखा जाय? यह महत्त्वपूर्ण है। भाषा शैली का इसमें विशेष महत्त्व है।

निबंध लेखन में महत्त्वपूर्ण बातें

उपर्युक्त क्रम से अंकित एक से बारह तत्त्वों को अनुच्छेद के अनुसार व्यक्त किया जा सकता है। इसी रूप में निबंध को विस्तार दिया जाता है। यदि इसको संक्षिप्त करना है तो दो तत्त्वों को एक अनुच्छेद में समाहित कर अभिव्यक्त किया जा सकता है।

विषय को भली प्रकार से समझ बूझकर उसकी भूमिका बाँधनी चाहिए और विषय प्रवेश के साथ उसके महत्त्व को उजागर करना चाहिए। विस्तार में विषय के प्रकार, शिक्षा विकास, सामाजिक महत्त्व आदि दिखाना चाहिए। विचार स्पष्ट, तर्कपूर्ण एवं सुलझा हुआ होना चाहिए। निबंध में विषयांतर एवं पुनरुक्ति दोष से बचना आवश्यक होता है।

निबंध के संपादन के साथ-समापन भी आकर्षक होना चाहिए। इसमें लेखक का अपना विचार होना आवश्यक होता है। निबंध की भाषा सरल, प्रभावी व व्याकरणनिष्ठ होनी चाहिए। वाक्य जितने छोटे व स्पष्ट होंगे, निबंध उतना ही प्रभावशाली होगा।

निबंध को प्रभावशाली बनाने के लिए प्रसिद्ध काव्य पंक्तियों, उक्तियों, मुहावरों, सटीक लोकोक्तियों व घटनाओं का प्रयोग किया जा सकता है। वर्तनी की शुद्धता के साथ विराम चिह्नों का प्रयोग कुशलता पूर्वक करना चाहिए।

निबंध के प्रकार : निबंध पाँच प्रकार के होते हैं :

1. वर्णनात्मक निबंध
2. कथात्मक या विवरणात्मक निबंध
3. कल्पनात्मक निबंध
4. आत्मकथात्मक निबंध
5. विचारात्मक निबंध।

(1) वर्णनात्मक निबंध : इस निबंध में वर्णन की प्रधानता रहती है। वर्णन में कभी-कभी निजी अनुभूति एवं कल्पना का रंग भी भरना पड़ता है। वस्तु, स्थान, घटना, प्रसंग, यात्रा, अनुभव आदि का रोचक वर्णन किया जाता है। प्राकृतिक दृश्य, त्योहार, उत्सव में एक घंटा आदि निबंध इसी प्रकार के अंतर्गत आते हैं। ‘वर्षा का एक दिन’ निबंध भी इसी के अंतर्गत आता है।

(2) कथात्मक या विवरणात्मक निबंध : किसी घटना अथवा कथा का विवरण, किसी प्रसंग का चित्रण या निरूपण, किसी की जीवन कथा, या आत्मकथा आदि का समावेश इस प्रकार के निबंधों में होता है। निर्जीव वस्तु की आत्मकथा भी यथार्थ का भ्रम करा सके, ऐसी शैली में लिखना चाहिए। जैसे – महात्मा गांधीजी, रेल दुर्घटना, बाढ़ का प्रकोप आदि निबंध।

(3) कल्पनात्मक निबंध : जिन निबंधों में कल्पना तत्त्व की प्रधानता होती है, उसे कल्पना प्रधान निबंध कहते हैं। इसके अंतर्गत जो बात नहीं होती, उसकी कल्पना की जाती है, कभी असंभव – सी बातों को संभव माना जाता है। लेखक कल्पना की ऊँची उड़ान ले सकता है। इस प्रकार के निबंधों के अंतर्गत यदि – होता, अगर …… न होता, मेरी अभिलाषा आदि विषय हैं। जैसे – यदि परीक्षा न होती, अगर मैं बंदी होता, अगर मैं प्रधानमंत्री होता आदि।

(4) आत्मकथात्मक निबंध : इसमें किसी वस्तु, प्राणी या व्यक्ति की आत्मकथा होती है। विद्यार्थी अपने आपको वह वस्तु, प्राणी या व्यक्ति मानकर निबंध लिखता है। इसमें लेखक कल्पना की उड़ान भर सकता है। इसमें जीवित व निर्जीव दोनों तरह की घटना का आरंभ उत्तम पुरुष से होता है। इसमें किसी के दुःख-सुख के साथ लेखक अपने विचारों को भी प्रस्तुत करता है। जैसे – कुर्सी की आत्मकथा, फूल की आत्मकथा, फटे पुस्तक की आत्मकथा आदि।

(5) विचारात्मक निबंध : ऐसे निबंधों में विचार प्रमुख होता है इसमें कल्पना का पुट न के बराबर होता है। इसका आधार तर्क या प्रमाण होता है। किसी के पक्ष या विपक्ष में सकारात्मक तथा नकारात्मक तथ्यों का संपादन बड़ी कुशलता से किया जाता है। समीक्षा व आकलन इस निबंध का आधार होता है।

गरीबी एक अभिशाप, माँ की ममता, वृक्ष लगाओ देश बचाओ, विविधता में एकता, वही मनुष्य है कि जो मनुष्य के लिए मरे, जीवन का लक्ष्य, आदर्श मित्र, आदर्श विदयार्थी, सदाचार का महत्त्व, समय का सदुपयोग, परोपकार, राष्ट्रभाषा की समस्या, समाचार पत्र, विज्ञान-वरदान या अभिशाप, स्त्री भ्रूण हत्या, भ्रष्टाचार उन्मूलन आदि विषय इसके अंतर्गत आते हैं।

Maharashtra Board Class 11 Hindi निबंध

1. होली का त्यौहार

हमारे यहाँ त्योहारों का सिलसिला वर्षभर चलता है। इसीलिए हमारे देश को त्योहारों का देश कहते हैं। ईद, बकरी ईद, ओणम, पोंगल, बैसाखी, रक्षा बंधन, होली, दशहरा, दीपावली, इत्यादि प्रमुख त्योहार हैं। होली रंगों का त्यौहार है।

होली का त्योहार मनाने के पीछे धार्मिक कारण है। कहते हैं कि हिरण्यकश्यप नामक शैतान, प्रहलाद जैसे ईश्वर भक्त बेटे का पिता था, जो घमंड के कारण अपने आप को ईश्वर समझता था। उसकी एक बहन होलिका थी जिसे वरदान था कि वह अग्नि में नहीं जलेगी।

होलिका अपने भाई की मदद के लिए प्रहलाद को लेकर जलती हुई अग्नि में बैठ गई। नारायण की कृपा से प्रहलाद तो बच गया लेकिन होलिका जल गई। तभी से होलिका दहन किया जाने लगा। यह असत्य पर सत्य की विजय का पर्व है। जिसके दूसरे दिन लोग रंगों से एक दूसरे का स्वागत करते हैं।

हमारा देश किसानों का देश है। यह उनकी फसलों का भी त्योहार है। फसल का रसास्वादन होली की खुशी लेकर आता है। लोग एक-दूसरे को अबीर-गुलाल लगाकर नाचते-गाते हैं। इस दिन शैतान को कबीरा सुनाकर ताना भी मारा जाता है। होली के गीत अत्यंत मनोरंजक व आकर्षक होते हैं।

भगवान श्री कृष्ण राधा के साथ होली खेलते थे। बरसाने और ब्रज की लठमार होली आज भी उसी उमंग से मनाई जाती है। लोग मिठाई बाँटते हैं, ठंडाई पीते हैं। अपने गिले-शिकवे मिटाकर एक-दूसरे को गले लगाते हैं। सभी होली के रंग में घुल-मिल जाते हैं।

कुछ गलत परंपराएँ चल पड़ी हैं जिसे रोकना अनिवार्य है। जैसे – गंदा पानी, कीचड़, गोबर, पेंट, शराब व भाँग का प्रचलन। नशे की हालत में किया गया व्यवहार इस सुंदर पर्व को बदरंग कर देता है, जिससे आर्थिक नुकसान के साथ आपसी दुश्मनी को बढ़ावा मिलता है। घातक रंगों के प्रयोग से आँखों की रोशनी पर भी कुप्रभाव पड़ता है।

होली के स्नेह सम्मेलन एक – दूसरे को आपस में जोड़ते हैं-

होली के दिन दिल मिल जाते हैं
रंगों में रंग मिल जाते हैं।
गिले-शिकवे सभी भूल कर
दुश्मन भी गले मिल जाते हैं।

यदि गंदगी फूहड़ता तथा नशे पर रोक लगाई जा सके, तो इससे उत्तम पर्व कोई भी नहीं हो सकता।

2. राष्ट्रभाषा हिंदी

राष्ट्रभाषा हमारे विचारों की संवाहक होती है। इसके माध्यम से हम अपने भावों और विचारों को अभिव्यक्त करते हैं। प्रत्येक देश की भाषा उसकी अपनी पहचान होती है। उसका संपूर्ण कार्य उसी भाषा में होता है। राष्ट्रभाषा किसी राष्ट्र के उद्गार का माध्यम होती है। फ्रांस, चीन, जर्मनी, जपान, रूस अपनी भाषा की बदौलत आज पूरे विश्व में अपनी पहचान बनाए हुए हैं और महाशक्ति के रूप में जाने जाते हैं।

हमारे देश की सर्वाधिक जनता हिंदी भाषा का प्रयोग करती है, इसी कारण महात्मा गांधीजी ने कहा था कि हिंदी ही राष्ट्रभाषा बनने योग्य हैं। इसीलिए 14 सितंबर 1949 को भारतीय संविधान में हिंदी को राष्ट्रभाषा के रूप में प्रस्तावित किया गया। पूरे देश को हिंदी सीखने के लिए 15 वर्ष का समय दिया गया। इसे 14 सिंतबर 1964 से कार्यान्वित करने का भी प्रस्ताव था किंतु राजनैतिक कारणों से हिंदी को राष्ट्रभाषा के रूप में आज भी संसद में पारित नहीं किया गया है।

जिस देश की अपनी कोई भाषा नहीं, वह देश या राष्ट्र गूंगा है।

भूतपूर्व प्रधान मंत्री अटल बिहारी वाजपेयीजी ने हिंदी को संयुक्त राष्ट्र संघ की भाषा तो बना दिया किंतु राष्ट्रभाषा हिंदी संसद की भाषा नहीं बन सकी। मारीशस, फिजी, त्रिनिदाद, सूरीनाम, गुयाना, कनाडा, इंग्लैण्ड, नेपाल आदि देशों में हिंदी की अपनी एक अलग पहचान है। भारत में यह षडयंत्र की शिकार है।

14 सिंतबर को हर वर्ष ‘हिंदी दिवस’ मनाया जाता है। जब तक हम व्यावहारिक रूप में राष्ट्रभाषा को स्वीकार नहीं करते तब तक भारत के संपूर्ण विकास पर प्रश्न चिह्न लगा रहेगा।

राष्ट्रभाषा हिंदी ही है, जो पूरे-देश को एक सूत्र में बाँधने की क्षमता रखती है। इसे शिक्षा का माध्यम बनाने से हमारे देश में अत्यधिक बहुमुखी प्रतिभाएँ निकल कर आगे आएँगी। महात्मा गांधीजी ने भी स्वीकार किया था कि शिक्षा मातृभाषा में होनी चाहिए; उच्च व तकनीकी शिक्षा भी हिंदी माध्यम से दी जा सकती है।

3. भ्रष्टाचार :

एक राष्ट्रीय अभिशाप । एक समय था जब चुनाव से पहले हर राजनैतिक दल इस देश से भ्रष्टाचार मिटाने का वादा किया करते थे। देश में चुनाव होते गए और राजनैतिक दल अदल-बदल कर सत्तारूढ़ होते गए। जैसे-जैसे दिन बीतता गया इस देश में भ्रष्टाचार बढ़ता गया, अब तो आकंठ डूबे भ्रष्टाचार और राजनेता एक-दूसरे के पर्याय बन गये हैं। अब कोई भी राजनैतिक दल भ्रष्टाचार मिटाने की बात नहीं करता। सभी इस विशालकाय दैत्य के सामने नतमस्तक हैं।

भ्रष्टाचार का अर्थ है दूषित आचरण या बेईमानी। आज भ्रष्टाचार की काली छाया संपूर्ण देश में अमावस्या की तरह व्याप्त हो गई है और सत्तासीन लोग भ्रष्टाचार मिटाने के नाम पर बहती गंगा में हाथ धो रहे हैं। अब भ्रष्टाचार के नाम पर नाक-भौं सिकोड़ने की बजाय इसे अंगीकार कर लिया गया है।

आज भी कुछ लोग ऐसे हैं, जो भ्रष्टाचार से कोसों दूर हैं किंतु वे भ्रष्टाचारियों का विरोध करने की हिम्मत नहीं जुटा पाते। दुःस्साहस करनेवाले मुँह की खाते हैं उनकी आवाज नक्कारखाने में तूती की आवाज बनकर रह जाती है।

वैसे तो भ्रष्टाचार कमोबेश पूरे विश्व में व्याप्त है किंतु हमारे देश में यह सिंहासनारूढ़ है। इसका कारण है हमारे देश की चुनाव पद्धति। जिसे जीतने के लिए प्रत्याशी पानी की तरह पैसा बहाते हैं। अपनी सेवानिष्ठा ईमानदारी, योग्यता के बल पर न ही कोई चुनाव लड़ता है और न ही जीत पाता है। चुनाव में सफल होने पर वह हर हाल में अपना खर्च किया हुआ पैसा ब्याज के साथ वसूलता है। पैसे की प्राप्ति की अधीरता ही उसे भ्रष्टाचारी बनने को मजबूर करती है।

इसका दूसरा कारण है भौतिकवादी सभ्यता का प्रसार और पाश्चात्य देशों का अंधानुकरण। लोग सारे नियम कानून को ताक पर रखकर पैसा कमाने के चक्कर में भ्रष्टाचारी बन जाते हैं। चारों तरफ धन बटोरने की अफरा-तफरी मची हुई है। लोग विदेशी बैंकों में पैसे जमा करते जा रहे हैं।

आज का प्रत्यक्ष आकड़ा बताता है कि भारतीय भ्रष्टाचारियों का चौदह हजार लाख करोड़ रुपया विदेशी बैंकों की शोभा बढ़ा रहा है जो निश्चित रूप से काला धन है। सबने इसे अपनी जीवन पद्धति में शामिल कर लिया है।

नशीले पदार्थो का व्यापार कानून व्यवस्था के रखवालों के हाथ की कठपुतली बन चुका है। देश का युवावर्ग भ्रष्टाचारियों को आदर्श मानकर उसी रास्ते पर चल रहा है। उनके मन से राष्ट्राभिमान और राष्ट्र-प्रेम लुप्त होता जा रहा है। तकनीकी और प्राथमिक शिक्षण व्यवसाय बन चुका है।

बाबा रामदेव, अन्ना हजारे जैसे लोग इसके खिलाफ आवाज उठाते हैं। यदि हम राष्ट्र को विश्व की प्रथम पंक्ति में बिठाना चाहते है तो भ्रष्टाचार रूपी रावण का दहन आवश्यक है। समाज सेवकों की मेहनत रंग लाएगी। सत्तासीनों की पोल खुलेगी, जनता जगेगी, निश्चित रूप से काला धन वापस आएगा।

देश का युवावर्ग जिस दिन जगेगा भ्रष्टाचार के रावण का अंत होगा और ध्वंस होगा भ्रष्टाचार का साम्राज्य। नए राष्ट्र का उदय होगा और तब साकार होगा। ‘मेरा भारत महान’ का स्वप्न।

4. मैं मोवाईल वोल रहा हूँ

आज विज्ञान प्रदत्त सुविधाओं को हम नकार नहीं सकते। दूरदर्शन, दूरध्वनि, ट्रांजिस्टर ,संगणक, विमान, राकेट, आदि की खोज ने मानव जीवन को एक नई दिशा दी है। कुछ दिन पहले ही पेजर आया बाद में लोगों को पता चला कि फोन भी आ रहा है। अब जब से मेरा आगमन हुआ है मैनें लोगों की दुनिया में क्रांति ला दी है।

जब मेरा बड़ा भाई टेलिफोन इस दुनिया में आया तो उसने पत्रलेखन की कमी को दूर कर लोगों के आपसी संबंध को जोड़ने का प्रयास किया। लेकिन जैसे ही मैंने इस दुनिया में कदम रखा बड़े भाई की परेशानी दूर कर दी। लोगों ने मुझे अपनी जेब में रखना शुरू किया।

मैंने भी लोगों की हर सुविधा का ध्यान रखा। फोटोग्राफी, खेल, सिनेमा, धारावाहिक, एफ एम रेडियो से लेकर हर सुविधा जो दृश्य – श्रव्य साधनों द्वारा प्राप्त होती है, मैंने दी। हाँ! आया, ठीक सुना आपने मैं मोबाइल बोल रहा हूँ। जब से मैंने इस दुनिया में कदम रखा है, तब से सारे संसार में एक क्रांति आ गई है।

विज्ञान ने जो कुछ भी दिया मैं भी उसी की एक कड़ी हूँ। मैं आप लोगों की दिन – रात सेवा कर रहा हूँ। मैंने ऐसी मुहब्बत दी है कि मुझे एक पल के लिए भी आप अपने से अलग नहीं कर पाते।

आपको मैंने सुविधा दी और आप ने भी अपनी जेब से मुझे निकाल कर हाथ की बजाय एक तार से जोड़कर अपने कान में लगा लिया और घंटों बातें करते रहते हैं।

मेरे दोस्तों मुझे दुःख है कि लोगों ने मेरा दुरुपयोग करना शुरू कर दिया है। पता नहीं लोग इतना झूठ क्यों बोलते हैं। मेरी मोहब्बत में अंधे होकर अपनी जान क्यों दे रहे हैं? लोगों का मुझ पर आरोप है कि मैं लोगों का समय बरबाद कर रहा हूँ।

मैंने लोगों को झूठ बोलना सिखाया है। मैंने माहौल को गंदा किया है। आतंकवाद और भ्रष्टाचार को बढ़ाने में भी मेरा उपयोग हो रहा है। परीक्षा के समय भी छात्र मेरा उपयोग नकल करने में करते हैं। लेकिन इसमें मेरी गलती नहीं है।

मैं सबकी मदद करता हूँ। लोगों के दुःख, दर्द को दूर करता हूँ। लोगों के आपसी संबंधों में मधुरता लाता हूँ। इंटरनेट पर होनेवाली, घटनाओं की जानकारी देता हूँ। लोग मेरा सदुपयोग करने की बजाए दुरुपयोग करें, तो इसमें मेरी क्या गलती? मेरी दीवानगी में यदि आप अपना काम छोड़कर निष्क्रिय बन रहे हैं तो मैं क्या करूँ? मेरे दोस्तों मेरा सही प्रयोग करके मुझे बदनामी से आप ही बचा सकते हैं।

यदि मेरा सदुपयोग करेंगे तो मैं कभी किसी को कोई नुकसान नहीं पहुंचा सकता। मैं सूचना पहुँचाने का माध्यम हूँ। मनोरंजन का साधन हूँ। ज्ञान का भंडार हूँ। आपकी हर समस्या का समाधान हूँ। मुझे वही बने रहने दीजिए। मैं तो हमेशा आपकी सेवा में संलग्न रहना चाहता हूँ।

5. दीपावली के पटाखे

पिछले पंद्रह दिनों से लगातार पटाखों के शोर ने मेरी नींद उड़ा दी है। मैं तंग आ गया हूँ घर में बीमार पत्नी कराह रही थी। मैंने नीचे जाकर लोगों से मिन्नतें की लेकिन त्योहार के नाम पर शोर मचानेवालों ने परंपरा की बात कहकर मेरा मजाक उड़ाया। नियम से दस बजे तक ही पटाखे फोड़ने चाहिए लेकिन पूरी रात तक इसका क्रम चलता रहा। दिवाली के दिन तो हद हो गई।

जिसने मुझे चिढ़ाया था, परंपरा की दुहाई दी थी, संस्कृति और पर्व के नाम पर भाषण सुनाया था, पटाखे के धमाके से उसके पिता को दिल का दौरा पड़ा। आधी रात को हम लोग उन्हें अस्पताल ले गए पर दुर्भाग्य कि अब वे एक जिंदा लाश बनकर रह गए हैं।

ध्वनि प्रदूषण का कुप्रभाव सारी खुशियों पर पानी फेर गया। मैंने सुबह सारे कचरे को इकट्ठा करवाकर जलाया, सफाई करवाई, युवकों, बड़ों व बच्चों को बुलाकर समझाया कि जितना पैसा पटाखों में खर्च किया जाता है, उतने पैसों से हम बगीचा बनवा सकते हैं, जो हमें प्रदूषण से राहत देगा।

फिर किसी को जिंदा लाश नहीं बनना पड़ेगा। त्योहार खुशियाँ बाँटने के लिए होते हैं, दर्द देने के लिए नहीं। थोड़े लोगों में सहमति बनी। आज हमारी सोसायटी का बगीचा अन्य लोगों के लिए आदर्श बन चुका है। सबने पटाखे न फोड़ने का संकल्प तो नहीं किया किंतु नियमानुसार फोड़कर पर्व को मनाने का निर्णय अवश्य लिया।

व्यक्ति संस्कारों से सँवरता है, निखरता है। उसके व्यक्तित्व को गढ़ने का कार्य भी संस्कार ही करते हैं। किशोरावस्था और कुमारावस्था में छात्रों के लिए संस्कारगत मूल्यों की शिक्षा अनिवार्य है। इसका मानव जीवन के आचरण पर अत्यधिक प्रभाव पड़ता है।

कुछ नीतिपरक मूल्य मनुष्य को आदर्श नागरिक बनाने में सहायक होते हैं। इस संदर्भ में किसी महान मानव के चरित्र के ऊपर भी कुछ लिखा जा सकता है।

उदाहरणार्थ कुछ संकेत निम्नलिखित हैं।

• जनमत का आदर करनेवाला मानव वास्तविक नायक बन जाता है। संसार के महान पुरुषों के चरित्र को आधार बनाकर इस कथन को अभिव्यक्ति दी जा सकती है।
• आज शहरी जीवन में स्वार्थांधता इतनी बढ़ गई है कि अपनत्व का भाव लुप्त होता जा रहा है। संवेदना धुंधली होती जा रही है, मानवता कहीं न कहीं लुप्त होती जा रही हैं।

6. अब्राहम लिंकन

अमेरिका के एक गरीब परिवार में जन्म लेनेवाला बालक अब्राहम लिंकन जिसने बचपन में अत्यंत अभावपूर्ण परिस्थिति में परवरिश पायी। घर की टूटी खिड़कियाँ और टूटी हुई छत, ऊपर से बिजली का अभाव, बचपन में पिता के साथ मजदूरी करने को मजबूर भरपेट भोजन का अभाव उसे घेरे रहता था।

कहते हैं “जहाँ चाह वहाँ राह” कुशाग्र बुद्धि, बहादुर, हँसी मजाक करने वाला बालक मित्रों से पुस्तकें माँगकर पढ़ उसे लौटा देता। बुद्धि इतनी तीव्र कि पुस्तक का एक-एक शब्द उसकी याददाश्त का हिस्सा बन जाते।

बिजली के अभाव में सड़क के खंभे से आते प्रकाश को पढ़ने के लिए प्रयोग करते देख एक अमीर ने उसको पढ़ने के लिए पुस्तकें उपलब्ध कराई। उसकी लगन, मेहनत और प्रतिभा ने उसे महान वकील बना दिया।

अमेरिका का कलंक वहाँ की दास प्रथा थी। उससे मुक्ति दिलाने का काम अब्राहम लिंकन ने किया। इसी दृढ संकल्प शक्ति से वे एक दिन अमेरिका के राष्ट्रपति बने। यदि हमारे अंदर दृढ़ इच्छा शक्ति है तो सृजनात्मक मूल्य अपने आप विकसित होते हैं और हमें ऊँचाई प्रदान करते है।

हमारे बीच ऐसी प्रतिभाओं की कमी नहीं है। हमें नहीं भूलना चाहिए कि गरीबी की कोख से पले- बढ़े, संघर्षरत, दृढ़ इच्छा शक्ति वाले गाँव के एक किसान बालक लालबहादुर शास्त्री ने भारत का प्रधान मंत्री बनकर देश को “जय जवान जय किसान” का नारा दिया।

संत महात्माओं, साहित्यकारों, मनीषियों ने अपने विचारों को अभिव्यक्त कर जो अमृत संदेश दिया, उसे भुलाया नहीं जा सकता। उनकी प्रसिद्ध उक्तियाँ ही सूक्तियाँ कहलाती हैं। उन उक्तियों या सूक्तियों को आधार मान कर आप अपने विचार अभिव्यक्त कर सकते हैं। कुछ उदाहरण निम्न हैं।

1. “ढाई आखर प्रेम का, पढ़े सो पंडित होय।”
2. है अंधेरी रात पर दीया जलाना कब मना है?
3. “तभी समर्थ भाव है कि तारता हुए तरे, वही मनुष्य है कि जो मनुष्य के लिए मरे।’
4. “नाश के दुःख से कभी, दबता नहीं निर्माण का सुख”
5. “मन के हारे हार है, मन के जीते जीत।”

इन कहावतों में मानव जीवन का महान सत्य प्रस्तुत किया गया है। मानव जीवन में उसका मन ही उसकी सारी गतिविधियों का संचालन करता है। जीवन में अनुकूल -प्रतिकूल परिस्थितियों का आना – जाना लगा रहता है। यदि प्रतिकूल परिस्थितियों में हम अपना धैर्य बनाए रखें, तो हम उस पर विजय पाने में सफल रहते हैं। इसके विपरीत यदि हम में निराशा और अधीरता घर कर जाए तो साधन संपन्न रहने पर भी पराजय ही हमारे हाथ लगती है।

सच्ची तंदुरुस्ती और आत्मनिर्भरता हमारे विजय का मार्ग प्रशस्त करती है। खेल में कभी हार तो कभी जीत मिलती है लेकिन हार में यदि हम निराश हो जाएँ तो सब कुछ बिखर जाएगा। हमें हर परिस्थिति में यह मानकर चलना है।

“क्या हार में क्या जीत में किंचित नहीं भयभीत मैं संघर्ष-पथ पर जो मिले, यह भी सही वह भी सही “हार मानूँगा नहीं, वरदान माँगूगा नहीं” इस सूत्र को जीवन का आधार बनाकर एक साधारण परिवार में जन्म लेने वाले छत्रपती शिवाजी महाराज ने अपनी दृढ़ इच्छा शक्ति से आदिलशाही सुलतानों, पुर्तगालियों, मुगलों से लोहा लिया और विजय पाई। समाज के तमाम विरोध के बावजूद महात्मा ज्योतिबा फुले ने महाराष्ट्र में स्त्री शिक्षा के प्रचार-प्रसार का महान कार्य किया।

7. 26 जुलाई

वाह रे! मुंबई और वाह रे मुंबईकर! ऐसी ताकत हिम्मत और हौसले को प्रणाम करता हूँ वरना हिम्मत, हौसला और दृढ इच्छाशक्ति के बिना उस परिस्थिति से उबर पाना आसान न था। क्या छोटा क्या बड़ा? क्या अमीर क्या गरीब। एकता की एक श्रृखंला बन गई। दुनिया के सामने एक मिसाल – लोग कह उठे वाह रे! मुंबई और वाह रे मुंबईकर!

जब से मनुष्य ने विज्ञान की शक्ति पाकर प्रकृति से छेड़छाड़ प्रारंभ की तथा उसका दोहन प्रारंभ किया, तभी से वह प्राकृतिक सुखों से वंचित होता गया। वह भूल गया कि मूक दिखाई देने वाली प्रकृति की वक्रदृष्टि सर्वनाश का कारण बन सकती है। 26 जुलाई की विभिषिणा ने हम मुंबई वासियों को आगाह किया है।

हमें इस बात का ध्यान रखना होगा कि आज हमारे परिवेश में पर्यावरण का संरक्षण निहायत जरूरी है। प्लास्टीक की। थैलिया हमारे स्वास्थ्य एवं पर्यावरण के लिए बेहद हानिकारक हैं क्योंकि 60 फीसदी प्लास्टीक ही रिसाइकिल हो पाती है।

प्लास्टीक का यह कचरा ज्यादातर नालियों और सीवेज को ठप्प कर देता है, शेष समुद्र पर होने वाले अतिक्रमण और वृक्षों की कटाई ने भी अपनी भूमिका अदा की है। जिसके कारण ही वर्षा का जल समुद्र की खाड़ी में नहीं जा पाता और जल जमाव से लोग त्रस्त होते हैं।

पर्यावरण की सुरक्षा से ही इस समस्या को सुलझाया जा सकता है। वन रोपण तथा वृक्ष लगाने से यह समस्या कम हो सकती है। जनसंख्या वृद्धि पर भी हमें अंकुश लगाना होगा। कंक्रीट के जंगल की सीमा बांधनी होगी। समुद्र के अतिक्रमण को रोकना होगा। वरना सुख देने वाली यह प्रकृति हमें गटक जाएगी।

26 जुलाई 2005 की वह कहर भरी शाम। समुद्री तूफान और बरसात का सिलसिला जो आरंभ हुआ, पूरी रात चलता रहा। हर गली पानी से भर गई। पहली मंजिल तक पानी पहुंचा, रेलवे प्लेट फार्म डूब गए, सड़कों पर पानी, गाड़ियों के ऊपर से पानी बह रहा था। सब तरफ अफरा-तफरी का माहौल।

सबकी सोच, कि अब क्या होगा? कैसे निपटा जाय। इस मुसीबत से लोगों ने हिम्मत नहीं हारी, पूरी रात कौन कहाँ रहा पता नहीं? मंदिरों, मस्जिदों, चर्चों के दरवाजे खुल गए। लोगों ने शरण ली। सबने जिसकी जितनी ताकत थी एक – दूसरे को सँभाला, हिम्मत बँधाए रखा। करोड़ों का नुकसान हुआ।

रेलवे, बस सबकी सेवाएं ठप्प हो गईं। वाह रे! हिम्मत चौबीस घंटे बाद धीरे-धीरे सब कुछ सामान्य होने लगा। हालात को सामान्य बनाने में सबका योगदान रहा। यह थी हमारी एकता वर्गगत, जातिगत, धर्मगत, दलगत, विचारों से ऊपर। सर्वधर्म समभाव का ऐसा उदाहरण जिसे हम आज भी नमन करते हैं।

Balbharti Maharashtra State Board Class 11 Maths Solutions Pdf Chapter 5 Straight Line Ex 5.3 Questions and Answers.

Maharashtra State Board 11th Maths Solutions Chapter 5 Straight Line Ex 5.3

Question 1.
Write the equation of the line:
i. parallel to the X-axis and at a distance of 5 units from it and above it.
ii. parallel to the Y-axis and at a distance of 5 units from it and to the left of it.
iii. parallel to the X-axis and at a distance of 4 units from the point (- 2,3).
Solution:
i. Equation of a line parallel to X-axis is y = k. Since the line is at a distance of 5 units above X-axis, k = 5
∴ The equation of the required line is y = 5.

ii. Equation of a line parallel to Y-axis is x = h. Since the line is at a distance of 5 units to the left of Y-axis, h = -5
∴ The equation of the required line is x = -5.
[Note: Answer given in the textbook is ‘y = -5
However, we found that ‘x = – 5’.]

iii. Equation of a line parallel to the X-axis is of the form y = k (k > 0 or k < 0).
Since the line is at a distance of 4 units from the point (- 2, 3),
k = 4 + 3 = 7 or k = 3- 4 = -1
∴ The equation of the required line is y = 1 or y = – 1.

Question 2.
Obtain the equation of the line:
i. parallel to the X-axis and making an intercept of 3 units on the Y-axis.
ii. parallel to the Y-axis and making an intercept of 4 units on the X-axis.
Solution:
i. Equation of a line parallel to X-axis with y-intercept ‘k’ isy = k.
Here, y-intercept = 3
∴ The equation of the required line is y = 3.

ii. Equation of a line parallel to Y-axis with x-intercept ‘h’ is x = h.
Here, x-intercept = 4
∴ The equation of the required line is x = 4.

Question 3.
Obtain the equation of the line containing the point:
i. A(2, – 3) and parallel to the Y-axis.
ii. B(4, – 3) and parallel to the X-axis.
Solution:
i. Equation of a line parallel to Y-axis is of the form x = h.
Since the line passes through A(2, – 3), h = 2
∴ The equation of the required line is x = 2.

ii. Equation of a line parallel to X-axis is of the formy = k.
Since the line passes through B(4, – 3), k = -3
∴ The equation of the required line is y = – 3.

Question 4.
Find the equation of the line:
i. passing through the points A(2, 0) and B(3,4)
ii. passing through the points P(2, 1) and Q(2,-1)
Solution:
i. The required line passes through the points A(2, 0) and B(3,4).
Equation of the line in two point form is \(\frac{y-y_{1}}{y_{2}-y_{1}}=\frac{x-x_{1}}{x_{2}-x_{1}}\)
Here, (x₁y₁) = (2,0) and (x₁,y₂) = (3,4)
∴ The equation of the required line is
∴ \(\frac{y-0}{4-0}=\frac{x-2}{3-2}\)
∴ \(\frac{y}{4}=\frac{x-2}{1}\)
∴ y = 4(x – 2)
∴ y = 4x – 8
∴ 4x – y – 8 = 0

ii. The required line passes through the points P(2, 1) and Q(2,-1).
Since both the given points have same
x co-ordinates i.e. 2,
the given points lie on the line x = 2.
∴ The equation of the required line is x = 2.

Question 5.
Find the equation of the line:
i. containing the origin and having inclination 60°.
ii. passing through the origin and parallel to AB, where A is (2,4) and B is (1,7).
iii. having slope 1/2 and containing the point (3, -2)
iv. containing the point A(3, 5) and having slope 2/3
v. containing the point A(4, 3) and having inclination 120°.
vi. passing through the origin and which bisects the portion of the line 3JC + y = 6 intercepted between the co-ordinate axes.
Solution:
i. Given, Inclination of line = θ = 60°
Slope of the line (m) = tan θ = tan 60°
= \(\sqrt{3}\)
Equation of the line having slope m and passing through origin (0, 0) is y = mx.
.‘. The equation of the required line is y = \(\sqrt{3}\) x

ii. Given, A (2, 4) and B (1, 7)
Slope of AB = \(\frac{7-4}{1-2}\) = -3 1-2
Since the required line is parallel to line AB, slope of required line (m) = slope of AB
∴ m = – 3 and the required line passes through the origin.
Equation of the line having slope m and passing through origin (0, 0) is y = mx.
∴ The equation of the required line is y = – 3x

iii. Given, slope(m) = \(=\frac{1}{2}\) and the line passes through (3, – 2).
Equation of the line in slope point form is
y-y ₁= m(x-x₁)
∴ The equation of the required line is
[y-(- 2)]=\(\frac{1}{2}\)(x-3)
∴ 2(y + 2)=x – 3
∴ 2y + 4 = x – 3
∴ x – 2y – 7 = 0

iv. Given, slope(m) = \(\frac{2}{3}\) and the line passes through (3, 5).
Equation of the line in slope point form is y-y₁ = m(x -x₁)
∴ The equation of the required line is y – 5 = \(\frac{2}{3}\)(x-3)
∴ 3 (y – 5) = 2 (x – 3)
∴ 3y – 15 = 2x – 6
∴ 2x – 3y + 9 = 0

v. Given, Inclination of line = θ = 120°
Slope of the line (m) = tan θ = tan 120°
= tan (90° + 30°)
= – cot 30°
= – \(\sqrt{3}\)
and the line passes through A(4, 3).
Equation of the line in slope point form is y-y₁ = m(x -x₁)
∴ The equation of the required line is
y- 3 = –\(\sqrt{3}\)(x-4)
∴ y – 3 = –\(\sqrt{3}\) x + 4\(\sqrt{3}\)
∴ \(\sqrt{3}\)x + y – 3 -4\(\sqrt{3}\) = 0

vi.

Given equation of the line is 3x +y = 6.
∴ \(\frac{x}{2}+\frac{y}{6}=1\)
This equation is of the form \(\frac{x}{\mathrm{a}}+\frac{y}{\mathrm{~b}}\) = 1,
where a = 2, b = 6
∴ The line 3x + y = 6 intersects the X-axis and Y-axis at A(2, 0) and B(0, 6) respectively. Required line is passing through the midpoint of AB.
∴ Midpoint of AB = ( \(\frac{2+0}{2}, \frac{0+6}{2}\) ) = (1,3)
∴ Required line passes through (0, 0) and (1,3).
Equation of the line in two point form is
\(\frac{y-y_{1}}{y_{2}-y_{1}}=\frac{x-x_{1}}{x_{2}-x_{1}}\)
∴ The equation of the required line is
\(\frac{y-0}{3-0}=\frac{x-0}{1-0}\)
\(\frac{y}{3}=\frac{x}{1}\)
∴ y = 3x
∴ 3x – y = 0

Alternate Method:
Given equation of the line is 3x + y = 6 …(i)
Substitute y = 0 in (i) to get a point on X-axis.
∴ 3x + 0 = 6
∴ x = 2
Substitute x = 0 in (i) to get a point on Y-axis.
∴ 3(0) + 7 = 6
∴ y = 6
∴ The line 3x + y = 6 intersects the X-axis and Y-axis at A(2,0) and B(0,6) respectively.
Let M be the midpoint of AB.
M = \(\left(\frac{2+0}{2}, \frac{0+6}{2}\right)\) = (1,3)
Slope of OM (m) = \(\frac{3-0}{1-0}\) = 3
Equation of OM is of the formy = mx.
∴ The equation of the required line is y = 3x
∴ 3x – y = 0

Question 6.
Line y = mx + c passes through the points A(2,1) and B(3,2). Determine m and c.
Solution:
Given, A(2, 1) and B(3,2)
Equation of the line in two point form is \(\frac{y-y_{1}}{y_{2}-y_{1}}=\frac{x-x_{1}}{x_{2}-x_{1}}\)
∴ The equation of the required line is
\(\frac{y-1}{2-1}=\frac{x-2}{3-2}\)
∴ \(\frac{y-1}{1}=\frac{x-2}{1}\)
∴ y – 1 = x – 2
∴ y = x – 1
Comparing this equation with y = mx + c, we get
m = 1 and c = – 1

Alternate Method:
Points A(2, 1) and B(3, 2) lie on the line y = mx + c.
∴ They must satisfy the equation.
∴ 2m + c = 1 …(i)
and 3m + c = 2 …(ii)
equation (ii) – equation (i) gives m = 1
Substituting m = 1 in (i), we get 2(1) + c = 1
∴ c = 1 – 2 = – 1

Question 7.
Find the equation of the line having inclination 135° and making x-intercept 7.
Solution:
Given, Inclination of line = 0 = 135°
∴ Slope of the line (m) = tan 0 = tan 135°
= tan (90° + 45°)
= – cot 45° = – 1 x-intercept of the required line is 7.
∴ The line passes through (7, 0).
Equation of the line in slope point form is y – y₁ = m(x – x₁)
∴ The equation of the required line is y — 0 = – 1 (x – 7)
∴ y = -x + 7
∴ x + y – 7 = 0

Question 8.
The vertices of a triangle are A(3, 4), B(2, 0) and C(- 1, 6). Find the equations of the lines containing
i. side BC
ii. the median AD
iii. the midpoints of sides AB and BC.
Solution:
Vertices of AABC are A(3, 4), B(2, 0) and C(- 1, 6).
i. Equation of the line in two point form is
\(\frac{y-y_{1}}{y_{2}-y_{1}}=\frac{x-x_{1}}{x_{2}-x_{1}}\)
∴ The equation of the side BC is
\(\frac{y-0}{6-0}=\frac{x-2}{-1-2}\)
\(\frac{y}{6}=\frac{x-2}{-3}\)
∴ – 3y = 6x – 12
∴ 6x + 3y – 12 = 0
∴ 2x + y – 4 = 0

ii. Let D be the midpoint of side BC.
Then, AD is the median through A.
∴ D = \(\left(\frac{2-1}{2}, \frac{0+6}{2}\right)=\left(\frac{1}{2}, 3\right)\)
The median AD passes through the points
A(3,4) and D( \(\frac{1}{2}\) , 3)

∴ The equation of the median AD is
\(\frac{y-4}{3-4}=\frac{x-3}{\frac{1}{2}-3}\)
\(\frac{y-4}{-1}=\frac{x-3}{-\frac{5}{2}}\)
\(\frac{5}{2}\)(y-4) = x – 3
∴ 5y – 20 = 2x – 6
∴ 2x – 5y + 14 = 0

iii. Let D and E be the midpoints of side AB and side BC respectively.
The equation of the line DE is

∴ -4(y-2) = 2x-5
∴ 2x + 4y – 13 = 0

Question 9.
Find the x and y-intercepts of the following lines:
i. \(\frac{x}{3}+\frac{y}{2}=1\)
ii. \(\frac{3 x}{2}+\frac{2 y}{3}=1\)
iii. 2x – 3y + 12 = 0
Solution:
i. Given equation of the line is latex]\frac{x}{3}+\frac{y}{2}=1[/latex]
This is of the form \(\frac{x}{a}+\frac{y}{b}\) = 1,
where x-intercept = a, y-intercept = b
∴ x-intercept = 3, y-intercept = 2

ii. Given equation of the line is \(\frac{3 x}{2}+\frac{2 y}{3}\) = 1
∴ \(\frac{x}{\left(\frac{2}{3}\right)}+\frac{y}{\left(\frac{3}{2}\right)}\) = 1
This is of the form = \(\frac{x}{a}+\frac{y}{b}\) = 1,
where x-intercept = a, y-intercept = b
∴ x-intercept = \(\frac{2}{3}\) and y-intercept = \(\frac{3}{2}\)

iii. Given equation of the line is 2x – 3y + 12 = 0
∴ 2x – 3y = – 12
∴ \(\frac{2 x}{(-12)}-\frac{3 y}{(-12)}=1\)
∴ \(\frac{x}{-6}+\frac{y}{4}=1\)
This is of the form \(\) = 1,
where x-intercept = a, y-intercept = b
∴ x-intercept = – 6 and y-intercept = 4

Question 10.
Find equations of the line which contains the point A(l, 3) and the sum of whose intercepts on the co-ordinate axes is zero.
Solution:
Case I: Line not passing through origin.
Let the equation of the line be
\(\frac{x}{a}+\frac{y}{b}=1\) ………..(i)
Since, the sum of the intercepts of the line is zero.
∴ a + b = 0
∴ b = – a
Substituting b = – a in (i), we get
\(\frac{x}{a}+\frac{y}{(-a)}=1\)
x – y = a .. .(ii)
Since, the line passes through A(1, 3).
∴ 1 – 3 = a
∴ a = – 2
Substituting the value of a in (ii), equation of the required line is
∴ x – y = – 2,
∴ x – y + 2 = 0

Case II: Line passing through origin.
Slope of line passing through origin and
A(1, 3) is m = \(\frac{3-0}{1-0}\) = 3
∴ Equation of the line having slope m and passing through origin (0, 0) is / = mx.
∴ The equation of the required line is y = 3x
∴ 3x – y = 0

Question 11.
Find equations of the line containing the point A(3, 4) and making equal intercepts on the co-ordinate axes.
Solution:
Case I: Line not passing through origin.
Let the equation of the line be \(\frac{x}{a}+\frac{y}{b}=1\) …………(i)
This line passes through A(3, 4).
∴ \(\frac{3}{a}+\frac{4}{b}=1\)……………..(ii)
Since, the required line make equal intercepts on the co-ordinate axes.
∴ a = b …(iii)
Substituting the value of b in (ii), we get
\(\frac{3}{a}+\frac{4}{a}=1\)
∴ \(\frac{7}{a}=1\)
∴ a = 7
∴ b = 7 …[From (iii)]
Substituting the values of a and b in (i), equation of the required line is
\(\frac{x}{7}+\frac{y}{7}=1\) = 1
∴ x + y = 7

Case II: Line passing through origin.
Slope of line passing through origin and A(3,4) is m = \(=\frac{4-0}{3-0}=\frac{4}{3}\)
∴ Equation of the line having slope m and passing through origin (0, 0) is y = mx.
∴ The equation of the required line is 4
y = \(\frac{4}{3}\)x
∴ 4x – 3y = 0

Question 12.
Find the equations of the altitudes of the triangle whose vertices are A(2, 5), B(6, – 1 ) and C(- 4, – 3).
Solution:

A(2, 5), B(6, – 1), C(- 4, – 3) are the vertices of ∆ABC.
Let AD, BE and CF be the altitudes through the vertices A, B and C respectively of ∆ABC.
∴ Slope of AD = -5 …[∵AD ⊥ BC]
Since altitude AD passes through (2, 5) and has slope – 5,
equation of the altitude AD is y – 5 = -5 (x – 2)
∴ y – 5 = – 5x + 10
∴ 5x +y -15 = 0
Now, slope of AC = \(\frac{-3-5}{-4-2}=\frac{-8}{-6}=\frac{4}{3}\)
Slope of BE = \(\frac{-3}{4}\)
…[∵ BE ⊥ AC]
Since altitude BE passes through (6,-1) and has slope \(\frac{-3}{4}\),
equation of the altitude BE is
y-(-1) = \(\frac{-3}{4}\) (x – 6)
∴ 4 (y + 1) = – 3 (x – 6)
∴ 4y + 4 =-3x+ 18
∴ 3x + 4y – 14 = 0
Also, slope of AB = \(\frac{-1-5}{6-2}=\frac{-6}{4}=\frac{-3}{2}\)
∴ Slope of CF = \({2}{3}\) ….[∵ CF ⊥ AB]
Since altitude CF passes through (- 4, – 3) and has slope , \(\frac{2}{3}\)
equation of the altitude CF is
y-(-3) = \(\frac{2}{3}\)[x-(-4)]
∴ 3 (y + 3) = 2 (x + 4)
∴ 3y + 9 = 2x + 8
∴ 2x – 3y – 1 = 0

Question 13.
Find the equations of perpendicular bisectors of sides of the triangle whose vertices are P(-1, 8), Q(4, – 2) and R(- 5, – 3).
Solution:

Let A, B and C be the midpoints of sides PQ, QR and PR respectively of APQR.
A is the midpoint of side PQ.

Slope of perpendicular bisector of PQ is \(\frac{1}{2}\) and it passes through (\(\frac{3}{2}\)), 3).
Equation of the perpendicular bisector of side PQ is
y – 3 = \(\frac{1}{2}\)(x – \(\frac{3}{2}\))
y – 3 = (\(\frac{1}{2}\left(\frac{2 x-3}{2}\right)\))
∴ 4(y – 3) = 2x – 3
∴ 4y – 12 = 2x – 3
∴ 2x – 4y + 9 = 0
B is the midpoint of side QR
∴ B = \(\left(\frac{4-5}{2}, \frac{-2-3}{2}\right)=\left(\frac{-1}{2}, \frac{-5}{2}\right)\)
Slope of side QR = \(\frac{-3-(-2)}{-5-4}=\frac{-1}{-9}=\frac{1}{9}\)
∴ Slope of perpendicular bisector of QR is -9 and it passes through \(\left(-\frac{1}{2},-\frac{5}{2}\right)\)
∴ Equation of the perpendicular bisector of side QR is

∴ 2y + 5 = -18x – 9
∴ 18x + 2y + 14 = 0
∴ 9x + y + 7 = 0
C is the midpoint of side PR.

Equation of the perpendicular bisector of PR is \(y-\frac{5}{2}=-\frac{4}{11}(x+3)\)
∴ \(11\left(\frac{2 y-5}{2}\right)\) =-4(x + 3)
∴ 11(2y – 5) = – 8 (x + 3)
∴ 22y – 55 = – 8x – 24
∴ 8x + 22y -31 = 0

Question 14.
Find the co-ordinates of the orthocentre of the triangle whose vertices are A(2, – 2), B(l, 1) and C(-1,0).
Solution:
Let O be the orthocentre of AABC.
Let AM and BN be the altitudes of sides BC and AC respectively.
Now, slope of BC = \(\frac{0-1}{-1-1}=\frac{-1}{-2}=\frac{1}{2}\)
Slope of AM = -2 ,..[∵ AM ⊥ BC]
Since AM passes through (2, – 2) and has slope -2,
equation of the altitude AM is y – (- 2) = – 2 (x – 2)
∴ y + 2 = -2x + 4
∴ 2x + y – 2 = 0 …(i)

Also, slope of AC = \(\frac{0-(-2)}{-1-2}=\frac{2}{-3}\)
∴ Slope of BN = \(\frac{3}{2}\) …[∵ BN ⊥ AC]
Since BN passes through (1,1) and has slope \(\frac{3}{2}\), equation of the altitude BN is
y – 1 = \(\frac{3}{2}\)(x-1)
∴ 2y – 2 = 3x – 3
∴ 3x – 2y – 1 = 0 …(ii)
To find co-ordinates of orthocentre, we have to solve equations (i) and (ii).
By (i) x 2 + (ii), we get
7x – 5 = 0
∴ x = \(\frac{5}{7}\)
substituting x = \(\frac{5}{7}\) in eq (i), we get
2(\(\frac{5}{7}\)) + y – 2 = 0
∴ y = -2(\(\frac{5}{7}\)) + 2
∴ y = \(\frac{-10+14}{7}=\frac{4}{7}\)
∴ Coordinates of orthocentre O = \(\left(\frac{5}{7}, \frac{4}{7}\right)\)

Question 15.
N(3, – 4) is the foot of the perpendicular drawn from the origin to line L. Find the equation of line L.
Solution:

Slope of ON = \(\frac{-4-0}{3-0}=\frac{-4}{3}\)
Since line L ⊥ ON,
slope of the line L is \(\frac{3}{4}\) and it passes through point N(3, -4).
Equation of the line in slope point form is y – y₁ = m(x – x₁)
Equation of line L is
y-(-4) = \(\frac{3}{4}\)(x-3)
∴ 4(y + 4) = 3(x – 3)
∴ 4y + 16 = 3x – 9
∴ 3x – 4y – 25 = 0