Class 11

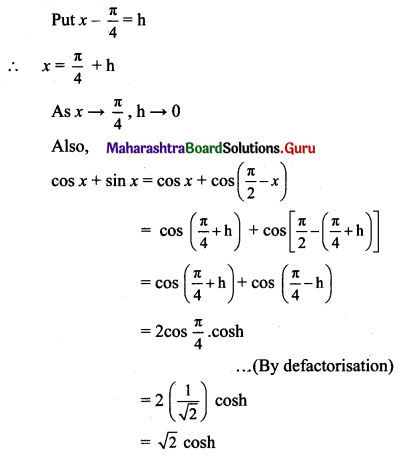
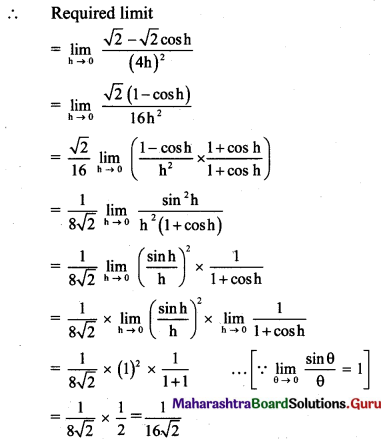
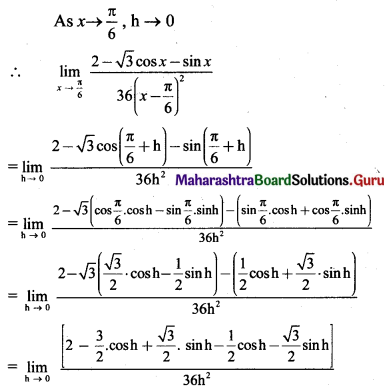
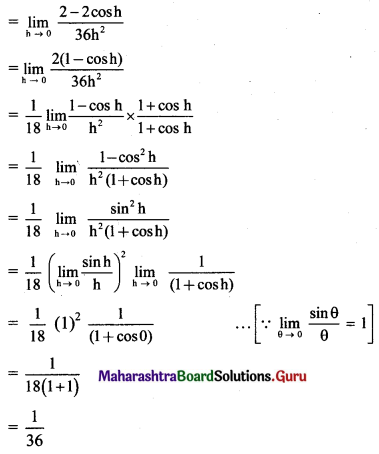
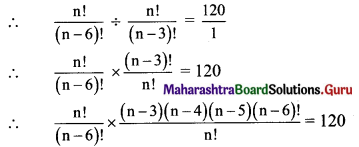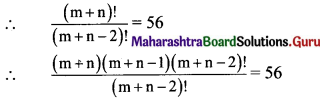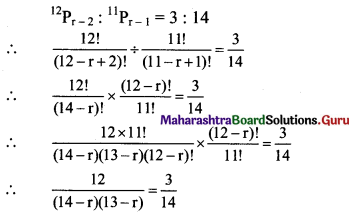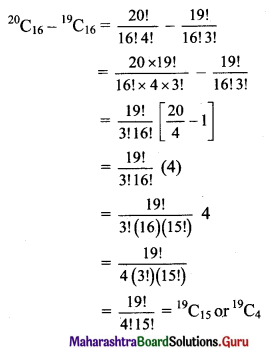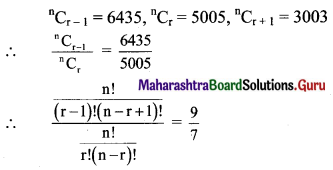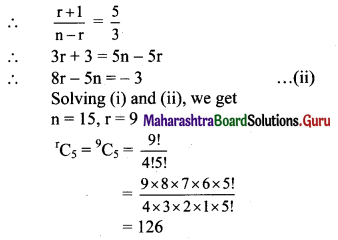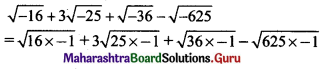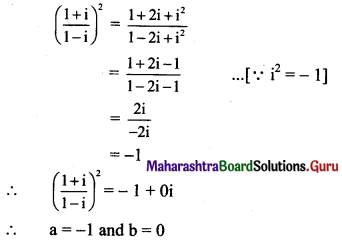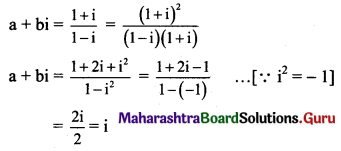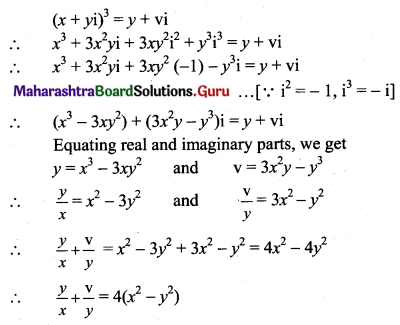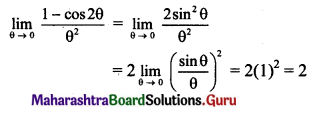Balbharti Maharashtra State Board 11th Maths Book Solutions Pdf Chapter 1 Complex Numbers Miscellaneous Exercise 1 Questions and Answers.

Maharashtra State Board 11th Maths Solutions Chapter 1 Complex Numbers Miscellaneous Exercise 1

(I) Select the correct answer from the given alternatives.

Question 1.
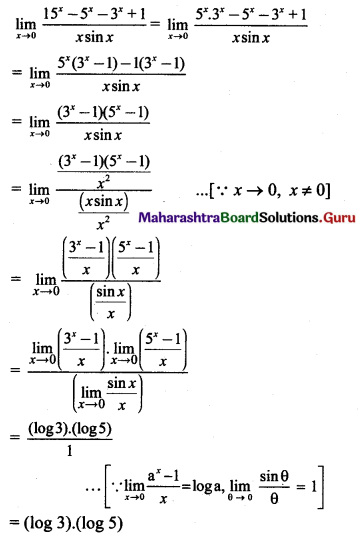
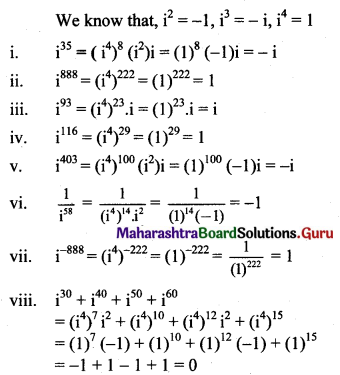
If n is an odd positive integer, then the value of 1 + (i)²ⁿ + (i)⁴ⁿ + (i)⁶ⁿ is:
(A) -4i
(B) 0
(C) 4i
(D) 4
Answer:
(B) 0
Hint:
1 + (i²)ⁿ + (i⁴)ⁿ + (i²)³ⁿ
= 1 – 1 + 1 – 1 …..(n odd positive integer)
= 0

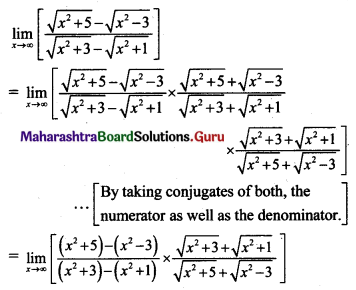
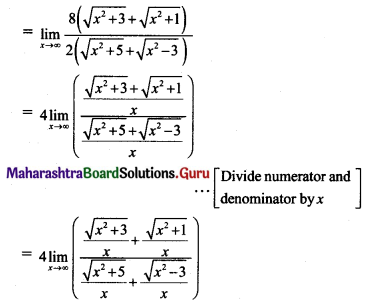
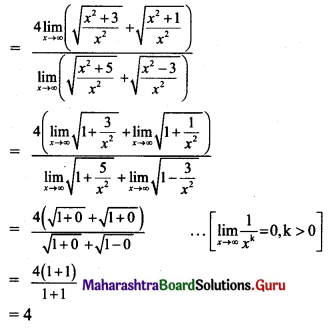
Question 2.
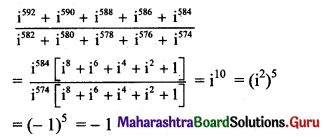
The value of \(\frac{i^{592}+i^{590}+i^{588}+i^{586}+i^{584}}{i^{582}+i^{580}+i^{578}+i^{576}+i^{574}}\) is equal to:
(A) -2
(B) 1
(C) 0
(D) -1
Answer:
(D) -1
Hint:

Question 3.
√-3 √-6 is equal to
(A) -3√2
(B) 3√2
(C) 3√2 i
(D) -3√2 i
Answer:
(A) -3√2
Hint:
√-3 √-6
= (√3 i) (√6 i)
= 3√2 (-1)
= -3√2

Question 4.
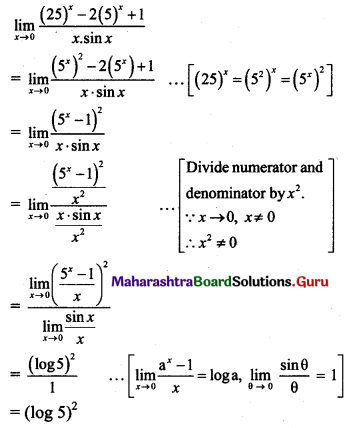
If ω is a complex cube root of unity, then the value of ω⁹⁹ + ω¹⁰⁰ + ω¹⁰¹ is:
(A) -1
(B) 1
(C) 0
(D) 3
Answer:
(C) 0
Hint:
ω⁹⁹ + ω¹⁰⁰ + ω¹⁰¹
= ω⁹⁹ (1 + ω + ω²)
= ω⁹⁹ (0)
= 0

Question 5.
If z = r(cos θ + i sin θ), then the value of \(\frac{z}{\bar{z}}+\frac{\bar{z}}{z}\) is
(A) cos 2θ
(B) 2cos 2θ
(C) 2cos θ
(D) 2sin θ
Answer:
(B) 2cos 2θ
Hint:

Question 6.
If ω(≠1) is a cube root of unity and (1 + ω)⁷ = A + Bω, then A and B are respectively the numbers
(A) 0, 1
(B) 1, 1
(C) 1, 0
(D) -1, 1
Answer:
(B) 1, 1
Hint:
(1 + ω)⁷
= (-ω²)⁷
= -ω¹⁴
= -ω²(ω³)⁴
= -ω²
= 1 + ω
A = 1, B = 1

Question 7.
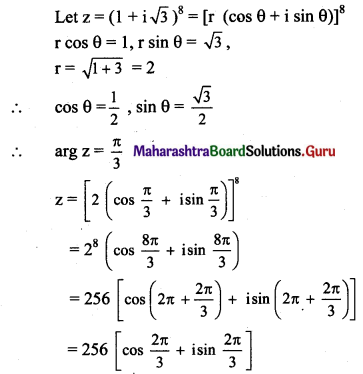
The modulus and argument of (1 + i√3)⁸ are respectively
(A) 2 and \(\frac{2 \pi}{3}\)
(B) 256 and \(\frac{8 \pi}{3}\)
(C) 256 and \(\frac{2 \pi}{3}\)
(D) 64 and \(\frac{4 \pi}{3}\)
Answer:
(C) 256 and \(\frac{2 \pi}{3}\)
Hint:

Question 8.
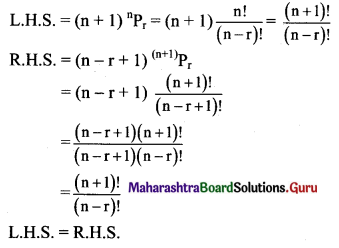
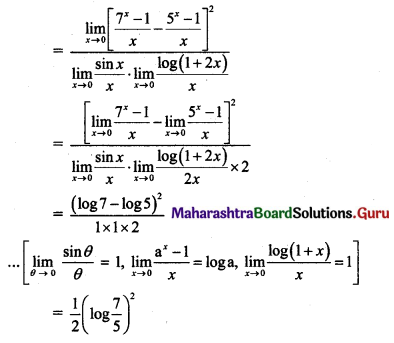
If arg (z) = θ, then arg \(\overline{(\mathrm{z})}\) =
(A) -θ
(B) θ
(C) π – θ
(D) π + θ
Answer:
(A) -θ
Hint:
Let z = \(\mathrm{re}^{\mathrm{i} \theta}\), then \(\overline{\mathrm{z}}=\mathrm{r} \mathrm{e}^{-\mathrm{i} \theta}\)
∴ arg \(\overline{\mathbf{z}}\) = -θ.

Question 9.
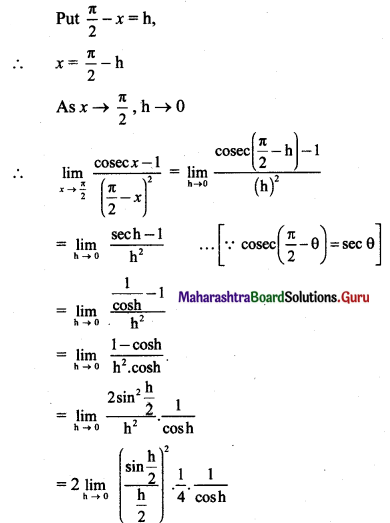
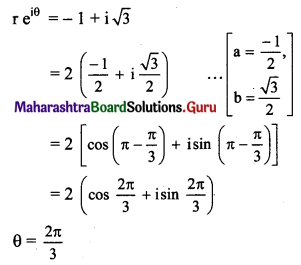
If -1 + √3 i = \(\mathrm{re}^{\mathrm{i} \theta}\), then θ =
(A) –\(\frac{2 \pi}{3}\)
(B) \(\frac{\pi}{3}\)
(C) –\(\frac{\pi}{3}\)
(D) \(\frac{2 \pi}{3}\)
Answer:
(D) \(\frac{2 \pi}{3}\)
Hint:

Question 10.
If z = x + iy and |z – zi| = 1, then
(A) z lies on X-axis
(B) z lies on Y-axis
(C) z lies on a rectangle
(D) z lies on a circle
Answer:
(D) z lies on a circle
Hint:
|z – zi | = |z| |1 – i| = 1
∴ |z| = \(\frac{1}{\sqrt{2}}\)
∴ x² + y² = \(\frac{1}{2}\)

(II) Answer the following:

Question 1.
Simplify the following and express in the form a + ib.
(i) 3 + √-64
Solution:
3 + √-64
= 3 + √64 √-1
= 3 + 8i

(ii) (2i³)²
Solution:
(2i³)²
= 4i⁶
= 4(i²)³
= 4(-1)³
= -4 …..[∵ i² = -1]
= -4 + 0i

(iii) (2 + 3i) (1 – 4i)
Solution:
(2 + 3i)(1 – 4i)
= 2 – 8i + 3i – 12i²
= 2 – 5i – 12(-1) …..[∵ i² = -1]
= 14 – 5i

(iv) \(\frac{5}{2}\)i(-4 – 3i)
Solution:

(v) (1 + 3i)² (3 + i)
Solution:
(1 + 3i)² (3 + i)
= (1 + 6i + 9i²)(3 + i)
= (1 + 6i – 9)(3 + i) ……[∵ i² = -1]
= (-8 + 6i)(3 + i)
= -24 – 8i + 18i + 6i²
= -24 + 10i + 6(-1)
= -24 + 10i – 6
= -30 + 10i

(vi) \(\frac{4+3 i}{1-i}\)
Solution:

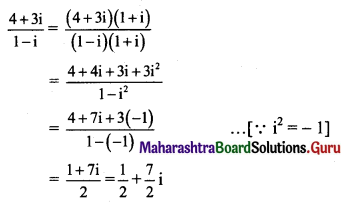
(vii) \(\left(1+\frac{2}{i}\right)\left(3+\frac{4}{i}\right)(5+i)^{-1}\)
Solution:

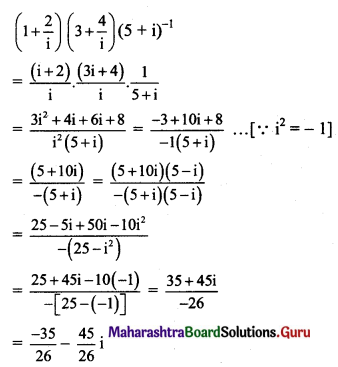
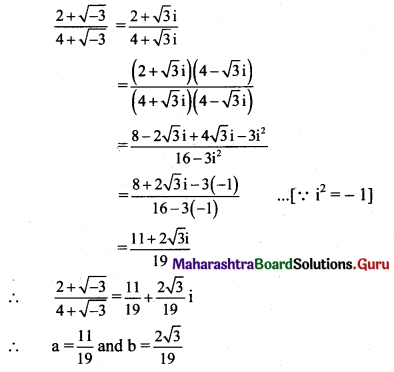
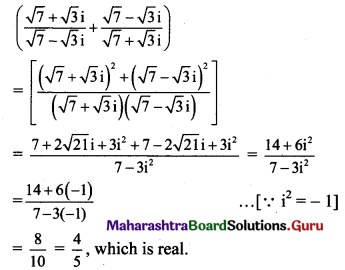
(viii) \(\frac{\sqrt{5}+\sqrt{3 i}}{\sqrt{5}-\sqrt{3} i}\)
Solution:

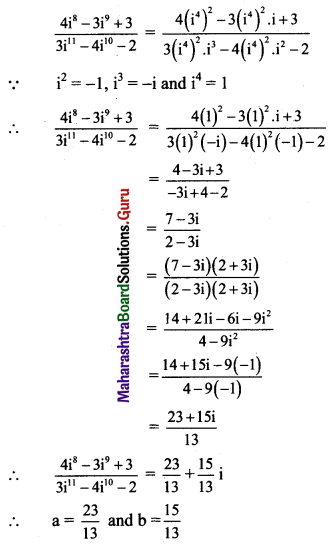
(ix) \(\frac{3 i^{5}+2 i^{7}+i^{9}}{i^{6}+2 i^{8}+3 i^{18}}\)
Solution:

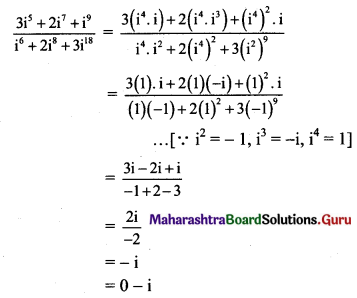
(x) \(\frac{5+7 i}{4+3 i}+\frac{5+7 i}{4-3 i}\)
Solution:

Question 2.
Solve the following equations for x, y ∈ R
(i) (4 – 5i)x + (2 + 3i)y = 10 – 7i
Solution:
(4 – 5i)x + (2 + 3i)y = 10 – 7i
(4x + 2y) + (3y – 5x) i = 10 – 7i
Equating real and imaginary parts, we get
4x + 2y= 10 i.e., 2x + y = 5 ……(i)
and 3y – 5x = -7 ……(ii)
Equation (i) × 3 – equation (ii) gives
11x = 22
∴ x = 2
Putting x = 2 in (i), we get
2(2) + y = 5
∴ y = 1
∴ x = 2 and y = 1

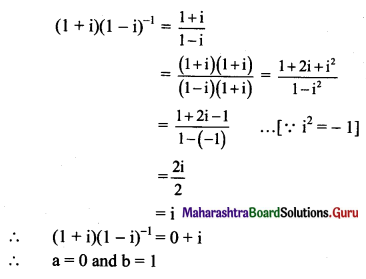
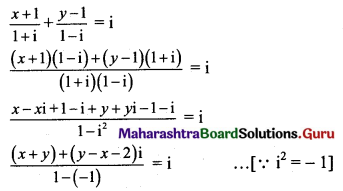
(ii) \(\frac{x+i y}{2+3 i}\) = 7 – i
Solution:
\(\frac{x+i y}{2+3 i}\) = 7 – i
x + iy = (7 – i)(2 + 3i)
x + iy = 14 + 21i – 2i – 3i²
x + iy = 14 + 19i – 3(-1)
x + iy = 17 + 19i
Equating real and imaginary parts, we get
∴ x = 17 and y = 19

(iii) (x + iy) (5 + 6i) = 2 + 3i
Solution:

(iv) 2x + i⁹ y(2 + i) = x i⁷ + 10 i¹⁶
Solution:
2x + i⁹ y(2 + i) = x i⁷ + 10 i¹⁶
2x + (i⁴)² . i . y(2 + i) = x(i²)³ . i + 10 . (i⁴)⁴
2x + (1)² . iy(2 + i) = x(-1)³ . i + 10(1)⁴ ……..[∵ i² = -1, i⁴ = 1]
2x + 2yi + y i² = -xi + 10
2x + 2yi – y + xi = 10
(2x – y) + (x + 2y)i = 10 + 0 . i
Equating real and imaginary parts, we get
2x – y = 10 ……(i)
and x + 2y = 0 ……..(ii)
Equation (i) × 2 + equation (ii) gives, we get
5x = 20
∴ x = 4
Putting x = 4 in (i), we get
2(4) – y = 10
y = 8 – 10
∴ y = -2
∴ x = 4 and y = -2

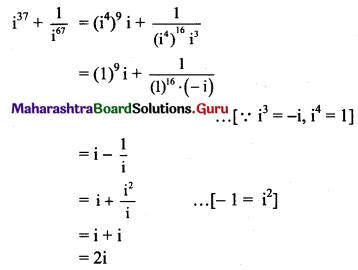
Question 3.
Evaluate
(i) (1 – i + i²)^-15
Solution:

(ii) i¹³¹ + i⁴⁹
Solution:
i¹³¹ + i⁴⁹
= (i⁴)³² . i³ + (i⁴)¹² . i
= (1)³² (-i) + (1)¹² . i
= -i + i
= 0

Question 4.
Find the value of
(i) x³ + 2x² – 3x + 21, if x = 1 + 2i
Solution:

(ii) x⁴ + 9x³ + 35x² – x + 164, if x = -5 + 4i
Solution:

Question 5.
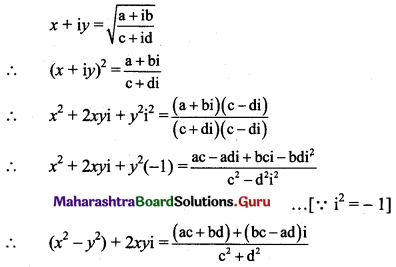
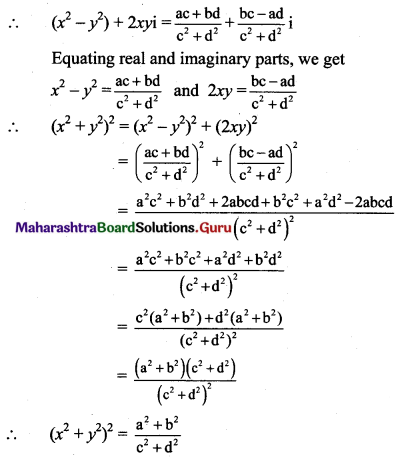
Find the square roots of
(i) -16 + 30i
Solution:
Let \(\sqrt{-16+30 \mathrm{i}}\) = a + bi, where a, b ∈ R.
Squaring on both sides, we get
-16 + 30i = a² + b² i² + 2abi
-16 + 30i = (a² – b²) + 2abi …..[∵ i² = -1]
Equating real and imaginary parts, we get
a² – b² = -16 and 2ab = 30
a² – b² = -16 and b = \(\frac{15}{a}\)

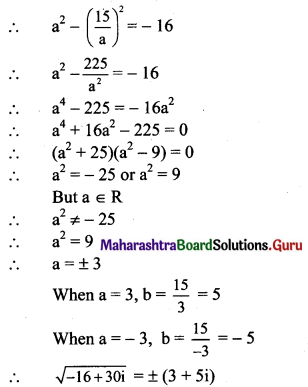
(ii) 15 – 8i
Solution:
Let \(\sqrt{15-8 i}\) = a + bi, where a, b ∈ R.
Squaring on both sides, we get
15 – 8i = a² + b² i² + 2abi
15 – 8i = (a² – b²) + 2abi …..[∵ i² = -1]
Equating real and imaginary parts, we get
a² – b² = 15 and 2ab = -8
a² – b² = 15 and b = \(\frac{-4}{a}\)

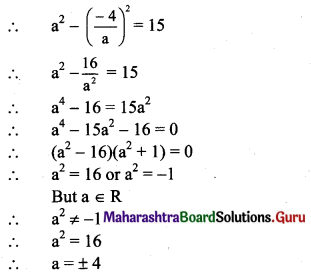
When a = 4, b = \(\frac{-4}{4}\) = -1
When a = -4, b = \(\frac{-4}{-4}\) = 1
∴ \(\sqrt{15-8 i}\) = ±(4 – i)

(iii) 2 + 2√3 i
Solution:
Let \(\sqrt{2+2 \sqrt{3}}\) = a + bi, where a, b ∈ R.
Squaring on both sides, we get
2 + 2√3 i = a² + b² i² + 2abi
2 + 2√3 i = a² – b² + 2abi …..[∵ i² = -1]
Equating real and imaginary parts, we get
a² – b² = 2 and 2ab = 2√3
a² – b² = 2 and b = \(\frac{\sqrt{3}}{a}\)

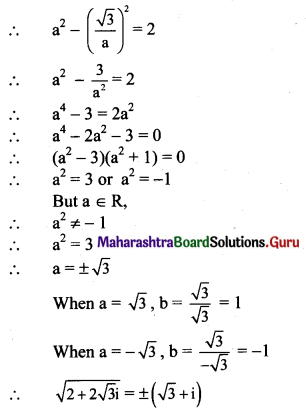
(iv) 18i
Solution:
Let √18i = a + bi, where a, b ∈ R.
Squaring on both sides, we get
18i = a² + b² i² + 2abi
0 + 18i = a² – b² + 2abi …..[∵ i² = -1]
Equating real and imaginary parts, we get
a² – b² = 0 and 2ab = 18
a² – b² = 0 and b = \(\frac{9}{a}\)
\(a^{2}-\left(\frac{9}{a}\right)^{2}=0\)
\(a^{2}-\frac{81}{a^{2}}=0\)
a⁴ – 81 = 0
(a² – 9) (a² + 9) = 0
a² = 9 or a² = -9
But a ∈ R
∴ a² ≠ -9
∴ a² = 9
∴ a = ± 3
When a = 3, b = \(\frac{9}{3}\) = 3
When a = -3, b = \(\frac{9}{-3}\) = -3
∴ √18i = ±(3 + 3i) = ±3(1 + i)

(v) 3 – 4i
Solution:
Let \(\sqrt{3-4 i}\) = a + bi, where a, b ∈ R.
Squaring on both sides, we get
3 – 4i = a² + b² i² + 2abi
3 – 4i = a² – b² + 2abi ……[∵ i² = -1]
Equating real and imaginary parts, we get
a² – b² = 3 and 2ab = -4
a² – b² = 3 and b = \(\frac{-2}{a}\)

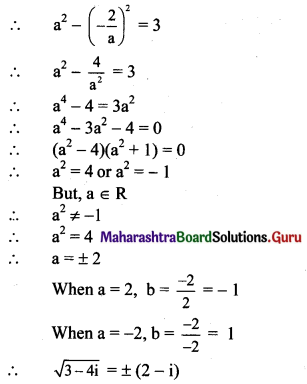
(vi) 6 + 8i
Solution:
Let \(\sqrt{6+8 i}\) = a + bi, where a, b ∈ R.
Squaring on both sides, we get
6 + 8i = a² + b² i² + 2abi
6 + 8i = a² – b² + 2abi ……[∵ i² = -1]
Equating real and imaginary parts, we get
a² – b² = 6 and 2ab = 8

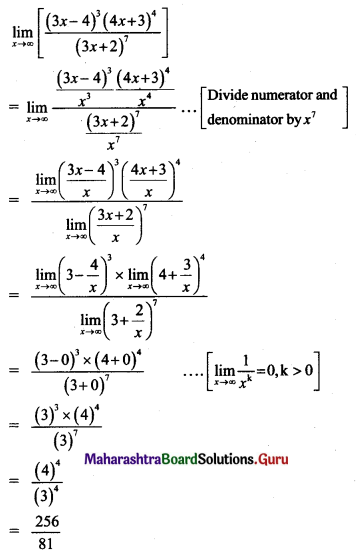
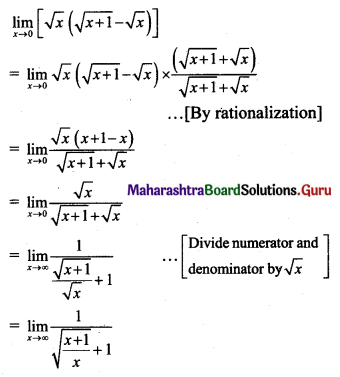
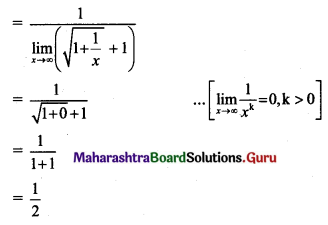
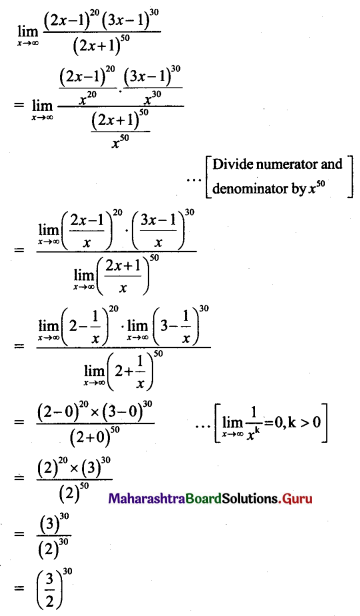
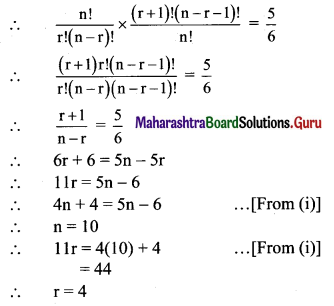
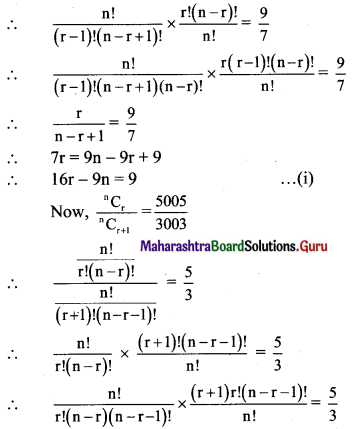
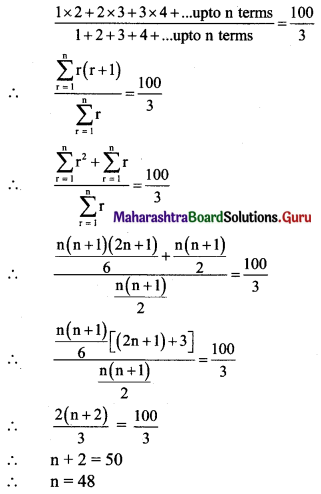
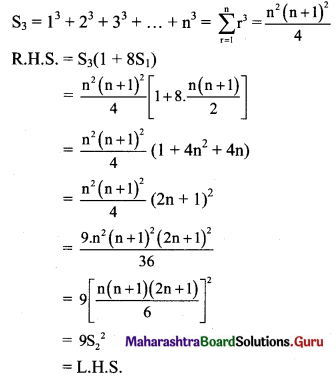
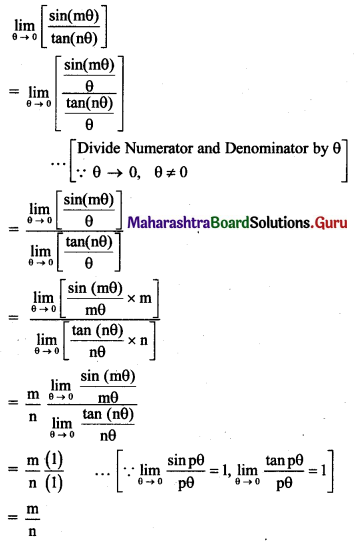
Question 6.
Find the modulus and argument of each complex number and express it in the polar form.
(i) 8 + 15i
Solution:

(ii) 6 – i
Solution:

(iii) \(\frac{1+\sqrt{3} \mathbf{i}}{2}\)
Solution:

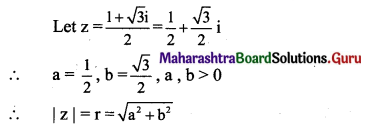
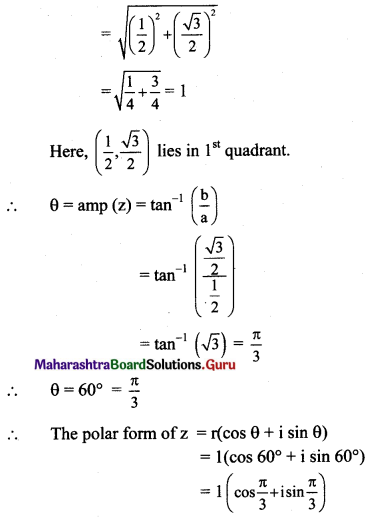
(iv) \(\frac{-1-\mathbf{i}}{\sqrt{2}}\)
Solution:

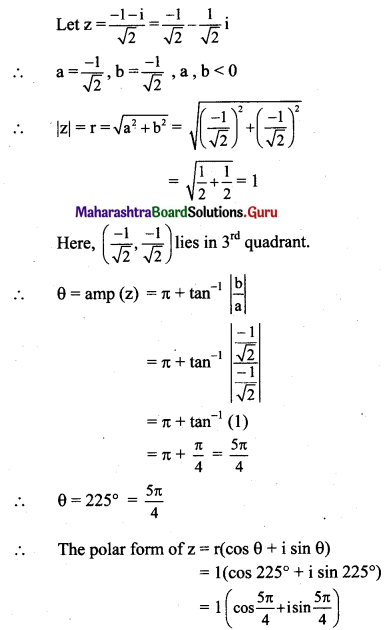
(v) 2i
Solution:

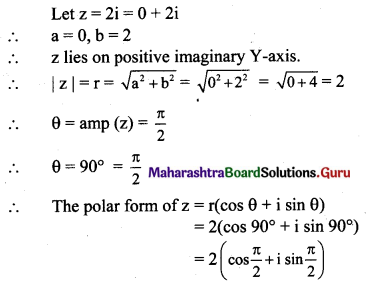
(vi) -3i
Solution:

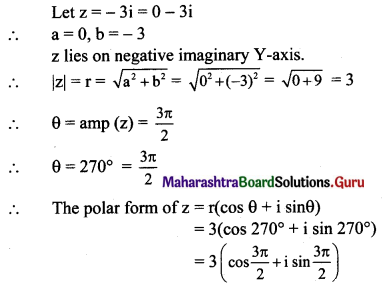
(vii) \(\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}} \mathbf{i}\)
Solution:

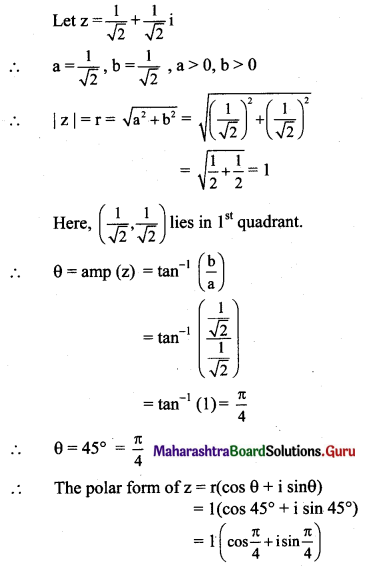
Question 7.
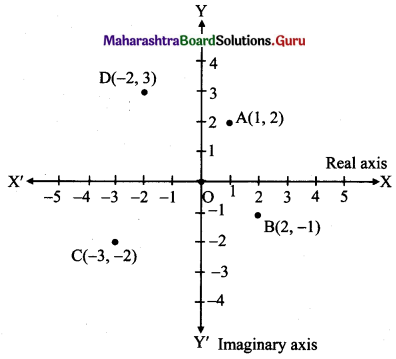
Represent 1 + 21, 2 – i, -3 – 2i, -2 + 3i by points in Argand’s diagram.
Solution:
The complex numbers 1 + 2i, 2 – i, -3 – 2i, -2 + 3i will be represented by the points A(1, 2), B(2, -1), C(-3, -2), D(-2, 3) respectively as shown below:

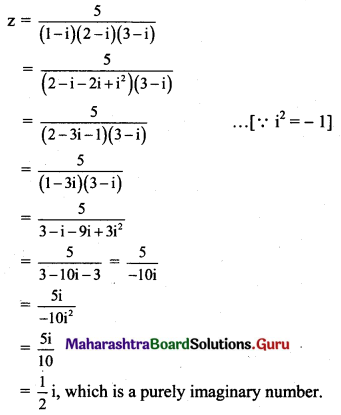
Question 8.
Show that z = \(\frac{5}{(1-i)(2-i)(3-i)}\) is purely imaginary number.
Solution:

Question 9.
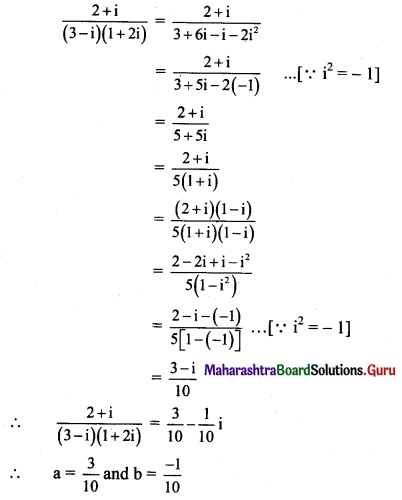
Find the real numbers x and y such that \(\frac{x}{1+2 i}+\frac{y}{3+2 i}=\frac{5+6 i}{-1+8 i}\)
Solution:
\(\frac{x}{1+2 i}+\frac{y}{3+2 i}=\frac{5+6 i}{-1+8 i}\)

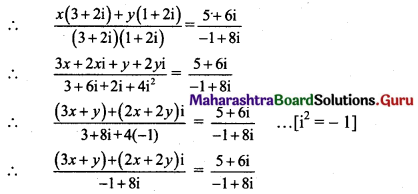
(3x + y) + 2(x + y)i = 5 + 6i
Equating real and imaginary parts, we get
3x + y = 5 ……(i)
and 2(x + y) = 6
i.e., x + y = 3 …….(ii)
Subtracting (ii) from (i), we get
2x = 2
∴ x = 1
Putting x = 1 in (ii), we get
1 + y = 3
∴ y = 2
∴ x = 1, y = 2

Question 10.
Show that \(\left(\frac{1}{\sqrt{2}}+\frac{i}{\sqrt{2}}\right)^{10}+\left(\frac{1}{\sqrt{2}}-\frac{i}{\sqrt{2}}\right)^{10}=0\)
Solution:

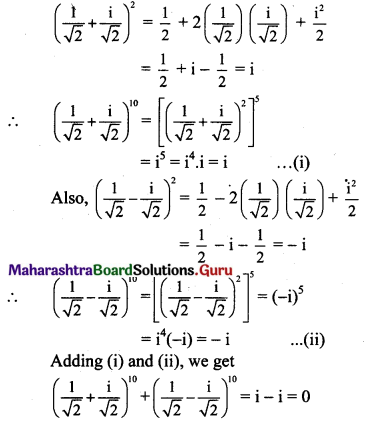
Question 11.
Show that \(\left(\frac{1+i}{\sqrt{2}}\right)^{8}+\left(\frac{1-i}{\sqrt{2}}\right)^{8}=2\)
Solution:

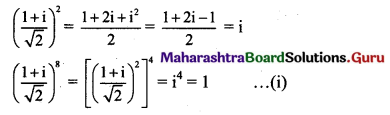
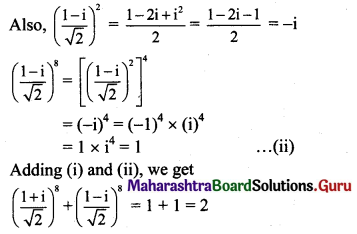
Question 12.
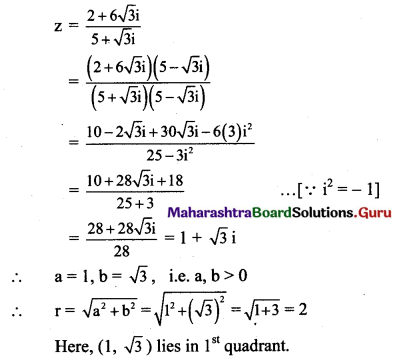
Convert the complex numbers in polar form and also in exponential form.
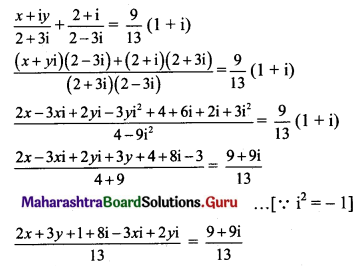
(i) z = \(\frac{2+6 \sqrt{3} i}{5+\sqrt{3} i}\)
Solution:

(ii) z = -6 + √2 i
Solution:
z = -6 + √2 i
∴ a = -6, b = √2
i.e. a < 0, b > 0

(iii) \(\frac{-3}{2}+\frac{3 \sqrt{3} i}{2}\)
Solution:

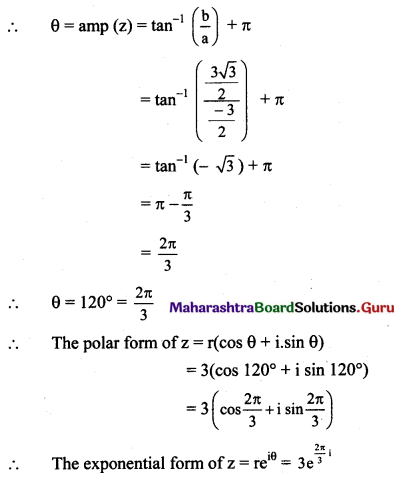
Question 13.
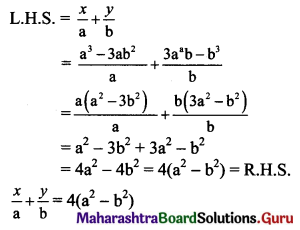
If x + iy = \(\frac{a+i b}{a-i b}\), prove that x² + y² = 1.
Solution:

Question 14.
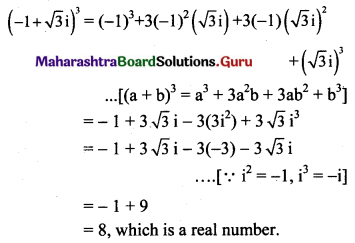
Show that z = \(\left(\frac{-1+\sqrt{-3}}{2}\right)^{3}\) is a rational number.
Solution:

Question 15.
Show that \(\frac{1-2 i}{3-4 i}+\frac{1+2 i}{3+4 i}\) is real.
Solution:

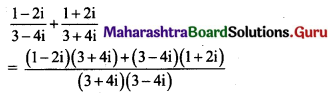
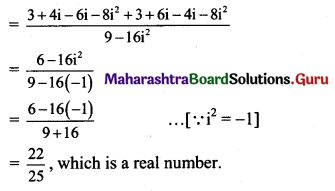
Question 16.
Simplify
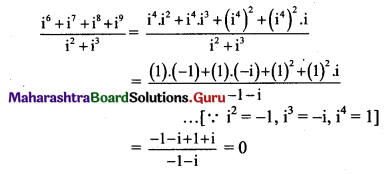
(i) \(\frac{\mathrm{i}^{29}+\mathrm{i}^{39}+\mathrm{i}^{49}}{\mathrm{i}^{30}+\mathrm{i}^{40}+\mathrm{i}^{50}}\)
Solution:

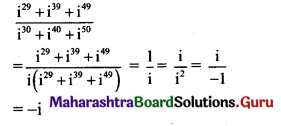
(ii) \(\left(\mathrm{i}^{65}+\frac{1}{\mathrm{i}^{145}}\right)\)
Solution:

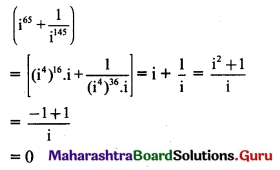
(iii) \(\frac{\mathrm{i}^{238}+\mathrm{i}^{236}+\mathrm{i}^{234}+\mathrm{i}^{232}+\mathrm{i}^{230}}{\mathrm{i}^{228}+\mathrm{i}^{226}+\mathrm{i}^{224}+\mathrm{i}^{222}+\mathrm{i}^{220}}\)
Solution:

Question 17.
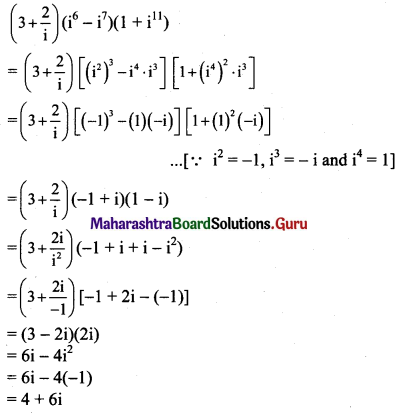
Simplify \(\left[\frac{1}{1-2 i}+\frac{3}{1+i}\right]\left[\frac{3+4 i}{2-4 i}\right]\)
Solution:

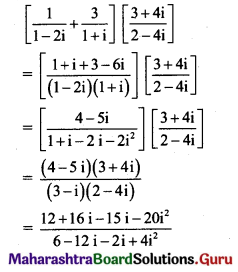
Question 18.
If α and β are complex cube roots of unity, prove that (1 – α) (1 – β) (1 – α²) (1 – β²) = 9.
Solution:
α and β are the complex cube roots of unity.

Question 19.
If ω is a complex cube root of unity, prove that (1 – ω + ω²)⁶ + (1 + ω – ω²)⁶ = 128.
Solution:
ω is the complex cube root of unity.
∴ ω³ = 1 and 1 + ω + ω² = 0
Also, 1 + ω² = -ω, 1 + ω = -ω²
∴ L.H.S. = (1 – ω + ω²)⁶ + (1 + ω – ω²)⁶
= [(1 + ω²) – ω]⁶ + [(1 + ω) – ω²]⁶
= (-ω – ω))⁶ + (-ω² – ω²)6
= (-2ω)⁶ + (-2ω²)⁶
= 64ω⁶ + 64ω¹²
= 64(ω³)² + 64(ω³)⁴
= 64(1)² + 64(1)⁴
= 128
= R.H.S.

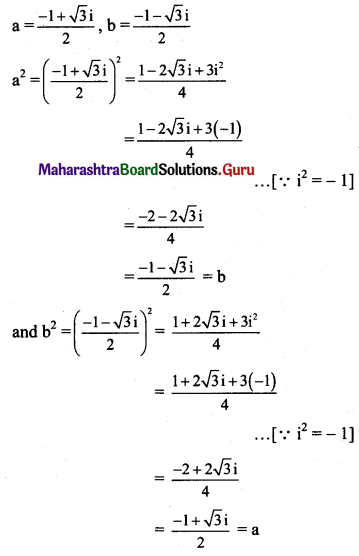
Question 20.
If ω is the cube root of unity, then find the value of \(\left(\frac{-1+\mathbf{i} \sqrt{3}}{2}\right)^{18}+\left(\frac{-1-\mathbf{i} \sqrt{3}}{2}\right)^{18}\)
Solution:
If ω is the complex cube root of unity, then

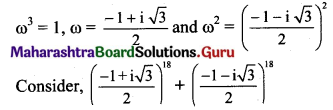
Given Expression = ω¹⁸ + (ω²)¹⁸
= ω¹⁸ + ω³⁶
= (ω³)⁶ + (ω³)¹²
= (1)⁶ + (1)¹²
= 2

Balbharti Maharashtra State Board 11th Maths Book Solutions Pdf Chapter 3 Permutations and Combination Ex 3.3 Questions and Answers.

Maharashtra State Board 11th Maths Solutions Chapter 3 Permutations and Combination Ex 3.3

Question 1.
Find n, if ⁿP₆ : ⁿP₃ = 120 : 1.
Solution:
ⁿP₆ : ⁿP₃ = 120 : 1

∴ (n – 3) (n – 4) (n – 5) = 120
∴ (n – 3) (n – 4) (n – 5) = 6 × 5 × 4
Comparing on both sides, we get
n – 3 = 6
∴ n = 9

Question 2.
Find m and n, if ^(m+n)P₂ = 56 and ^(m-n)P₂ = 12.
Solution:
^(m+n)P₂ = 56

(m + n) (m + n – 1) = 56
Let m + n = t
t(t – 1) = 56
t² – t – 56 = 0
(t – 8) (t + 7) = 0
t = 8 or t = -7
m + n = 8 or m + n = -7
But m + n ≠ -7
∴ m + n = 8 ……(i)
Also, ^(m-n)P₂ = 12

(m – n) (m – n – 1) = 12
Let m – n = a
a(a – 1) = 12
a² – a – 12 = 0
(a – 4)(a + 3) = 0
a = 4 or a = -3
m – n = 4 or m – n = -3
But m – n ≠ -3
∴ m – n = 4 ……(ii)
Adding (i) and (ii), we get
2m = 12
∴ m = 6
Substituting m = 6 in (ii), we get
6 – n = 4
∴ n = 2

Question 3.
Find r, if ¹²P_r-2 : ¹¹P_r-1 = 3 : 14.
Solution:

(14 – r)(13 – r) = 8 × 7
Comparing on both sides, we get
14 – r = 8
∴ r = 6

Question 4.
Show that (n + 1) (ⁿP_r) = (n – r + 1) [⁽ⁿ⁺¹⁾P_r]
Solution:

Question 5.
How many 4 letter words can be formed using letters in the word MADHURI, if (a) letters can be repeated (b) letters cannot be repeated.
Solution:
There are 7 letters in the word MADHURI.
(a) A 4 letter word is to be formed from the letters of the word MADHURI and repetition of letters is allowed.
∴ 1st letter can be filled in 7 ways.
2nd letter can be filled in 7 ways.
3rd letter can be filled in 7 ways.
4th letter can be filled in 7 ways.
∴ Total no. of ways a 4-letter word can be formed = 7 × 7 × 7 × 7 = 2401

(b) When repetition of letters is not allowed, the number of 4-letter words formed from the letters of the word MADHURI is ⁷P₄ = \(\frac{7 !}{(7-4) !}=\frac{7 \times 6 \times 5 \times 4 \times 3 !}{3 !}\) = 840

Question 6.
Determine the number of arrangements of letters of the word ALGORITHM if
(a) vowels are always together.
(b) no two vowels are together.
(c) consonants are at even positions.
(d) O is the first and T is the last letter.
Solution:
There are 9 letters in the word ALGORITHM.
(a) When vowels are always together.
There are 3 vowels in the word ALGORITHM (i.e., A, I, O).
Let us consider these 3 vowels as one unit.
This unit with 6 other letters is to be arranged.
∴ The number of arrangement = ⁷P₇ = 7! = 5040
3 vowels can be arranged among themselves in ³P₃ = 3! = 6 ways.
∴ Required number of arrangements = 7! × 3!
= 5040 × 6
= 30240

(b) When no two vowels are together.
There are 6 consonants in the word ALGORITHM,
they can be arranged among themselves in ⁶P₆ = 6! = 720 ways.
Let consonants be denoted by C.
_C _C_ C _C_C_C
There are 7 places marked by ‘_’ in which 3 vowels can be arranged.
∴ Vowels can be arranged in ⁷P₃ = \(\frac{7 !}{(7-3) !}=\frac{7 \times 6 \times 5 \times 4 !}{4 !}\) = 210 ways.
∴ Required number of arrangements = 720 × 210 = 151200

(c) When consonants are at even positions.
There are 4 even places and 6 consonants in the word ALGORITHM.
∴ 6 consonants can be arranged at 4 even positions in 6P4 = \(\frac{6 !}{(6-4) !}=\frac{6 \times 5 \times 4 \times 3 \times 2 !}{2 !}\) = 360 ways.
Remaining 5 letters (3 vowels and 2 consonants) can be arranged in odd position in ⁵P₅ = 5! = 120 ways.
∴ Required number of arrangements = 360 × 120 = 43200

(d) When O is the first and T is the last letter.
All the letters of the word ALGORITHM are to be arranged among themselves such that arrangement begins with O and ends with T.
∴ Position of O and T are fixed.
∴ Other 7 letters can be arranged between O and T among themselves in ⁷P₇ = 7! = 5040 ways.
∴ Required number of arrangements = 5040

Question 7.
In a group photograph, 6 teachers and principal are in the first row and 18 students are in the second row. There are 12 boys and 6 girls among the students. If the middle position is reserved for the principal and if no two girls are together, find the number of arrangements.
Solution:
In 1st row, 6 teachers can be arranged among themselves in ⁶P₆ = 6! ways.
In the 2nd row, 12 boys can be arranged among themselves in ¹²P₁₂ = 12! ways.
No two girls are together.
So, there are 13 places formed by 12 boys in which 6 girls occupy any 6 places in ¹³P₆ ways.
∴ Required number of arrangements

Question 8.
Find the number of ways so that letters of the word HISTORY can be arranged as
(a) Y and T are together
(b) Y is next to T
(c) there is no restriction
(d) begin and end with a vowel
(e) end in ST
(f) begin with S and end with T
Solution:
There are 7 letters in the word HISTORY
(a) When ‘Y’ and ‘T’ are together.
Let us consider ‘Y’ and ‘T’ as one unit.
This unit with other 5 letters are to be arranged.
∴ The number of arrangement of one unit and 5 letters = ⁶P₆ = 6! = 720
Also, ‘Y’ and ‘T’ can be arranged among themselves in ²P₂ = 2! = 2 ways.
∴ A total number of arrangements when Y and T are always together = 6! × 2!
= 120 × 2
= 1440

(b) When ‘Y’ is next to ‘T’.
Let us take this (‘Y’ next to ‘T’) as one unit.
This unit with 5 other letters is to be arranged.
∴ The number of arrangements of 5 letters and one unit = ⁶P₆ = 6! = 720
Also, ‘Y’ has to be always next to ‘T’.
∴ They can be arranged among themselves in 1 way only.
∴ Total number of arrangements possible when Y is next to T = 720 × 1 = 720

(c) When there is no restriction.
7 letters can be arranged among themselves in ⁷P₇ = 7! ways.
∴ The total number of arrangements possible if there is no restriction = 7!

(d) When begin and end with a vowel.
There are 2 vowels in the word HISTORY.
All other letters of the word HISTORY are to be arranged between 2 vowels such that the arrangement begins and ends with a vowel.
The other 5 letters can be filled between the two vowels in ⁵P₅ = 5! = 120 ways.
Also, 2 vowels can be arranged among themselves at first and last places in ²P₂ = 2! = 2 ways.
∴ Total number of arrangements when the word begins and ends with vowel = 120 × 2 = 240

(e) When a word ends in ST.
As the arrangement ends with ST,
the remaining 5 letters can be arranged among themselves in ⁵P₅ = 5! = 120 ways.
∴ Total number of arrangements when the word ends with ST = 120

(f) When a word begins with S and ends with T.
As arrangement begins with S and ends with T,
the remaining 5 letters can be arranged between S and T among themselves in ⁵P₅ = 5! = 120 ways.
Total number of arrangements when the word begins with S and ends with T = 120

Question 9.
Find the number of arrangements of the letters in the word SOLAPUR so that consonants and vowels are placed alternately.
Solution:
There are 4 consonants S, L, P, R, and 3 vowels A, O, U in the word SOLAPUR.
Consonants and vowels are to be alternated.
∴ Vowels must occur in even places and consonants in odd places.
∴ 3 vowels can be arranged at 3 even places in ³P₃ = 3! = 6 ways.
Also, 4 consonants can be arranged at 4 odd places in ⁴P₄ = 4! = 24 ways.
Required number of arrangements = 6 × 24 = 144

Question 10.
Find the number of 4-digit numbers that can be formed using the digits 1, 2, 4, 5, 6, 8 if
(a) digits can be repeated.
(b) digits cannot be repeated.
Solution:
(a) A 4 digit number is to be made from the digits 1, 2, 4, 5, 6, 8 such that digits can be repeated.
∴ Unit’s place digit can be filled in 6 ways.
10’s place digit can be filled in 6 ways.
100’s place digit can be filled in 6 ways.
1000’s place digit can be filled in 6 ways.
∴ Total number of numbers that can be formed = 6 × 6 × 6 × 6 = 1296

(b) A 4 different digit number is to be made from the digits 1, 2, 4, 5, 6, 8 without repetition of digits.
∴ 4 different digits are to be arranged from 6 given digits which can be done in ⁶P₄ ways.
∴ Total number of numbers that can be formed
= \(\frac{6 !}{(6-4) !}\)
= \(\frac{6 \times 5 \times 4 \times 3 \times 2 !}{2 !}\)
= 360

Question 11.
How many numbers can be formed using the digits 0, 1, 2, 3, 4, 5 without repetition so that resulting numbers are between 100 and 1000?
Solution:
A number between 100 and 1000 that can be formed from the digits 0, 1, 2, 3, 4, 5 is of 3 digits, and repetition of digits is not allowed.
∴ 100’s place can be filled in 5 ways as it is a non-zero number.
10’s place digits can be filled in 5 ways.
Unit’s place digit can be filled in 4 ways.
∴ Total number of ways the number can be formed = 5 × 5 × 4 = 100

Question 12.
Find the number of 6-digit numbers using the digits 3, 4, 5, 6, 7, 8 without repetition. How many of these numbers are (a) divisible by 5 (b) not divisible by 5
Solution:
A number of 6 different digits is to be formed from the digits 3, 4, 5, 6, 7, 8 which can be done in ⁶P₆ = 6! = 720 ways.
(a) If the number is to be divisible by 5,
the unit’s place digit can be 5 only.
∴ it can be arranged in 1 way only.
The other 5 digits can be arranged among themselves in ⁵P₅ = 5! = 120 ways.
∴ Required number of numbers divisible by 5 = 1 × 120 = 120

(b) If the number is not divisible by 5,
unit’s place can be any digit from 3, 4, 6, 7, 8.
∴ it can be arranged in 5 ways.
Other 5 digits can be arranged in ⁵P₅ = 5! = 120 ways.
∴ Required number of numbers not divisible by 5 = 5 × 120 = 600

Question 13.
A code word is formed by two different English letters followed by two non-zero distinct digits. Find the number of such code words. Also, find the number of such code words that end with an even digit.
Solution:
There is a total of 26 alphabets.
A code word contains 2 English alphabets.
∴ 2 alphabets can be filled in ²⁶P₂
= \(\frac{26 !}{(26-2) !}\)
= \(\frac{26 \times 25 \times 24 !}{24 !}\)
= 650 ways.
Also, alphabets to be followed by two distinct non-zero digits from 1 to 9 which can be filled in
⁹P₂ = \(\frac{9 !}{(9-2) !}=\frac{9 \times 8 \times 7 !}{7 !}\) = 72 ways.
∴ Total number of a code words = 650 × 72 = 46800.
To find the number of codewords end with an even integer.
2 alphabets can be filled in 650 ways.
The digit in the unit’s place should be an even number between 1 to 9, which can be filled in 4 ways.
Also, 10’s place can be filled in 8 ways.
∴ Total number of codewords = 650 × 4 × 8 = 20800

Question 14.
Find the number of ways in which 5 letters can be posted in 3 post boxes if any number of letters can be posted in a post box.
Solution:
There are 5 letters and 3 post boxes and any number of letters can be posted in all three post boxes.
∴ Each letter can be posted in 3 ways.
∴ Total number of ways 5 letters can be posted = 3 × 3 × 3 × 3 × 3 = 243

Question 15.
Find the number of arranging 11 distinct objects taken 4 at a time so that a specified object (a) always occurs (b) never occurs
Solution:
There are 11 distinct objects and 4 are to be taken at a time.
(a) The number of permutations of n distinct objects, taken r at a time, when one particular object will always occur is \(\mathbf{r} \times{ }^{(\mathbf{n}-1)} \mathbf{P}_{(\mathbf{r}-1)}\)

∴ In 2880 permutations of 11 distinct objects, taken 4 at a time, one particular object will always occur.

(b) When one particular object will not occur, then 4 objects are to be arranged from 10 objects which can be done in ¹⁰P₄ = 10 × 9 × 8 × 7 = 5040 ways.
∴ In 5040 permutations of 11 distinct objects, taken 4 at a time, one particular object will never occur.

Question 16.
In how many ways can 5 different books be arranged on a shelf if
(i) there are no restrictions
(ii) 2 books are always together
(iii) 2 books are never together
Solution:
(i) 5 books arranged in ⁵P₅ = 5! = 120 ways.

(ii) 2 books are together.
Let us consider two books as one unit. This unit with the other 3 books can be arranged in ⁴P₄ = 4! = 24 ways.
Also, two books can be arranged among themselves in ²P₂ = 2 ways.
∴ Required number of arrangements = 24 × 2 = 48

(iii) Say books are B₁, B₂, B₃, B₄, B₅ are to be arranged with B₁, B₂ never together.
B₃, B₄, B₅ can be arranged among themselves in ³P₃ = 3! = 6 ways.
B₃, B₄, B₅ create 4 gaps in which B₁, B₂ are arranged in ⁴P₂ = 4 × 3 = 12 ways.
∴ Required number of arrangements = 6 × 12 = 72

Question 17.
3 boys and 3 girls are to sit in a row. How many ways can this be done if
(i) there are no restrictions.
(ii) there is a girl at each end.
(iii) boys and girls are at alternate places.
(iv) all-boys sit together.
Solution:
3 boys and 3 girls are to be arranged in a row.
(i) When there are no restrictions.
∴ Required number of arrangements = 6! = 720

(ii) When there is a girl at each end.
3 girls can be arranged at two ends in
³P₂ = \(\frac{3 !}{1 !}\) = 3 × 2 = 6 ways.
And remaining 1 girl and 3 boys can be arranged between the two girls in ⁴P₄ = 4! = 24 ways.
∴ Required number of arrangements = 6 × 24 = 144

(iii) Boys and girls are at alternate places.
We can first arrange 3 girls among themselves in ³P₃ = 3! = 6 ways.
Let girls be denoted by G.
G – G – G –
There are 3 places marked by ‘-’ where 3 boys can be arranged in 3! = 6 ways.
∴ Total number of such arrangements = 6 × 6 = 36
OR
Similarly, we can first arrange 3 boys in 3! = 6 ways
and then arrange 3 girls alternately in 3! = 6 ways.
∴ Total number of such arrangements = 6 × 6 = 36
∴ Required number of arrangements = 36 + 36 = 72

(iv) All boys sit together.
Let us consider all boys as one group.
This one group with the other 3 girls can be arranged ⁴P₄ = 4! = 24 ways.
Also, 3 boys can be arranged among themselves in ³P₃ = 3! = 6 ways.
∴ Required number of arrangements = 24 × 6 = 144

Balbharti Maharashtra State Board 11th Maths Book Solutions Pdf Chapter 7 Limits Ex 7.7 Questions and Answers.

Maharashtra State Board 11th Maths Solutions Chapter 7 Limits Ex 7.7

I. Evaluate the following:

Question 1.
\(\lim _{x \rightarrow \infty}\left[\frac{a x^{3}+b x^{2}+c x+d}{e x^{3}+f x^{2}+g x+h}\right]\)
Solution:

Question 2.
\(\lim _{x \rightarrow \infty}\left[\frac{x^{3}+3 x+2}{(x+4)(x-6)(x-3)}\right]\)
Solution:

Question 3.
\(\lim _{x \rightarrow \infty}\left[\frac{7 x^{2}+5 x-3}{8 x^{2}-2 x+7}\right]\)
Solution:

II. Evaluate the following:

Question 1.
\(\lim _{x \rightarrow \infty}\left[\frac{7 x^{2}+2 x-3}{\sqrt{x^{4}+x+2}}\right]\)
Solution:

Question 2.
\(\lim _{x \rightarrow \infty}\left[\sqrt{x^{2}+4 x+16}-\sqrt{x^{2}+16}\right]\)
Solution:

Question 3.
\(\lim _{x \rightarrow \infty}\left[\sqrt{x^{4}+4 x^{2}}-x^{2}\right]\)
Solution:

III. Evaluate the following:

Question 1.
\(\lim _{x \rightarrow \infty}\left[\frac{\left(3 x^{2}+4\right)\left(4 x^{2}-6\right)\left(5 x^{2}+2\right)}{4 x^{6}+2 x^{4}-1}\right]\)
Solution:

Question 2.
\(\lim _{x \rightarrow \infty}\left[\frac{(3 x-4)^{3}(4 x+3)^{4}}{(3 x+2)^{7}}\right]\)
Solution:

Question 3.
\(\lim _{x \rightarrow \infty}[\sqrt{x}(\sqrt{x+1}-\sqrt{x})]\)
Solution:

Question 4.
\(\lim _{x \rightarrow \infty}\left[\frac{(2 x-1)^{20}(3 x-1)^{30}}{(2 x+1)^{50}}\right]\)
Solution:

Question 5.
\(\lim _{x \rightarrow \infty}\left[\frac{\sqrt{x^{2}+5}-\sqrt{x^{2}-3}}{\sqrt{x^{2}+3}-\sqrt{x^{2}+1}}\right]\)
Solution:

Balbharti Maharashtra State Board 11th Maths Book Solutions Pdf Chapter 3 Permutations and Combination Ex 3.6 Questions and Answers.

Maharashtra State Board 11th Maths Solutions Chapter 3 Permutations and Combination Ex 3.6

Question 1.
Find the value of
(a) ¹⁵C₄
Solution:

(b) ⁸⁰C₂
Solution:

(c) ¹⁵C₄ + ¹⁵C₅
Solution:

(d) ²⁰C₁₆ – ¹⁹C₁₆
Solution:

Question 2.
Find n if
(a) ⁶P₂ = n(⁶C₂)
Solution:

(b) ²ⁿC₃ : ⁿC₂ = 52 : 3
Solution:

(c) ⁿC_n-3 = 84
Solution:

Question 3.
Find r if ¹⁴C_2r : ¹⁰C_2r-4 = 143 : 10.
Solution:

∴ 2r(2r – 1) (2r – 2) (2r – 3) = 14 × 12 × 10
∴ 2r(2r – 1) (2r – 2) (2r – 3) = 8 × 7 × 6 × 5
Comparing on both sides, we get
∴ r = 4

Question 4.
Find n and r if,
(a) ⁿP_r = 720 and ⁿC_n-r = 120
Solution:

(b) ⁿC_r-1 : ⁿC_r : ⁿC_r+1 = 20 : 35 : 42
Solution:

Question 5.
If ⁿP_r = 1814400 and ⁿC_r = 45, find ⁿ⁺⁴C_r+3.
Solution:

Question 6.
If ⁿC_r-1 = 6435, ⁿC_r = 5005, ⁿC_r+1 = 3003, find ^rC₅.
Solution:

Question 7.
Find the number of ways of drawing 9 balls from a bag that has 6 red balls, 8 green balls, and 7 blue balls so that 3 balls of every colour are drawn.
Solution:
9 balls are to be selected from 6 red, 8 green, 7 blue balls such that the selection consists of 3 balls of each colour.
∴ 3 red balls can be selected from 6 red balls in ⁶C₃ ways.
3 reen balls can be selected from 8 green balls in ⁸C₃ ways.
3 blue balls can be selected from 7 blue balls in ⁷C₃ ways.
∴ Number of ways selection can be done if the selection consists of 3 balls of each colour

Question 8.
Find the number of ways of selecting a team of 3 boys and 2 girls from 6 boys and 4 girls.
Solution:
There are 6 boys and 4 girls.
A team of 3 boys and 2 girls is to be selected.
∴ 3 boys can be selected from 6 boys in ⁶C₃ ways.
2 girls can be selected from 4 girls in ⁴C₂ ways.
∴ Number of ways the team can be selected

Question 9.
After a meeting, every participant shakes hands with every other participants. If the number of handshakes is 66, find the number of participants in the meeting.
Solution:
Let there be n participants present in the meeting.
A handshake occurs between 2 persons.
∴ Number of handshakes = ⁿC₂
Given 66 handshakes were exchanged.
66 = ⁿC₂
66 = \(\frac{\mathrm{n} !}{2 !(\mathrm{n}-2) !}\)
66 × 2 = \(\frac{n(n-1)(n-2) !}{(n-2) !}\)
132 = n (n – 1)
n(n – 1) = 12 × 11
Comparing on both sides, we get n = 12
∴ 12 participants were present at the meeting.

Question 10.
If 20 points are marked on a circle, how many chords can be drawn?
Solution:
To draw a chord we need to join two points on the circle.
There are 20 points on a circle.
∴ Total number of chords possible from these points

Question 11.
Find the number of diagonals of an n-sided polygon. In particular, find the number of diagonals when
(i) n = 10
(ii) n = 15
(iii) n = 12
(iv) n = 8
Solution:
In n-sided polygon, there are ‘n’ points and ‘n’ sides.
∴ Through ‘n’ points we can draw ⁿC₂ lines including sides.
∴ Number of diagonals in n sided polygon = ⁿC₂ – n (n = number of sides)

Question 12.
There are 20 straight lines in a plane so that no two lines are parallel and no three lines are concurrent. Determine the number of points of intersection.
Solution:
There are 20 lines such that no two of them are parallel and no three of them are concurrent.
Since no two lines are parallel, they intersect at a point.
∴ Number of points of intersection if no two lines are parallel and no three lines are concurrent = ²⁰C₂
= \(\frac{20 !}{2 ! 18 !}\)
= \(\frac{20 \times 19 \times 18 !}{2 \times 1 \times 18 !}\)
= 190

Question 13.
Ten points are plotted on a plane. Find the number of straight lines obtained by joining these points if (a) no three points are collinear (b) four points are collinear
Solution:
There are 10 points on a plane.
(a) When no three of them are collinear.
A line is obtained by joining 2 points.
∴ Number of lines passing through these points = ¹⁰C₂
= \(\frac{10 !}{2 ! 8 !}\)
= \(\frac{10 \times 9 \times 8 !}{2 \times 1 \times 8 !}\)
= 5 × 9
= 45

(b) When 4 of them are collinear.
If no three points are collinear, we get a total of ¹⁰C₂ = 45 lines by joining them. …..[From (i)]
Since 4 points are collinear, only one line passes through these points instead of ⁴C₂ lines.
∴ ⁴C₂ – 1 extra lines are included in 45 lines.
Number of lines passing through these points
= 45 – (⁴C₂ – 1)
= 45 – \(\frac{4 !}{2 ! 2 !}\) + 1
= 45 – \(\frac{4 \times 3 \times 2 !}{2 \times 2 !}\) + 1
= 45 – 6 + 1
= 40

Question 14.
Find the number of triangles formed by joining 12 points if
(a) no three points are collinear
(b) four points are collinear
Solution:
There are 12 points on the plane.
(a) When no three of them are collinear.
A triangle can be drawn by joining any three non-collinear points.
∴ Number of triangles that can be obtained from these points = ¹²C₃
= \(\frac{12 !}{3 ! 9 !}\)
= \(\frac{12 \times 11 \times 10 \times 9 !}{3 \times 2 \times 1 \times 9 !}\)
= 220

(b) When 4 of these points are collinear.
If no three points are collinear, total we get ¹²C₃ = 220 triangles by joining them. ……[From (i)]
Since 4 points are collinear, no triangle can be formed by joining these four points.
∴ ⁴C₃ extra triangles are included in 220 triangles.
∴ Number of triangles that can be obtained from these points = ¹²C₃ – ⁴C₃
= 220 – \(\frac{4 !}{3 ! \times 1 !}\)
= 220 – \(\frac{4 \times 3 !}{3 !}\)
= 220 – 4
= 216

Question 15.
A word has 8 consonants and 3 vowels. How many distinct words can be formed if 4 consonants and 2 vowels are chosen?
Solution:
There are 8 consonants and 3 vowels.
From 8 consonants, 4 can be selected in ⁸C₄
= \(\frac{8 !}{4 ! 4 !}\)
= \(\frac{8 \times 7 \times 6 \times 5 \times 4 !}{4 \times 3 \times 2 \times 1 \times 4 !}\)
= 70 ways.
From 3 vowels, 2 can be selected in ³C₂
= \(\frac{3 !}{2 ! 1 !}\)
= \(\frac{3 \times 2 !}{2 !}\)
= 3 ways.
Now, to form a word, these 6 ietters (i.e., 4 consonants and 2 vowels) can be arranged in ⁶P₆ = 6! ways.
∴ Total number of words that can be formed = 70 × 3 × 6!
= 70 × 3 × 720
= 151200
∴ 151200 words of 4 consonants and 2 vowels can be formed.

Question 16.
Find n if,
(i) ⁿC₈ = ⁿC₁₂
Solution:
ⁿC₈ = ⁿC₁₂
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ 8 = 12 or 8 = n – 12
But 8 = 12 is not possible
∴ 8 = n – 12
∴ n = 20

(ii) ²³C_3n = ²³C_2n+3
Solution:
²³C_3n = ²³C_2n+3
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ 3n = 2n + 3 or 3n = 23 – 2n – 3
∴ n = 3 or n = 4

(iii) ²¹C_6n = \({ }^{21} \mathrm{C}_{\left(\mathrm{n}^{2}+5\right)}\)
Solution:
²¹C_6n = \({ }^{21} \mathrm{C}_{\left(\mathrm{n}^{2}+5\right)}\)
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ 6n = n² + 5 or 6n = 21 – (n² + 5)
∴ n² – 6n + 5 = 0 or 6n = 21 – n² – 5
∴ n² – 6n + 5 = 0 or n² + 6n – 16 = 0
If n² – 6n + 5 = 0, then (n – 1)(n – 5) = 0
∴ n = 1 or n = 5
If n = 5 then
n² + 5 = 30 > 21
∴ n ≠ 5
∴ n = 1
If n² + 6n – 16 = 0, then (n + 8)(n – 2) = 0
n = -8 or n = 2
n ≠ -8
∴ n = 2
∴ n = 1 or n = 2

Check:
n = 2
∴ n² + 5 = 2² + 5 = 9
²¹C_6n = ²¹C₁₂
and \({ }^{21} \mathrm{C}_{\left(\mathrm{n}^{2}+5\right)}\) = ²¹C₉
∴ ⁿC_r = ⁿC_n-r
∴ ²¹C₁₂ = ²¹C₉
∴ n = 2 is a right answer.

(iv) ²ⁿC_r-1 = ²ⁿC_r+1
Solution:
²ⁿC_r-1 = ²ⁿC_r+1
If ⁿC_x = ⁿC_y, then either x = y or x = n – y
∴ r – 1 = r + 1 or r – 1 = 2n – (r + 1)
But r – 1 = r + 1 is not possible
∴ r – 1 = 2n – (r + 1)
∴ r + r = 2n
∴ r = n

Check:
²ⁿC_r-1 = ²ⁿC_n-1
and ²ⁿC_r+1 = ²ⁿC_n+1
using ⁿC_r = ⁿC_n-r, we have
²ⁿC_n+1 = ²ⁿC_2n-(n+1) = ²ⁿC_n-1
∴ ²ⁿC_r-1 = ²ⁿC_r+1

(v) ⁿC_n-2 = 15
Solution:
ⁿC_n-2 = 15
∴ ⁿC₂ = 15 …..[∵ ⁿC_r = ⁿC_n-r]
∴ \(\frac{n !}{(n-2) ! 2 !}=15\)
∴ \(\frac{n(n-1)(n-2) !}{(n-2) ! \times 2 \times 1}=15\)
∴ n(n – 1) = 30
∴ n(n – 1) = 6 × 5
Equating both sides, we get
∴ n = 6

Question 17.
Find x if ⁿP_r = x ⁿC_r.
Solution:

Question 18.
Find r if ¹¹C₄ + ¹¹C₅ + ¹²C₆ + ¹³C₇ = ¹⁴C_r.
Solution:

Question 19.
Find the value of \(\sum_{r=1}^{4}{ }^{(21-r)} \mathrm{C}_{4}\).
Solution:

Question 20.
Find the differences between the greatest values in the following:
(a) ¹⁴C_r and ¹²C_r
Solution:
Greatest value of ¹⁴C_r.
Here, n = 14, which is even.
Greatest value of nCr occurs at r = \(\frac{n}{2}\) if n is even.
∴ r = \(\frac{n}{2}\)
∴ r = \(\frac{14}{2}\) = 7

∴ Difference between the greatest values of ¹⁴C_r and ¹²C_r = ¹⁴C_r – ¹²C_r
= 3432 – 924
= 2508

(b) ¹³C_r and ⁸C_r
Solution:
Greatest value of ¹³C_r.
Here n = 13, which is odd.
Greatest value of nCr occurs at r = \(\frac{n-1}{2}\) if n is odd.
∴ r = \(\frac{\mathrm{n}-1}{2}\)
∴ r = \(\frac{13-1}{2}\) = 6

∴ Difference between the greatest values of ¹³C_r and ⁸C_r = ¹³C_r – ⁸C_r
= 1716 – 70
= 1646

(c) ¹⁵C_r and ¹¹C_r
Solution:
Greatest value of ¹⁵C_r.
Here n = 15, which is odd.
Greatest value of nCr occurs at r = \(\frac{n-1}{2}\) if n is odd.
∴ r = \(\frac{n-1}{2}\)
∴ r = \(\frac{15-1}{2}\) = 7

∴ Difference between the greatest values of ¹⁵C_r and ¹¹C_r = ¹⁵C_r – ¹¹C_r
= 6435 – 462
= 5973

Question 21.
In how many ways can a boy invite his 5 friends to a party so that at least three join the party?
Solution:
Boy can invite = (3 or 4 or 5 friends)
Consider the following table:

∴ Number of ways a boy can invite his friends to a party so that three or more of join the party = 10 + 5 + 1 = 16

Question 22.
A group consists of 9 men and 6 women. A team of 6 is to be selected. How many of possible selections will have at least 3 women?
Solution:
There are 9 men and 6 women.
A team of 6 persons is to be formed such that it consist of at least 3 women.
Consider the following table:

∴ No. of ways this can be done = 1680 + 540 + 54 + 1 = 2275
∴ 2275 teams can be formed if team consists of at least 3 women.

Question 23.
A committee of 10 persons is to be formed from a group of 10 women and 8 men. How many possible committees will have at least 5 women? How many possible committees will have men in majority?
Solution:
(i) A committee of 10 persons is to be formed from 10 women and 8 men such that the committee contains at least 5 women.
Consider the following table:

∴ Number of committees with at least 5 women
= 14112 + 14700 + 6720 + 1260 + 81
= 36873

(ii) Number of committees with men in majority = Total number of committees – (Number of committees with women in majority + women and men equal in number)
= ¹⁸C₁₀ – 36873
= ¹⁸C₈ – 36873
= 43758 – 36873
= 6885

Question 24.
A question paper has two sections. Section I has 5 questions and section II has 6 questions. A student must answer at least two questions from each section among 6 questions he answers. How many different choices does the student have in choosing questions?
Solution:
There are 11 questions, out of which 5 questions are from section I and 6 questions are from section II.
The student has to select 6 questions taking at least 2 questions from each section.
Consider the following table:

∴ Number of choices = 150 + 200 + 75 = 425
∴ In 425 ways students can select 6 questions, taking at least 2 questions from each section.

Question 25.
There are 3 wicketkeepers and 5 bowlers among 22 cricket players. A team of 11 player is to be selected so that there is exactly one wicketkeeper and at least 4 bowlers in the team. How many different teams can be formed?
Solution:
There are 22 cricket players, of which 3 are wicketkeepers and 5 are bowlers.
A team of 11 players is to be chosen such that exactly one wicket keeper and at least 4 bowlers are to be included in the team.
Consider the following table:

∴ Number of ways a team of 11 players can be selected
= 45045 + 6006
= 51051

Question 26.
Five students are selected from 11. How many ways can these students be selected if
(a) two specified students are selected?
(b) two specified students are not selected?
Solution:
5 students are to be selected from 11 students.
(a) When 2 specified students are included,
then remaining 3 students can be selected from (11 – 2) = 9 students.
∴ Number of ways of selecting 3 students from 9 students = ⁹C₃
= \(\frac{9 !}{3 ! \times 6 !}\)
= \(\frac{9 \times 8 \times 7 \times 6 !}{3 \times 2 \times 1 \times 6 !}\)
= 84
∴ Selection of students is done in 84 ways when 2 specified students are included.

(b) When 2 specified students are not included, then 5 students can be selected from the remaining (11 – 2) = 9 students.
∴ Number of ways of selecting 5 students from 9 students = ⁹C₅
= \(\frac{9 !}{5 ! 4 !}\)
= \(\frac{9 \times 8 \times 7 \times 6 \times 5 !}{5 ! \times 4 \times 3 \times 2 \times 1}\)
= 126
∴ Selection of students is done in 126 ways when 2 specified students are not included.

Balbharti Maharashtra State Board 11th Maths Book Solutions Pdf Chapter 7 Limits Ex 7.6 Questions and Answers.

Maharashtra State Board 11th Maths Solutions Chapter 7 Limits Ex 7.6

I. Evaluate the following limits:

Question 1.
\(\lim _{x \rightarrow 0}\left[\frac{9^{x}-5^{x}}{4^{x}-1}\right]\)
Solution:

Question 2.
\(\lim _{x \rightarrow 0}\left[\frac{5^{x}+3^{x}-2^{x}-1}{x}\right]\)
Solution:

Question 3.
\(\lim _{x \rightarrow 0}\left(\frac{a^{x}+b^{x}+c^{x}-3}{\sin x}\right)\)
Solution:

Question 4.
\(\lim _{x \rightarrow 0}\left(\frac{6^{x}+5^{x}+4^{x}-3^{x+1}}{\sin x}\right)\)
Solution:

Question 5.
\(\lim _{x \rightarrow 0}\left(\frac{8^{\sin x}-2^{\tan x}}{e^{2 x}-1}\right)\)
Solution:

II. Evaluate the following limits:

Question 1.
\(\lim _{x \rightarrow 0}\left[\frac{3^{x}+3^{-x}-2}{x \cdot \tan x}\right]\)
Solution:

Question 2.
\(\lim _{x \rightarrow 0}\left[\frac{3+x}{3-x}\right]^{\frac{1}{x}}\)
Solution:

Question 3.
\(\lim _{x \rightarrow 0}\left[\frac{5 x+3}{3-2 x}\right]^{\frac{2}{x}}\)
Solution:

Question 4.
\(\lim _{x \rightarrow 0}\left[\frac{\log (3-x)-\log (3+x)}{x}\right]\)
Solution:

Question 5.
\(\lim _{x \rightarrow 0}\left[\frac{4 x+1}{1-4 x}\right]^{\frac{1}{x}}\)
Solution:

Question 6.
\(\lim _{x \rightarrow 0}\left[\frac{5+7 x}{5-3 x}\right]^{\frac{1}{3 x}}\)
Solution:

III. Evaluate the following limits:

Question 1.
\(\lim _{x \rightarrow 0}\left[\frac{a^{x}-b^{x}}{\sin (4 x)-\sin (2 x)}\right]\)
Solution:

Question 2.
\(\lim _{x \rightarrow 0}\left[\frac{\left(2^{x}-1\right)^{3}}{\left(3^{x}-1\right) \cdot \sin x \cdot \log (1+x)}\right]\)
Solution:

Question 3.
\(\lim _{x \rightarrow 0}\left[\frac{15^{x}-5^{x}-3^{x}+1}{x \cdot \sin x}\right]\)
Solution:

Question 4.
\(\lim _{x \rightarrow 0}\left[\frac{(25)^{x}-2(5)^{x}+1}{x \cdot \sin x}\right]\)
Solution:

Question 5.
\(\lim _{x \rightarrow 0}\left[\frac{(49)^{x}-2(35)^{x}+(25)^{x}}{\sin x \cdot \log (1+2 x)}\right]\)
Solution:

Balbharti Maharashtra State Board 11th Maths Book Solutions Pdf Chapter 7 Limits Ex 7.5 Questions and Answers.

Maharashtra State Board 11th Maths Solutions Chapter 7 Limits Ex 7.5

I. Evaluate the following:

Question 1.
\(\lim _{x \rightarrow \frac{\pi}{2}}\left[\frac{cosec x-1}{\left(\frac{\pi}{2}-x\right)^{2}}\right]\)
Solution:

Question 2.
\(\lim _{x \rightarrow a} \frac{\sin x-\sin a}{\sqrt[5]{x}-\sqrt[5]{a}}\)
Solution:

Question 3.
\(\lim _{x \rightarrow \pi}\left[\frac{\sqrt{5+\cos x}-2}{(\pi-x)^{2}}\right]\)
Solution:

Question 4.
\(\lim _{x \rightarrow \frac{\pi}{6}}\left[\frac{\cos x-\sqrt{3} \sin x}{\pi-6 x}\right]\)
Solution:

Question 5.
\(\lim _{x \rightarrow 1}\left[\frac{1-x^{2}}{\sin \pi x}\right]\)
Solution:

II. Evaluate the following:

Question 1.
\(\lim _{x \rightarrow \frac{\pi}{6}}\left[\frac{2 \sin x-1}{\pi-6 x}\right]\)
Solution:

Question 2.
\(\lim _{x \rightarrow \frac{\pi}{4}}\left[\frac{\sqrt{2}-\cos x-\sin x}{(4 x-\pi)^{2}}\right]\)
Solution:

Question 3.
\(\lim _{x \rightarrow \frac{\pi}{6}}\left[\frac{2-\sqrt{3} \cos x-\sin x}{(6 x-\pi)^{2}}\right]\)
Solution:

Question 4.
\(\lim _{x \rightarrow a}\left[\frac{\sin (\sqrt{x})-\sin (\sqrt{a})}{x-a}\right]\)
Solution:

Question 5.
\(\lim _{x \rightarrow \frac{\pi}{2}}\left[\frac{\cos 3 x+3 \cos x}{(2 x-\pi)^{3}}\right]\)
Solution:

Balbharti Maharashtra State Board 11th Maths Book Solutions Pdf Chapter 1 Complex Numbers Ex 1.1 Questions and Answers.

Maharashtra State Board 11th Maths Solutions Chapter 1 Complex Numbers Ex 1.1

Question 1.
Simplify:
(i) √-16 + 3√-25 + √-36 – √-625
Solution:

= 4i + 3(5i) + 6i – 25i
= 25i – 25i
= 0

(ii) 4√-4 + 5√-9 – 3√-16
Solution:

Question 2.
Write the conjugates of the following complex numbers
(i) 3 + i
Solution:
Conjugate of (3 + i) is (3 – i).

(ii) 3 – i
Solution:
Conjugate of (3 – i) is (3 + i).

(iii) √-5 – √7 i
Solution:
Conjugate of (√-5 – √7 i) is (√-5 + √7 i).

(iv) -√-5
Solution:
-√-5 = -√5 × √-1 = -√5 i
Conjugate of (-√-5) is √5 i

(v) 5i
Solution:
Conjugate of (5i) is (-5i).

(vi) √5 – i
Solution:
Conjugate of (√5 – i) is (√5 + i).

(vii) √2 + √3 i
Solution:
Conjugate of (√2 + √3 i) is (√2 – √3 i)

(viii) cos θ + i sin θ
Solution:
Conjugate of (cos θ + i sin θ) is (cos θ – i sin θ)

Question 3.
Find a and b if
(i) a + 2b + 2ai = 4 + 6i
Solution:
a + 2b + 2ai = 4 + 6i
Equating real and imaginary parts, we get
a + 2b = 4 …..(i)
2a = 6 ……(ii)
∴ a = 3
Substituting, a = 3 in (i), we get
3 + 2b = 4
∴ b = \(\frac{1}{2}\)
∴ a = 3 and b = \(\frac{1}{2}\)

Check:
For a = 3 and b = \(\frac{1}{2}\)
Consider, L.H.S. = a + 2b + 2ai
= 3 + 2(\(\frac{1}{2}\)) + 2(3)i
= 4 + 6i
= R.H.S.

(ii) (a – b) + (a + b)i = a + 5i
Solution:
(a – b) + (a + b)i = a + 5i
Equating real and imaginary parts, we get
a – b = a ……(i)
a + b = 5 ……(ii)
From (i), b = 0
Substituting b = 0 in (ii), we get
a + 0 = 5
∴ a = 5
∴ a = 5 and b = 0

(iii) (a + b) (2 + i) = b + 1 + (10 + 2a)i
Solution:
(a + b) (2 + i) = b + 1 + (10 + 2a)i
2(a + b) + (a + b)i = (b + 1) + (10 + 2a)i
Equating real and imaginary parts, we get
2(a + b) = b + 1
∴ 2a + b = 1 ……(i)
and a + b = 10 + 2a
-a + b = 10 …….(ii)
Subtracting equation (ii) from (i), we get
3a = -9
∴ a = -3
Substituting a = – 3 in (ii), we get
-(-3) + b = 10
∴ b = 7
∴ a = -3 and b = 7

(iv) abi = 3a – b + 12i
Solution:
abi = 3a – b + 12i
∴ 0 + abi = (3a – b) + 12i
Equating real and imaginary parts, we get
3a – b = 0
∴ 3a = b …..(i)
and ab = 12
∴ b = \(\frac{12}{a}\) ……..(ii)
Substituting b = \(\frac{12}{a}\) in (i), we get
3a = \(\frac{12}{a}\)
3a² = 12
a² = 4
a = ±2
When a = 2, b = \(\frac{12}{a}\) = \(\frac{12}{2}\) = 6
When a = -2, b = \(\frac{12}{a}\) = \(\frac{12}{-2}\) = -6
∴ a = 2 and b = 6 or a = -2 and b = -6

(v) \(\frac{1}{a+i b}\) = 3 – 2i
Solution:

(vi) (a + ib) (1 + i) = 2 + i
Solution:
(a + ib)(1 + i) = 2 + i
a + ai + bi + bi² = 2 + i
a + (a + b)i + b(-1) = 2 + i ……(∵ i² = -1)
(a – b) + (a + b)i = 2 + i
Equating real and imaginary parts, we get
a – b = 2 ……(i)
a + b = 1 …….(ii)
Adding equations (i) and (ii), we get
2a = 3
∴ a = \(\frac{3}{2}\)
Substituting a = \(\frac{3}{2}\) in (ii), we get
\(\frac{3}{2}\) + b = 1
∴ b = 1 – \(\frac{3}{2}\) = \(\frac{-1}{2}\)
∴ a = \(\frac{3}{2}\) and b = \(\frac{-1}{2}\)

Question 4.
Express the following in the form of a + ib, a, b ∈ R, i = √-1. State the values of a and b:
(i) (1 + 2i)(-2 + i)
Solution:
(1 + 2i)(-2 + i) = -2 + i – 4i + 2i²
= -2 – 3i + 2(-1) ……[∵ i² = -1]
∴ (1 + 2i)(-2 + i) = -4 – 3i
∴ a = -4 and b = -3

(ii) (1 + i)(1 – i)-1
Solution:

(iii) \(\frac{i(4+3 i)}{1-i}\)
Solution:

(iv) \(\frac{(2+i)}{(3-i)(1+2 i)}\)
Solution:

(v) \(\left(\frac{1+i}{1-1}\right)^{2}\)
Solution:

(vi) \(\frac{3+2 i}{2-5 i}+\frac{3-2 i}{2+5 i}\)
Solution:

(vii) (1 + i)^-3
Solution:

(viii) \(\frac{2+\sqrt{-3}}{4+\sqrt{-3}}\)
Solution:

(ix) (-√5 + 2√-4 ) + (1 – √-9 ) + (2 + 3i)(2 – 3i)
Solution:
(-√5 + 2√-4) + (1 – √-9) + (2 + 3i)(2 – 3i)
= (-√5 + 2√4.√-1) + (1 – √9.√-1) + 4 – 9i²
= [-√5 + 2(2)i] + (1 – 3i) + 4 – 9i²
= -√5 + 4i + 1 – 3i + 4 – 9(-1) ……[∵ i² = -1]
= (14 – √5) + i
∴ a = 14 – √5 and b = 1

(x) (2 + 3i)(2 – 3i)
Solution:
(2 + 3i)(2 – 3i)
= 4 – 9i²
= 4 – 9(-1) …[∵ i² = -1]
= 4 + 9
= 13
∴ (2 + 3i)(2 – 3i) = 13 + 0i
∴ a = 13 and b = 0

(xi) \(\frac{4 i^{8}-3 i^{9}+3}{3 i^{11}-4 i^{10}-2}\)
Solution:

Question 5.
Show that (-1 + √3i)³ is a real number.
Solution:

Question 6.
Find the value of (3 + \(\frac{2}{\mathrm{i}}\)) (i⁶ – i⁷) (1 + i¹¹).
Solution:

Question 7.
Evaluate the following:
(i) i³⁵
(ii) i⁸⁸⁸
(iii) i⁹³
(iv) i¹¹⁶
(v) i⁴⁰³
(vi) \(\frac{1}{i^{58}}\)
(vii) i^-888
(viii) i³⁰ + i⁴⁰ + i⁵⁰ + i⁶⁰
Solution:

Question 8.
Show that 1 + i¹⁰ + i²⁰ + i³⁰ is a real number.
Solution:

= 1 – 1 + 1 – 1
= 0, which is a real number.

Question 9.
Find the value of
(i) i⁴⁹ + i⁶⁸ + i⁸⁹ + i¹¹⁰
(ii) i + i² + i³ + i⁴
Solution:

Question 10.
Simplify: \(\frac{\mathbf{i}^{592}+\mathbf{i}^{590}+\mathbf{i}^{588}+\mathbf{i}^{586}+\mathbf{i}^{584}}{\mathbf{i}^{582}+\mathbf{i}^{580}+\mathbf{i}^{578}+\mathbf{i}^{576}+\mathbf{i}^{574}}\)
Solution:

Question 11.
Find the value of 1 + i² + i⁴ + i⁶ + i⁸ + …… + i²⁰.
Solution:

Question 12.
Show that 1 + i¹⁰ + i¹⁰⁰ – i¹⁰⁰⁰ = 0.
Solution:

Question 13.
Is (1 + i¹⁴ + i¹⁸ + i²²) a real number? Justify your answer.
Solution:

Question 14.
Evaluate: \(\left(\mathbf{i}^{37}+\frac{1}{\mathbf{i}^{67}}\right)\)
Solution:

Question 15.
Prove that: (1 + i)⁴ × \(\left(1+\frac{1}{\mathrm{i}}\right)^{4}\) = 16
Solution:

Question 16.
Find the value of \(\frac{\mathbf{i}^{6}+\mathbf{i}^{7}+\mathbf{i}^{8}+\mathbf{i}^{9}}{\mathbf{i}^{2}+\mathbf{i}^{3}}\)
Solution:

Question 17.
If a = \(\frac{-1+\sqrt{3} i}{2}\), b = \(\frac{-1-\sqrt{3} i}{2}\), then show that a² = b and b² = a.
Solution:

Question 18.
If x + iy = (a + ib)³, show that \(\frac{x}{a}+\frac{y}{b}\) = 4(a² – b²)
Solution:
x + iy = (a + ib)³
x + iy = a³ + 3a²bi + 3ab²i² + b³i³
x + iy = a³ + 3a²bi – 3ab² – b³i ……[∵ i² = -1, i³ = -i]
x + iy = (a³ – 3ab²) + (3a²b – b³)i
Equating real and imaginary parts, we get
x = a³ – 3ab² and y = 3a²b – b³
\(\frac{x}{a}\) = a² – 3b² and \(\frac{y}{b}\) = 3a² – b²
\(\frac{x}{a}+\frac{y}{b}\) = a² – 3b + 3a² – b²
\(\frac{x}{a}+\frac{y}{b}\) = 4a² – 4b²
\(\frac{x}{a}+\frac{y}{b}\) = 4(a² – b²)

Alternate Method:
x + iy = (a + ib)³
x + iy = a³ + 3a²bi + 3ab²i² + b³i³
x + iy = a³ + 3a²bi – 3ab² – b³i …..[∵ i² = -1, i³ = -i]
x + iy = (a³ – 3ab²) + (3a²b – b³)i
Equating real and imaginary parts, we get
x = a³ – 3ab² and y = 3a²b – b³
Consider

Question 19.
If \(\frac{a+3 i}{2+i b}\) = 1 – i, show that (5a – 7b) = 0.
Solution:
\(\frac{a+3 i}{2+i b}\) = 1 – i
a + 3i = (1 – i)(2 + ib)
= 2 + bi – 2i – bi²
= 2 + (b – 2)i – b(-1) ……[∵ i² = -1]
a + 3i = (2 + b) + (b – 2)i
Equating real and imaginary parts, we get
a = 2 + b and 3 = b – 2
a = 2 + b and b = 5
a = 2 + 5 = 7
5a – 7b = 5(7) – 7(5) = 35 – 35 = 0

Question 20.
If x + iy = \(\sqrt{\frac{a+i b}{c+i d}}\), prove that \(\left(x^{2}+y^{2}\right)^{2}=\frac{a^{2}+b^{2}}{c^{2}+d^{2}}\)
Solution:

Question 21.
If (a + ib) = \(\frac{1+i}{1-i}\), then prove that a² + b² = 1.
Solution:

∴ a + bi = 0 + i
Equating real and imaginary parts, we get
a = 0 and b = 1
a² + b² = 0² + 1² = 1

Question 22.
Show that \(\left(\frac{\sqrt{7}+i \sqrt{3}}{\sqrt{7}-i \sqrt{3}}+\frac{\sqrt{7}-i \sqrt{3}}{\sqrt{7}+i \sqrt{3}}\right)\) is real.
Solution:

Question 23.
If (x + iy)³ = y + vi, then show that \(\frac{y}{x}+\frac{v}{y}\) = 4(x² – y²).
Solution:

Question 24.
Find the values of x and y which satisfy the following equations (x, y ∈ R)
(i) (x + 2y) + (2x – 3y)i + 4i = 5
Solution:
(x + 2y) + (2x – 3y)i + 4i = 5
(x + 2y) + (2x – 3y)i = 5 – 4i
Equating real and imaginary parts, we get
x + 2y = 5 ……(i)
and 2x – 3y = -4 …..(ii)
Equation (i) x 2 – equation (ii) gives
7y = 14
∴ y = 2
Substituting y = 2 in (i), we get
x + 2(2) = 5
x + 4 = 5
∴ x = 1
∴ x = 1 and y = 2

Check:
For x = 1 and y = 2
Consider, L.H.S. = (x + 2y) + (2x – 3y)i + 4i
= (1 + 4) + (2 – 6)i + 4i
= 5 – 4i + 4i
= 5
= R.H.S.

(ii) \(\frac{x+1}{1+i}+\frac{y-1}{1-i}=i\)
Solution:

(x + y) + (y – x – 2)i = 2i
(x + y) + (y – x – 2)i = 0 + 2i
Equating real and imaginary parts, we get
x + y = 0 and y – x – 2 = 2
∴ x + y = 0 …….(i)
and -x + y = 4 …….(ii)
Adding (i) and (ii), we get
2y = 4
∴ y = 2
Substituting y = 2 in (i), we get
x + 2 = 0
∴ x = -2
∴ x = -2 and y = 2

(iii) \(\frac{x+i y}{2+3 i}+\frac{2+i}{2-3 i}=\frac{9}{13}(1+i)\)
Solution:

(2x + 3y + 1) + (8 – 3x + 2y)i = 9 + 9i
Equating real and imaginary parts, we get
2x + 3y + 1 = 9 and 8 – 3x + 2y = 9
2x + 3y = 8 ……(i)
and 3x – 2y = – 1 ……(ii)
Equation (i) × 2 + equation (ii) × 3 gives
13x = 13
∴ x = 1
Substituting x = 1 in (i), we get
2(1) + 3y = 8
3y = 6
∴ y = 2
∴ x = 1 and y = 2

(iv) If x(1 + 3i) + y(2 – i) – 5 + i³ = 0, find x + y
Solution:
x(1 + 3i) + y(2 – i) – 5 + i³ = 0
x + 3xi + 2y – yi – 5 – i = 0 ……[∵ i³ = -i]
(x + 2y – 5) + (3x – y – 1)i = 0 + 0i
Equating real and imaginary parts, we get
x + 2y – 5 = 0 …..(i)
and 3x – y – 1 = 0 ……(ii)
Equation (i) + equation (ii) × 2 gives
7x – 7 = 0
7x = 1
∴ x = 1
Substituting x = 1 in (i), we get
1 + 2y – 5 = 0
2y = 4
y = 2
∴ x = 1 and y = 2
∴ x + y = 1 + 2 = 3

(v) If x + 2i + 15i⁶y = 7x + i³(y + 4), find x + y
Solution:
x + 2i + 15i⁶y = 7x + i³(y + 4)
x + 2i + 15(i²)³ y = 7x + i³(y + 4)
x + 2i + 15(-1)³ y = 7x – i(y + 4) ……[∵ i² = -1, i³ = -i]
x + 2i – 15y – 7x + iy + 4i = 0
(-6x – 15y) + i(y + 6) = 0 + 0i
Equating real and imaginary parts, we get
-6x – 15y = 0 and y + 6 = 0
-6x – 15y = 0 and y = -6
-6x – 15(-6) = 0
-6x + 90 = 0
∴ x = 15
∴ x + y = 15 – 6 = 9

Balbharti Maharashtra State Board 11th Maths Book Solutions Pdf Chapter 2 Sequences and Series Ex 2.6 Questions and Answers.

Maharashtra State Board 11th Maths Solutions Chapter 2 Sequences and Series Ex 2.6

Question 1.
Find the sum \(\sum_{r=1}^{n}(r+1)(2 r-1)\).
Solution:

Question 2.
Find \(\sum_{r=1}^{n}\left(3 r^{2}-2 r+1\right)\)
Solution:

Question 3.
Find \(\sum_{r=1}^{n}\left(\frac{1+2+3 \ldots .+r}{r}\right)\)
Solution:

= \(\frac{n}{4}\) [(n + 1) + 2]
= \(\frac{n}{4}\) (n + 3)

Question 4.
Find \(\sum_{r=1}^{n}\left(\frac{1^{3}+2^{3}+\ldots . .+r^{3}}{r(r+1)}\right)\)
Solution:

Question 5.
Find the sum 5 × 7 + 9 × 11 + 13 × 15 + ….. upto n terms.
Solution:
5 × 7 + 9 × 11 + 13 × 15 + ….. upto n terms
Now, 5, 9, 13, … are in A.P. with
rth term = 5 + (r – 1) (4) = 4r + 1
7, 11, 15, ….. are in A.P. with
rth term = 7 + (r – 1) (4) = 4r + 3
∴ 5 × 7 + 9 × 11 + 13 × 15 + …… upto n terms

Question 6.
Find the sum 2² + 4² + 6² + 8² + ….. upto n terms.
Solution:

Question 7.
Find (70² – 69²) + (68² – 67²) + (66² – 65²) + …… + (2² – 1²)
Solution:
Let S = (70² – 69²) + (68² – 67²) + …… + (2² – 1²)
∴ S = (2² – 1²) + (4² – 3²) + ….. + (70² – 69²)
Here, 2, 4, 6,…, 70 are in A.P. with rth term = 2r
and 1, 3, 5, …,69 are in A.P. with rth term = 2r – 1

Question 8.
Find the sum 1 × 3 × 5 + 3 × 5 × 7 + 5 × 7 × 9 + …… + (2n – 1) (2n + 1) (2n + 3)
Solution:
Let S = 1 × 3 × 5 + 3 × 5 × 7 + ….. upto n terms
Here, 1, 3, 5, 7 … are in A.P. with rth term = 2r – 1,
3, 5, 7, 9,… are in A.P. with rth term = 2r + 1,
5, 7, 9, 11,… are in A.P. with rth term = 2r + 3

Question 9.
If \(\frac{1 \times 2+2 \times 3+3 \times 4+4 \times 5+\ldots \text { upto } n \text { terms }}{1+2+3+4+\ldots \text { upto } n \text { terms }}\) = \(\frac{100}{3}\), find n.
Solution:

Question 10.
If S₁, S₂ and S₃ are the sums of first n natural numbers, their squares and their cubes respectively, then show that 9\(\mathrm{S}_{2}{ }^{2}\) = S₃(1 + 8S₁).
Solution:

Balbharti Maharashtra State Board 11th Maths Book Solutions Pdf Chapter 7 Limits Ex 7.4 Questions and Answers.

Maharashtra State Board 11th Maths Solutions Chapter 7 Limits Ex 7.4

I. Evaluate the following limits:

Question 1.
\(\lim _{\theta \rightarrow 0}\left[\frac{\sin (m \theta)}{\tan (n \theta)}\right]\)
Solution:

Question 2.
\(\lim _{\theta \rightarrow 0}\left[\frac{1-\cos 2 \theta}{\theta^{2}}\right]\)
Solution:

Question 3.
\(\lim _{x \rightarrow 0}\left[\frac{x \cdot \tan x}{1-\cos x}\right]\)
Solution:

Question 4.
\(\lim _{x \rightarrow 0}\left(\frac{\sec x-1}{x^{2}}\right)\)
Solution:

II. Evaluate the following limits:

Question 1.
\(\lim _{x \rightarrow 0}\left[\frac{1-\cos (n x)}{1-\cos (m x)}\right]\)
Solution:

Question 2.
\(\lim _{x \rightarrow \frac{\pi}{6}}\left[\frac{2-{cosec} x}{\cot ^{2} x-3}\right]\)
Solution:

Question 3.
\(\lim _{x \rightarrow \frac{\pi}{4}}\left[\frac{\cos x-\sin x}{\cos 2 x}\right]\)
Solution:

III. Evaluate the following limits:

Question 1.
\(\lim _{x \rightarrow 0}\left[\frac{\cos (a x)-\cos (b x)}{\cos (c x)-1}\right]\)
Solution:

Question 2.
\(\lim _{x \rightarrow \pi}\left[\frac{\sqrt{1-\cos x}-\sqrt{2}}{\sin ^{2} x}\right]\)
Solution:

Question 3.
\(\lim _{x \rightarrow \frac{\pi}{4}}\left[\frac{\tan ^{2} x-\cot ^{2} x}{\sec x-{cosec} x}\right]\)
Solution:

Question 4.
\(\lim _{x \rightarrow \frac{\pi}{6}}\left[\frac{2 \sin ^{2} x+\sin x-1}{2 \sin ^{2} x-3 \sin x+1}\right]\)
Solution:

Balbharti Maharashtra State Board 11th Maths Book Solutions Pdf Chapter 3 Permutations and Combination Ex 3.2 Questions and Answers.

Maharashtra State Board 11th Maths Solutions Chapter 3 Permutations and Combination Ex 3.2

Question 1.
Evaluate:
(i) 8!
Solution:
8!
= 8 × 7 × 6 × 5 × 4 × 3 × 2 × 1
= 40320

(ii) 10!
Solution:
10!
= 10 × 9 × 8 × 7 × 6 × 5 × 4 × 3 × 2 × 1
= 3628800

(iii) 10! – 6!
Solution:
10! – 6!
= 10 × 9 × 8 × 7 × 6! – 6!
= 6! (10 × 9 × 8 × 7 – 1)
= 6! (5040 – 1)
= 6 × 5 × 4 × 3 × 2 × 1 × 5039
= 3628080

(iv) (10 – 6)!
Solution:
(10 – 6)!
= 4!
= 4 × 3 × 2 × 1
= 24

Question 2.
Compute:
(i) \(\frac{12 !}{6 !}\)
Solution:
\(\frac{12 !}{6 !}=\frac{12 \times 11 \times 10 \times 9 \times 8 \times 7 \times 6 !}{6 !}\)
= 12 × 11 × 10 × 9 × 8 × 7
= 665280

(ii) \(\left(\frac{12}{6}\right) !\)
Solution:
\(\left(\frac{12}{6}\right) !\)
= 2!
= 2 × 1
= 2

(iii) (3 × 2)!
Solution:
(3 × 2)!
= 6!
= 6 × 5 × 4 × 3 × 2 × 1
= 720

(iv) 3! × 2!
Solution:
3! × 2!
= 3 × 2 × 1 × 2 × 1
= 12

(v) \(\frac{9 !}{3 ! 6 !}\)
Solution:
\(\frac{9 !}{3 ! 6 !}=\frac{9 \times 8 \times 7 \times 6 !}{(3 \times 2 \times 1) \times 6 !}=84\)

(vi) \(\frac{6 !-4 !}{4 !}\)
Solution:
\(\frac{6 !-4 !}{4 !}=\frac{6 \times 5 \times 4 !-4 !}{4 !}=\frac{4 !(6 \times 5-1)}{4 !}=29\)

(vii) \(\frac{8 !}{6 !-4 !}\)
Solution:
\(\frac{8 !}{6 !-4 !}=\frac{8 \times 7 \times 6 \times 5 \times 4 !}{6 \times 5 \times 4 !-4 !}\)
= \(\frac{8 \times 7 \times 6 \times 5 \times 4 !}{4 !(6 \times 5-1)}\)
= \(\frac{1680}{29}\)
= 57.93

(viii) \(\frac{8 !}{(6-4) !}\)
Solution:
\(\frac{8 !}{(6-4) !}=\frac{8 !}{2 !}\)
= \(\frac{8 \times 7 \times 6 \times 5 \times 4 \times 3 \times 2 !}{2 !}\)
= 20160

Question 3.
Write in terms of factorials
(i) 5 × 6 × 7 × 8 × 9 × 10
Solution:
5 × 6 × 7 × 8 × 9 × 10 = 10 × 9 × 8 × 7 × 6 × 5
Multiplying and dividing by 4!, we get
= \(\frac{10 \times 9 \times 8 \times 7 \times 6 \times 5 \times 4 !}{4 !}\)
= \(\frac{10 \times 9 \times 8 \times 7 \times 6 \times 5 \times 4 \times 3 \times 2 \times 1}{4 !}\)
= \(\frac{10 !}{4 !}\)

(ii) 3 × 6 × 9 × 12 × 15
Solution:
3 × 6 × 9 × 12 × 15
= 3 × (3 × 2) × (3 × 3) × (3 × 4) × (3 × 5)
= (3⁵) (5 × 4 × 3 × 2 × 1)
= 3⁵ (5!)

(iii) 6 × 7 × 8 × 9
Solution:
6 × 7 × 8 × 9 = 9 × 8 × 7 × 6
Multiplying and dividing by 5!, we get
= \(\frac{9 \times 8 \times 7 \times 6 \times 5 !}{5 !}\)
= \(\frac{9 \times 8 \times 7 \times 6 \times 5 \times 4 \times 3 \times 2 \times 1}{5 !}\)
= \(\frac{9 !}{5 !}\)

(iv) 5 × 10 × 15 × 20
Solution:
5 × 10 × 15 × 20
= (5 × 1) × (5 × 2) × (5 × 3) × (5 × 4)
= (5⁴) (4 × 3 × 2 × 1)
= (5⁴) (4!)

Question 4.
Evaluate: \(\frac{n !}{r !(n-r) !}\) for
(i) n = 8, r = 6
(ii) n = 12, r = 12
(iii) n = 15, r = 10
(iv) n = 15, r = 8
Solution:

Question 5.
Find n, if
(i) \(\frac{n}{8 !}=\frac{3}{6 !}+\frac{1 !}{4 !}\)
Solution:

(ii) \(\frac{n}{6 !}=\frac{4}{8 !}+\frac{3}{6 !}\)
Solution:

(iii) \(\frac{1 !}{n !}=\frac{1 !}{4 !}-\frac{4}{5 !}\)
Solution:

(iv) (n + 1)! = 42 × (n -1)!
Solution:
(n + 1)! = 42(n – 1)!
∴ (n + 1) n (n – 1)! = 42(n – 1)!
∴ n² + n = 42
∴ n² + n – 42 = 0
∴ (n + 7)(n – 6) = 0
∴ n = -7 or n = 6
But n ≠ -7 as n ∈ N
∴ n = 6

(v) (n + 3)! = 110 × (n + 1)!
Solution:
(n + 3)! = (110) (n + 1)!
∴ (n + 3)(n + 2)(n + 1)! = 110(n + 1)!
∴ (n + 3) (n + 2) = (11) (10)
Comparing on both sides, we get
n + 3 = 11
∴ n = 8

Question 6.
Find n, if:
(i) \(\frac{(17-n) !}{(14-n) !}=5 !\)
Solution:

∴ (17 – n) (16 – n) (15 – n) = 6 × 5 × 4
Comparing on both sides, we get
17 – n = 6
∴ n = 11

(ii) \(\frac{(15-n) !}{(13-n) !}=12\)
Solution:
\(\frac{(15-n) !}{(13-n) !}=12\)
∴ \(\frac{(15-n)(14-n)(13-n) !}{(13-n) !}=12\)
∴ (15 – n) (14 – n) = 4 × 3
Comparing on both sides, we get
∴ 15 – n = 4
∴ n = 11

(iii) \(\frac{n !}{3 !(n-3) !}: \frac{n !}{5 !(n-5) !}=5: 3\)
Solution:

∴ 12 = (n – 3)(n – 4)
(n – 3)(n – 4) = 4 × 3
Comparing on both sides, we get
n – 3 = 4
∴ n = 7

(iv) \(\frac{n !}{3 !(n-3) !}: \frac{n !}{5 !(n-7) !}=1: 6\)
Solution:

∴ 120 = (n – 3)(n – 4) (n – 5)(n – 6)
∴ (n – 3)(n – 4) (n – 5)(n – 6) = 5 × 4 × 3 × 2
Comparing on both sides, we get
n – 3 = 5
∴ n = 8

(v) \(\frac{(2 n) !}{7 !(2 n-7) !}: \frac{n !}{4 !(n-4) !}=24: 1\)
Solution:


(2n – 1)(2n – 3)(2n – 5) = \(\frac{24 \times 7 \times 6 \times 5}{16}\)
∴ (2n – 1)(2n – 3)(2n – 5) = 9 × 7 × 5
Comparing on both sides. We get
∴ 2n – 1 = 9
∴ n = 5

Question 7.
Show that \(\frac{n !}{r !(n-r) !}+\frac{n !}{(r-1) !(n-r+1) !}=\frac{(n+1) !}{r !(n-r+1) !}\)
Solution:

Question 8.
Show that \(\frac{9 !}{3 ! 6 !}+\frac{9 !}{4 ! 5 !}=\frac{10 !}{4 ! 6 !}\)
Solution:

Question 9.
Show that \(\frac{(2 n) !}{n !}\) = 2n (2n – 1)(2n – 3)…5.3.1
Solution:

Question 10.
Simplify
(i) \(\frac{(2 n+2) !}{(2 n) !}\)
Solution:

(ii) \(\frac{(n+3) !}{\left(n^{2}-4\right)(n+1) !}\)
Solution:

(iii) \(\frac{1}{n !}-\frac{1}{(n-1) !}-\frac{1}{(n-2) !}\)
Solution:

(iv) n[n! + (n – 1)!] + n²(n – 1)! + (n + 1)!
Solution:

(v) \(\frac{n+2}{n !}-\frac{3 n+1}{(n+1) !}\)
Solution:

(vi) \(\frac{1}{(n-1) !}+\frac{1-n}{(n+1) !}\)
Solution:

(vii) \(\frac{1}{n !}-\frac{3}{(n+1) !}-\frac{n^{2}-4}{(n+2) !}\)
Solution:

(viii) \(\frac{n^{2}-9}{(n+3) !}+\frac{6}{(n+2) !}-\frac{1}{(n+1) !}\)
Solution: