Maharashtra Board Class 11 Chemistry Important Questions Chapter 2 Introduction to Analytical Chemistry

Balbharti Maharashtra State Board 11th Chemistry Important Questions Chapter 2 Introduction to Analytical Chemistry Important Questions and Answers.

Maharashtra State Board 11th Chemistry Important Questions Chapter 2 Introduction to Analytical Chemistry

Question 1.
Explain the statement: Analytical chemistry provides physical or chemical information about a sample.
Answer:

• Analytical chemistry facilitates the investigation of the chemical composition of substances.
• It uses the instruments and methods to separate, identify and quantify a sample under study.

Thus, analytical chemistry provides chemical or physical information about a sample.

Question 2.
What is the difference between qualitative analysis and quantitative analysis?
Answer:

1. Qualitative analysis deals with the detection of the presence or absence of elements in compounds and of chemical compounds in mixtures.
2. Quantitative analysis deals with the determination of the relative proportions of elements in compounds and of chemical compounds in mixtures.

Question 3.
Explain the importance of chemical analysis.
Answer:

• Chemical analysis is one of the most important methods of monitoring the composition of raw materials, intermediates and finished products, and also the composition of air in streets and premises of industrial plants.
• In agriculture, chemical analysis is used to determine the composition of soils and fertilizers.
• In medicine, it is used to determine the composition of medicinal preparations.

Question 4.
Write a note on the applications of analytical chemistry.
Answer:

• Analytical chemistry has applications in forensic science, engineering and industry.
• Analytical chemistry is also useful in the field of agriculture and pharmaceutical industry.
• Industrial process as a whole and the production of new kinds of materials are closely associated with analytical chemistry.

Question 5.
What is semi-microanalysis?
Answer:

• When the amount of a solid or liquid sample taken for analysis is a few grams, the analysis is called semi-microanalysis.
• It is of two types: qualitative and quantitative analysis.

Question 6.
What does classical qualitative analysis method include?
Answer:
Classical qualitative analysis method includes separation and identification of compounds.

• Separations may be done by methods such as precipitation, extraction and distillation.
• Identification may be based on differences in colour, odour, melting point, boiling point, and reactivity.

Question 7.
Name two methods of classical quantitative analysis.
Answer:

1. Volumetric analysis (Titrimetric analysis)
2. Gravimetric analysis (i.e., decomposition, precipitation)

Question 8.
What are the two stages involved in the chemical analysis of a sample?
Answer:
The chemical analysis of a sample is carried out mainly in two stages: by the dry method and by the wet method. In dry method, the sample under test is not dissolved and in wet method, the sample under test is first dissolved and then analysed to determine its composition.

Question 9.
Explain: Qualitative analysis of organic compounds
Answer:

• The majority of organic compounds are composed of a relatively small number of elements.
• The most important ones are: carbon, hydrogen, oxygen, nitrogen, sulphur, halogen, phosphorus.
• Elementary qualitative analysis is concerned with the detection of the presence of these elements.
• The identification of an organic compound involves tests such as detection of functional group, determination of melting/boiling points, etc.

Question 10.
What does qualitative analysis of inorganic compounds involve?
Answer:
The qualitative analysis of simple inorganic compounds involves detection and confirmation of cationic and anionic species (basic and acidic radical) in them.

Question 11.
Explain: Chemical methods of quantitative analysis
Answer:

• Quantitative analysis of organic compounds involves methods such as determination of percentage of constituent elements, concentrations of a known compound in the given sample, etc.
• Quantitative analysis of simple inorganic compounds involves methods such as gravimetric analysis (i.e., decomposition, precipitation, etc.) and the titrimetric or volumetric analysis (i.e., progress of reaction between two solutions till its completion).
• The quantitative analytical methods involve measurement of quantities such as mass and volume using some equipment/apparatus such as weighing machine, burette, etc.

Question 12.
Why is accurate measurement crucial in science?
Answer:

• The accuracy of measurement is of a great concern in analytical chemistry. This is because faulty equipment, poor data processing or human error can lead to inaccurate measurements. Also, there can be intrinsic errors in the analytical measurement.
• When measurements are not accurate, this provides incorrect data that can lead to wrong conclusions. For example, if a laboratory experiment requires a specific amount of a chemical, then measuring the wrong amount may result in an unsafe or unexpected outcome.
• Hence, the numerical data obtained experimentally are treated mathematically to reach some quantitative conclusion.
• Also, an analytical chemist has to know how to report the quantitative analytical data, indicating the extent of the accuracy of measurement, perform the mathematical operation and properly express the quantitative error in the result.

Question 13.
Why are scientific notations (exponential notations) used?
Answer:
A chemist has to deal with numbers as large as 602,200,000,000,000,000,000,000 for the molecules of 2 g of hydrogen gas or as small as 0.00000000000000000000000166 g. that is, mass of a H atom. To avoid the writing of so many zeros in mathematical operations, scientific notations i.e. exponential notations are used.

Question 14.
How are numbers expressed in scientific notations (exponential notations)?
Answer:
In scientific notations, numbers are expressed in the form of N × 10ⁿ, where ‘n’ is an exponent with positive or negative values and N can have a value between 1 to 10.
e.g. i. The number, 602,200,000,000,000,000,000,000 is expressed as 6.022 × 10²³.
ii. The mass of a H atom, 0.00000000000000000000000166 g is expressed as 1.66 × 10^-24 g.
iii. The number 123.546 is written as 1.23546 × 10².
iv. The number 0.00015 is written as 1.5 × 10^-4.

Question 15.
Express the following quantities in scientific notations (exponential notations).
i. 0.0345
ii . 0.08
iii. 653.00
iv. 34.768
Answer:
i. 0.0345 = 3.45 × 10^-2
ii. 0.08 = 8 × 10^-2
iii. 653.00 = 6.5300 × 10²
iv. 34.768 = 3.4768 × 10¹

Question 16.
Define: Accuracy of measurement
Answer:
Nearness of the measured value to the true value is called the accuracy of measurement.

Question 17.
Explain with the help of a diagram how accuracy depends upon the sensitivity or least count of the measuring equipment.
Answer:
A burette reading of 10.2 mL is as shown in the diagram below:

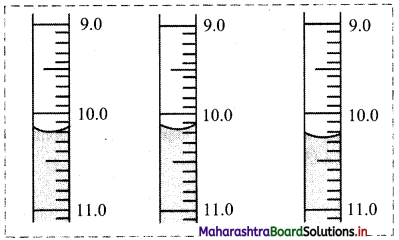
• For all the three situations in the above figure, the reading would be noted is 10.2 mL.
• It means that there is an uncertainty about the digit appearing after the decimal point in the reading 10.2 mL because the least count of the burette is 0.1 mL.
• The meaning of the reading 10.2 mL is that the true value of the reading lies between 10.1 mL and 10.3 mL.
• This is indicated by writing 10.2 ± 0.1 mL.
• Here, the burette reading has an error of ± 0.1 mL.
• Smaller the error, higher is the accuracy.

Question 18.
How is absolute error calculated?
Answer:
Absolute error is calculated by subtracting true value from observed value.
Absolute error = Observed value – Tme value

Question 19.
Explain the term: Relative error
Answer:

1. Relative error is the ratio of an absolute error to the true value.
2. Relative error is generally a more useful quantity than absolute error.
3. Relative error is expressed as a percentage and can be calculated as follows:
   Relative error = \(\frac{\text { Absolute error }}{\text { True value }} \times 100 \%\)

Question 20.
Explain the term: Precision in measurement
Answer:

• Multiple readings of the same quantity are noted to minimize the error.
• If the multiple readings of the same quantity match closely, they are said to have high precision.
• High precision implies reproducibility of the readings.
• High precision is a prerequisite for high accuracy.
• Precision is expressed in terms of deviation (i.e. absolute deviation and relative deviation).

Question 21.
Explain the following terms with respect to precise measurement:
i. Absolute deviation
ii. Mean absolute deviation
iii. Relative deviation
Answer:
i. Absolute deviation: An absolute deviation is the modulus of the difference between an observed value and the arithmetic mean for the set of several measurements made in the same way. It is a measure of absolute error in the repeated observation. It is expressed as follows:
Absolute deviation = |Observed value – Mean|

ii. Mean absolute deviation: Arithmetic mean of all the absolute deviations is called the mean absolute deviation in the measurements.

iii. Relative deviation: The ratio of mean absolute deviation to its arithmetic mean is called relative deviation. It is expressed as follows:
Relative deviation = \(\frac{\text { Mean absolute deviation }}{\text { Mean }}\) × 100%

Question 22.
Explain the need of significant figures in measurement.
Answer:

• Uncertainty in measured value leads to uncertainty in calculated result.
• Uncertainty in a value is indicated by mentioning the number of significant figures in that value. e.g. Consider, the column reading 10.2 ± 0.1 mL recorded on a burette having the least count of 0.1 mL. Here, it is said that the last digit ‘2’ in the reading is uncertain, its uncertainty is ±0.1 mL. On the other hand, the figure ‘10’ is certain.
• The significant figures in a measurement or result are the number of digits known with certainty plus one uncertain digit.
• In a scientific experiment, a result is obtained by doing calculation in which values of a number of quantities measured with equipment of different least counts are used.

Question 23.
How many significant figures are present in the following measurements?
i. 4.065 m
ii. 0.32 g
iii. 57.98 cm³
iv. 0.02 s
v. 4.0 × 10^-4 km
vi. 604.0820 kg
vii. 307.100 × 10^-5 cm
Answer:
i. 4
ii. 2
iii. 4
iv. 1
v. 2
vi. 7
vii. 6

Question 24.
How many significant figures are present in each of the following?
i. 45.0
ii. 0.001
iii. 2.10 × 10^-8
iv. 340000
v. 0.0100
vi. 7890320
vii. 100.00
viii. 100
Answer:
i. 3
ii. 1
iii. 3
iv. 2
v. 3
vi. 6
vii. 5
viii. 1

Question 25.
State the rules used to round off a number to the required number of significant figures.
Answer:
The following rules are used to round off a number to the required number of significant figures:

• If the digit following the last digit to be kept is less than five, the last digit is left unchanged, e.g. 46.32 rounded off to two significant figures is 46.
• If the digit following the last digit to be kept is five or more, the last digit to be kept is increased by one. e.g. 52.87 rounded to three significant figures is 52.9.

Question 26.
Round off each of the following to the number of significant digits indicated:
i. 1.223 to two digits
ii. 12.56 to three digits
iii. 122.17 to four digits
iv. 231.5 to three digits
Answer:
i. 1.223 to two digits = 1.2
This is because the third digit is less than 5, so we drop it and all the other digits to its right.
ii. 12.56 to three digits = 12.6
This is because the fourth digit is greater than 5, so we drop it and add 1 to the third digit.
iii. 122.17 to four digits = 122.2
This is because the fifth digit is greater than 5, so we drop it and add 1 to the fourth digit.
iv. 231.5 to three digits = 232
This is because the fourth digit is 5, so we drop it and add 1 to the third digit.

Question 27.
Add 5.55 × 10⁴ and 6.95 × 10³ and express the result in scientific notation.
Solution:
To perform addition operation, first the numbers are written in such a way that they have the same exponent. The coefficients are then added.
(5.55 × 10⁴) + (6.95 × 10³)= (5.55 × 10⁴) + (0.695 × 10⁴)
= (5.55 +0.695) × 10⁴
= 6.245 × 10⁴

Question 28.
Add 1.77 × 10² and 2.23 × 10³ and express the result in scientific notation.
Solution:
To perform addition operation, first the numbers are written in such a way that they have the same exponent. The coefficients are then added.
(1.77 × 10²) + (2.23 × 10³) = (0.177 × 10³) + (2.23 × 10³)
= (0.177 + 2.23) × 10³
= 2.407 × 10³

Question 29.
Subtract 5.8 × 10^-3 from 3.5 × 10^-2 and express result in scientific notation.
Solution:
To perform subtraction operation, first the numbers are written in such a way that they have the same exponent. Then subtraction of coefficients can be done.
(3.5 × 10^-2)- (5.8 × 10^-3) = (3.5 × 10^-2) – (0.58 × 10^-2)
= (3.5 – 0.58) × 10^-2
= 2.92 × 10^-2

Question 30.
Subtract 6.90 × 10^-5 from 5.11 × 10^-4 and express the result in scientific notation.
Solution:
To perform subtraction operation, first the numbers are written in such a way that they have the same exponent. Then subtraction of coefficients can be done.
(5.11 × 10^-4) – (6.90 × 10^-5) = (5.11 × 10^-4) – (0.690 × 10^-4)
= (5.11 – 0.690) × 10^-4
= 4.42 × 10^-4

Question 31.
Perform following calculations and express results in scientific notations (exponential notations),
i. (1.5 × 10^-6) – (5.8 × 10^-7)
ii. (9.8 × 10^-3) – (8.8 × 10^-3)
iii. (6.5 × 10^-8) – (5.5 × 10^-9)
Solution:
To perform subtraction operation, first the numbers are written in such a way that they have the same exponent. Then subtraction of coefficients can be done.
i. (1.5 × 10^-6) – (5.8 × 10^-7) = (1.5 × 10^-6) – (0.58 × 10^-6)
= (1.5 – 0.58) × 10^-6
= 0.92 × 10^-6
= 9.2 × 10^-7

ii. (9.8 × 10^-3) – (8.8 × 10^-3) = (9.8 – 8.8) × 10^-3
= 1.0 × 10^-3

iii. (6.5 × 10^-8) – (5.0 × 10^-9) = (6.5 × 10^-8) – (0.50 × 10^-8)
= (6.5 – 0.50) × 10^-8
= 6.0 × 10^-8

Question 32.
Multiply 5.6 × 10⁵ and 6.9 × 10⁸ and express result in scientific notation.
Solution:
(5.6 × 10⁵) × (6.9 × 10⁸) = (5.6 × 6.9) (10⁵⁺⁸)
= 38.64 × 10¹³
= 3.864 × 10¹⁴

Question 33.
Multiply 9.8 × 10^-2 and 2.5 × 10^-6 and express result in scientific notation.
Solution:
(9.8 × 10^-2) × (2.5 × 10^-6) = (9.8 × 2.5) (10^-2+(-6))
= (9.8 × 2.5) × (10^-2-6)
= 24.5 × 10^-8
= 2.45 × 10^-7

Question 34.
Perform following calculations and express results in scientific notations (exponential notations).
i. (2.5 × 10^-6) × (1.8 × 10^-7)
ii. (4.5 × 10^-3) × (1.8 × 10³)
iii. (8.5 × 10⁷) × (3.5 × 10⁹)
Solution:
i. (2.5 × 10^-6) × (1.8 × 10^-7) = (2.5 × 1.8) (10^-6+(-7))
= (2.5 × 1.8) × (10^-6-7)
= 4.5 × 10^-13

ii. (4.5 × 10-3) × (1.8 × 103) = (4.5 × 1.8) (10^-3+3)
= (4.5 × 1.8) × (10⁰)
= 8.1

iii. (8.5 × 10⁷) × (3.5 × 10⁹) = (8.5 × 3.5) (10⁷⁺⁹)
= (8.5 × 3.5) × 10¹⁶
= 29.75 × 10¹⁶
= 2.975 × 10¹⁷
[Note: To express number in scientific notation, the number has to be greater than or equal to 10 or less than 1. The number, 8.1 is greater than 1, but less than 10 and hence, it cannot be expressed in scientific notation.]

Question 35.
In laboratory experiment, 10 g potassium chlorate sample on decomposition gives following data: The sample contains 3.8 g of oxygen and the actual mass of oxygen in the quantity of potassium chlorate is 3.92 g. Calculate absolute error and relative error.
Solution:
The observed value is 3.8 g and accepted (true) value is 3.92 g.
i. Absolute error = Observed value – True value
= 3.8 – 3.92
= -0.12 g

[Note: The negative sign indicates that experimental result is lower than the true value.]
Ans: i. Absolute error = -0.12 g
ii. Relative error = -3.06%

Question 36.
12.15 g of magnesium gives 20.20 g of magnesium oxide on burning. The actual mass of magnesium oxide that should be produced is 20.15 g. Calculate absolute error and relative error.
Solution:
The observed value is 20.20 g and accepted (true) value is 20.15 g.
i. Absolute error = Observed value – True value
= 20.20 – 20.15
= 0.05 g

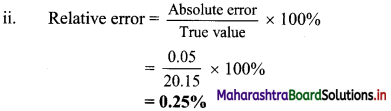
Ans: i. Absolute error = 0.05 g
ii. Relative error = 0.25 %

Question 37.
The three identical samples of potassium chlorate are decomposed. The mass of oxygen is determined to be 3.87 g, 3.95 g and 3.89 g for the set. Calculate absolute deviation and relative deviation.
Solution:
Mean = \(\frac{3.87+3.95+3.89}{3}\) = 3.90

Sample	Mass of oxygen	Absolute deviation = | Observed value – Mean |
1	3.87 g	0.03 g
2	3.95 g	0.05 g
3	3.89 g	0.01 g

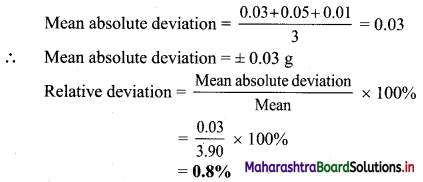
Ans: i. Absolute deviation in each observation = 0.03 g, 0.05 g, 0.01 g
Relative deviation = 0.8%

Question 38.
In repeated measurements in volumetric analysis, the end-points were observed as 11.15 mL, 11.17 mL, 11.11 mL and 11.17 mL. Calculate mean absolute deviation and relative deviation.
Solution:
Mean = \(\frac{11.15+11.17+11.11+11.17}{4}\) = 11.15

Measurement	End-point	Absolute deviation = |Observed value – Mean|
1	11.15 mL	0
2	11.17 mL	0.02 mL
3	11.11 mL	0.04 mL
4	11.17 mL	0.02 mL

Ans: i. Mean absolute deviation = ±0.02 mL
ii. Relative deviation = 0.2%

Question 39.
Calculate the mass percentages of H, P and O in phosphoric acid if atomic masses are H = 1, P = 31 and O = 16.
Solution:
Atomic mass of H = 1, P = 31 and 0=16
The mass percentage of hydrogen, phosphorus, oxygen in H₃PO₄
Formula:

Calculation: Molecular formula of phosphoric acid: H₃PO₄
∴ Molar mass of H₃PO₄ = 3 × (1) + 1 × (31) + 4 × (16)
= 3 + 31 + 64
= 98 g mol^-1
Percentage of H = \(\frac {3}{98}\) × 100 = 3.06%
Percentage of P = \(\frac {31}{98}\) × 100 = 31.63%
Percentage of O = \(\frac {64}{98}\) × 100 = 65.31%
Ans: Mass percentage of H, P and O in phosphoric acid are 3.06%, 31.63% and 65.31% respectively.

Question 40.
Calculate the percentage composition of the elements in HNO₃ (H = 1, N = 14, O = 16).
Solution:
Atomic mass of H = 1, N = 14 and O = 16
The mass percentage of H, N and O in HNO₃
Formula:

Calculation: Molecular formula of HNO₃
∴ Molar mass = 1 × (1) + 1 × (14) + 3 × (16) = 1 + 14 + 48 = 63 g mol^-1
∴ Percentage of H = \(\frac {1}{63}\) × 100 = 1.59%
Percentage of N = \(\frac {14}{63}\) × 100 = 22.22%
Percentage of O = \(\frac {48}{63}\) × 100 = 76.19%
Ans: Mass percentage of H, N and O in HNO₃ are 1.59%, 22.22% and 76.19% respectively.

Question 41.
A compound contains 4.07% hydrogen, 24.27% carbon and 71.65% chlorine by mass. Its molar mass is 98.96 g mol^-1. What is its empirical formula and molecular formula? Atomic masses of hydrogen, carbon and chlorine are 1.008,12.000 and 35.453 u, respectively
Solution:
Given: Percentage of H, C and Cl = 4.07% , 24.27% and 71.65% by mass respectively.
To find: Empirical formula and molecular formula
Calculation: Step I:
Check whether the sum of all the percentages is 100.
4.07 + 24.27 + 71.65 = 99.99 ≈ 100
Therefore, no need to consider presence of oxygen atom in the molecule.

Step II:
Conversion of mass percent to grams. Since we are having mass percent, it is convenient to use 100 g of the compound as the starting material. Thus, in the 100 g sample of the above compound, 4.07 g hydrogen 24.27 g carbon and 71.65 g chlorine are present.

Step III:
Convert into number of moles of each element. Divide the masses obtained above by respective atomic masses of various elements.
Moles of hydrogen = \(\frac{4.07 \mathrm{~g}}{1.008 \mathrm{~g}}\) = 4.04 mol
Moles of carbon = \(\frac{24.27 \mathrm{~g}}{12.000 \mathrm{~g}}\) = 2.0225 mol
Moles of chlorine = \(\frac{71.65 \mathrm{~g}}{35.453 \mathrm{~g}}\) = 2.021 mol

Steps IV:
Divide the mole values obtained above by the smallest value among them.

Hence, the ratio of number of moles of 2 : 1 : 1 for H : C : Cl.
In case the ratios are not whole numbers, then they may be converted into whole number by multiplying by the suitable coefficient.

Step V:
Write empirical formula by mentioning the numbers after writing the symbols of respective elements. CH₂Cl is thus, the empirical formula of the above compound.

Step VI:
Writing molecular formula
a. Determine empirical formula mass: Add the atomic masses of various atoms present in the empirical formula.
For CH₂Cl, empirical formula mass = 12.000 + 2 × 1.008 + 35.453 = 49.469 g mol^-1
b. Divide molar mass by empirical formula mass

c. Multiply empirical formula by r obtained above to get the molecular formula:
Molecular formula = r × empirical formula
∴ Molecular formula is 2 × CH₂Cl i.e. C₂H₄Cl₂.
Ans: The empirical formula of the compound is CH₂Cl and the molecular formula of the compound is C₂H₄Cl₂.
[Note: The question is modified to include the determination of molecular formula of the compound.]

Question 42.
A compound with molar mass 159 was found to contain 39.62% copper and 20.13% sulphur. Suggest molecular formula for the compound (Atomic masses: Cu = 63, S = 32 and O = 16).
Solution:
Given: Atomic mass of Cu = 63, S = 32, and O = 16
Percentage of copper and sulphur = 39.62% and 20.13% respectively.
To find: The molecular formula of the compound
Calculation: % copper + % sulphur = 39.62 + 20.13 = 59.75
This is less than 100 %. Hence, compound contains adequate oxygen so that the total percentage of elements is 100%.
Hence, % of oxygen = 100 – 59.75 = 40.25%

Hence, empirical formula is CuSO₄.
Empirical formula mass = 63 + 32 +16 × 4 = 159 g mol^-1
Hence,
Molar mass = Empirical formula mass
∴ Molecular formula = Empirical formula = CuSO₄
Ans: Molecular formula of the compound = CuSO₄

Question 43.
An inorganic compound contained 24.75% potassium and 34.75% manganese and some other common elements. Give the empirical formula of the compound. (K = 39 u, Mn = 54.9 u, O = 16 u)
Solution:
Given: Atomic mass of K = 39 u, Mn = 59 u, and O = 16 u.
Percentage of potassium and manganese = 24.75 % and 34.75% respectively
To find: The empirical formula of the given inorganic compound
Calculation: Percentage of potassium = 24.75%
Percentage of manganese = 34.75%
Total percentage = 59.50%
∴ Remaining must be that of oxygen
∴ Percentage of oxygen = 100 – 59.50 = 40.50%
Moles of K = \(\frac{\% \text { of } \mathrm{K}}{\text { Atomic mass of } \mathrm{K}}=\frac{24.75}{39}\) = 0.635 mol

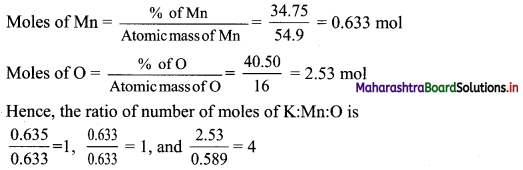
Empirical formula = KMnO₄
Ans: The empirical formula of given inorganic compound is KMnO₄.

Question 44.
An organic compound contains 40.92% carbon by mass, 4.58% hydrogen and 54.50% oxygen. Determine the empirical formula of the compound.
Solution:
Given: Percentage mass of carbon = 40.92%; Percentage mass of hydrogen = 4.58%
Percentage mass of oxygen = 54.50%
To find: The empirical formula of compound
Calculation:

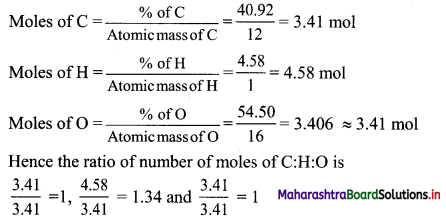
∴ Ratio = 1 : 1.34 : 1
Multiply by 3 to get whole number
∴ Ratio = 3 : 4.02 : 3 ≈ 3 : 4 : 3
∴ The empirical formula of the compound is C₃H₄O₃.
Ans: Empirical formula of the compound is C₃H₄O₃.

Question 45.
Define stoichiometric calculations.
Answer:
Calculations based on a balanced chemical equation are known as stoichiometric calculations.

Question 46.
Give reason: Balanced chemical equation is useful in solving problems based on chemical equations.
Answer:
Balanced chemical equation is symbolic representation of a chemical reaction. It provides the following information, which is useful in solving problems based on chemical equations:

• It indicates the number of moles of the reactants involved in a chemical reaction and the number of moles of the products formed.
• It indicates the relative masses of the reactants and products linked with a chemical change, and
• It indicates the relationship between the volume/s of the gaseous reactants and products, at STP.

Hence, balanced chemical equation is useful in solving problems based on chemical equations.

Question 47.
What are different types of stoichiometric problems? Write steps involved in solving stoichiometric problems.
Answer:
i. Generally, problems based on stoichiometry are of the following types:

• Problems based on mass-mass relationship
• Problems based on mass-volume relationship
• Problems based on volume-volume relationship.

ii. Steps involved in problems based on stoichiometric calculations:

• Write down the balanced chemical equation representing the chemical reaction.
• Write the number of moles and the relative masses or volumes of the reactants and products below the respective formulae.
• Relative masses or volumes should be calculated from the respective formula mass referring to the condition of STP.
• Apply the unitary method to calculate the unknown factors) as required by the problem.

Question 48.
Calculate the mass of carbon dioxide and water formed on complete combustion of 24 g of methane gas. (Atomic masses, C = 12 u, H = 1 u, O = 16 u)
Solution:
Mass of methane consumed in reaction = 24 g
Atomic mass: C = 12 u, H = 1 u, O = 16 u
To find: Mass of carbon dioxide and water formed
Calculation: The balanced chemical equation is,

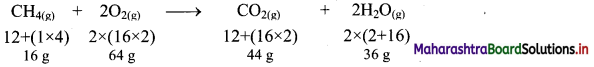
Hence, 16 g of CH₄ on complete combustion will produce 44 g of CO₂.
∴ 24 g of CH₄ = \(\frac {24}{16}\) × 44 = 66 g of CO₂
Similarly, 16 g of CH₄ will produce 36 g of water.
∴ 24 g of CH₄ = \(\frac {24}{16}\) × 36 = 54 g of water
Ans: Mass of carbon dioxide and water formed respectively are 66 g and 54 g.

Question 49.
How much CaO will be produced by decomposition of 5 g CaCO₃?
Solution:
Given: Mass of CaCO₃ consumed in reaction = 5 g
To find: Mass of CaO produced
Calculation: Calcium carbonate decomposes according to the balanced equation,

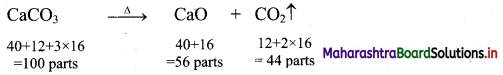
So, 100 g of CaCO₃ produce 56 g of CaO.

Ans: Mass of CaO produced = 2.8 g

Question 50.
How many litres of oxygen at STP are required to burn completely 2.2 g of propane, C₃H₈ ?
Solution:
Given: Mass of propane used up in reaction = 2.2 g
To find: Volume of oxygen required at STP
Calculation:
The balanced chemical equation for the combustion of propane is,

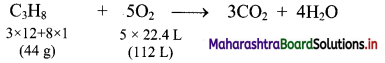
(∵ 1 mol of ideal gas occupies 22.4 L of volume at STP)
Thus, 44 g of propane require 112 litres of oxygen at STP for complete combustion.
∴ 2.2 g of propane will require
\(\frac {112}{44}\) × 2.2 = 5.6 litres of O₂ at STP for complete combustion.
Ans: Volume of O₂ (at STP) required to bum 2.2 g propane = 5.6 litres

Question 51.
A piece of zinc weighing 0.635 g when treated with excess of dilute H₂SO₄ liberated 200 cm³ hydrogen at STP. Calculate the percentage purity of the zinc sample.
Solution:
Given: Mass of zinc = 0.635 g, volume of H₂ liberated = 200 cm³
To find: % purity of zinc sample
Calculation: The relevant balanced chemical equation is,
Zn + H₂SO₄ → ZnSO₄ + H₂
It indicates that 22.4 L of hydrogen at STP = 65 g of Zn.
(where, atomic mass of Zn = 65 u)
∴ 0.200 L of hydrogen at STP
= \(\frac{65 \mathrm{~g}}{22.4 \mathrm{~L}}\) × 200 L
= 0.5803 g of Zn
∴ Percentage purity of Zn = \(\frac{0.5803}{0.635}\) × 100
= 91.37 % (by using log tables)
Ans: Percentage purity of Zn sample = 91.37%

[Calculation using log table:
\(\frac{65 \times 0.200}{22.4}\)
= Antilog₁₀ [log₁₀ (65) + log₁₀ (0.200) – log₁₀ (22.4)]
= Antilog₁₀ [1.8129 + \(\overline{1} .3010\) – 1.3502]
= Antilog₁₀ [latex]\overline{1} .7637[/latex] = 0.5803
\(\frac{0.5803}{0.635} \times 100=\frac{58.03}{0.635}\)
= Antilog₁₀ [log₁₀ (58.03) – log₁₀ (0.635)]
= Antilog₁₀ [1.7636 – \(\overline{1} .8028\)]
= Antilog₁₀ [1.9608] = 91.37]

Question 52.
Explain with the help of a chemical reaction how limiting reagent works.
Answer:

• Consider the formation of nitrogen dioxide (NO₂) from nitric oxide (NO) and oxygen.
  2NO_(g) + O_2(g) → 2NO_2(g)
• Suppose initially, we take 8 moles of NO and 7 moles of O₂.
• To determine the limiting reagent, calculate the number of moles NO₂ produced from the given initial quantities of NO and O₂. The limiting reagent will yield the smaller amount of the product.
• Starting with 8 moles of NO, the number of NO₂ produced is,
  8 mol NO × \(\frac{2 \mathrm{~mol} \mathrm{NO}_{2}}{2 \mathrm{~mol} \mathrm{NO}}\) = 8 mol NO₂
• Starting with 7 moles of O₂, the number of moles NO₂ produced is,
  7 mol O₂ × \(\frac{2 \mathrm{~mol} \mathrm{NO}_{2}}{1 \mathrm{~mol} \mathrm{NO}}\) = 14 mol NO₂
• Since, 8 moles NO result in a smaller amount of NO₂, NO is the limiting reagent, and O₂ is the excess reagent, before reaction has started.

Question 53.
Urea [(NH₂)₂CO] is prepared by reacting ammonia with carbon dioxide.
2NH_3(g) + CO_2(g) → (NH₂)₂CO_(aq) + H₂O_(l)
In one process, 637.2 g of NH₃ are treated with 1142 g of CO₂.
i. Which of the two reactants is the limiting reagent?
ii. Calculate the mass of (NH₂)₂CO formed.
iii. How much excess reagent (in grams) is left at the end of the reaction?
Solution:
i. If 637.2 g of NH₃ react completely, calculate the number of moles of (NH₂)₂CO, that could be produced, by the following relation.
Mass of NH₃ → Moles of NH₃ → Moles of (NH₂)₂CO

If 1142 g of CO₂ react completely, calculate the number of moles of (NH₂)₂CO, that could be produced, by the following relation.
Mass of CO₂ → Moles of CO₂ → Moles of (NH₂)₂CO

Since NH₃ produces smaller amount of (NH₂)₂CO, the limiting reagent is NH₃.

iii. Starting with 18.71 moles of (NH₂)₂CO, we can determine the mass of CO₂ that reacted using the mole ratio from the balanced equation and the molar mass of CO₂ by the following relation.
Moles of (NH₂)₂CO → Moles of CO₂ → Grams of CO₂

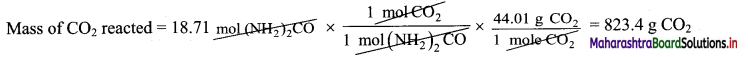
The amount of CO₂ remaining = 1142 g – 823.4 g = 318.6 g ≈ 319 g CO₂ remaining
Ans: i. Limiting reagent = NH₃
ii. Mass of (NH₂)₂CO produced = 1124 g
iii. Mass of CO₂ remaining = 319 g

Question 54.
6 g of H₂ reacts with 32 g of O₂ to yield water. Which is the limiting reactant? Find the mass of water produced and the amount of excess reagent left.
Solution:
i. The reaction is: 2H_2(g) + O_2(g) → 2H₂O_(l)
If 6 g of H₂ react completely, calculate the number of moles of H₂O, that could be produced, by the following relation.
Mass of H₂ → Moles of H₂ → Moles of H₂O

If 32 g of O₂ react completely, calculate the number of moles of H₂O, that could be produced, by the following relation.
Mass of O₂ → Moles of O₂ → Moles of H₂O

Since O₂ produces smaller amount of H₂O, the limiting reagent is O₂.

iii. Starting with 2 moles of H₂O, we can determine the mass of H₂ that reacted using the mole ratio from the balanced equation and the molar mass of H₂ by the following relation.
Moles of H₂O → Moles of H₂ → Grams of H₂

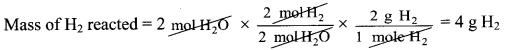
The amount of H₂ remaining = 6g – 4g = 2g H₂ remaining
Ans: i. Limiting reagent = O₂
ii. Mass of H₂O produced = 36 g
iii. Mass of H₂ remaining = 2 g

Question 55.
Name different ways to express the concentration of a solution (or the amount of substance in a given volume of solution).
Answer:

• Mass percent or weight percent (w/w %)
• Mole fraction
• Molarity (M)
• Molality (m)

Question 56.
State the formula to obtain mass percent.
Answer:
Mass percent (w/w %) = \(\frac{\text { Mass of Solute }}{\text { Massof solution }} \times 100\)

Question 57.
Why is molality NOT affected by temperature?
Answer:

• Molality is the number of moles of solute present in 1 kg of solvent. Therefore, molality is mass dependent.
• Mass remains unaffected with temperature.

Hence, molality is not affected by temperature.

Question 58.
State TRUE or FALSE. Correct the statement if false.
i. A majority of reactions in the laboratory are carried out in in gaseous forms.
ii. Molarity is the most widely used to express the concentration of solution.
iii. Molality of a solution changes with temperature.
Answer:
i. False
A majority of reactions in the laboratory are carried out in solution forms.
ii. Tme
iii. False
Molality of a solution does not change with temperature.

Question 59.
A solution is prepared by adding 2 g of a substance A to 18 g of water. Calculate the mass percent of the solute.
Solution:
Given: Mass of substance = 2 g, mass of water = 18 g
To find: Mass % of solute

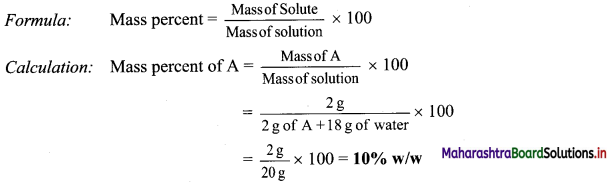
Ans: Mass percent of A = 10 % w/w

Question 60.
Calculate the molarity of NaOH in the solution prepared by dissolving its 4 g in enough water to form 250 mL of the solution.
Solution:
Given: Mass of solute (NaOH) = 4 g, volume of solution = 250 mL = 0.250 L
To find: Molarity of the solution

Ans: Molarity of the NaOH solution = 0.4 M

Question 61.
The density of 3 M solution of NaCl is 1.25 g mL^-1. Calculate molality of the solution.
Solution:
Given: Molarity of the solution = 3 M, density of the solution = 1.25 g mL^-1
To find: Molality of the solution
Formula:

Calculation: Molarity = 3 mol L^-1
∴ Mass of NaCl in 1 L solution = 3 × 58.5 = 175.5 g
Mass of 1 L solution = 1000 × 1.25 = 1250 g (∵ Density = 1.25 g mL^-1)
Mass of water in solution = 1250 – 175.5 = 1074.5 g = 1.0745 kg

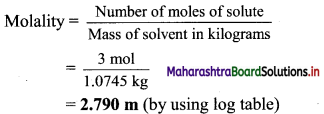
Ans: Molality of the NaCl solution = 2.790 m

[Calculation using log table:
\(\frac{3}{1.0745}\)
= Antilog₁₀ [log₁₀ (3) – log₁₀ (1.0745)]
= Antilog₁₀ [0.4771 – 0.0315]
= Antilog₁₀ [0.4456] = 2.790]

Question 62.
Calculate the molarity of 1.8 g HNO₃ dissolved in 250 mL aqueous solution.
Solution:
Mass of HNO₃ = 1.8 g,
volume of solution = 250 mL = 0.250 L
To find: Molarity
Formulae:

Calculation: Molar mass of HNO₃ = 63 g mol^-1
Using formula (i),

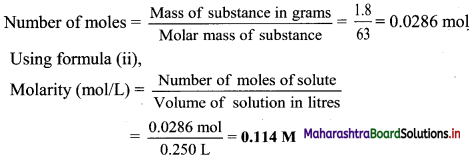
Ans: Molarity of the HNO₃ solution = 0.114 M

Question 63.
What volume in mL of a 0.1 M H₂SO₄ solution will contain 0.5 moles of H₂SO₄?
Solution:
Given: Molarity of H₂SO₄ solution = 0.1 M
Moles of H₂SO₄ = 0.5 mol
To find: Volume of H₂SO₄ solution

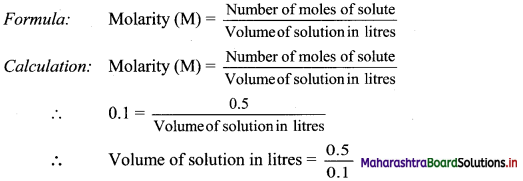
= 5 L
= 5000 L
Ans: Volume of a 0.1 M H₂SO₄ solution = 5000 mL

Question 64.
A mixture has 18 g water and 414 g ethanol. What are the mole fractions of water and ethanol?
Solution:
Given: Mass of water = 18 g, mass of ethanol = 414 g
To find: Mole fractions of water and ethanol
Formulae:


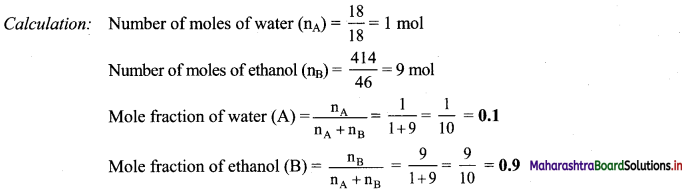
Ans: Mole fraction of water = 0.1
Mole fraction of ethanol = 0.9

Question 65.
Explain in brief: Use of graphs in analytical chemistry.
Answer:
i. Analytical chemistry often involves deducing some relation between two or more properties of matter under study.
ii. For example, the relation between temperature and volume of a given amount of gas.
iii. A set of experimentally measured values of volume and temperature of a definite mass of a gas upon plotting on a graph paper appears as in the figure below:

iv. When the points are directly connected, a zig zag pattern results as shown below:

From the above pattern, no meaningful result can be deduced.
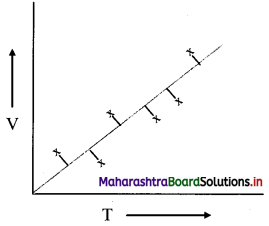
v. A smooth curve (or average curve) passing through these points can be drawn as shown below. This straight line is consistent with the V ∝ T .

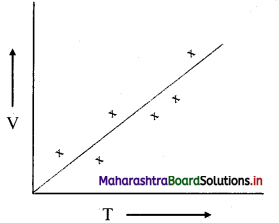
vi. While fitting the points into a smooth curve, all the plotted points should be evenly distributed. This can be verified mathematically, by drawing a perpendicular from each point to the curve. The perpendicular represents deviation of each point from the curve. Take sum of all the perpendiculars on side of the line and sum of all the perpendiculars on another side of the line separately. If the two sums are equal (or nearly equal), the curve drawn shows the experimental points in the best possible representation

Question 66.
1.5 g of an impure sample of sodium sulphate dissolved in water was treated with excess of barium chloride solution when 1.74 g of BaSO₄ was obtained as dry precipitate. Calculate the percentage purity of the sample.
Solution:

The chemical equation representing the reaction is:

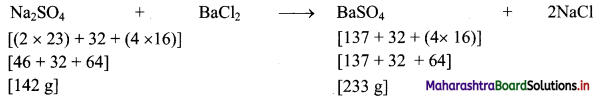
To calculate the mass of Na₂SO₄ from which 1.74 g of BaSO₄ is obtained:
233 g of BaSO₄ is produced from 142 g of Na₂SO₄.
∴ Mass of Na₂SO₄ from which 1.74 g of BaSO₄ would be obtained = \(\frac {142}{233}\) × 1.74 = 1.06 g
∴ The mass of pure Na₂SO₄ present in 1.5 g of impure sample = 1.06 g
To calculate the percentage purity of the impure sample:
1.5 g of impure sample contains 1.06 g of pure Na₂SO₄
∴ 100 g of the impure sample will contain = \(\frac{1.06}{1.5}\) × 100 = 70.67 g of pure Na₂SO₄
Ans: Percentage purity of the sample is 70.67 %.

Question 67.
Calculate the amount of lime Ca(OH)₂, required to remove hardness of 50,000 L of well water which has been found to contain 1.62 g of calcium bicarbonate per 10 L .
Solution:
Calculation of total Ca(HCO₃)₂ present:
10 L of water contains 1.62 g of Ca(HCO₃)
∴ 50,000 L of water will contain \(\frac{1.62}{10}\) × 50,000 = 8100 g of Ca(HCO₃)
Calculation of lime required:
The balanced equation for the reaction:

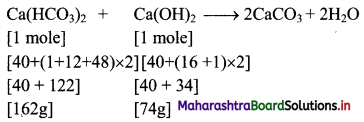
∴ 162 g of Ca(HCO₃) requires 74 g of lime.
Mass of lime required by 8100 g of Ca(HCO₃) = \(\frac {74}{162}\) × 8100 g = 3700 g = 3.7 kg
Ans: The amount of lime required to remove hardness of 50,000 L of well water, with 1.62 g of calcium bicarbonate per 10 L, is 3.7 kg.

Multiple Choice Questions

1. The number of significant figures in 0.0110 is …………….
(A) 2
(B) 3
(C) 4
(D) 5
Answer:
(B) 3

2. For the following measurements in which the true value is 4.0 g, find the CORRECT statement.

Student	Readings (g)
A	4.01	3.99
B	4.05	3.95

(A) Results of both the students are neither accurate nor precise.
(B) Results of student A are both precise and accurate.
(C) Results of student A are neither precise nor accurate.
(D) Results of student B are both precise and accurate.
Answer:
(B) Results of student A are both precise and accurate.

3. 18.238 is rounded off to four significant figures as ………….
(A) 18.20
(B) 18.23
(C) 18.2360
(D) 18.24
Answer:
(D) 18.24

4. The % of H₂O in Fe(CNS)₃.3H₂O is ……………..
(A) 34
(B) 11
(C) 19
(D) 46
Answer:
(C) 19

5. The molecular mass of an organic compound is 78 g mol^-1. Its empirical formula is CH. The molecular formula is ………….
(A) C₂H₄
(B) C₂H₂
(C) C₆H₆
(D) C₄H₄
Answer:
(C) C₆H₆

6. The percentage of oxygen in NaOH is ……………
(A) 40%
(B) 60%
(C) 8%
(D) 10%
Answer:
(A) 40%

7. 1.2 g of Mg (At. mass 24) will produce MgO equal to …………….
(A) 0.05 mol
(B) 0.03 mol
(C) 0.01 mol
(D) 0.02 mol
Answer:
(A) 0.05 mol

8. …………. reagent is the reactant that reacts completely but limits further progress of the reaction.
(A) Oxidizing
(B) Reducing
(C) Limiting
(D) Excess
Answer:
(C) Limiting

9. If a solution is made up of 1 mol ethanol and 9 mol water, then mole fraction of water in the solution is ……………
(A) 0.1
(B) 0.5
(C) 0.9
(D) 1.0
Answer:
(C) 0.9

10. 0.9 glucose (C₆H₁₂O₆) is present in 1 L of solution. Find molarity.
(A) 5 M
(B) 50 M
(C) 0.005 M
(D) 0.5 M
Answer:
(C) 0.005 M

11. Molality of a solution is the ……………
(A) number of moles of solute present in 1 kg of solvent
(B) number of moles of solute present in 1 L of solution
(C) mass of solute present in 1 kg of solvent
(D) number of moles of solute present in 1 kg of solution
Answer:
(A) number of moles of solute present in 1 kg of solvent