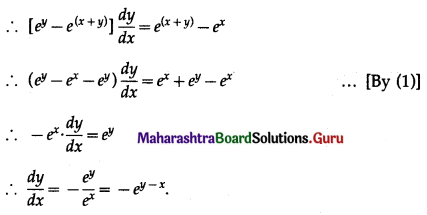Maharashtra Board 12th Commerce Maths Solutions Chapter 3 Differentiation Ex 3.4

Balbharati Maharashtra State Board 12th Commerce Maths Solution Book Pdf Chapter 3 Differentiation Ex 3.4 Questions and Answers.

Maharashtra State Board 12th Commerce Maths Solutions Chapter 3 Differentiation Ex 3.4

1. Find \(\frac{d y}{d x}\) if:

Question 1.
√x + √y = √a
Solution:
√x + √y = √a
Differentiating both sides w.r.t. x, we get

Question 2.
x³ + y³ + 4x³y = 0
Solution:
x³ + y³ + 4x³y = 0
Differentiating both sides w.r.t. x, we get

Question 3.
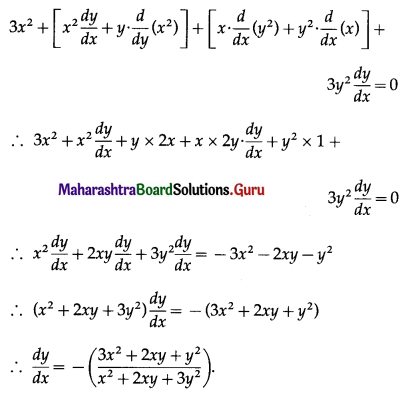
x³ + x²y + xy² + y³ = 81
Solution:
x³ + x²y + xy² + y³ = 81
Differentiating both sides w.r.t. x, we get

2. Find \(\frac{d y}{d x}\) if:

Question 1.
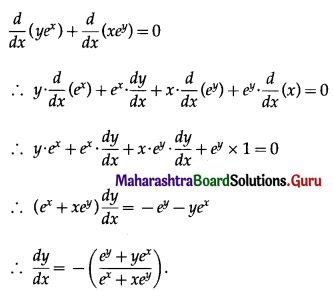
y.e^x + x.e^y = 1
Solution:
y.e^x + x.e^y = 1
Differentiating both sides w.r.t. x, we get

Question 2.
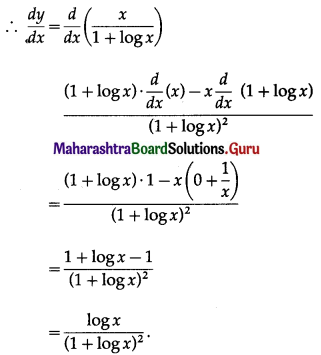
x^y = e^(x-y)
Solution:
x^y = e^(x-y)
∴ log x^y = log e^(x-y)
∴ y log x = (x – y) log e
∴ y log x = x – y …..[∵ log e = 1]
∴ y + y log x = x
∴ y(1 + log x) = x
∴ y = \(\frac{x}{1+\log x}\)

Question 3.
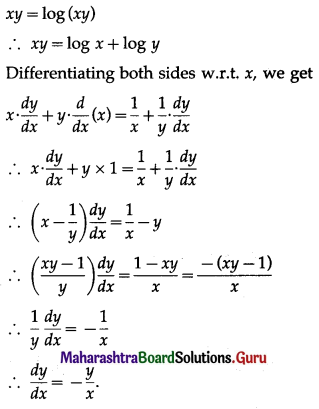
xy = log(xy)
Solution:
xy = log (xy)
∴ xy = log x + log y
Differentiating both sides w.r.t. x, we get

3. Solve the following:

Question 1.
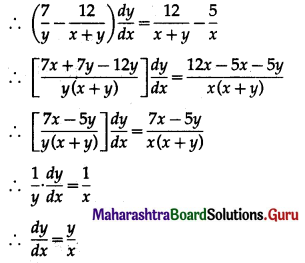
If x⁵ . y⁷ = (x + y)¹², then show that \(\frac{d y}{d x}=\frac{y}{x}\)
Solution:
x⁵ . y⁷ = (x + y)¹²
∴ log(x⁵ . y⁷) = log(x + y)¹²
∴ log x⁵ + log y⁷ = log(x + y)¹²
∴ 5 log x + 7 log y = 12 log (x + y)
Differentiating both sides w.r.t. x, we get

Question 2.
If log(x + y) = log(xy) + a, then show that \(\frac{d y}{d x}=\frac{-y^{2}}{x^{2}}\)
Solution:
log (x + y) = log (xy) + a
∴ log(x + y) = log x + log y + a
Differentiating both sides w.r.t. x, we get

Question 3.
If e^x + e^y = e^(x+y), then show that \(\frac{d y}{d x}=-e^{y-x}\).
Solution:
e^x + e^y = e^(x+y) ……….(1)
Differentiating both sides w.r.t. x, we get